Optical Textures and Orientational Structures in Cholesteric Droplets with Conical Boundary Conditions
Abstract
:1. Introduction
2. Results and Discussion
2.1. Twisted Axial-Bipolar Structure
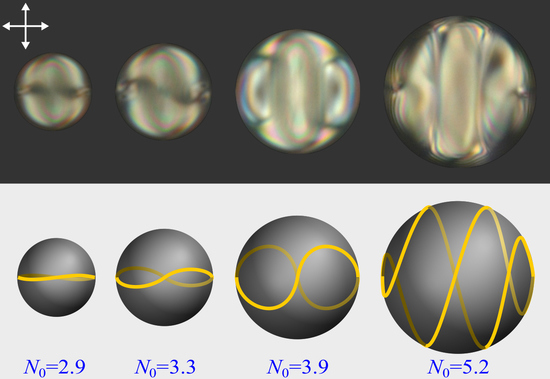
2.2. Layer-Like Structure
3. Materials and Methods
4. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Drzaic, P.S. Liquid Crystal Dispersions; World Scientific: Singapore, 1995. [Google Scholar]
- Urbanski, M.; Reyes, C.G.; Noh, J.; Sharma, A.; Geng, Y.; Subba Rao Jampani, V.; Lagerwall, J.P.F. Liquid crystals in micron-scale droplets, shells and fibers. J. Phys. Condens. Matter 2017, 29, 133003. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Lancia, F.; Yamamoto, T.; Ryabchun, A.; Yamaguchi, T.; Sano, M.; Katsonis, N. Reorientation behavior in the helical motility of light-responsive spiral droplets. Nat. Commun. 2019, 10, 5238. [Google Scholar] [CrossRef] [PubMed]
- Shvetsov, S.; Orlova, T.; Emelyanenko, A.V.; Zolot’ko, A. Thermo-optical generation of particle-like structures in frustrated chiral nematic film. Crystals 2019, 9, 574. [Google Scholar] [CrossRef] [Green Version]
- Lopez-Leon, T.; Fernandez-Nieves, A. Drops and shells of liquid crystal. Colloid Polym. Sci. 2011, 289, 345–359. [Google Scholar] [CrossRef]
- Kitzerow, H.S. Polymer-dispersed liquid crystals from the nematic curvilinear aligned phase to ferroelectric films. Liq. Cryst. 1994, 16, 1–31. [Google Scholar] [CrossRef]
- Darmon, A.; Benzaquen, M.; Čopar, S.; Dauchot, O.; Lopez-Leon, T. Topological defects in cholesteric liquid crystal shells. Soft Matter 2016, 12, 9280–9288. [Google Scholar] [CrossRef] [Green Version]
- Tran, L.; Lavrentovich, M.O.; Beller, D.A.; Li, N.; Stebe, K.J.; Kamien, R.D. Lassoing saddle splay and the geometrical control of topological defects. Proc. Natl. Acad. Sci. USA 2016, 113, 7106–7111. [Google Scholar] [CrossRef] [Green Version]
- Tran, L.; Kim, H.N.; Li, N.; Yang, S.; Stebe, K.J.; Kamien, R.D.; Haase, M.F. Shaping nanoparticle fingerprints at the interface of cholesteric droplets. Sci. Adv. 2018, 4, eaat8597. [Google Scholar] [CrossRef] [Green Version]
- Erdmann, J.H.; Žumer, S.; Doane, J.W. Configuration transition in a nematic liquid crystal confined to a small spherical cavity. Phys. Rev. Lett. 1990, 64, 1907–1910. [Google Scholar] [CrossRef]
- Rudyak, V.Y.; Emelyanenko, A.V.; Loiko, V.A. Structure transitions in oblate nematic droplets. Phys. Rev. E 2013, 88, 052501. [Google Scholar] [CrossRef]
- Rudyak, V.Y.; Krakhalev, M.N.; Prishchepa, O.O.; Sutormin, V.S.; Emelyanenko, A.V.; Zyryanov, V.Y. Orientational structures in nematic droplets with conical boundary conditions. JETP Lett. 2017, 106, 384–389. [Google Scholar] [CrossRef] [Green Version]
- Prishchepa, O.O.; Shabanov, A.V.; Zyryanov, V.Y. Director configurations in nematic droplets with inhomogeneous boundary conditions. Phys. Rev. E 2005, 72, 031712. [Google Scholar] [CrossRef] [PubMed]
- Zyryanov, V.Y.; Krakhalev, M.N.; Prishchepa, O.O.; Shabanov, A.V. Orientational structure transformations caused by the electric-field-induced ionic modification of the interface in nematic droplets. JETP Lett. 2007, 86, 383–388. [Google Scholar] [CrossRef]
- Zyryanov, V.Y.; Krakhalev, M.N.; Prishchepa, O.O.; Shabanov, A.V. Inverse regime of ionic modification of surface anchoring in nematic droplets. JETP Lett. 2008, 88, 597–601. [Google Scholar] [CrossRef]
- Gupta, J.K.; Abbott, N.L. Principles for manipulation of the lateral organization of aqueous-soluble surface-active molecules at the liquid crystal-aqueous interface. Langmuir 2009, 25, 2026–2033. [Google Scholar] [CrossRef] [Green Version]
- Krakhalev, M.N.; Sutormin, V.S.; Prishchepa, O.O.; Kuz’menok, N.M.; Mikhalyonok, S.G.; Bezborodov, V.S.; Zyryanov, V.Y. Anionic-cationic surfactant mixture providing the electrically controlled homeotropic surface anchoring of liquid crystals. J. Mol. Liq. 2019, 282, 57–62. [Google Scholar] [CrossRef] [Green Version]
- Doane, J.W.; Vaz, N.A.; Wu, B.; Žumer, S. Field controlled light scattering from nematic microdroplets. Appl. Phys. Lett. 1986, 48, 269–271. [Google Scholar] [CrossRef]
- Bouteiller, L.; Lebarny, P. Polymer-dispersed liquid crystals: Preparation, operation and application. Liq. Cryst. 1996, 21, 157–174. [Google Scholar] [CrossRef]
- Manna, U.; Zayas-Gonzalez, Y.M.; Carlton, R.J.; Caruso, F.; Abbott, N.L.; Lynn, D.M. Liquid crystal chemical sensors that cells can wear. Angew. Chem. Int. Ed. 2013, 52, 14011–14015. [Google Scholar] [CrossRef]
- Wang, Y.; Zhao, L.; Xu, A.; Wang, L.; Zhang, L.; Liu, S.; Liu, Y.; Li, H. Detecting enzymatic reactions in penicillinase via liquid crystal microdroplet-based pH sensor. Sens. Actuators B Chem. 2018, 258, 1090–1098. [Google Scholar] [CrossRef]
- Wang, Y.; Li, H.; Zhao, L.; Liu, Y.; Liu, S.; Yang, J. Tunable whispering gallery modes lasing in dye-doped cholesteric liquid crystal microdroplets. Appl. Phys. Lett. 2016, 109, 231906. [Google Scholar] [CrossRef]
- Humar, M. Liquid-crystal-droplet optical microcavities. Liq. Cryst. 2016, 43, 1937–1950. [Google Scholar] [CrossRef]
- Bloisi, F.; Ruocchio, C.; Terrecuso, P.; Vicari, L. Optoelectronic polarizer by PDLC. Liq. Cryst. 1996, 20, 377–379. [Google Scholar] [CrossRef]
- Krakhalev, M.N.; Prishchepa, O.O.; Sutormin, V.S.; Zyryanov, V.Y. Polymer dispersed nematic liquid crystal films with conical boundary conditions for electrically controllable polarizers. Opt. Mater. 2019, 89, 1–4. [Google Scholar] [CrossRef] [Green Version]
- Xu, F.; Crooker, P.P. Chiral nematic droplets with parallel surface anchoring. Phys. Rev. E 1997, 56, 6853–6860. [Google Scholar] [CrossRef]
- Zhou, Y.; Bukusoglu, E.; Martínez-González, J.A.; Rahimi, M.; Roberts, T.F.; Zhang, R.; Wang, X.; Abbott, N.L.; de Pablo, J.J. Structural transitions in cholesteric liquid crystal droplets. ACS Nano 2016, 10, 6484–6490. [Google Scholar] [CrossRef] [PubMed]
- Gardymova, A.P. Orientation structures of the chiral nematic droplets in a polymer matrix. Liq. Cryst. Their Appl. 2015, 15, 73–80. [Google Scholar]
- Seč, D.; Porenta, T.; Ravnik, M.; Žumer, S. Geometrical frustration of chiral ordering in cholesteric droplets. Soft Matter 2012, 8, 11982. [Google Scholar] [CrossRef]
- Prishchepa, O.O.; Zyryanov, V.Y.; Gardymova, A.P.; Shabanov, V.F. Optical textures and orientational structures of nematic and cholesteric droplets with heterogeneous boundary conditions. Mol. Cryst. Liq. Cryst. 2008, 489, 84/[410]–93/[419]. [Google Scholar] [CrossRef]
- Bezić, J.; Žumer, S. Structures of the cholesteric liquid crystal droplets with parallel surface anchoring. Liq. Cryst. 1992, 11, 593–619. [Google Scholar] [CrossRef]
- Kurik, M.V.; Lavrentovich, O.D. Negative-positive monopole transitions in cholesteric liquid crystals. JETP Lett. 1982, 35, 444–447. [Google Scholar]
- Bouligand, Y.; Livolant, F. The organization of cholesteric spherulites. J. Phys. 1984, 45, 1899–1923. [Google Scholar] [CrossRef]
- Yang, D.K.; Crooker, P.P. Field-induced textures of polymer-dispersed chiral liquid crystal microdroplets. Liq. Cryst. 1991, 9, 245–251. [Google Scholar] [CrossRef]
- Kitzerow, H.S.; Crooker, P. Electric field effects on the droplet structure in polymer dispersed cholesteric liquid crystals. Liq. Cryst. 1993, 13, 31–43. [Google Scholar] [CrossRef]
- Posnjak, G.; Čopar, S.; Muševič, I. Points, skyrmions and torons in chiral nematic droplets. Sci. Rep. 2016, 6, 26361. [Google Scholar] [CrossRef]
- Posnjak, G.; Čopar, S.; Muševič, I. Hidden topological constellations and polyvalent charges in chiral nematic droplets. Nat. Commun. 2017, 8, 14594. [Google Scholar] [CrossRef]
- Krakhalev, M.N.; Gardymova, A.P.; Prishchepa, O.O.; Rudyak, V.Y.; Emelyanenko, A.V.; Liu, J.H.; Zyryanov, V.Y. Bipolar configuration with twisted loop defect in chiral nematic droplets under homeotropic surface anchoring. Sci. Rep. 2017, 7. [Google Scholar] [CrossRef]
- Seč, D.; Čopar, S.; Žumer, S. Topological zoo of free-standing knots in confined chiral nematic fluids. Nat. Commun. 2014, 5, 3057. [Google Scholar] [CrossRef]
- Orlova, T.; Asshoff, S.J.; Yamaguchi, T.; Katsonis, N.; Brasselet, E. Creation and manipulation of topological states in chiral nematic microspheres. Nat. Commun. 2015, 6, 7603. [Google Scholar] [CrossRef] [Green Version]
- Krakhalev, M.N.; Rudyak, V.Y.; Gardymova, A.P.; Zyryanov, V.Y. Toroidal configuration of a cholesteric liquid crystal in droplets with homeotropic anchoring. JETP Lett. 2019, 109, 478–481. [Google Scholar] [CrossRef] [Green Version]
- Krakhalev, M.N.; Rudyak, V.Y.; Prishchepa, O.O.; Gardymova, A.P.; Emelyanenko, A.V.; Liu, J.H.; Zyryanov, V.Y. Orientational structures in cholesteric droplets with homeotropic surface anchoring. Soft Matter 2019, 15, 5554–5561. [Google Scholar] [CrossRef] [PubMed]
- Oswald, P. Lehmann rotation of cholesteric droplets subjected to a temperature gradient: Role of the concentration of chiral molecules. Eur. Phys. J. E 2009, 28, 377–383. [Google Scholar] [CrossRef] [PubMed]
- Ito, F.; Yoshioka, J.; Tabe, Y. Heat-driven rotation in cholesteric droplets with a double twisted structure. J. Phys. Soc. Jpn. 2016, 85, 114601. [Google Scholar] [CrossRef]
- Yoshioka, J.; Ito, F.; Tabe, Y. Stability of a double twisted structure in spherical cholesteric droplets. Soft Matter 2016, 12, 2400–2407. [Google Scholar] [CrossRef] [PubMed]
- Poy, G.; Bunel, F.; Oswald, P. Role of anchoring energy on the texture of cholesteric droplets: Finite-element simulations and experiments. Phys. Rev. E 2017, 96, 012705. [Google Scholar] [CrossRef] [Green Version]
- Krakhalev, M.N.; Prishchepa, O.O.; Sutormin, V.S.; Zyryanov, V.Y. Director configurations in nematic droplets with tilted surface anchoring. Liq. Cryst. 2017, 44, 355–363. [Google Scholar] [CrossRef] [Green Version]
- Rudyak, V.Y.; Krakhalev, M.N.; Sutormin, V.S.; Prishchepa, O.O.; Zyryanov, V.Y.; Liu, J.H.; Emelyanenko, A.V.; Khokhlov, A.R. Electrically induced structure transition in nematic liquid crystal droplets with conical boundary conditions. Phys. Rev. E 2017, 96, 052701. [Google Scholar] [CrossRef] [Green Version]
- Drzaic, P.S. A case of mistaken identity: Spontaneous formation of twisted bipolar droplets from achiral nematic materials. Liq. Cryst. 1999, 26, 623–627. [Google Scholar] [CrossRef]
- Krakhalev, M.N.; Gardymova, A.P.; Emel’yanenko, A.V.; Liu, J.H.; Zyryanov, V.Y. Untwisting of the helical structure of cholesteric droplets with homeotropic surface anchoring. JETP Lett. 2017, 105, 51–54. [Google Scholar] [CrossRef] [Green Version]
- Rofouie, P.; Pasini, D.; Rey, A.D. Morphology of elastic nematic liquid crystal membranes. Soft Matter 2017, 13, 5366–5380. [Google Scholar] [CrossRef]
- Krakhalev, M.N.; Bikbaev, R.G.; Sutormin, V.S.; Timofeev, I.V.; Zyryanov, V.Y. Nematic and cholesteric liquid crystal structures in cells with tangential-conical boundary conditions. Crystals 2019, 9, 249. [Google Scholar] [CrossRef] [Green Version]
- Belmonte, A.; Ussembayev, Y.Y.; Bus, T.; Nys, I.; Neyts, K.; Schenning, A.P.H.J. Dual Light and Temperature Responsive Micrometer-Sized Structural Color Actuators. Small 2020, 16, 1905219. [Google Scholar] [CrossRef] [PubMed] [Green Version]






| m | 19.2 | 24.7 | 30.1 | 36.8 | 43.3 |
| 2.0 | 3.0 | 4.0 | 5.0 | 6.0 | |
| 3.96 | 5.09 | 6.21 | 7.59 | 8.93 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gardymova, A.P.; Krakhalev, M.N.; Zyryanov, V.Y. Optical Textures and Orientational Structures in Cholesteric Droplets with Conical Boundary Conditions. Molecules 2020, 25, 1740. https://doi.org/10.3390/molecules25071740
Gardymova AP, Krakhalev MN, Zyryanov VY. Optical Textures and Orientational Structures in Cholesteric Droplets with Conical Boundary Conditions. Molecules. 2020; 25(7):1740. https://doi.org/10.3390/molecules25071740
Chicago/Turabian StyleGardymova, Anna P., Mikhail N. Krakhalev, and Victor Ya. Zyryanov. 2020. "Optical Textures and Orientational Structures in Cholesteric Droplets with Conical Boundary Conditions" Molecules 25, no. 7: 1740. https://doi.org/10.3390/molecules25071740
APA StyleGardymova, A. P., Krakhalev, M. N., & Zyryanov, V. Y. (2020). Optical Textures and Orientational Structures in Cholesteric Droplets with Conical Boundary Conditions. Molecules, 25(7), 1740. https://doi.org/10.3390/molecules25071740