Systematic Investigation of a Large Two-Stroke Engine Crankshaft Dynamics Model
Abstract
:1. Introduction
- The benefits in the implementation of the constant inertia-speed approach, which significantly simplifies the crankshaft dynamics model, have not been investigated for engines with significantly larger rotating and reciprocating masses such as the large two-stroke engines.
2. Materials and Methods
- Reference system selection: The reference system of a large two-stroke engine is selected for this investigation, and its operational and geometrical characteristics are described.
- Crankshaft dynamics model development: The model governing equations are derived considering both the variable and constant inertia-speed approaches for the reference system.
- Engine friction submodels: Three friction submodels are described and their integration into the model governing equations (Step 2) is explained.
- Numerical schemes: The implementation of the two most prominent numerical schemes for solving the set of differential equations of the reference system’s crankshaft dynamics model are described.
- Time step study: A time step study for the numerical schemes is performed where applicable, and the recommended time step is identified by using the convergence criterion and computational time.
- Identification of the KPIs to be used for the systematic comparison of the considered model alternatives.
- Systematic comparison of the investigated alternatives: The model results of all the possible combinations are compared based on the KPIs developed in step 6.
- Recommendations: The recommendations are discussed for the setup and usage of large two-stroke engines’ crankshaft dynamics models.
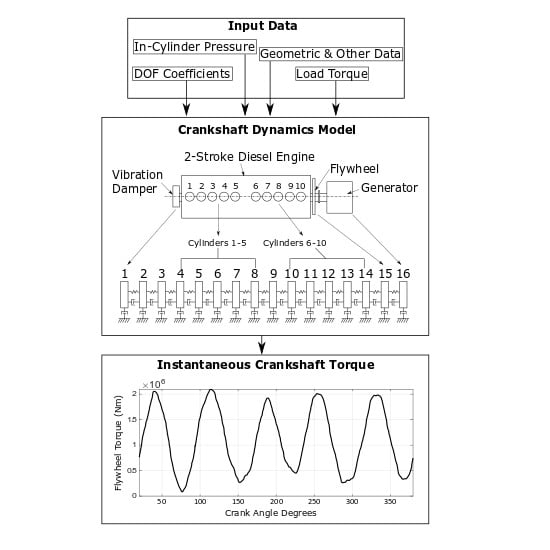
2.1. Reference System
2.2. Crankshaft Dynamics Model
2.2.1. Combustion Gas Torque
2.2.2. Mass Torque
2.2.3. Load Torque
2.2.4. Crankshaft Dynamics Governing Equation
2.3. Engine Friction Submodels
2.4. Numerical Schemes
2.4.1. ODE23tb Numerical Scheme
2.4.2. Piecewise Linear Time-Invariant Numerical Scheme
2.4.3. Convergence Criterion and Time Step Study
2.5. Key Performance Indicators for Systematic Comparison
2.6. Systematic Comparison Procedure
- Determination of the overall best performing crankshaft dynamics model by comparing case studies 1–12 via the PM.
- Comparison of selective case studies via the non-normalised KPIs:
- (a)
- Comparison of the engine friction submodels to determine the best performing submodel. This is performed by considering the crankshaft dynamics model with the variable inertia-speed approach for both numerical schemes; hence, the results of the case studies 2, 4, 6, 8, 10, and 12 (Table 2) are compared. For this comparison, the variable inertia-speed approach was used as it represents the real physical system more closely compared to the constant inertia-speed approach, which includes simplifications.
- (b)
- Comparison of the inertia-speed approaches considering the crankshaft dynamics model with the best performing engine friction submodel as determined in the previous step. This comparison is performed for both numerical schemes. Hence, the simulation results for the following case studies are compared:
- i
- If the constant engine friction submodel is the best performing: case studies 1, 2, 7, 8 are compared.
- ii
- If the coefficients matrix engine friction submodel is the best performing: case studies 3, 4, 9, 10 are compared.
- iii
- If the variable engine friction submodel is the best performing: case studies 5, 6, 11, 12 are compared.
- Comparison of the selected case studies listed below via the NRMSE, with the measured torque data or the reference case study (case study 12) where applicable, at the locations of cylinder 1, chain drive, and flywheel:
- (a)
- Numerical schemes comparison, by considering the most complex crankshaft dynamics model with the LTI numerical scheme (case study 6).
- (b)
- Engine friction submodel comparison, by considering the ODE23tb numerical scheme and the variable inertia-speed approach (case studies 8 and 10).
- (c)
- Inertia-speed approaches comparison, by considering the ODE23tb numerical scheme and the best performing engine friction submodel. Hence, the simulation results for the following case studies are compared:
- i
- If the constant engine friction submodel is the best performing: case studies 7 and 8 are compared.
- ii
- If the coefficients matrix engine friction submodel is the best performing: case studies 9 and 10 are compared.
- iii
- If the variable engine friction submodel is the best performing: case studies 11 and 12 are compared.
2.7. Crankshaft Dynamics Model Input Data and Assumptions
3. Results and Discussion
3.1. Crankshaft Dynamics Model Time Step Study
3.2. Crankshaft Dynamics Model Validation
3.3. Crankshaft Dynamics Model Performance Systematic Comparison
3.3.1. Performance Measure (PM)
3.3.2. Key Performance Indicators (KPIs)
3.3.3. Predicted Torque at Individual Crankshaft Locations
4. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Abbreviations
| DOF | Degree of Freedom |
| ICT | Instantaneous Crankshaft Torque |
| ICS | Instantaneous Crankshaft Speed |
| KPI | Key Performance Indicator |
| LTI | Linear Time-Invariant |
| MCR | Maximum Continuous Rating |
| NRMSE | Normalised Root Mean Squared Error |
| PM | Performance Measure |
| SS | Steady State |
Appendix A. Reference System Coefficients
| DOF | Stiffnes | Inertia | Rel. Damping | Abs. Damping |
|---|---|---|---|---|
| (kg m) | (Nms/rad) | (Nms/rad) | (Nms/rad) | |
| 1 | 1.00 | 8.54 | 5.70 | 0 |
| 2 | 1.00 | 4.48 | 0 | 0 |
| 3 | 1.34 | 4.42 | 0 | 0 |
| 4 | 1.20 | 6.52 | 5.00 | 2.29 |
| 5 | 1.20 | 6.89 | 5.00 | 2.29 |
| 6 | 1.20 | 6.52 | 5.00 | 2.29 |
| 7 | 1.21 | 6.62 | 5.00 | 2.29 |
| 8 | 1.30 | 6.89 | 5.00 | 2.29 |
| 9 | 1.41 | 3.81 | 5.00 | 2.29 |
| 10 | 1.21 | 6.89 | 5.00 | 2.29 |
| 11 | 1.20 | 6.89 | 5.00 | 2.29 |
| 12 | 1.20 | 6.52 | 5.00 | 2.29 |
| 13 | 1.20 | 6.90 | 5.00 | 2.29 |
| 14 | 8.15 | 6.52 | 0 | 2.29 |
| 15 | 6.93 | 3.06 | 0 | 3.29 |
| 16 | 5.94 | 8.54 | 1.45 | 2.69 |
Appendix B. Reference System Coefficient Matrices
Appendix C. Cylinder Selection Matrix
- The 4th DOF corresponds to the 1st cylinder, hence .
- The 5th DOF corresponds to the 2nd cylinder, hence .
- ⋮
- The DOF corresponds to the cylinder, hence .
Appendix D. Governing Equations of Crankshaft System
- and correspond to the variable and constant inertia-speed approach respectively.
- , with defined in Equation (4).
- , with p being the in-cylinder pressure as a function of crank angle.
- defined as the element-wise derivative of .
- defined as the element wise multiplication of the respective matrix with itself.
References
- Mattarelli, E.; Cantore, G.; Rinaldini, C.A. Advances in the Design of Two-Stroke, High Speed, Compression Ignition Engines; IntechOpen: London, UK, 2013. [Google Scholar]
- Okabe, M.; Sakaguchi, K.; Sugihara, M.; Miyanagi, A.; Hiraoka, N.; Murata, S. World’s largest marine 2-stroke diesel test engine, the 4UE-X3-development in compliance with the next version of environmental regulations and gas engine technology. Mitsubishi Heavy Ind. Tech. Rev. 2013, 50, 55. [Google Scholar]
- Reynaert, M. Abatement Strategies and the Cost of Environmental Regulation: Emission Standards on the European Car Market; CEPR Discussion Paper No. DP13756; SSRN: Rochester, NY, USA, 2019. [Google Scholar]
- Van, T.C.; Ramirez, J.; Rainey, T.; Ristovski, Z.; Brown, R.J. Global impacts of recent IMO regulations on marine fuel oil refining processes and ship emissions. Transp. Res. Part D Transp. Environ. 2019, 70, 123–134. [Google Scholar] [CrossRef]
- Stanton, D.W. Systematic development of highly efficient and clean engines to meet future commercial vehicle greenhouse gas regulations. SAE Int. J. Engines 2013, 6, 1395–1480. [Google Scholar] [CrossRef]
- Woodyard, D. Pounder’s Marine Diesel Engines and Gas Turbines, 9th ed.; Butterworth-Heinemann: Oxford, UK, 2009. [Google Scholar]
- Llamas, X.; Eriksson, L. Control-oriented modeling of two-stroke diesel engines with exhaust gas recirculation for marine applications. Proc. Inst. Mech. Eng. Part M J. Eng. Marit. Environ. 2019, 233, 551–574. [Google Scholar] [CrossRef] [Green Version]
- Theotokatos, G.; Guan, C.; Chen, H.; Lazakis, I. Development of an extended mean value engine model for predicting the marine two-stroke engine operation at varying settings. Energy 2018, 143, 533–545. [Google Scholar] [CrossRef] [Green Version]
- Romero, C.; Piedrahita, R.; Fabio, H.; Riaza, Q. Prediction of In-Cylinder Pressure, Temperature, and Loads Related to the Crank Slider Mechanism of I. C. Engines: A Computational Model; SAE Technical Paper; SAE International: Warrendale, PA, USA, 2003. [Google Scholar] [CrossRef]
- Guerrero, D.P.; Jiménez-Espadafor, F.J. Torsional system dynamics of low speed diesel engines based on instantaneous torque: Application to engine diagnosis. Mech. Syst. Signal Process. 2019, 116, 858–878. [Google Scholar] [CrossRef]
- Schagerberg, S.; Mckelvey, T. Instantaneous Crankshaft Torque Measurements—Modeling and Validation; SAE Technical Papers; SAE International: Warrendale, PA, USA, 2003. [Google Scholar] [CrossRef]
- Metallidis, P.; Natsiavas, S. Linear and nonlinear dynamics of reciprocating engines. Int. J. Non-Linear Mech. 2003, 38, 723–738. [Google Scholar] [CrossRef]
- Meng, J.; Liu, Y.; Liu, R. Finite element analysis of 4-cylinder diesel crankshaft. Int. J. Image Graph. Signal Process. 2011, 3, 22. [Google Scholar] [CrossRef]
- Yingkui, G.; Zhibo, Z. Strength analysis of diesel engine crankshaft based on PRO/E and ANSYS. In Proceedings of the 2011 Third International Conference on Measuring Technology and Mechatronics Automation, Shanghai, China, 6–7 January 2011; IEEE: Piscataway, NJ, USA, 2011; Volume 3, pp. 362–364. [Google Scholar]
- Fan, R.L.; Zhang, C.Y.; Yin, F.; Feng, C.C.; Ma, Z.D.; Gong, H.B. Finite element analysis for engine crankshaft torsional stiffness. Int. J. Simul. Process Model. 2019, 14, 389–396. [Google Scholar] [CrossRef]
- Armentani, E.; Caputo, F.; Esposito, L.; Giannella, V.; Citarella, R. Multibody simulation for the vibration analysis of a turbocharged diesel engine. Appl. Sci. 2018, 8, 1192. [Google Scholar] [CrossRef] [Green Version]
- Talikoti, B.S.; Kurbet, S.; Kuppast, V. A review on vibration analysis of crankshaft of internal combustion engine. Int. Res. J. Eng. Technol. (IRJET) 2015, 2. e-ISSN: 2395-0056. [Google Scholar]
- Mendes, A.; Meirelles, P.; Zampieri, D. Analysis of torsional vibration in internal combustion engines: Modelling and experimental validation. Proc. Inst. Mech. Eng. Part K J. Multi-Body Dyn. 2008, 222, 155–178. [Google Scholar] [CrossRef]
- Garg, R.; Baghla, S. Finite element analysis and optimization of crankshaft design. Int. J. Eng. Manag. Res. (IJEMR) 2012, 2, 26–31. [Google Scholar]
- Deshbhratar, R.; Suple, Y. Analysis and optimization of Crankshaft using FEM. Int. J. Mod. Eng. Res. 2012, 2, 3086–3088. [Google Scholar]
- De Silva, C.W. Vibration Damping, Control, and Design; CRC Press Taylor & Francis Group: Vancouver, BC, Canada, 2007. [Google Scholar]
- Fu, Z.F.; He, J. Modal Analysis; Elsevier: Amsterdam, The Netherlands, 2001. [Google Scholar]
- Thor, M.; Egardt, B.; McKelvey, T.; Andersson, I. Closed-loop diesel engine combustion phasing control based on crankshaft torque measurements. Control Eng. Pract. 2014, 33, 115–124. [Google Scholar] [CrossRef]
- Aulin, H.; Tunestal, P.; Johansson, T.; Johansson, B. Extracting cylinder individual combustion data from a high precision torque sensor. In Proceedings of the ASME 2010 Internal Combustion Engine Division Fall Technical Conference. American Society of Mechanical Engineers, San Antonio, TX, USA, 12–15 September 2010; pp. 619–625. [Google Scholar]
- Larsson, S.; Schagerberg, S. SI-Engine Cylinder Pressure Estimation using Torque Sensors; SAE Technical Paper; SAE International: Warrendale, PA, USA, 2004. [Google Scholar] [CrossRef]
- Kambrath, J.K.; Wang, Y.; Yoon, Y.J.; Alexander, A.A.; Liu, X.; Wilson, G.; Gajanayake, C.J.; Gupta, A.K. Modeling and control of marine diesel generator system with active protection. IEEE Trans. Transp. Electrif. 2017, 4, 249–271. [Google Scholar] [CrossRef]
- Espadafor, F.J.J.; Villanueva, J.A.B.; Guerrero, D.P.; García, M.T.; Trujillo, E.C.; Vacas, F.F. Measurement and analysis of instantaneous torque and angular velocity variations of a low speed two stroke diesel engine. Mech. Syst. Signal Process. 2014, 49, 135–153. [Google Scholar] [CrossRef]
- Desbazeille, M.; Randall, R.; Guillet, F.; El Badaoui, M.; Hoisnard, C. Model-based diagnosis of large diesel engines based on angular speed variations of the crankshaft. Mech. Syst. Signal Process. 2010, 24, 1529–1541. [Google Scholar] [CrossRef]
- Espadafor, F.; Guerrero, D.; Vacas, F.; Amo, M. Torsional System Modelling: Balancing and Diagnosis Application in Two-Stroke Low Speed Power Plant Diesel Engine. In Proceedings of the 28th CIMAC World Congress 2016, Helsinki, Finland, 6–10 June 2016. [Google Scholar]
- Kulah, S.; Forrai, A.; Rentmeester, F.; Donkers, T.; Willems, F. Robust cylinder pressure estimation in heavy-duty diesel engines. Int. J. Engine Res. 2018, 19, 179–188. [Google Scholar] [CrossRef] [Green Version]
- Liu, F.; Amaratunga, G.; Collings, N.; Soliman, A. An Experimental Study on Engine Dynamics Model Based In-Cylinder Pressure Estimation; SAE Technical Papers; SAE International: Warrendale, PA, USA, 2012. [Google Scholar] [CrossRef] [Green Version]
- Margaronis, I.E. The torsional vibrations of marine Diesel engines under fault operation of its cylinders. Forsch. Ingenieurwesen 1992, 58, 13–25. [Google Scholar] [CrossRef]
- Gawande, S.; Navale, L.; Nandgaonkar, M.; Butala, D. Detecting power imbalance in multi-cylinder inline diesel engine generator set. In Proceedings of the 2nd International Conference on Computer and Automation Engineering (ICCAE), Singapore, 26–28 February 2010; Volume 1, pp. 218–223. [Google Scholar]
- Filipi, Z.S.; Assanis, D.N. A nonlinear, transient, single-cylinder diesel engine simulation for predictions of instantaneous engine speed and torque. J. Eng. Gas Turbines Power 2001, 123, 951–959. [Google Scholar] [CrossRef]
- Zweiri, Y.; Whidborne, J.; Seneviratne, L. Dynamic simulation of a single-cylinder diesel engine including dynamometer modelling and friction. Proc. Inst. Mech. Eng. Part D J. Autom. Eng. 1999, 213, 391–402. [Google Scholar] [CrossRef]
- Zhang, X.; Guo, J.; Zhang, W. Dynamic Analysis of the Crank Train in a Single Cylinder Diesel Engine Using a Lumped Parameter Method. In Proceedings of the ASME 2016 Internal Combustion Engine Division Fall Technical Conference, Greenville, SC, USA, 9–12 October 2016. [Google Scholar]
- Rakopoulos, C.; Giakoumis, E.; Dimaratos, A. Evaluation of Various Dynamic Issues during Transient Operation of Turbocharged Diesel Engine with Special Reference to Friction Development; SAE Technical Papers; SAE International: Warrendale, PA, USA, 2007. [Google Scholar] [CrossRef] [Green Version]
- Milašinović, A.; Knežević, D.; Milovanović, Z.; Škundrić, J. Instantaneous Flywheel Torque of IC Engine Grey-Box Identification; IOP Conference Series: Materials Science and Engineering; IOP Publishing: Bristol, UK, 2018; Volume 294, p. 012080. [Google Scholar]
- Kiencke, U.; Nielsen, L. Automotive Control Systems: For Engine, Driveline, and Vehicle; Springer: New York, NY, USA, 2000. [Google Scholar]
- Franco, J.; Franchek, M.A.; Grigoriadis, K. Real-time brake torque estimation for internal combustion engines. Mech. Syst. Signal Process. 2008, 22, 338–361. [Google Scholar] [CrossRef]
- Giakoumis, E.; Dodoulas, I.; Rakopoulos, C. Instantaneous crankshaft torsional deformation during turbocharged diesel engine operation. Int. J. Veh. Des. 2010, 54, 217–237. [Google Scholar] [CrossRef] [Green Version]
- MAN Diesel & Turbo. Basic Principles of Ship Propulsion. Online. 2011. Available online: https://spain.mandieselturbo.com/docs/librariesprovider10/sistemas-propulsivos-marinos/basic-principles-of-ship-propulsion.pdf?sfvrsn=2 (accessed on 13 February 2020).
- Rezeka, S.F.; Henein, N.A. A New Approach to Evaluate Instantaneous Friction and Its Components in Internal Combustion Engines. SAE Trans. 1984, 93, 932–944. [Google Scholar]
- Thor, M.; Egardt, B.; McKelvey, T.; Andersson, I. Using combustion net torque for estimation of combustion properties from measurements of crankshaft torque. Control Eng. Pract. 2014, 26, 233–244. [Google Scholar] [CrossRef]
- Butcher, J.C. High order A-stable numerical methods for stiff problems. J. Sci. Comput. 2005, 25, 51–66. [Google Scholar] [CrossRef]
- MATLAB. Version 9.7.0.1319299 (R2019b); The MathWorks Inc.: Natick, MA, USA, 2019. [Google Scholar]
- Moler, C.B. Numerical Computing with MATLAB: Revised Reprint; SIAM: Philadelphia, PA, USA, 2008; Volume 87. [Google Scholar]
- Bank, R.E.; Coughran, W.M.; Fichtner, W.; Grosse, E.H.; Rose, D.J.; Smith, R.K. Transient simulation of silicon devices and circuits. IEEE Trans. Comput.-Aided Des. Integr. Circuits Syst. 1985, 4, 436–451. [Google Scholar] [CrossRef]
- Karnopp, D.C.; Margolis, D.L.; Rosenberg, R.C. System Dynamics: Modeling, Simulation, and Control of Mechatronic Systems; John Wiley & Sons: Hoboken, NJ, USA, 2012. [Google Scholar]
- Rowell, D. 2.14 Analysis and Design of Feedback Control Systems Time-Domain Solution of LTI State Equations. 2002. Available online: http://web.mit.edu/2.14/www/Handouts/StateSpaceResponse.pdf (accessed on 13 May 2020).
- Yang, W.Y.; Cao, W.; Chung, T.S.; Morris, J. Applied Numerical Methods Using MATLAB; John Wiley & Sons: Hoboken, NJ, USA, 2005. [Google Scholar]
- Yen, J.; Petzold, L.R. An efficient Newton-type iteration for the numerical solution of highly oscillatory constrained multibody dynamic systems. SIAM J. Sci. Comput. 1998, 19, 1513–1534. [Google Scholar] [CrossRef] [Green Version]
- Deokar, R.; Shimada, M.; Lin, C.; Tamma, K.K. On the treatment of high-frequency issues in numerical simulation for dynamic systems by model order reduction via the proper orthogonal decomposition. Comput. Methods Appl. Mech. Eng. 2017, 325, 139–154. [Google Scholar] [CrossRef]
- Nsiampa, N.; Ponthot, J.P.; Noels, L. Comparative study of numerical explicit schemes for impact problems. Int. J. Impact Eng. 2008, 35, 1688–1694. [Google Scholar] [CrossRef] [Green Version]











| KPI no. | Description | Calculation |
|---|---|---|
| KPI 1 | NRMSE between predicted and measured torque | Equation (30) |
| KPI 2 | Computational time | User defined |
| KPI 3 | Energy balance norm | Equations (34) and (35) |
| Case Study Description | Constant Inertia-Speed | Variable Inertia-Speed |
|---|---|---|
| Approach | Approach | |
| Constant Engine Friction with LTI Scheme | Case Study 1 | Case Study 2 |
| Coefficients Matrix Engine Friction with LTI Scheme | Case Study 3 | Case Study 4 |
| Variable Engine Friction with LTI Scheme | Case Study 5 | Case Study 6 |
| Constant Engine Friction with ODE23tb Scheme | Case Study 7 | Case Study 8 |
| Coefficients Matrix Engine Friction with ODE23tb scheme | Case Study 9 | Case Study 10 |
| Variable Engine Friction with ODE23tb Scheme | Case Study 11 | Case Study 12 * |
| Engine brake power at MCR | MW |
| Engine nominal speed | r/min |
| Reciprocating mass () | m |
| Piston bore (B) | m |
| Connecting rod length (l) | m |
| Stroke () | m |
| Firing Order | 1-9-4–6-3-10-2-7-5-8 |
| Convergence Criterion | LTI Time Step | ODE23tb Time Step |
|---|---|---|
| = 0.5% | Internally Derived |
| Convergence Criterion | LTI Time Step 1 * | LTI Time Step 2 ** | ODE23tb Time Step |
|---|---|---|---|
| Internally Derived |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Tsitsilonis, K.-M.; Theotokatos, G.; Xiros, N.; Habens, M. Systematic Investigation of a Large Two-Stroke Engine Crankshaft Dynamics Model. Energies 2020, 13, 2486. https://doi.org/10.3390/en13102486
Tsitsilonis K-M, Theotokatos G, Xiros N, Habens M. Systematic Investigation of a Large Two-Stroke Engine Crankshaft Dynamics Model. Energies. 2020; 13(10):2486. https://doi.org/10.3390/en13102486
Chicago/Turabian StyleTsitsilonis, Konstantinos-Marios, Gerasimos Theotokatos, Nikolaos Xiros, and Malcolm Habens. 2020. "Systematic Investigation of a Large Two-Stroke Engine Crankshaft Dynamics Model" Energies 13, no. 10: 2486. https://doi.org/10.3390/en13102486
APA StyleTsitsilonis, K. -M., Theotokatos, G., Xiros, N., & Habens, M. (2020). Systematic Investigation of a Large Two-Stroke Engine Crankshaft Dynamics Model. Energies, 13(10), 2486. https://doi.org/10.3390/en13102486