3.1. Morphology: Effect of Reaction Time and Surfactant
TEM and SEM observations of the synthesized oxide indicated significant differences in morphology depending on synthesis time (
Figure 2). The commercial ZnO reference was rod-shaped with sizes in the range of 0.5 to 1 µM in length (
Figure 2A). The TEM image of ZnO generated after 2 h of polarization at ~5 °C (sample labeled as 2H,
Figure 2B insert) and collected via filtration was composed of connected spheres of varying sizes (20–40 nm) arranged in a chaplet-like manner. Once deposited onto the FTO substrate and annealed, these structures formed porous clusters as shown in SEM (
Figure 2B). ZnO generated at room temperature over 3 h of polarization (sample named 3H) and collected via evaporation resulted in larger star-shaped structures of approximately 0.5 µm in length (
Figure 2D). As demonstrated in the supporting information (
Supplementary Figure S4), an increase in particle size was related to the agglomeration of the ZnO nanoparticles from the prolonged exposure to water during the evaporation process [
9], not to longer synthesis time. This was further supported by the TEM image of 3H collected via filtration carried out immediately after synthesis, revealing a mixture of previously seen spherical nanoparticle clusters as well as larger agglomerates (
Figure 2C). The microscopy led to the conclusions that exposure of ZnO to water can drastically affect the resulting structures, and ZnO synthesis in aqueous media must be carefully monitored. The structure evolution from the spherical nano-beads to the star-shaped larger structures was demonstrated by another group [
10]. The proposed mechanism indicated that upon the prolonged exposure to water, the structure of ZnO changed and an initial amount of water affected the nucleation process of ZnO significantly. As observed, an extended contact with water can impede the (0001) growth and accelerate the (1100) growth. This can be controlled by changing the water content via mixing it with methanol [
10]. In this way, the shape and size of ZnO can be tailored by adjusting the volume ratio. The optimized conditions resulting in uniform size and shape of nanomaterial (connected nano-spheres) were 2 h runs carried out at 5 °C (
Figure 2B).
Figure S5 demonstrates TEM images of these structures synthesized at room temperature (
Supplementary Figure S5A) and in an ice bath (~5 °C,
Supplementary Figure S5B). The smaller particles size of product fabricated at ~5 °C can be related to slower formation of the ionic species (kinetically controlled electrolysis). This was further confirmed by a smaller current recorded for low-temperature trials (
Supplementary Figure S6), and thus resulting in a slower rate of agglomeration of ZnO nanoparticles.
The use of surfactant (HMT and PVP) at various concentrations was also investigated and optimized for 2- and 3-h synthesis trials and collection method (filtration and evaporation). The generated current profiles were not significantly influenced by the addition of surfactants (
Supplementary Figure S6); therefore, they showed promise as an easy way to reduce the overall agglomeration without lowering the yield. It was later discovered that the effect of filtration surpassed the benefit gained from surfactant addition. As such, the use of surfactants, specifically HMT, although beneficial to an extent (
Supplementary Figure S7 A shows good particle separation in the presence of HMT for synthesis carried out for 2 h), was inhibited by an additional washing step (ZnO agglomerated as soon as the surfactant was removed). The PVP showed no significant effect of particle separation as compared to HMT (
Supplementary Figure S7).
3.2. Structure
X-Ray diffraction (XRD) allowed the identification of ZnO crystallographic structure as well as the estimation of crystalline size. The XRD patterns are shown in
Figure 3, and, for both the synthesized and commercial ZnO, the diffraction peaks indicated the wurtzite hexagonal phase (JCPDS 00-036-1451). The crystallite size (
D) was calculated using the Debye–Sherrer formula [
11].
where
D is the crystallite size in nm,
λ is the wavelength of X-ray radiation (0.0154178 nm),
θ is the Bragg angle in radians and
β is the full-width at half-maximum (FWHM), corresponding to the most intense peak in radians (summary of XRD analysis can be seen in
Table 1). The largest crystallite size was obtained for the commercial standard, followed by the 3H evaporated sample. The difference in particle size of 2H and 3H filtrated samples was very similar, since their morphologies were fairly similar. The XRD analysis of crystalline size followed a similar trend in ZnO particle size analyzed by TEM imaging (shown in the insert in
Figure 2). In comparison to ZnO synthesized by the bipolar electrochemical process by Allagui et al. [
5], there was a similarity in XRD patterns with respect to the 3H evaporated trials, with <011> as a predominant face. For the 2H and 3H filtered samples, the XRD analysis revealed the <101> face as the strongest pattern. In fact, XRD scan for ZnO synthesized for 3H and collected by evaporation was identical as in the referred work (star-shaped [
5]). This demonstrated the preferential growth of ZnO crystals along <011> plane upon prolonged exposure to water.
X-ray photoelectron spectroscopy (XPS) is further employed to analyze the chemical shifts that are summarized in
Table 1. All binding energies were corrected for charge shifting using the most intense peak of C 1s to have a true value of 284.8 eV [
12]. The binding energies of the Zn 2p signals for the synthesized samples were slightly shifted towards higher energies compared to the commercial (
Figure 4A). These shifts can be related to the difference in morphology and particle size between samples; however, relative shifts in the peak positions had been primarily associated with oxygen deficiencies in the lattice [
12,
13]. This was also related to the shift of O 1s signal. To further investigate any oxygen-dependent defects in the synthesized samples, the O 1s spectra were resolved by deconvolution into two peaks (
Figure 4C–F). The peak at the lower binding energy (P1) was related to the O
2− ions in the normal ZnO lattice, and the peak at higher energies (P2) to the O
2− ions in the oxygen-deficient regions within the ZnO (defective) structure [
12,
14]. The ratio between these two peaks had been shown to give an insight into the amount of oxygen defects [
15]. The ratio of P1 to P2 decreased when compared to commercial ZnO (Com), Com > 3He > 3Hf > 2H, indicating increasing levels of defects in the ZnO structure [
13].
3.3. Optical Analysis
Optical characterization was analyzed using UV–Vis DR spectrophotometry and used to measure bandgap and quantum confinement effects. The diffuse reflectance,
R, of the samples is related to the Kubelka–Munk function
F(R) by the relation
F(R) = (1−R)2/2R (
Figure 5A), where
R is the percentage reflectance [
16]. The bandgap energies of all samples were calculated from their diffuse reflectance spectra (
Figure 5B) by plotting the square of the Kubelka–Munk function
F(R)2 versus energy in electron volts (
Figure 5A). The linear part of the curve was extrapolated to
F(R)2 = 0 to get the direct bandgap energy. It is observed that the energy increased as the particle size decreased (
Figure 5A). The commercial ZnO particles were bigger with much larger particle-size distribution and demonstrated a lower bandgap when compared to 2H and 3Hf. As demonstrated by others, this phenomenon corresponded to a quantum effect [
17]. In particular, if the size of the particle became comparable with exciton radius (nanosize and quantum dots), optical properties of semiconductors changed significantly. The main reason mainly came from the carriers being confined in a very small space, which made the electrons and holes move only in a potential well. At the same time, it can improve the coupling between charges. Since the particle size of ZnO made using a bipolar electrochemical route were significantly larger than the exciton Bohr radius of bulk ZnO (2.34 nm [
18]), the confinement effect was moderate.
For the synthesized oxide, the absorption edge is shifted to a slightly shorter wavelength (blue-shift;
Figure 5B). The presence of blue-shifting can also indicate the defects in the ZnO lattice, resulting in the formation of shallow levels in the bandgap in this material [
15,
18]. This is consistent with an increase in bandgap energy for oxides synthesized in this work (
Figure 5A). These defects cause the off-white color of the synthesized ZnO (
Supplementary Figure S8). Specifically, yellow ZnO has been related to oxygen deficiencies in the ZnO lattice, resulting in a non-stoichiometric phase Zn
1+xO [
19].
Nevertheless, the bandgap of commercial and synthesized ZnO falls within the range reported in literature ~3.3 eV at room temperature. Advantages associated with a large bandgap include higher breakdown voltages, ability to sustain large electric fields, lower electronic noise, and high-temperature and high-power operation. This is further analyzed by electrochemical methods.
3.4. Electrochemistry
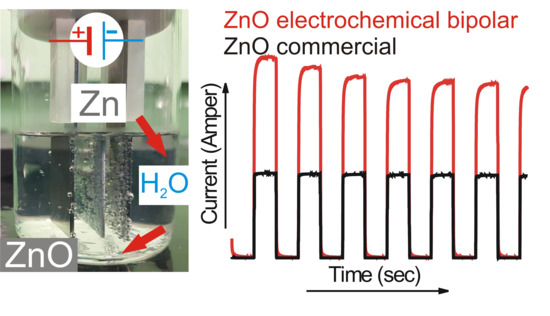
Single-potential amperometry and linear sweep voltammetry data were converted to I-time curves (
Figure 6A) and Tafel plots (
Figure 6B), respectively. Under illumination, the 2H sample resulted in the highest photocurrent followed by the 3Hf, 3He, and the lowest for commercial ZnO (
Figure 6A). The trend in measured photocurrent correlated well with the Tafel plots (
Figure 6B) and values of kinetic parameters, such as exchange current density (
Jo) and charge-transfer resistance (
Rct). The exchange current density can be estimated from the extrapolated intercepts of the anodic and cathodic branches and is related to the charge-transfer resistance according to [
20].
where
R is the gas constant,
Ta is the absolute temperature,
n is the number of electrons involved,
F is the Faraday constant, and
Rct is the charge-transfer resistance. As demonstrated in
Figure 6B, slopes of the anodic branches are in the order of 2H > 3Hf > 3He > Commercial. Correlating this with the above equation (Equation (12), with the J
o being inversely proportional to
Rct), it is concluded that the 2H samples resulted in lowest resistance, followed by 3Hf, 3He and Com. This analysis is in good agreement with the trend in photoresponse observed in
Figure 6A.
Concerning conductivity, stoichiometric ZnO is an insulator with very high resistivity, while the presence of different defects changes the concentration of charge carrier, resulting in the formation of a degenerate semiconductor. Reduced ZnO, which is highly deficient in oxygen, is thus expected to be more conductive as compared to commercial ZnO. This explains the higher current measured for ZnO made with the electrochemical bipolar process. This is further correlated with resistances of the ZnO-based electrodes analyzed by the impedance spectroscopy.
The theoretical Nyquist plots of an illuminated ZnO photoanode (
Figure 7A) shows two semicircles. A bigger one, in the frequency range of 1–10
3 Hz, is attributed to electron transfer at the ZnO/dye/electrolyte interface and within the ZnO film (
Rct); and to the electron ejection at the counter electrode/electrolyte interface. The transport in the electrolyte (transport resistance,
Rtr) is noted by the smaller semicircle in the frequency range of 10
3–10
5 Hz. The onset of the first semicircles at the real part of impedance are non-zero and are attributed to the charge transport through the FTO back contact together with solution resistance of the electrolyte (
Rs). The impedance measurements were conducted under dark and illuminated conditions, and the OCP potential was applied for each electrode based on
Figure 7A, to probe the recombination between the electrons at the conduction band (CB) or the electrons with the acceptor species in the electrolyte. The diameter of charge-transfer resistance for the illuminated electrode was several orders of magnitude lower as compared to the test in dark (
Figure 7C). This was further correlated with the Bode diagram (
Figure 7B), where the absolute value of total resistance of the system was plotted against the applied frequency. The illuminated system showed significantly lower total resistivity, which we ascribed to an increase in current generated during illumination.
Table 2 shows calculated values of the electrical elements proposed in
Figure 7, along with impedance analysis data from
Figure 7 and
Figure 8.
Rct decreased upon irradiation for all ZnO-based electrodes, because of the improved charge-transfer along the ZnO films. The higher charge-transfer resistance (
Rct) in Com and 3He samples as compared with 2H and 3Hf suggested a slower interfacial recombination, which can be attributed to a weaker interaction between the ZnO surface and the electrolyte solution. The porous structure in the 2H and 3Hf may facilitate penetration of sensitizer molecules into the structures. Consequently, the injected electrons and the oxidized ions in the electrolyte can be suppressed. Both the morphology and structural effect (oxygen vacancies) contribute to the observed resistivity. For example, as shown by SEM imaging, the electrode prepared from the 3Hf ZnO (
Figure 2C) was still relatively porous after annealing, although there were ZnO agglomerates which were bigger as compared to the 2H sample (
Figure 2B). The overall morphology of this electrode facilitated the efficient charge and electrolyte transport, which was manifested in lower
Rct as compared to 3He and Com samples. The SEM imaging for the latter two (
Figure 2A,D) showed significantly less porosity, which led to a reduced interaction between ZnO surface and the electrolyte solution. Yet, the oxygen-deficient ZnO was expected to be more conductive due to the increased mobility of charge carriers. This manifested in lower
Rct and
Rtr resistivity for all anodes made from ZnO synthesized in this work (
Table 2).
Another important parameter is the electron diffusion length (
Ln) analyzed from the impedance response [
21]. This parameter characterizes the maximum travelling distance of electrons before recombining with an acceptor species in the electrolyte. An efficient photoelectrode ideally reveals diffusion lengths, which are larger than the thickness of the photosensitive oxide layer, (
L). Notice, in order to examine the thickness of the active materials, an epoxy cross-section of the photoanodes were measured using SEM imaging as shown in the supporting information (
Supplementary Figure S9). All sample thicknesses were determined using ImageJ software and corrected to a true loading value of 1.0 mg per sample.
The electron diffusion length can be expressed as follows [
21].
where
Rct is the charge recombination resistance,
Rtr is the macroscopic transport resistance,
τn is the charge lifetime, and (
τd) is the charge extraction time. The diffusion length would exceed the thickness of the ZnO electrode if
Rtr <
Rct or
τd <
τn. It was apparent in the Nyquist plot that
Rct was larger than
Rtr indicating an efficient charge collection. The shortest
Ln was observed for 2H, followed by commercial sample and 3Hf, and the longest for 3He. This also confirmed that the porosity of the electrode had significant impact on the efficiency of charge transport and was correlated with lower
Rct for 2H ZnO, and thus better coverage of the electrode surface. This related to its high photocurrent generation as compared to other samples (
Figure 6A) and lower resistivity (
Figure 8).
Furthermore, the conduction band free-electron lifetimes (
τn) in the photoelectrodes can be evaluated from the peak frequency (
fpeak) at the minimum phase angle in the Bode plots (
Supplementary Figure S10 in supporting information) for illuminated anodes, using the correlation [
21]
The calculated electron lifetimes are summarized in
Table 2. Although the differences in charge lifetime were very small, it can be observed that the shortest lifetime of charge carriers, and thus relatively higher charge recombination rates, occurred for the ZnO anode made from the 2H sample.