Experimental Study of Lateral-Torsional Buckling of Class 4 Beams at Elevated Temperature
Abstract
:1. Introduction
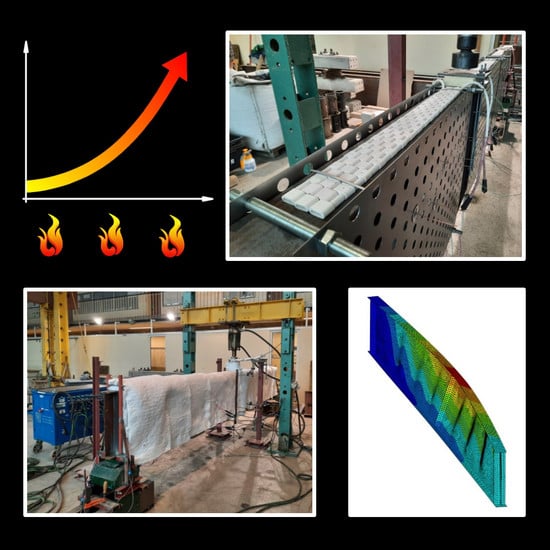
2. Experimental Setup
3. Results
3.1. Measurements of Geometrical Imperfections and Mechanical Properties of Steel
3.2. Results of Experiments at Room Temperature and Their Comparison with Computer Simulations
3.3. Results of Experimental Research at Fire Temperature and Their Comparison with Computer Simulations
4. Discussion
5. Conclusions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Appendix A



Appendix B
- C1, C2—coefficients depending on the shape of the bending moment.
- k, kw—effective length factors that depend on the support conditions.
- E—is the Young’s modulus.
- G—is the shear modulus.
- Iz—the second moment of area about the weak axis.
- IT—is the torsion constant.
- Iw—is the warping constant.
- L—is the length between laterally braced cross-sections of the beam.
- zg—is the distance between the point of load application and the shear centre.
References
- EN 1993-1-2. Eurocode 3 of: Design of Steel Structures—Part 1-2: General Rules—Structural Fire Design; European Committee for Standardization: Brussels, Belgium, 2005. [Google Scholar]
- Franssen, J.M.; Vila Real, P. Fire Design of Steel Structures, 2nd ed.; Wiley-VCH Verlag GmbH & Co. KGaA: Weinheim, Germany, 2016; ISBN 978-3-433-03143-8. [Google Scholar]
- Prachar, M.; Jandera, M.; Wald, F. Fire tests on beams with class 4 cross-section subjected to lateral torsional buckling. In Proceedings of the Application of Structural Fire Engineering, Prague, Czech Republic, 19–20 April 2013; pp. 19–20. [Google Scholar]
- Prachar, M.; Hricak, J.; Jandera, M.; Wald, F.; Zhao, B. Experiments of Class 4 open section beams at elevated temperature. Thin-Walled Struct. 2016, 98, 2–18. [Google Scholar] [CrossRef]
- Prachar, M.; Jandera, M.; Wald, F.; Zhao, B. Fire resistance of slender section beams. Steel Constr. 2014, 7, 188–192. [Google Scholar] [CrossRef]
- Prachar, M. Lateral-torsional Buckling of Class 4 Section Beams at Elevated Temperature. Ph.D. Dissertation, Czech Technical University, Prague, Czechia, 2017. [Google Scholar]
- Jandera, M.; Prachar, M.; Wald, F. Lateral-torsional buckling of class 4 section uniform and web tapered beams at elevated temperature. Thin-Walled Struct. 2020, 146. [Google Scholar] [CrossRef]
- Fire design of steel members with welded or hot-rolled class 4 cross-section (FIDESC4); Grant Agreement RFSR-CT-2011-00030; Publications Office: Luxembourg, 2014.
- Couto, C.; Vila Real, P.; Lopes, N.; Zhao, B. Effective width method to account for the local buckling of steel thin plates at elevated temperatures. Thin-Walled Struct. 2014, 84, 134–149. [Google Scholar] [CrossRef]
- Couto, C.; Vila Real, P.; Lopes, N.; Zhao, B. Resistance of steel cross-sections with local buckling at elevated temperatures. J. Constr. Steel Res. 2015, 109, 101–114. [Google Scholar] [CrossRef]
- Couto, C.; Vila Real, P.; Lopes, N.; Zhao, B. Numerical investigation of the lateral-torsional buckling of beams with slender cross sections for the case of fire. Eng. Struct. 2016, 106, 410–421. [Google Scholar] [CrossRef]
- Maia, É.; Couto, C.; Vila Real, P.; Lopes, N. Critical temperatures of class 4 cross-sections. J. Constr. Steel Res. 2016, 121, 370–382. [Google Scholar] [CrossRef]
- Vila Real, P.; Lopes, N.; Simões da Silva, L.; Franssen, J.M. Parametric analysis of the lateral-torsional buckling resistance of steel beams in case of fire. Fire Saf. J. 2007, 42, 416–424. [Google Scholar] [CrossRef]
- Rebelo, C.; Lopes, N.; Simões da Silva, L.; Nethercot, D.; Vila Real, P. Statistical evaluation of the lateral-torsional buckling resistance of steel I-beams, Part 1: Variability of the Eurocode 3 resistance model. J. Constr. Steel Res. 2009, 65, 818–831. [Google Scholar] [CrossRef]
- Simões da Silva, L.; Rebelo, C.; Nethercot, D.; Marques, L.; Simões, R.; Vila Real, P. Statistical evaluation of the lateral-torsional buckling resistance of steel I-beams, Part 2: Variability of steel properties. J. Constr. Steel Res. 2009, 65, 832–849. [Google Scholar] [CrossRef]
- Yin, Y.Z.; Wang, Y.C. Numerical simulations of the effects of non-uniform temperature distributions on lateral torsional buckling resistance of steel I-beams. J. Constr. Steel Res. 2003, 59, 1009–1033. [Google Scholar] [CrossRef]
- Zhang, C.; Gross, J.L.; McAllister, T.P. Lateral torsional buckling of steel W-beams subjected to localized fires. J. Constr. Steel Res. 2013, 88, 330–338. [Google Scholar] [CrossRef]
- Gardner, L.; Baddoo, N.R. Fire testing and design of stainless steel structures. J. Constr. Steel Res. 2006, 62, 532–543. [Google Scholar] [CrossRef]
- Franssen, J.M.; Gernay, T. Modeling structures in fire with SAFIR®: Theoretical background and capabilities. J. Struct. Fire Eng. 2017, 8, 300–323. [Google Scholar] [CrossRef]
- EN 1993-1-1. Eurocode 3: Design of Steel Structures—Part 1-1: General Rules and Rules for Buildings; European Committee for Standardization: Brussels, Belgium, 2005. [Google Scholar]
- EN ISO 6892-1. Metallic Materials—Tensile Testing—Part 1: Method of Test at Room Temperature; European Committee for Standardization: Brussels, Belgium, 2019. [Google Scholar]
- EN 1090-2. Execution of Steel Structures and Aluminium Structures—Part 2:Technical Requirements for Steel Structures; European Committee for Standardization: Brussels, Belgium, 2018. [Google Scholar]
- Bednarek, Z.; Kamocka, R. The heating rate impact on parameters characteristic of steel behaviour under fire conditions. J. Civ. Eng. Manag. 2006, 12, 269–275. [Google Scholar] [CrossRef]
- Franssen, J.M.; Talamona, D. New quadrangular shell element in SAFIR. In Proceedings of the First International Workshop “Structures in Fire”, Copenhagen, Denmark, 19–20 June 2000; pp. 195–210. [Google Scholar]
- Talamona, D.; Franssen, J.M. A quadrangular shell finite element for concrete and steel structures subjected to fire. J. Fire Prot. Eng. 2005, 15, 237–264. [Google Scholar] [CrossRef] [Green Version]
- Clark, J.; Hill, H. Lateral Buckling of Beams. J. Struct. Div. 1960, 86, 175–196. [Google Scholar] [CrossRef]
- Wang, W.; Qin, S. Experimental investigation of residual stresses in thin-walled welded H-sections after fire exposure. Thin-Walled Struct. 2016, 101, 109–119. [Google Scholar] [CrossRef]
- EN 1363-1. Fire Resistance Test—Part 1: General Requirements; European Committee for Standardization: Brussels, Belgium, 2012. [Google Scholar]
- Maslak, M.; Zwirski, G. Changes in structural steel microstructures following heating and cooling episodes in fires. Saf. Fire Technol. 2017, 48, 34–52. [Google Scholar] [CrossRef]
- Maslak, M.; Pazdanowski, M.; Stankiewicz, M.; Zajdel, P. Post-Fire Susceptibility to Brittle Fracture of Selected Steel Grades Used in Construction Industry—Assessment Based on the Instrumented Impact Test. Materials 2021, 14, 3922. [Google Scholar] [CrossRef]
- Woźniczka, P. Performance-Based Analysis of Older-Type Large-Space Hall in Fire. Arch. Civ. Eng. 2019, 65, 17–29. [Google Scholar] [CrossRef] [Green Version]
- Woźniczka, P. Fire resistance assessment of the long-span steel truss girder. Arch. Civ. Eng. 2020, 66, 63–75. [Google Scholar] [CrossRef]
- AxisVM X5 User’s Manual; Inter-Cad Kft.: Gusztáv, Hungary, 2020.





















| Plate Girder | Global Bow Imperfection Top Flange (mm) | Global Bow Imperfection Bottom Flange (mm) | Local Imperfection Web (mm) |
|---|---|---|---|
| B1 | +14.0 | +8.0 | ±8.5 |
| B2 | +1.0 | +2.0 | ±2.0 |
| B3 | +3.0 | +4.0 | ±2.0 |
| B4 | −1.0 | −12.0 | ±9.0 to ±11.0 |
| Plate Girder | Plate (mm) | Mean Value ReH 1 | Mean Value ReL 1 |
|---|---|---|---|
| B1, B4 | 4 mm | 304 (0.3%) 2 | 293 (0.5%) |
| 12 mm | 269 (2.5%) | 261 (1.8%) | |
| B2, B3 | 4 mm | 291 (2.9%) | 289 (1.1%) |
| 12 mm | 278 (3.4%) | 267 (2.8%) |
| Plate Girder | Plate [mm] | Mean Value |
|---|---|---|
| B1, B4 | 4 mm | 199.0 (2.8%) 1 |
| 12 mm | 189.6 (5.4%) | |
| B2, B3 | 4 mm | 187.4 (2.0%) |
| 12 mm | 182.0 (2.7%) |
| Plate Girder | Θcr.TEST (°C) | Θcr.SAFIR (°C) | ||
|---|---|---|---|---|
| Web | Flange | Web | Flange | |
| B1 | 558 | 434/516 1 | 574 | 450/533 1 |
| B2 | 553 | 472/535 1 | 604 | 524/577 1 |
| B3 | 605 | 520 | 613 | 518 |
| Plate Girder | Global Bow Imperfection Top Flange (mm) | Global Bow Imperfection Bottom Flange (mm) | Local Imperfection Web (mm) |
|---|---|---|---|
| B1 | +50.0 | +20.0 | ±4.0 |
| B2 | +45.0 | +3.0 | ±2.0 |
| B3 | +23.0 | −4.0 | ±2.0 |
| Plate Girder | Θcr.TEST 1 (°C) | MbRd.fi (kNm) | MbRd.fi.TEST (kNm) |
|---|---|---|---|
| B1 | 434.0 | 27.3 | 17.0 |
| B2 | 472.0 | 25.8 | 17.0 |
| B3 | 520.0 | 22.3 | 17.0 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Woźniczka, P. Experimental Study of Lateral-Torsional Buckling of Class 4 Beams at Elevated Temperature. Materials 2021, 14, 4825. https://doi.org/10.3390/ma14174825
Woźniczka P. Experimental Study of Lateral-Torsional Buckling of Class 4 Beams at Elevated Temperature. Materials. 2021; 14(17):4825. https://doi.org/10.3390/ma14174825
Chicago/Turabian StyleWoźniczka, Piotr. 2021. "Experimental Study of Lateral-Torsional Buckling of Class 4 Beams at Elevated Temperature" Materials 14, no. 17: 4825. https://doi.org/10.3390/ma14174825
APA StyleWoźniczka, P. (2021). Experimental Study of Lateral-Torsional Buckling of Class 4 Beams at Elevated Temperature. Materials, 14(17), 4825. https://doi.org/10.3390/ma14174825