Adsorption of Azo-Dye Orange II from Aqueous Solutions Using a Metal-Organic Framework Material: Iron- Benzenetricarboxylate
Abstract
:1. Introduction
| Adsorbent | T (K) | Q0 (mg·g−1) | Reference |
|---|---|---|---|
| Porous titania aerogel | 323 | 420 | [9] |
| Modified bentonite | 306 | 239.5 | [10] |
| Activated carbon (xerogels) | 323 | 499.1 | [11] |
| Activated carbon (fibers) | 283 | 438 | [12] |
| Activated carbon | 303 | 384.3 | [13] |
| Chitosan bead (cross-linked) | 303 | 1940 | [14] |
| Phosphoric acid-modified clam shell | 286 | 1017.1 | [15] |
| Amino-functionalized titanosilicate | 298 | 189.1 | [16] |
| Ammonia-tailored ordered mesoporous carbon | 298 | 596 | [17] |
| Carbon-alumina core-shell spheres | 298 | 210 | [18] |
| Nanoporous carbon from tomato waste | 323 | 312.5 | [19] |
| Canola stalks | 298 | 25.6 | [20] |
| Clay–alginate composites | 298 | 980.5 | [21] |
| Apricot shell activated carbon | 298 | 13.98 | [22] |

2. Results and Discussion
2.1. Materials Characterization


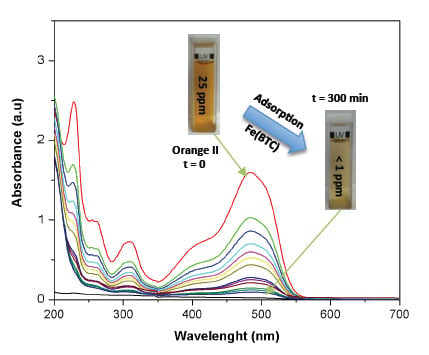
2.2. Effect of Adsorbent Concentration

2.3. Effect of Initial Concentration of Orange II Dye

2.4. Adsorption Isotherms
2.4.1. Langmuir Isotherm
| T (K) | Langmuir isotherm | Freundlich isotherm | D-R isotherm | Tempkin isotherm | |||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Q0 (mg·g−1) | b (L·mg−1) | RL | R2 | n | KF (mg·g−1) | R2 | E (kJ·mol−1) | QD-R (mg·g−1) | R2 | bT | KT (L·mg−1) | R2 | |
| 298 * | 85 | 0.3656 | 0.011–0.215 | 0.999 | 5.59 | 41.10 | 0.968 | 1.469 | 76.13 | 0.804 | 246.16 | 54.538 | 0.953 |
| 298 | 435 | 0.1643 | 0.024–0.378 | 0.998 | 3.06 | 95.61 | 0.914 | 1.469 | 336.46 | 0.899 | 39.06 | 5.596 | 0.985 |
| 308 | 417 | 0.1154 | 0.033–0.148 | 0.997 | 2.78 | 77.41 | 0.857 | 1.519 | 335.89 | 0.937 | 37.55 | 2.754 | 0.977 |
| 318 | 333 | 0.0860 | 0.044–0.538 | 0.999 | 2.57 | 51.05 | 0.840 | 1.568 | 265.79 | 0.937 | 44.50 | 1.456 | 0.971 |
2.4.2. Freundlich Isotherm
2.4.3. Dubinin–Radushkevich Isotherm
2.4.4. Tempkin Isotherm

2.5. Adsorption Kinetics

| C0 (mg·g−1) | qe,exp (mg·g−1) | Pseudo-first-order | Pseudo-second-order | ||||
|---|---|---|---|---|---|---|---|
| qe,cal (mg·g−1) | k1 (min−1) | R2 | qe,cal (mg·g−1) | k2 (g·mg·−1·min−1) | R2 | ||
| 10 | 43.98 | 10.67 | 1.24 × 10−2 | 0.611 | 44.64 | 4.14 × 10−3 | 0.999 |
| 25 | 115.49 | 45.91 | 1.31 × 10−2 | 0.820 | 117.09 | 1.46 × 10−3 | 0.999 |
| 50 | 212.49 | 91.94 | 1.08 × 10−2 | 0.750 | 220.26 | 5.64 × 10−4 | 0.999 |

2.6. Thermodynamic Analysis
| Temperature (K) | ln b (L·mol−1) | ΔG° (kJ·mol−1) | ΔH° (kJ·mol−1) | ΔS° (J·mol−1·K−1) |
|---|---|---|---|---|
| 298 | 10.96 | −27.16 | −25.53 | −5.40 |
| 308 | 10.60 | −27.16 | - | - |
| 318 | 10.31 | −27.26 | - | - |

2.7. Adsorption Mechanism

2.8. Regeneration of Adsorbent

3. Experimental Section
3.1. Synthesis of Fe(BTC) Material
3.2. Characterization
3.3. Adsorption Tests
4. Conclusions
Supplementary Materials
Acknowledgments
Conflicts of Interest
References
- Demirbas, A. Agricultural based activated carbons for the removal of dyes from aqueous solutions: A review. J. Hazard. Mater. 2009, 167, 1–9. [Google Scholar] [CrossRef] [PubMed]
- Tsuboy, M.S.; Angeli, J.P.F.; Mantovani, M.S.; Knasmüller, S.; Umbuzeiro, G.A.; Ribeiro, L.R. Genotoxic, mutagenic and cytotoxic effects of the commercial dye CI Disperse Blue 291 in the human hepatic cell line HepG2. Toxicol. in Vitro 2007, 21, 1650–1655. [Google Scholar] [CrossRef] [PubMed]
- Golka, K.; Kopps, S.; Myslak, Z.W. Carcinogenicity of azo colorants: Influence of solubility and bioavailability. Toxicol. Lett. 2004, 151, 203–210. [Google Scholar] [CrossRef] [PubMed]
- Chung, K.T. Mutagenicity and carcinogenicity of aromatic amines metabolically produced from azo dyes. Environ. Carcino. Ecotox. Revs. 2000, 18, 51–74. [Google Scholar] [CrossRef]
- Beaudoin, A.R.; Pickering, M.J. Teratogenic activity of several synthetic compounds structurally related to trypan blue. Anat. Record 1960, 137, 297–305. [Google Scholar] [CrossRef]
- Crini, G. Non-conventional low-cost adsorbents for dye removal: A review. Bioresour. Technol. 2006, 97, 1061–1085. [Google Scholar] [CrossRef] [PubMed]
- Kornaros, M.; Lyberatos, G. Biological treatment of wastewaters from a dye manufacturing company using a trickling filter. J. Hazard. Mater. 2006, 136, 95–102. [Google Scholar] [CrossRef] [PubMed]
- Hall, K.; Eagleton, L.; Acrivos, A.; Vermeulen, T. Pore-and solid-diffusion kinetics in fixed-bed adsorption under constant pattern conditions. Ind. Eng. Chem. Fundamen. 1966, 5, 212–223. [Google Scholar] [CrossRef]
- Abramian, L.; El-Rassy, H. Adsorption kinetics and thermodynamics of azo-dye Orange II onto highly porous titania aerogel. Chem. Eng. J. 2009, 150, 403–410. [Google Scholar] [CrossRef]
- Ma, J.; Qi, J.; Yao, C.; Cui, B.; Zhang, T.; Li, D. A novel bentonite-based adsorbent for anionic pollutant removal from water. Chem. Eng. J. 2012, 200, 97–103. [Google Scholar] [CrossRef]
- Ribeiro, R.S.; Fathy, N.A.; Attia, A.A.; Silva, A.M.T.; Faria, J.L.; Gomes, H.T. Activated carbon xerogels for the removal of the anionic azo dyes Orange II and Chromotrope 2R by adsorption and catalytic wet peroxide oxidation. Chem. Eng. J. 2012, 195, 112–121. [Google Scholar] [CrossRef]
- Hsiu-Mei, C.; Ting-Chien, C.; San-De, P.; Hung-Lung, C. Adsorption characteristics of Orange II and Chrysophenine on sludge adsorbent and activated carbon fibers. J. Hazard. Mater. 2009, 161, 1384–1390. [Google Scholar] [CrossRef]
- Rodríguez, A.; García, J.; Ovejero, G.; Mestanza, M. Adsorption of anionic and cationic dyes on activated carbon from aqueous solutions: Equilibrium and kinetics. J. Hazard. Mater. 2009, 172, 1311–1320. [Google Scholar] [CrossRef]
- Chiou, M.S.; Ho, P.Y.; Li, H.Y. Adsorption of anionic dyes in acid solutions using chemically cross-linked chitosan beads. Dyes Pigments 2004, 60, 69–84. [Google Scholar] [CrossRef]
- Ma, J.; Zou, J.; Cui, B.; Yao, C.; Li, D. Adsorption of Orange II dye from aqueous solutions using phosphoric-acid modified clam shell powder. Desalin. Water Treat. 2013, 51, 6536–6544. [Google Scholar] [CrossRef]
- Marçal, L.; de Faria, E.H.; Saltarelli, M.; Calefi, P.S.; Nassar, E.J.; Ciuffi, K.J. Amine-functionalized titanosilicates prepared by the sol-gel process as adsorbents of the azo-dye Orange II. Ind. Eng. Chem. Res. 2011, 50, 239–246. [Google Scholar] [CrossRef]
- He, C.; Hu, X. Anionic dye adsorption on chemically modified ordered mesoporous carbons. Ind. Eng. Chem. Res. 2011, 50, 14070–14083. [Google Scholar] [CrossRef]
- Zhou, J.; Tang, C.; Cheng, B.; Yu, J.; Jaroniec, M. Rattle-type carbon−alumina core−shell spheres: Synthesis and application for adsorption of organic dyes. ACS Appl. Mater. Interfaces 2012, 4, 2174–2179. [Google Scholar] [CrossRef]
- Güzel, F.; Saygli, H.; Saygli, G.A.; Koyuncu, F. Elimination of anionic dye by using nanoporous carbon prepared from an industrial biowaste. J. Mol. Liq. 2014, 194, 130–140. [Google Scholar]
- Hamzeh, Y.; Ashori, A.; Azadeh, E.; Abdulkhani, A. Removal of acid Orange 7 and remazol black 5 reactive dyes from aqueous solutions using a novel biosorbent. Mater. Sci. Eng. 2012, 32, 1394–1400. [Google Scholar] [CrossRef]
- Mandal, S.; Patil, V.S.; Mayadevi, S. Alginate and hydrotalcite-like anionic clay composite systems: Synthesis, characterization and application studies. Micropor. Mesopor. Mat. 2012, 158, 241–246. [Google Scholar] [CrossRef]
- Cao, J.; Wu, Y.; Jin, Y.; Yilihan, P.; Yang, S. Dynamic adsorption of anionic dyes by apricot shell activated carbon. Desalin. Water Treat. 2013, 1–9. [Google Scholar] [CrossRef]
- Haque, E.; Lee, J.E.; Jang, I.T.; Hwang, Y.K.; Chang, J.S.; Jegal, J.; Jhung, S.H. Adsorptive removal of methyl orange from aqueous solution with metal-organic frameworks, porous chromium-benzenedicarboxylates. J. Hazard. Mater. 2010, 181, 535–542. [Google Scholar] [CrossRef]
- Férey, G. Hybrid porous solids: Past, present, future. Chem. Soc. Rev. 2008, 37, 191–214. [Google Scholar] [CrossRef] [PubMed]
- Millward, A.R.; Yaghi, O.M. Metal-Organic Frameworks with exceptionally high capacity for storage of carbon dioxide at room temperature. J. Am. Chem. Soc. 2005, 127, 17998–17999. [Google Scholar] [CrossRef] [PubMed]
- Farrusseng, D.; Aguado, S.; Pinel, C. Metal–Organic Frameworks: Opportunities for catalysis. Angew. Chem. Int. Ed. 2009, 48, 7502–7513. [Google Scholar] [CrossRef]
- Li, S.L.; Xu, Q. Metal–organic frameworks as platforms for clean energy. Energy Environ. Sci. 2013, 6, 1656–1683. [Google Scholar] [CrossRef]
- Li, J.-R.; Sculley, J.; Zhou, H-C. Metal-Organic Frameworks for separations. Chem. Rev. 2012, 112, 869–932. [Google Scholar] [CrossRef] [PubMed]
- Haque, E.; Jun, J.W.; Jhung, S.H. Adsorptive removal of methyl orange and methylene blue from aqueous solution with a metal-organic framework material, iron terephthalate (MOF-235). J. Hazard. Mater. 2011, 185, 507–511. [Google Scholar] [CrossRef] [PubMed]
- Opanasenko, M.; Dhakshinamoorthy, A.; Cêjka, J.; Garcia, H. Deactivation pathways of the catalytic activity of metal-organic frameworks in condensation reactions. Chem. Cat. Chem. 2013, 5, 1553–1561. [Google Scholar]
- Zhu, B.J.; Yu, X.Y.; Jia, Y.; Peng, F.M.; Sun, B.; Zhang, M.Y.; Luo, T.; Liu, J.H.; Huang, X.J. Iron and 1,3,5-benzenetricarboxylic metal−organic coordination polymers prepared by solvothermal method and their application in efficient As(V) removal from aqueous solutions. J. Phys. Chem. C 2012, 116, 8601–8607. [Google Scholar] [CrossRef]
- Hosseini-Monfared, H.; Näther, C.; Winkler, H.; Janiak, C. Highly selective and “green” alcohol oxidations in water using aqueous 10% H2O2 and iron-benzenetricarboxylate metal–organic gel. Inorg. Chim. Acta 2012, 391, 75–82. [Google Scholar] [CrossRef]
- Shahid, S.; Nijmeijer, K. High pressure gas separation performance of mixed-matrix poly mermembranes containing mesoporous Fe(BTC). J. Membr. Sci. 2014, 459, 33–44. [Google Scholar] [CrossRef]
- Dhakshinamoorthy, A.; Alvaro, M.; Chevreau, H.; Horcajada, P.; Devic, T.; Serre, C.; Garcia, H. Iron(III) metal–organic frameworks as solid Lewis acids for the isomerization of α-pinene oxide. Catal. Sci. Technol. 2012, 2, 324–330. [Google Scholar] [CrossRef]
- Ploegmakers, J.; Japip, S.; Nijmeijer, K. Mixed matrix membranes containing MOFs for ethylene/ethane separation Part A: Membrane preparation and characterization. J. Membrane Sci. 2013, 428, 445–453. [Google Scholar] [CrossRef]
- Dhakshinamoorthy, A.; Alvaro, M.; Garcia, H. Aerobic oxidation of cycloalkenes catalyzed by iron metal organic framework containing N-hydroxyphthalimide. J. Catal. 2012, 289, 259–265. [Google Scholar] [CrossRef]
- Malekbala, M.R.; Hosseini, S.; Kazemi-Yazdi, S.; Masoudi Soltani, S.; Malekbala, M.R. On the utilization of a lignocellulosic waste as an excellent dye remover: Modification, characterization and mechanism analysis. Chem. Eng. J. 2013, 229, 257–266. [Google Scholar] [CrossRef]
- Wang, T.; Kailasam, K.; Xiao, P.; Chen, G.; Chen, L.; Wang, L.; Li, J.; Zhu, J. Adsorption removal of organic dyes on covalent triazine framework (CTF). Micropor. Mesopor. Mater. 2014, 187, 63–70. [Google Scholar] [CrossRef]
- Sarkar, B.; Xi, Y.; Megharaj, M.; Naidu, R. Orange II adsorption on palygorskites modified with alkyl trimethylammonium and dialkyl dimethylammonium bromide—An isothermal and kinetic study. Appl. Clay Sci. 2011, 51, 370–374. [Google Scholar] [CrossRef]
- Gil, A.; Assis, F.C.C.; Albeniz, S.; Korili, S.A. Removal of dyes from wastewaters by adsorption on pillared clays. Chem. Eng. J. 2011, 168, 1032–1040. [Google Scholar] [CrossRef]
- Langmuir, I. The constitution and fundamental properties of solids and liquids. Part I. Solids. J. Am. Chem. Soc. 1916, 38, 2221–2295. [Google Scholar] [CrossRef]
- Freundlich, H.M.F. Over the adsorption in solution. J. Phys. Chem. 1906, 57, 1100–1107. [Google Scholar]
- Dubinin, M.M.; Zaverina, E.D.; Radushkevich, L.V. Sorption and structure of active carbons. J. Phy. Chem. 1947, 2, 1351–1362. [Google Scholar]
- Tempkin, M.I.; Pyzhev, V. Kinetics of ammonia synthesis on promoted iron catalyst. J. Phys. Chem. USSR 1939, 13, 851–867. [Google Scholar]
- Günay, A.; Arslankaya, E.; Tosun, I. Lead removal from aqueous solution by natural and pretreated clinoptilolite: Adsorption equilibrium and kinetics. J. Hazard. Mater. 2007, 146, 362–371. [Google Scholar] [CrossRef] [PubMed]
- Chiou, M.S.; Li, H.Y. Equilibrium and kinetic modeling of adsorption of reactive dye on cross-linked chitosan beads. J. Hazard. Mater. 2002, 93, 233–248. [Google Scholar] [CrossRef] [PubMed]
- Dubinin, M.M. The potential theory of adsorption of gases and vapors for adsorbents with energetically non-uniform surface. Chem. Rev. 1960, 60, 235–241. [Google Scholar] [CrossRef]
- Kousha, M.; Daneshvar, E.; Salar-Sohrabi, M.; Jokar, M.; Bhatnagar, A. Adsorption of acid orange II dye by raw and chemically modified brown macroalga Stoechospermum marginatum. Chem. Eng. J. 2012, 192, 67–76. [Google Scholar] [CrossRef]
- Ho, Y.S.; McKay, G. Sorption of dye from aqueous solution by peat. Chem. Eng. J. 1998, 70, 115–124. [Google Scholar] [CrossRef]
- Silva, J.P.; Sousa, S.; Rodrigues, J.; Antunes, H.; Porter, J.J.; Gonçalves, I.; Ferreira-Dias, S. Adsorption of acid orange 7 dye in aqueous solutions by spent brewery grains. Sep. Purif. Technol. 2004, 40, 309–315. [Google Scholar] [CrossRef]
- Ho, Y.S.; McKay, G. Pseudo-second order model for sorption processes. Process Biochem. 1999, 34, 451–465. [Google Scholar] [CrossRef]
- Kara, M.; Yuzer, H.; Sabah, E.; Celik, M.S. Adsorption of cobalt from aqueous solutions onto sepiolite. Water Res. 2003, 37, 224–232. [Google Scholar] [CrossRef] [PubMed]
- Weber, W.J.; Morris, J.C. Kinetics of adsorption on carbon from solution. J. Sanit. Eng. Div. 1963, 89, 31–60. [Google Scholar]
- Petit, C.; Burress, J.; Bandosz, T.J. The synthesis and characterization of copper- based metal-organic framework/graphite oxide composites. Carbon 2011, 49, 563–572. [Google Scholar] [CrossRef]
© 2014 by the authors; licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
García, E.R.; Medina, R.L.; Lozano, M.M.; Hernández Pérez, I.; Valero, M.J.; Franco, A.M.M. Adsorption of Azo-Dye Orange II from Aqueous Solutions Using a Metal-Organic Framework Material: Iron- Benzenetricarboxylate. Materials 2014, 7, 8037-8057. https://doi.org/10.3390/ma7128037
García ER, Medina RL, Lozano MM, Hernández Pérez I, Valero MJ, Franco AMM. Adsorption of Azo-Dye Orange II from Aqueous Solutions Using a Metal-Organic Framework Material: Iron- Benzenetricarboxylate. Materials. 2014; 7(12):8037-8057. https://doi.org/10.3390/ma7128037
Chicago/Turabian StyleGarcía, Elizabeth Rojas, Ricardo López Medina, Marcos May Lozano, Isaías Hernández Pérez, Maria J. Valero, and Ana M. Maubert Franco. 2014. "Adsorption of Azo-Dye Orange II from Aqueous Solutions Using a Metal-Organic Framework Material: Iron- Benzenetricarboxylate" Materials 7, no. 12: 8037-8057. https://doi.org/10.3390/ma7128037
APA StyleGarcía, E. R., Medina, R. L., Lozano, M. M., Hernández Pérez, I., Valero, M. J., & Franco, A. M. M. (2014). Adsorption of Azo-Dye Orange II from Aqueous Solutions Using a Metal-Organic Framework Material: Iron- Benzenetricarboxylate. Materials, 7(12), 8037-8057. https://doi.org/10.3390/ma7128037