Thickness Measurement of Water Film/Rivulets Based on Grayscale Index
Abstract
:1. Introduction
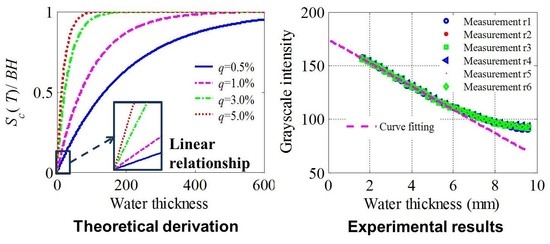
2. Theoretical Analysis
3. Experimental Validation
3.1. Experimental Setup
3.2. Data Processing Method
3.2.1. Image Processing
3.2.2. Identification of
3.3. Calibration Results
4. Measurement of the Profile of a Water Rivulet Flowing on a Cable Surface
5. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Zhang, K.; Wei, T.; Hu, H. An experimental investigation on the surface water transport process over an airfoil by using a digital image projection technique. Exp. Fluids 2015, 56, 173. [Google Scholar] [CrossRef]
- Flamand, O. Rain-wind induced vibration of cables. J. Wind Eng. Ind. Aerodyn. 1995, 57, 353–362. [Google Scholar] [CrossRef]
- Li, F.C.; Chen, W.L.; Li, H.; Zhang, R. An ultrasonic transmission thickness measurement system for study of water rivulets characteristics of stay cables suffering from wind–rain-induced vibration. Sens. Actuators A Phys. 2010, 159, 12–23. [Google Scholar] [CrossRef]
- Li, Y.; Jing, H.; Xia, Y.; Xu, Y.; Xiang, H. Measurement of rivulet movement on inclined cables during rain–wind induced vibration. Sens. Actuators A Phys. 2015, 231, 17–24. [Google Scholar] [CrossRef]
- Jing, H.; Xia, Y.; Xu, Y.; Li, Y. Measurement of rivulet movement and thickness on inclined cable using videogrammetry. Smart Struct. Syst. 2016, 18, 485–500. [Google Scholar] [CrossRef]
- Jing, H.; Xia, Y.; Li, H.; Xu, Y.; Li, Y. Excitation mechanism of rain–wind induced cable vibration in a wind tunnel. J. Fluids Struct. 2017, 68, 33–47. [Google Scholar] [CrossRef]
- Liu, J. Three-dimensional instabilities of film flows. Phys. Fluids 1995, 7, 55–67. [Google Scholar] [CrossRef]
- Johnson, M.F.G.; Schluter, R.A.; Bankoff, S.G. Fluorescent imaging system for global measurement of liquid film thickness and dynamic contact angle in free surface flows. Rev. Sci. Instrum. 1997, 68, 4097–4102. [Google Scholar] [CrossRef]
- Johnson, M.F.G.; Schluter, R.A.; Miksis, M.J.; Bankoff, S.G. Experimental study of rivulet formation on an inclined plate by fluorescent imaging. J. Fluid Mech. 1999, 394, 339–354. [Google Scholar] [CrossRef]
- He, X.H.; Yu, X.D.; Chen, Z.Q. Nonstationarity analysis in wind-rain-induced vibration of stay cables. J. Civ. Eng. Manag. 2012, 18, 821–827. [Google Scholar] [CrossRef]
- Cobelli, P.J.; Maurel, A.; Pagneux, V.; Petitjeans, P. Global measurement of water waves by Fourier transform profilometry. Exp. Fluids 2009, 46, 1037. [Google Scholar] [CrossRef]
- Hu, H.; Wang, B.; Zhang, K.; Lohry, W.; Zhang, S. Quantification of transient behavior of wind-driven surface droplet/rivulet flows using a digital fringe projection technique. J. Vis. 2014, 18, 1–14. [Google Scholar] [CrossRef]
- Salvi, J.; Fernandez, S.; Pribanic, T.; Llado, X. A state of the art in structured light patterns for surface profilometry. Pattern Recognit. 2010, 43, 2666–2680. [Google Scholar] [CrossRef]
- Zhang, Q.C.; Su, X.Y. An optical measurement of vortex shape at a free surface. Opt. Laser Technol. 2002, 34, 107–113. [Google Scholar] [CrossRef]
- Chen, W.L.; Tang, S.R.; Li, H.; Hu, H. Influence of dynamic properties and position of rivulet on rain–wind-induced vibration of stay cables. J. Bridge Eng. 2013, 18, 1021–1032. [Google Scholar] [CrossRef] [Green Version]
- Liu, Y.; Chen, W.L.; Bond, L.J.; Hu, H. An experimental study on the characteristics of wind-driven surface water film flows by using a multi-transducer ultrasonic pulse-echo technique. Phys. Fluids 2017, 30, 012102. [Google Scholar] [CrossRef]
- Benetazzo, A. Measurements of short water waves using stereo matched image sequences. Coast. Eng. 2006, 53, 1013–1033. [Google Scholar] [CrossRef]
- Benetazzo, A.; Barbariol, F.; Bergamasco, F.; Torsello, A.; Carniel, S.; Sclavo, M. Stereo wave imaging from moving vessels: Practical use and applications. Coast. Eng. 2016, 109, 114–127. [Google Scholar] [CrossRef]
- Wright, W.B.; Budakian, R.; Putterman, S.J. Diffusing light photography of fully developed isotropic ripple turbulence. Phys. Rev. Lett. 1996, 76, 4528–4532. [Google Scholar] [CrossRef]
- Zhang, X.; Dabiri, D.; Gharib, M. Optical mapping of fluid density interfaces: Concepts and implementations. Rev. Sci. Instrum. 1996, 67, 1858–1868. [Google Scholar] [CrossRef] [Green Version]
- Lel, V.V.; Al-Sibai, F.; Leefken, A.; Renz, U. Local thickness and wave velocity measurement of wavy films with a chromatic confocal imaging method and a fluorescence intensity technique. Exp. Fluids 2005, 39, 856–864. [Google Scholar] [CrossRef]
- Chinnov, E.A.; Kharlamov, S.M.; Saprykina, A.V.; Zhukovskaya, O.V. Measuring deformations of the heated liquid film by the fluorescence method. Thermophys. Aeromech. 2007, 14, 241–246. [Google Scholar] [CrossRef]
- Schagen, A.; Modigell, M. Local film thickness and temperature distribution measurement in wavy liquid films with a laser-induced luminescence technique. Exp. Fluids 2007, 43, 209–221. [Google Scholar] [CrossRef]
- Xue, T.; Lin, X.; Yang, L. Measurement of circumferential liquid film based on LIF and virtual stereo vision sensor. J. Sens. 2016, 2872947. [Google Scholar] [CrossRef] [Green Version]
- Cochard, S.; Ancey, C. Tracking the free surface of time-dependent flows: Image processing for the dam-break problem. Exp. Fluids 2008, 44, 59–71. [Google Scholar] [CrossRef]
- Tsubaki, R.; Fujita, I. Stereoscopic measurement of a fluctuating free surface with discontinuities. Meas. Sci. Technol. 2005, 16, 1894. [Google Scholar] [CrossRef]
- Ye, X.-W.; Dong, C.-Z.; Liu, T. Image-based structural dynamic displacement measurement using different multi-object tracking algorithms. Smart Struct. Syst. 2016, 17, 935–956. [Google Scholar] [CrossRef]
- Ye, X.-W.; Yi, T.-H.; Dong, C.-Z.; Liu, T. Vision-based structural displacement measurement: System performance evaluation and influence factor analysis. Measurement 2016, 88, 372–384. [Google Scholar] [CrossRef] [Green Version]
- Ye, X.-W.; Ni, Y.-Q.; Wai, T.-T.; Wong, K.-Y.; Zhang, X.-M.; Xu, F. A vision-based system for dynamic displacement measurement of long-span bridges: Algorithm and verification. Smart Struct. Syst. 2013, 12, 363–379. [Google Scholar] [CrossRef]
- Zhou, X.-Q.; Xia, Y.; Wei, Z.-L.; Wu, Q.-X. A videogrammetric technique for measuring the vibration displacement of stay cables. Geo Spat. Inf. Sci. 2012, 15, 135–141. [Google Scholar] [CrossRef] [Green Version]
- Ferraz, A.; Carvalho, V.; Soares, F.; Leão, C.P. Characterization of blood samples using image processing techniques. Sens. Actuators A Phys. 2011, 172, 318–324. [Google Scholar] [CrossRef]
- Qu, Y.; Huang, J.; Zhang, X. Rapid 3D Reconstruction for Image Sequence Acquired from UAV Camera. Sensors 2018, 18, 225. [Google Scholar]
- Li, H.; Yang, M. A nonlinear inverse perspective transform based method for camera distortion calibration. J. Shanghai Jiaotong Univ. 2008, 42, 1736–1739. [Google Scholar]
- Mao, J.X.; Wang, H.; Feng, D.M.; Tao, T.Y.; Zheng, W.Z. Investigation of dynamic properties of long-span cable-stayed bridges based on one-year monitoring data under normal operating condition. Struct Control Health Monit. 2018, e2146. [Google Scholar] [CrossRef]

 q = 0.5%,
q = 0.5%,  q = 1.0%,
q = 1.0%,  q = 3.0%,
q = 3.0%,  q = 5.0%).
q = 5.0%).
 q = 0.5%,
q = 0.5%,  q = 1.0%,
q = 1.0%,  q = 3.0%,
q = 3.0%,  q = 5.0%).
q = 5.0%).











© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Jing, H.; Cheng, Y.; He, X.; Zhou, X.; He, J. Thickness Measurement of Water Film/Rivulets Based on Grayscale Index. Remote Sens. 2019, 11, 2871. https://doi.org/10.3390/rs11232871
Jing H, Cheng Y, He X, Zhou X, He J. Thickness Measurement of Water Film/Rivulets Based on Grayscale Index. Remote Sensing. 2019; 11(23):2871. https://doi.org/10.3390/rs11232871
Chicago/Turabian StyleJing, Haiquan, Yi Cheng, Xuhui He, Xu Zhou, and Jia He. 2019. "Thickness Measurement of Water Film/Rivulets Based on Grayscale Index" Remote Sensing 11, no. 23: 2871. https://doi.org/10.3390/rs11232871
APA StyleJing, H., Cheng, Y., He, X., Zhou, X., & He, J. (2019). Thickness Measurement of Water Film/Rivulets Based on Grayscale Index. Remote Sensing, 11(23), 2871. https://doi.org/10.3390/rs11232871