Dynamic Tensile Properties of CFRP Manufactured by PCM and WCM: Effect of Strain Rate and Configurations
Abstract
:1. Introduction
2. Materials and Methods
3. Results
3.1. (0)s Configuration
3.2. (90)s Configuration
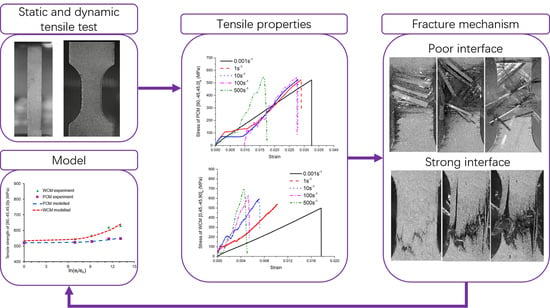
3.3. Typical Configurations
4. Discussion
4.1. Fracture Mechanism of Different Configurations
4.2. Strain Rate Sensitivity of Different Configurations
5. Conclusions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Hinrichsen, J.; Bautista, C. The challenge of reducing both airframe weight and manufacturing cost. Air Space Eur. 2001, 3, 119–121. [Google Scholar] [CrossRef]
- Wang, J.; Zhao, X. Lightweight research of carbon fiber/epoxy battery box. Fiber Reinf. Plast. /Compos. 2016, 12, 99–102. [Google Scholar] [CrossRef]
- Zhang, C.; Zhu, P.; Feng, Q.; He, J.; Zhou, Q. Optimization study of carbon fiber reinforced plastic vehicle fender. Automot. Eng. 2015, 5, 367–374. [Google Scholar] [CrossRef]
- Jacob, G.C.; Starbuck, J.M.; Fellers, J.F.; Simunovic, S.; Boeman, R.G. Strain rate effects on the mechanical properties of polymer composite materials. J. Appl. Polym. Sci. 2004, 94, 296–301. [Google Scholar] [CrossRef]
- Ma, Z.; Li, W.; Yang, S.; Huang, B.; Tong, S.; Li, C.; Zhao, H.; Ren, L. Strain rate-dependent deformation behaviors of multi-layer carbon fiber reinforced polymer laminates. J. Appl. Polym. Sci. 2021, 138, 50910. [Google Scholar] [CrossRef]
- Zhang, X.; Hao, H.; Shi, Y.; Cui, J.; Zhang, X. Static and dynamic material properties of CFRP/epoxy laminates. Constr. Build. Mater. 2016, 114, 638–649. [Google Scholar] [CrossRef] [Green Version]
- Okoli, O.I. The effects of strain rate and failure modes on the failure energy of fibre reinforced composites. Compos. Struct. 2001, 54, 299–303. [Google Scholar] [CrossRef]
- Duan, S.; Mo, F.; Yang, X.; Tao, Y.; Wu, D.; Peng, Y. Experimental and numerical investigations of strain rate effects on mechanical properties of LGFRP composite. Compos. Part B: Eng. 2016, 88, 101–107. [Google Scholar] [CrossRef]
- Kimura, H.; Itabashi, M.; Kawata, K. Mechanical characterization of unidirectional CFRP thin strip and CFRP cables under quasi-static and dynamic tension. Adv. Compos. Mater. 2001, 10, 177–187. [Google Scholar] [CrossRef]
- Naresh, K.; Shankar, K.; Rao, B.; Velmurugan, R. Effect of high strain rate on glass/carbon/hybrid fiber reinforced epoxy laminated composites. Compos. Part B: Eng. 2016, 100, 125–135. [Google Scholar] [CrossRef]
- Gilat, A.; Goldberg, R.K.; Roberts, G.D. Experimental study of strain-rate-dependent behavior of carbon/epoxy composite. Compos. Sci. Technol. 2002, 62, 1469–1476. [Google Scholar] [CrossRef]
- Wang, Z.; Zhao, G.; Ma, J.; Zhang, J. Experiment study on the strain rate behavior of carbon/epoxy composite materials. Acta Mater. Compos. Sin. 2007, 24, 113–119. [Google Scholar]
- Kwon, J.; Choi, J.; Huh, H.; Lee, J. Evaluation of the effect of the strain rate on the tensile properties of carbon–epoxy composite laminates. J. Compos. Mater. 2016, 51, 3197–3210. [Google Scholar] [CrossRef]
- Taniguchi, N.; Nishiwaki, T.; Kawada, H. Tensile strength of unidirectional CFRP laminate under high strain rate. Adv. Compos. Mater. 2007, 16, 167–180. [Google Scholar] [CrossRef]
- Oya, N.; Hamada, H. Mechanical properties and failure mechanisms of carbon fibre reinforced thermoplastic laminates. Compos. Part A Appl. Sci. Manuf. 1997, 28, 823–832. [Google Scholar] [CrossRef]
- Azadi, M.; Sayar, H.; Ghasemi-Ghalebahman, A.; Jafari, S.M. Tensile loading rate effect on mechanical properties and failure mechanisms in open-hole carbon fiber reinforced polymer composites by acoustic emission approach. Compos. Part B Eng. 2019, 158, 448–458. [Google Scholar] [CrossRef]
- Maekawa, Z.-I.; Hamada, H.; Kitagawa, T.; Lee, K. The difference of Fracture Behaviour between T300 and T800 Quasi-Isotropic Composites. Adv. Compos. Lett. 1992, 1. [Google Scholar] [CrossRef]
- Naresh, K.; Shankar, K.; Velmurugan, R. Reliability analysis of tensile strengths using Weibull distribution in glass/epoxy and carbon/epoxy composites. Compos. Part B Eng. 2018, 133, 129–144. [Google Scholar] [CrossRef]
- Wu, W.; Liu, Q.; Wu, L. Mechanical Properties of Carbon Fiber Reinforced Plastic Structures with Different Forming Process. Fiber Reinf. Plast./Compos. 2017, 10, 23–28. [Google Scholar] [CrossRef]
- Rao, P.S.; Hardiman, M.; O’Dowd, N.P.; Sebaey, T.A. Comparison of progressive damage between thermoset and thermoplastic CFRP composites under in-situ tensile loading. J. Compos. Mater. 2020, 55, 1473–1484. [Google Scholar] [CrossRef]
- Johnson, W.; Masters, J.; Subramanian, S.; Reifsnider, K.; Stinchcomb, W. Tensile Strength of Unidirectional Composites: The Role of Efficiency and Strength of Fiber-Matrix Interface. J. Compos. Technol. Res. 1995, 17, 289. [Google Scholar] [CrossRef]
- Zhou, Y.; Wang, Y.; Xia, Y.; Jeelani, S. Tensile behavior of carbon fiber bundles at different strain rates. Mater. Lett. 2010, 64, 246–248. [Google Scholar] [CrossRef]
- Sadeghian, P.; Rahai, A.R.; Ehsani, M.R. Effect of Fiber Orientation on Nonlinear Behavior of CFRP Composites. J. Reinf. Plast. Compos. 2008, 28, 2261–2272. [Google Scholar] [CrossRef]
- Yen, C.F. Ballistic impact modelling of composite materials. In Proceedings of the 7th internal LS-DYNA user conference, Dearborn, MI, USA, 19 May 2002; pp. 15–26. [Google Scholar]










| Tensile Test Conditions | (0)s | (90)s | [0/45/−45/90]s | [90/−45/45/0]s | ||
|---|---|---|---|---|---|---|
| Quasi-static tensile | PCM | 0.001 s−1 | √ | √ | √ | √ |
| WCM | ||||||
| Dynamic tensile | PCM | 1 s−1 | √ | √ | √ | √ |
| WCM | ||||||
| PCM | 10 s−1 | √ | √ | √ | √ | |
| WCM | ||||||
| PCM | 100 s−1 | √ | √ | √ | √ | |
| WCM | ||||||
| PCM | 500 s−1 | √ | √ | √ | √ | |
| WCM | ||||||
| Configurations | (0)s | (90)s | [0/45/−45/90]s | [90/−45/45/0]s | ||
|---|---|---|---|---|---|---|
| Tensile strength | PCM | a | 0.097 | 0.0239 | 0.0000417 | 0.00000479 |
| b | 0.62 | 1.41 | 3.15 | 3.67 | ||
| WCM | a | 0.145 | 0.091 | 0.0015 | 0.0000187 | |
| b | 0.53 | 0.75 | 2.16 | 3.60 | ||
| Modulus | PCM | a | 0.254 | 0.0000053 | 0.075 | 0.00067 |
| b | 0.69 | 5.17 | 1.59 | 2.82 | ||
| WCM | a | 0.137 | 0.0000036 | 0.384 | 0.081 | |
| b | 0.60 | 5.29 | 0.81 | 0.82 | ||
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yang, Y. Dynamic Tensile Properties of CFRP Manufactured by PCM and WCM: Effect of Strain Rate and Configurations. Crystals 2021, 11, 1491. https://doi.org/10.3390/cryst11121491
Yang Y. Dynamic Tensile Properties of CFRP Manufactured by PCM and WCM: Effect of Strain Rate and Configurations. Crystals. 2021; 11(12):1491. https://doi.org/10.3390/cryst11121491
Chicago/Turabian StyleYang, Yujin. 2021. "Dynamic Tensile Properties of CFRP Manufactured by PCM and WCM: Effect of Strain Rate and Configurations" Crystals 11, no. 12: 1491. https://doi.org/10.3390/cryst11121491
APA StyleYang, Y. (2021). Dynamic Tensile Properties of CFRP Manufactured by PCM and WCM: Effect of Strain Rate and Configurations. Crystals, 11(12), 1491. https://doi.org/10.3390/cryst11121491