Influence of Graphene Nanoplatelet Lateral Size on the Electrical Conductivity and Electromagnetic Interference Shielding Performance of Polyester Nanocomposites
Abstract
:1. Introduction
2. Materials and Methods
2.1. Materials
2.2. Morphological Characterization
2.3. Thermogravimetric Analysis (TGA)
2.4. Electrical Characterization
2.5. EMI Measurements
3. Results
3.1. Electrical Conductivity
3.2. Thermal Stability
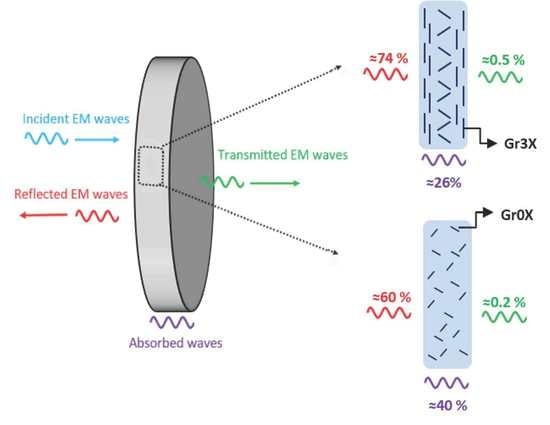
3.3. EMI Measurements
4. Discussion
- I
- II
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Redlarski, G.; Lewczuk, B.; Żak, A.; Koncicki, A.; Krawczuk, M.; Piechocki, J.; Jakubiuk, K.; Tojza, P.; Jaworski, J.; Ambroziak, D.; et al. The Influence of Electromagnetic Pollution on Living Organisms: Historical Trends and Forecasting Changes. Biomed Res. Int. 2015, 2015, 234098. [Google Scholar] [CrossRef] [Green Version]
- Hardell, L.; Carlberg, M. Mobile phones, cordless phones and the risk for brain tumours. Int. J. Oncol. 2009, 35, 5–17. [Google Scholar] [CrossRef] [Green Version]
- Sengupta, D.L.; Liepa, V.V. Applied Electromagnetics and Electromagnetic Compatibility; John Wiley & Sons, Inc.: Hoboken, NJ, USA, 2006. [Google Scholar]
- Geetha, S.; Kumar, K.K.S.; Rao, C.R.K.; Vijayan, M.; Trivedi, D.C. EMI shielding: Methods and materials—A review. Appl. Polym. Sci. 2009, 112, 2073–2086. [Google Scholar] [CrossRef]
- Dassan, E.G.B.; Rahman, A.A.A.; Abidin, M.S.Z.; Akil, H.M. Carbon nanotube–reinforced polymer composite for electromagnetic interference application: A review. Nanotechnol. Rev. 2020, 9, 768–788. [Google Scholar] [CrossRef]
- Wu, N.; Hu, Q.; Wei, R.; Mai, X.; Naik, N.; Pan, D.; Guo, Z.; Shi, Z. Review on the electromagnetic interference shielding properties of carbon based materials and their novel composites: Recent progress, challenges and prospects. Carbon 2021, 176, 88–105. [Google Scholar] [CrossRef]
- Gonzalez, M.; Pozuelo, J.; Baselga, J. Electromagnetic Shielding Materials in GHz Range. Chem. Rec. 2018, 18, 1000–1009. [Google Scholar] [CrossRef] [PubMed]
- Al-Saleh, M.H.; Sundararaj, U. Electromagnetic interference shielding mechanisms of CNT/polymer composites. Carbon 2009, 47, 1738–1746. [Google Scholar] [CrossRef]
- Sankaran, S.; Deshmukh, K.; Ahamed, B.M.; Pasha, S.K.K. Recent advances in electromagnetic interference shielding properties of metal and carbon filler reinforced flexible polymer composites: A review. Compos. Part A Appl. Sci. Manuf. 2018, 114, 49–71. [Google Scholar] [CrossRef]
- Kuester, S.; Demarquette, N.R.; Ferreira, J.C.; Soares, B.G.; Barra, G.M.O. Hybrid nanocomposites of thermoplastic elastomer and carbon nanoadditives for electromagnetic shielding. Eur. Polym. J. 2017, 88, 328–339. [Google Scholar] [CrossRef]
- Thomassin, J.-M.; Jérôme, C.; Pardoen, T.; Bailly, C.; Huynen, I.; Detrembleur, C. Polymer/Carbon based composites as electromagnetic interference (EMI) shielding materials. Mater. Sci. Eng. 2013, 74, 211–232. [Google Scholar] [CrossRef]
- Kuester, S.; Barra, G.M.O.; Demarquette, N.R. Morphology, mechanical properties and electromagnetic shielding effectiveness of poly (styrene-b-ethylene-ran-butylene-b-styrene)/carbon nanotube nanocomposites: Effects of maleic anhydride, carbon nanotube loading and processing method. Polym. Int. 2018, 67, 1229–1240. [Google Scholar] [CrossRef]
- Mahmoodi, M.; Arjmand, M.; Sundararaj, U.; Park, S. The electrical conductivity and electromagnetic interference shielding of injection molded multi-walled carbon nanotube/polystyrene. Carbon 2012, 50, 1455–1464. [Google Scholar] [CrossRef]
- Yousefi, N.; Sun, X.; Lin, X.; Shen, X.; Jia, J.; Zhang, B.; Tang, B.; Chan, M.; Kim, J. Highly Aligned Graphene/Polymer Nanocomposites with Excellent Dielectric Properties for High-Performance Electromagnetic Interference Shielding. Adv. Mater. 2014, 26, 5480–5487. [Google Scholar] [CrossRef] [PubMed]
- Song, P.; Liu, B.; Qiu, H.; Shi, X.; Cao, D.; Gu, J. MXenes for polymer matrix electromagnetic interference shielding composites: A review. Compos. Commun. 2021, 24, 100653. [Google Scholar] [CrossRef]
- Karimi, S.; Helal, E.; Gutierrez, G.; Moghimian, N.; Madinehei, M.; David, E.; Samara, M.; Demarquette, N. A Review on Graphene’s Light Stabilizing Effects for Reduced Photodegradation of Polymers. Crystals 2021, 11, 3. [Google Scholar] [CrossRef]
- Sarafraz, M.M.; Tlili, I.; Tian, Z.; Bakouri, M.; Safaei, M.R.; Goodarzi, M. Thermal Evaluation of Graphene Nanoplatelets Nanofluid in a Fast-Responding HP with the Potential Use in Solar Systems in Smart Cities. Appl. Sci. 2019, 9, 2101. [Google Scholar] [CrossRef] [Green Version]
- Moghimian, N.; Nazarpour, S. The Future of Carbon: An Update on Graphene’s Dermal, Inhalation, and Gene Toxicity. Crystals 2020, 10, 718. [Google Scholar] [CrossRef]
- Marsden, A.J.; Papageorgiou, D.G.; Valles, C.; Liscio, A.; Palermo, V.; Bissett, M.A.; Young, R.J.; Kinloch, I.A. Electrical percolation in graphene–polymer composites. 2D Mater. 2018, 5, 032003. [Google Scholar] [CrossRef] [Green Version]
- Kashi, S.; Gupta, R.K.; Baum, T.; Kao, N.; Bhattacharya, S.N. Dielectric properties and electromagnetic interference shielding effectiveness of graphene-based biodegradable nanocomposites. Mater. Des. 2016, 109, 68–78. [Google Scholar] [CrossRef]
- Wang, Z.; Luo, J.; Zhao, G. Dielectric and microwave attenuation properties of graphene nanoplatelet–epoxy composites. AIP Adv. 2014, 4, 017139. [Google Scholar] [CrossRef] [Green Version]
- Serenari, F.; Madinehei, M.; Moghimian, N.; Fabiani, D.; David, E. Development of Reinforced Polyester/Graphene Nanocomposite Showing Tailored Electrical Conductivity. Polymers 2020, 12, 2358. [Google Scholar] [CrossRef] [PubMed]
- Jiang, X.; Drzal, L.T. Multifunctional high-density polyethylene nanocomposites produced by incorporation of exfoliated graphene nanoplatelets: Crystallization, thermal and electrical properties. Polym. Compos. 2012, 33, 636–642. [Google Scholar] [CrossRef]
- Kashi, S.; Gupta, R.; Baum, T.; Kao, N.; Bhattacharya, S. Morphology, electromagnetic properties and electromagnetic interference shielding performance of poly lactide/graphene nanoplatelet nanocomposites. Mater. Des. 2016, 95, 119–126. [Google Scholar] [CrossRef]
- Sabzi, M.; Jiang, L.; Liu, F.; Ghasemi, I.; Atai, M. Graphene nanoplatelets as poly (lactic acid) modifier: Linear rheological behavior and electrical conductivity. J. Mater. Chem. A 2013, 1, 8253–8261. [Google Scholar] [CrossRef]
- Zhao, B.; Zhao, C.; Hamidinejad, M.; Wang, C.; Li, R.; Wang, S.; Yasamin, K.; Park, C. Incorporating a microcellular structure into PVDF/graphene–nanoplatelet composites to tune their electrical conductivity and electromagnetic interference shielding properties. J. Mater. Chem. C 2018, 6, 10292–10300. [Google Scholar] [CrossRef]
- Oskouyi, A.B.; Sundaraj, U.; Mertiny, P. Tunneling Conductivity and Piezoresistivity of Composites containing randomly dispersed conductive nano-platelets. Materials 2014, 7, 2501–2521. [Google Scholar] [CrossRef] [PubMed]
- Wang, Q.; Dai, J.; Li, W.; Wei, Z.; Jiang, J. The effects of CNT alignment on electrical conductivity and mechanical properties of SWNT/epoxy nanocomposites. Compos. Sci. Technol. 2008, 68, 1644–1648. [Google Scholar] [CrossRef]
- Kunz, K.; Krause, B.; Kretzschmar, B.; Juhasz, L.; Kobsch, O.; Jenschke, W.; Ullrich, M.; Pötschke, P. Direction Dependent Electrical Conductivity of Polymer/Carbon Filler Composites. Polymers 2019, 11, 591. [Google Scholar] [CrossRef] [Green Version]
- Marinho, B.; Ghislandi, M.; Tkalya, E.; Koning, C.E.; With, G.d. Electrical conductivity of compacts of graphene, multi-wall carbon nanotubes, carbon black, and graphite powder. Powder Technol. 2012, 221, 351–358. [Google Scholar] [CrossRef]
- Helal, E.; Kurusu, R.S.; Moghimian, N.; Gutierrez, G.; David, E.; Demarquette, N.R. Correlation between morphology, rheological behavior, and electrical behavior of conductive cocontinuous LLDPE/EVA blends containing commercial graphene nanoplatelets. J. Rheol. 2019, 63, 961. [Google Scholar] [CrossRef]
- David, É.; Fréchette, M.F.; Rosi, G.F.; Moghimian, N. Hysteresis Effect in the Electrical Conductivity of Graphene-enhanced Polyethylene Composites. In Proceedings of the IEEE International Conference on Nanotechnology, Pittsburgh, PA, USA, 25–28 July 2017; pp. 280–283. [Google Scholar]
- Moghimian, N.; Saeidlou, S.; Lentzakis, H.; Rosi, G.F.; Song, N.; David, É. Electrical Conductivity of Commercial Graphene Polyethylene Nanocomposites. In Proceedings of the 17th IEEE International Conference on Nanotechnology, Pittsburgh, PA, USA, 25–28 July 2017; pp. 757–761. [Google Scholar]
- Evans, S.; Haines, P.; Skinner, G. The effects of structure on the thermal degradation of polyester resins. Thermochim. Acta 1996, 278, 77–89. [Google Scholar] [CrossRef]
- Skinner, G.A.; Haines, P.J.; Lever, T.J. Thermal degradation of polyester thermosets prepared using dibromoneopentyl glycol. J. Appl. Polym. Sci. 1984, 29, 763–776. [Google Scholar] [CrossRef]
- Bansal, R.K.; Mittal, J. Thermal Stability and Degradation Studies of Polyester Resins. J. Appl. Polym. Sci. 1989, 37, 1901–1908. [Google Scholar] [CrossRef]
- Arjmand, M.; Apperley, T.; Okoniewski, M.; Sundararaj, U. Comparative study of electromagnetic interference shielding properties of injection molded versus compression molded multi-walled carbon nanotube/polystyrene composites. Carbon 2012, 50, 5126–5134. [Google Scholar] [CrossRef]
- Hamidinejad, M.; Zhao, B.; Zandieh, A.; Moghimian, N.; Filleter, T.; Park, C.B. Enhanced Electrical and Electromagnetic Interference Shielding Properties of Polymer-Graphene Nanoplatelet Composites Fabricated via Supercritical-fluid Treatment and Physical Foaming. ACS Appl. Mater. Interfaces 2018, 10, 30752–30761. [Google Scholar] [CrossRef]
- Duan, H.; Zhu, H.; Gao, J.; Yan, D.-X.; Dai, K.; Yang, Y.; Zhao, G.; Liu, Y.; Li, Z.-M. Asymmetric conductive polymer composite foam for absorption dominated ultra-efficient electromagnetic interference shielding with extremely low reflection characteristics. J. Mater. Chem. A 2020, 8, 9146. [Google Scholar] [CrossRef]
- Green, M.; Chen, X. Recent progress of nanomaterials for microwave absorption. J. Mater. 2019, 5, 503–541. [Google Scholar] [CrossRef]
- Kim, T.; Do, H.W.; Choi, K.; Kim, S.; Lee, M.; Kim, T.; Yu, B.; Cheon, J.; Min, B.-W.; Shim, W. Layered Aluminum for Electromagnetic Wave Absorber with Near-Zero Reflection. Nano Lett. 2021, 21, 1132–1140. [Google Scholar] [CrossRef] [PubMed]
- Zhao, B.; Wang, R.; Li, Y.; Ren, Y.; Li, X.; Guo, X.; Zhang, R.; Park, C.B. Dependence of electromagnetic interference shielding ability of conductive polymer composite foams with hydrophobic properties on cellular structure. J. Mater. Chem. C 2020, 8, 740. [Google Scholar] [CrossRef]









Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Madinehei, M.; Kuester, S.; Kaydanova, T.; Moghimian, N.; David, É. Influence of Graphene Nanoplatelet Lateral Size on the Electrical Conductivity and Electromagnetic Interference Shielding Performance of Polyester Nanocomposites. Polymers 2021, 13, 2567. https://doi.org/10.3390/polym13152567
Madinehei M, Kuester S, Kaydanova T, Moghimian N, David É. Influence of Graphene Nanoplatelet Lateral Size on the Electrical Conductivity and Electromagnetic Interference Shielding Performance of Polyester Nanocomposites. Polymers. 2021; 13(15):2567. https://doi.org/10.3390/polym13152567
Chicago/Turabian StyleMadinehei, Milad, Scheyla Kuester, Tatiana Kaydanova, Nima Moghimian, and Éric David. 2021. "Influence of Graphene Nanoplatelet Lateral Size on the Electrical Conductivity and Electromagnetic Interference Shielding Performance of Polyester Nanocomposites" Polymers 13, no. 15: 2567. https://doi.org/10.3390/polym13152567
APA StyleMadinehei, M., Kuester, S., Kaydanova, T., Moghimian, N., & David, É. (2021). Influence of Graphene Nanoplatelet Lateral Size on the Electrical Conductivity and Electromagnetic Interference Shielding Performance of Polyester Nanocomposites. Polymers, 13(15), 2567. https://doi.org/10.3390/polym13152567