3.1. Scattering Process and Convergence Analyses
The scattering process of gas molecules on solid surfaces can be composed of the adsorption, trapping, and escaping stages. One of the latter two stages may not show up during the scattering.
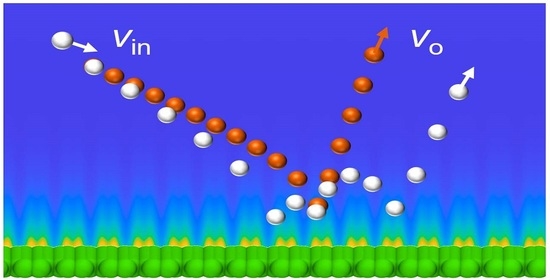
Figure 1c demonstrates two characteristic scattering processes of incident helium on the monolayer graphene surface at 1000 K. The speed, zenith angle, and azimuth angle of the incident helium of both samples are 17.652 Å/ps, 90°, and 0°, respectively. It is worth noting that the incident angle relative to the approaching local graphene surface may deviate slightly from the original values. In our simulation, we found that that was not significant. Here, we define the incident angles in a way consistent with the definition in the molecular beam experiments. The normal velocity component,
vz, of incident helium was zero, such that the helium atom was adsorbed onto the graphene surface by the van der Waals interactions. As shown in
Figure 1c, the distance of helium from the graphene surface and the normal velocity component of helium are depicted by the black and red curves, respectively. As the helium atom approached the graphene surface,
vz decreased from zero to the negative maximum, where the negative sign indicates helium moving towards the graphene surface. During this process, helium atoms initially moved slowly downward and then accelerated. Afterward, the interaction between helium and graphene changed from attraction to repulsion, and the normal velocity component of helium declined. When helium arrived at the first minimum position above the graphene surface,
vz became zero. After that, the helium atom bounced back from the graphene surface due to the repulsion forces between helium and graphene. The
vz of helium atom increased quickly to the local maximum, where the attraction and repulsion between helium and graphene were balanced. Then,
vz decreased as the attraction of graphene became weaker, dominating the graphene–helium interaction as helium moved away from the graphene. There were several different following behaviors. As shown in sample 1, the helium was far away enough from the graphene surface and had enough kinetic energy to escape from attraction by the graphene, leading to a scattering process with a single bounce. Another case is that the kinetic energy stored in the graphene might not be large enough for the first bounce-back process, and the helium atom would be attracted to the graphene surface again until it obtained enough kinetic energy to escape from attraction by the graphene. This is illustrated by sample 2, in which there were five bounces of helium until it flew away from the interaction region. In this study, we did not find any cases where the helium atom was trapped on the graphene surface within the simulation time.
The scattering behavior shown above varies randomly depending on the initial status of the graphene and the helium atom, such as the initial location, incident velocity, and incident angle of the helium atom. As shown in
Figure S2, we found that the cumulative averages of TACs converged when 500 systems were sampled with the same incident velocities but random initial in-plane locations of the helium atom. For the illustrated samples in
Figure S2, the incident speed of the helium atom was 17.652 Å/ps, which corresponds to the root mean square velocity of helium at 500 K; the zenith angle, θ, varied from 0° to 50° with an increment of 10°; and the azimuth angle was selected as 0° or 90°. The cumulative averages of the thermal accommodation coefficient (TAC), real normal thermal accommodation coefficient (RNTAC), real tangential thermal accommodation coefficient (RTTAC), and nominal normal thermal accommodation coefficient (NNTAC) converged with an increasing number of samples for all systems. For all data discussed below, the mean value and standard deviation were obtained via four evaluations with different initial velocity seeds of graphene, and each evaluation was calculated by averaging results from 500 samples with the same initial conditions except for the random in-plane initial position of the helium atom.
3.2. Effect of Zenith Angle of Incident Helium Atoms
Systems of monolayer graphene and a helium atom were simulated to elucidate the zenith-angle effect of the incident helium on the scattering process. The zenith angle (
θin) ranged from 0° to 90° with an increment of 10°, while the azimuthal angle (
φin) was specified at 0°, 30°, 60°, and 90°, respectively, as shown in
Figure 2. Given
φin, TAC values increased significantly with larger values of
θin. Furthermore, TAC was insensitive to the
φin with the given
θin. For instance, TAC was 0.127 for a
θin of 0° and 0.319 for a
θin of 90°, which was 2.525 times that of the former value. For
θin of 0°, the incident velocity of the helium atom was normal to the graphene surface, and it was parallel to the surface for
θin of 90°. To evaluate the contribution of the normal and in-plane velocity components, we decomposed the TAC into nominal normal thermal accommodation (NNTAC) and nominal tangential thermal accommodation coefficients (NTTAC) in Equations (2) and (3).
Figure 2b,c shows that NNTAC increased with an increasing zenith angle, while NTTAC decreased with a larger zenith angle. It is worthy of note that the value of NTTAC was much smaller than that of NNTAC. In particular, NTTAC was negative when
θin was greater than 60°, which means that in-plane kinetic energy was transferred from the helium to the graphene. As a result, we found that the kinetic energy component of the normal velocity contributed to a higher TAC and higher energy transfer along with a larger zenith angle. According to the definition in the Maxwell model, the real normal thermal accommodation coefficient (RNTAC,
αn*) and real tangential thermal accommodation coefficient (RTTAC,
ατ*) were calculated by Equations (5) and (6), respectively.
Figure 2e,f shows that the trends of RNTAC and RTTAC were consistent with those of NNTAC and NTTAC, respectively. There were larger variances in RNTAC at small zenith angles and RTTAC at large zenith angles. The reason is that the normal kinetic energy of the incident helium atom was very close to that of the diffusively scattered helium atom, which resulted in a small value as the denominator and hence a larger variation in kinetic energy transferred in various systems. In detail, the normal kinetic energy of the helium atom after diffusive scattering (
kB·
Ts) was 0.086 eV, and the kinetic energy of the incident helium atom at 500 K was 0.0646 eV, which was 75.1% of the former. When the zenith angle was smaller than 30°, the normal kinetic energy transferred by ideal diffusive scattering was a small value, which is the denominator. The case was the same when the zenith angle was larger than 70°. Interestingly, the variance in RNTAC at a small
θin was larger than that of RTTAC at a high value of
θin, which also demonstrates the importance of the normal velocity of the incident helium and the large contribution of the normal kinetic energy transfer during the scattering process.
A linear correlation between the zenith angles of incident and scattered gas molecules was previously reported for zenith angles in the range of 30°~70°. The zenith angles were limited due to restrictions in the experimental operations. In this study, the relationship was found to be nonlinear across the whole range of the incident angles of the helium atoms. As shown in
Figure 2d, the curve deviated significantly from the dashed line as the zenith angle decreased or increased from this middle region between 30° and 70°. Here, the dashed line denotes that the zenith angles of the incidence were the same as those of the scattered helium. The scattered helium atom escaped from the graphene surface at a zenith angle of 19.03° when the incident helium was normal to the graphene. The in-plane force component applied to the helium atom could not be balanced due to the random vibration of carbon atoms of graphene, and the scattered helium atom deviated from the normal of the graphene surface depending on the attraction force. The zenith angle of the scattered helium was 57.84° for a
θin of 90°. In those cases, the normal velocity of the incident helium atoms was zero, and helium was adsorbed onto the graphene surface purely by the van der Waals interactions and gained kinetic energy normal to the surface during scattering. In-plane kinetic energy was transferred from the helium to the graphene, but the energy loss of helium was very low. The gain in normal kinetic energy was larger than the loss in in-plane kinetic energy, and the resultant energy transfer was enhanced. Moreover, the trends of zenith angles were consistent with that of the TAC and almost coincident with different azimuthal angles.
Figure 2g shows the bounce numbers of helium on graphene with different incident angles. The normal velocity of the outgoing helium atom determined whether the helium atom could escape from the surface. The curve presents three sections. First, the average bounce number increased slowly from 1.20 at a
θin of 0° until
θin reached 30°. For those cases, the normal velocities of helium were much larger than the in-plane velocities. After scattering, the helium atom could obtain both in-plane and normal kinetic energy and escape from the graphene surface. Second, the bounce number gradually increased with
θin above 30° until it reached 60°. In this range of
θin, the in-plane and normal velocity components of helium were comparable, the in-plane kinetic energy gain was marginal, and the major energy transfer was due to enhancement of the normal kinetic energy. Subsequently, the bounce number sharply increased with
θin, up to 1.82 at a
θin of 80°, and then decreased slightly from 80° to 90° except for the case of
φin of 30°. In this range, the in-plane velocity component of helium was larger than the normal component, and the in-plane energy transfer was from helium to graphene. Helium had to bounce more often on the graphene surface to gain enough energy to escape from the surface. The TAC averaged by the bounce number of helium increased from 0.105 to 0.177 as the zenith angle increased from 0° to 90°.
The interaction time between helium atoms and the graphene surface provides another angle to understand the scattering process of helium on the surface, as shown in
Figure 2h. Interaction time was considered to start when the helium atom entered the cutoff distance above the graphene surface and to end when the scattered helium eventually returned beyond that distance. Curves of
t12 denoting 12 Å above the graphene surface (the cutoff distance of the van der Waals interaction) were set as the threshold planes of the scattering. Those curves showed that, similarly to the bounce number, the interaction time had a correlation with the zenith angle of the incident helium except for the special cases of a
θin of 90°. The interaction time gradually increased from 1.839 ps to 4.968 ps for a
θin from 0° to 80°. The TAC averaged by the interaction time changed slightly. For
θin of 90°, the value rose sharply to 16.681 ps. To evaluate the influence of the normal velocity of the incident helium, we evaluated the interaction time by setting the threshold planes as 7 Å above the graphene surfaces. The dashed curves represent the results in
Figure 2h. As shown in
Figure 1c, the normal velocity of the incident helium atom changed significantly when the distance of the helium from the surface was less than 7 Å. By this definition, the interaction time increased with zenith angle, without the sharp jump. Helium atoms took a significantly longer time to accelerate, since the normal velocity of helium is zero with
θin of 90°, which decreased the efficiency of the heat energy transfer.
To understand why the zenith angle of incident helium significantly affected the TAC and scattering behaviors, we plotted the contour of the potential energy for the helium–graphene system. The potential energy was calculated by averaging the potential energy of the system in a dynamic run. First, a helium atom was introduced above the well-equilibrated monolayer graphene. Then, the potential energy was sampled by placing the helium atom in the x–z plane shown by an inlet with a resolution of 0.1Å. The reference potential energy was set when the helium atom was 20 Å above the graphene surface. Then, the system ran 1000 steps in the NVE ensemble with a timestep of 1.0 fs between each sample of the potential energy. In this way, the system was sampled 1000 times, and the averaged potential energies were plotted in the x–z plane in
Figure 2i and in the y–z plane in
Figure S3. It is worth noting that the location of the helium atom in the shown snapshot is not the origin point of the coordinate system. Both
Figure 2i and
Figure S3 show that the averaged potential energy was heterogeneous, and the gradient of the contour lines varied at different spots below the plane of 3.6 Å above the graphene surface. The variation is significant, especially in the region close to the surface. Hence, incident helium atoms of different zenith angles were subjected to various forces. Approaching the surface at different trajectories resulted in a significant change in the heat energy transfer.
3.3. Effect of Azimuthal Angle of Incident Helium Atoms
Figure 2 shows that the scattering process of helium on graphene was insensitive to the azimuths of 0°, 30°, 60°, and 90°. In order to better understand the effect of the azimuth of incident helium, we investigated the scattering behavior of helium atoms by increasing the azimuth from 0° to 90° at increments of 10°. The zenith angle was specified at 10° or 90° to consider two representative cases. The former represents a scenario where the helium atom is almost perpendicular to the graphene surface, while the latter is for a case where the incident velocity of the helium atom is parallel to the graphene surface. An angle of 10° was chosen instead of 0° because there was no difference in the in-plane velocity component of incident helium with a
θin of 0°.
Figure 3a shows that the TAC values for a zenith angle of 10° varied from 0.104 to 0.142, with an average of 0.129 over the range of azimuthal angles. The TAC values of the zenith angle of 90° ranged from 0.290 to 0.347, with an average of 0.316. The variance in TACs was within 30% and 20% in the cases of
θin of 10° and 90°, respectively. Those results confirm that the energy transfer of helium on graphene was insensitive to the azimuthal angle.
Figure 3b,c indicates that the effect of the azimuth was negligible for the normal and in-plane components of TAC. In the case of a
θin of 10°, the NNTAC values ranged from 0.043 to 0.079, while the NTTAC values fluctuated from 0.061 to 0.067. We found that the relative variance in the normal kinetic energy transfer was larger than that in the in-plane counterpart. The absolute values of NTTAC were comparable to those of the NNTAC. In the case of a
θin of 90°, the NNTAC values ranged from 0.304 to 0.353, while the NTTAC values fluctuated from −0.023 to −0.0006. It is notable that the in-plane energy transfers from helium to graphene and the change in NNTAC were much larger than those in NTTAC in absolute values. In terms of RNTAC and RTTAC, the red curve of RNTAC and blue cure of RTTAC show larger fluctuations in
Figure 3e,f, which represent the cases of
θin of 10° and
θin of 90°, respectively. The reasons are the same as described above. On the other hand, we found that the variation in RNTAC with a
θin of 90˚ and RTTAC with a
θin of 10˚ was much lower than their counterparts, and the absolute values of those curves were much larger. We can conclude that energy transfer between helium and graphene is insensitive to the azimuth.
The zenith angle of scattered helium (
θo), average bounce number of helium, and interaction time of helium with graphene (
t) were plotted with the various azimuthal angles in
Figure 3d,g,h, respectively. The values of
θo for a
θin of 10° ranged between 21.056° and 21.932°, while the range of
θo for a
θin of 90° was from 57.463° to 59.042°. The variance in both cases was less than 4% and was much smaller than the change in TAC.
Regarding the parameter of the bounce number, we found that the values for a θin of 10° varied between 1.167 and 1.212, and the difference was less than 4% of the lowest value. In the case of a θin of 90°, the values lay between 1.713 and 1.896, and the change was within 10%. For interaction time, the range was from 1.803 ps to 1.867 ps in the case of a θin of 10° and from 16.541 ps to 16.786 ps in the other case. Given these three parameters, the effect of the azimuthal angle was not significant and much smaller than the effect of the zenith angle, shown by the large differences between the red and blue curves.
To understand why the azimuth angle plays a negligible role in the scattering effect, we observed the potential energy of the helium–graphene system by fixing the helium at six in-plane locations, as shown by the inset of
Figure 3i. The helium atom was placed 20 Å above the graphene surface, and the reference potential energy of the system was set. Then, we performed molecular dynamics simulations in the NVE ensemble for 1000 steps with a time step of 1.0 fs. Next, the potential energy of the system was sampled by placing the helium atom at the six in-plane locations and varying the distance between the helium and the graphene surface. In this way, the first loop was completed. Then, these steps were repeated until the potential energy was sampled for 1000 loops.
Figure 3i shows the potential energy of the six in-plane locations with respect to the distance between the helium and the graphene. Given a plane parallel to the graphene surface, the potential-energy difference depends on the distance between the helium and the graphene surface. When the plane was much closer to the surface, we found that the average potential energy differed greatly. When the system was observed, the helium was placed at a fixed observation point, and the graphene dynamically vibrated during the dynamic run, which led to variance in the distance between them and hence a large range of potential energies. This variance was larger than the difference caused by the in-plane locations. As the distance of helium became further away from the graphene surface, both the variance from the dynamic vibration of graphene and that from the in-plane locations decreased. The former was more significant than the latter. In this way, the influence of dynamic vibration overwhelmed that of the in-plane location. As a result, the influence of the azimuth on the scattering process was less significant than random sampling, and can be negligible.
3.4. Effect of Temperature of Graphene Surfaces
Here, we fixed the speed of helium at the temperature of 500 K and changed the temperature of the graphene from 1000 K to 1500 K and 2000 K to understand the effect of graphene temperature. Based on the significance of the zenith angle on the surface scattering, we also explored systems with ten different zenith angles and the azimuthal angle at 0° and 90°.
Figure 4a shows that the TAC was still very sensitive to the zenith angle and insensitive to the azimuthal angle in the cases of different graphene temperatures. TAC increased with larger zenith angles. However, the increase in TAC was restrained by higher graphene temperatures. When the zenith angle was small, and the normal velocity component of helium dominated, systems with higher graphene temperatures had larger TACs. When the zenith angle was large and the in-plane velocity component prevailed, systems with graphene of lower temperatures had larger TACs. TAC values changed slightly when the zenith angle was 40° and 50°. Given various graphene temperatures, the trend for NNTAC was the same as that for TAC, while NTTAC had the opposite trend, as shown in
Figure 4b,c. The normal kinetic energy transfer dominated in all cases of different graphene temperatures.
Figure 4e,f shows that the RNTAC and RTTAC with different temperatures of graphene were consistent with NNTAC and NTTAC, respectively, except for a larger fluctuation at lower zenith angles for NNTAC and higher zenith angles for NTTAC. The zenith angle effect became insensitive with higher graphene temperatures. In all, the TACs and their components became less sensitive to zenith angle when increasing the graphene temperature.
The zenith angle of the scattered helium (
θo) shown in
Figure 4d remained nonlinear relative to the zenith angle of the incident helium. As the graphene temperature increased, the nonlinearity reduced. When
θin was larger than 30°, the zenith angle
θo became smaller with higher graphene temperatures. This change increased as
θin increased. In addition, the change in
θo with graphene temperature was much lower than that in TAC and TAC components, and changes at a high
θin were larger than those at a low
θin. In the cases of the three temperatures, the
θin of specular reflection was between 30° and 40° and became smaller with higher graphene temperatures. The linear region of
θin was between 30° and 60°, and the slope became smaller with higher graphene temperatures.
Higher graphene temperatures resulted in a lower bounce number and slightly lower interaction time of helium with graphene, as shown in
Figure 4g,h. The change in the bounce number became more prominent at a high zenith angle of incident helium with a higher graphene temperature. For instance, the bounce number of helium with an incident azimuth of 0° and zenith of 90° reduced from 1.798 to 1.573 and 1.428 as the temperature of the graphene increased from 1000 K to 1500 K and 2000 K, respectively. Furthermore, a large bounce number could not guarantee a high TAC. When
θin was larger than 40°, and the in-plane velocity of helium was larger than the normal velocity, a higher bounce number corresponded to a larger TAC. However, when
θin was small and the normal velocity prevailed, a higher bounce number corresponded to a lower TAC. The bounce number of helium with incident azimuth of 0° and zenith of 90° reduced from 1.200 to 1.174 and 1.158 as the graphene temperature increased.
Figure 4h shows that the interaction time was consistent with the bounce number. A higher graphene temperature led to a slightly shorter interaction time. These results show that energy transfer between helium and graphene is very complicated, and the common wisdom that energy transfer is positively correlated with the bounce number was not valid in this study.
To further understand the effect of surface temperature, we plotted the relative potential energy of the system by fixing the in-plane position of helium at the location of point six and changing the normal distance of helium from the graphene.
Figure 4i indicates that a higher graphene temperature leads to a more significant fluctuation in relative potential energy and the mean value of the potential energy was larger with a higher graphene temperature. In detail, when helium was 2.6 Å away from the graphene surface, the relative potential energy was 0.103 eV, 0.070 eV, and 0.034 eV, with fluctuations of 0.195 eV, 0.138 eV, and 0.067 eV, when the temperature was 2000 K, 1500 K, and 1000 K, respectively. In the dynamic run, a higher graphene temperature led to stronger vibration of the graphene surface and larger variation in the distances between helium and graphene and the ones between the adjacent carbon atoms in the graphene. Since the relation between potential energy and the atomic distance was nonlinear in this range, a larger fluctuation and mean value of the relative potential was observed, and the importance of the zenith angle effect was reduced.
3.5. Effect of Helium Speed
To understand how the speed of helium affects energy transfer, we fixed the temperature of the graphene at 1000 K and initialized incident velocities of helium corresponding to 100 K, 300 K, 500 K, and 700 K.
Figure 5a shows that the dependency of the TAC on the zenith angle of incident helium reduced with decreasing helium temperatures. In the cases of helium at 300 K, 500 K, and 700 K, systems with larger
θin showed higher TACs, but the slope of TAC relative to
θin became smaller from 700 K to 300 K. In the case of 100 K, the TACs of the systems were insensitive to the
θin. When the incident angle was smaller than 50°, we found that TACs decreased with higher helium temperatures. In contrast, TACs increased with higher incident speeds of helium when
θin was larger than 50°.
Figure 5b,c shows that TAC had the same trend with various zenith angles as NNTAC but the opposite trend to NTTAC. Generally, the energy transfer via the normal velocity component still outperformed that via the in-plane velocity component. However, in the case of 700 K and a
θin of 0°, the NNTAC was smaller than the NTTAC, where the in-plane energy transfer dominated. In the case of 100 K, both NNTAC and NTTAC were not dependent on
θin. RNTAC and RTTAC showed a similar pattern with helium temperature and
θin as NNTAC and NTTAC, respectively, as shown in
Figure S4. The fluctuation in RNTAC at low
θin and RTTAC at high
θin was larger with higher incident speeds of helium. It is worth noting that both the in-plane and normal velocity components changed at the same proportion when we changed the speed of helium with a fixed
θin.
The nonlinearity between
θo and
θin remained for all cases with helium temperatures from 100 K to 700 K, as shown in
Figure 5d. For systems with lower helium temperatures, the enhancement in
θo with a larger
θin would be suppressed as
θin was greater than 20°, and the slope of the linear segment of the
θo against
θin curve decreased. In this way, the specular reflectance angle of the incident helium was in the range of 20°~30°, 30°~40°, 40°~50°, and 40°~50° for helium temperatures of 100 K, 300 K, 500 K, and 700 K, respectively. When the incident helium had no normal velocity component and in-plane velocity along the
x-axis, the corresponding
θo was 39.36°, 52.32°, 57.47°, and 61.09°, respectively. In the case of 100 K, the nonlinearity was significantly reduced. In this way, reducing the temperature of helium could suppress the effect of the zenith angle of incident helium.
Different from the above-mentioned results,
Figure 5g,h depicts that the bounce number and the interaction time of helium were susceptible to the speed of incident helium. Moreover, the influence of the azimuth on the bounce number of helium was enlarged with a lower incident helium speed. Especially at a low
θin, the bounce number increased drastically with a lower incident helium speed. For instance, the values were 1.58, 1.32, 1.20, and 1.11 with increasing helium speed and a
θin of 0°. When the
θin was smaller than 50°, the bounce number was consistent with the TAC. A larger
θin reduced the difference in the normal velocity component of the helium atom, shown in
Figure 5i, and the difference in the bounce number. When
θin was larger than 50°, the larger bounce number caused by the lower speed of helium corresponded to a lower TAC. Furthermore, the lower incident speed of helium significantly increased the interaction time due to the lower normal velocity of helium and higher bounce number. All these results show the significance of the incident speed of helium on scattering behavior.
3.6. Conversion of Angle-Resolved TAC to TAC with Gas Molecules from Hemispherical Space
Since the scattering process of helium on graphene is insensitive to the azimuth, we obtained the zenith-angle-dependent TAC, NNTAC, NTTAC, bounce number, and interaction time of helium on graphene. Then, we could describe the TAC considering all molecules from the hemispherical space above the graphene surface. The relative frequencies in ten different ranges of
θin converged when the number of samples was above 25,000 in the Monte Carlo simulations, as shown in
Figure 6a. The range ID from one to ten represents the zenith ranges of [0°,5°], [5°,15°], … [85°,90°]; i.e., for each middle range ID, the interval width was 10°.
Figure 6b,c shows scatterplots of the in-plane and normal velocity distributions. For a clearer scatterplot, we present a distribution with 10,000 samples. The in-plane velocity plot of
Vx against
Vy represents a uniform distribution along the azimuth and becomes sparser along the radial direction from the zenith point. However, this does not mean that there would be a higher distribution with lower helium speed and zenith angle. The relative frequencies of
Vx,
Vy,
Vz, and
V are shown in
Figure S5.
Vx and
Vy followed a normal distribution, while
Vz and
V were in a right-skewed distribution. At a higher incident helium speed,
Vx and
Vy were narrower around the mean value, but
Vz and
V were right-shifted.
Figure 6c depicts that the distribution with low and high zenith angles was low. Instead, the most probable distribution of the zenith angle of incident helium was between 45° and 55°. The expected
θin was 45.55° according to the given relative frequency in each range of the zenith angle shown in
Figure 6d, which was used to weight the zenith angle-resolved TAC for the TAC of helium molecules in the hemispherical space.
Figure 6e presents the TAC and NNTAC for systems with various graphene temperatures and speeds of incident helium denoted by top and bottom tick labels, respectively. The blue bars for NNTAC are on top of the red bars for TAC, and indicate that the NNTAC contributed more than 90% to the TAC for all cases. Interestingly, TAC, NNTAC, and NTTAC (see
Figure 6f) exhibited a similar dependence on the graphene temperature and the helium incident speed. All of them increased with increasing helium incident speed from 100 K to 700 K, except for a minor drop in NTTAC from 0.0174 to 0.0169 as
Tg increased from 100 K to 300 K. In terms of the zenith-angle-resolved counterparts, these three parameters had various relationships with zenith angle and helium temperature. They displayed the opposite dependence on helium temperature at low and high zenith angles. However, they were balanced and resulted in the trend observed for the integrated TAC. Moreover, all three parameters decreased with higher graphene temperatures. In this regard, we could improve the TAC via lower-temperature graphene and higher-temperature helium gas.
Figure 6g–i display the corresponding zenith angle of scattered helium, bounce number, and interaction time of helium with graphene. Given a graphene temperature of 1000 K,
θo increased from 31.23° to 39.32°, 42.62°, and 44.55° as helium temperature increased from 100 K to 700 K. The higher the temperature of helium, the closer its scattering behavior approached the expected value, 45.55°, of the incident zenith angle. Furthermore,
θo decreased from 42.62° to 40.78° and 39.14° when increasing the graphene temperature from 1000 K to 1500 K and 2000 K, respectively, and fixing the temperature of helium at 500 K. These data indicate that
θo became closer to the expected value of
θin upon reducing the temperature difference between helium and graphene. Contrary to common wisdom,
Figure 6h,i demonstrated that higher bounce numbers and interaction times of helium with graphene could not guarantee a higher energy transfer between helium and graphene. When the graphene temperature was fixed at 1000 K, the bounce number of helium decreased from 1.70 to 1.52, 1.39, and 1.32 for helium temperatures of 100 K, 300 K, 500 K, and 700 K, respectively. In this case, the interaction time declined, which was consistent with the trend for the bounce number. However, this trend was opposite to that for the TAC. On the other hand, the bounce number and interaction time decreased with higher graphene temperatures, which agreed very well with the trend for the TAC.