Phonon Transport through Nanoscale Contact in Tip-Based Thermal Analysis of Nanomaterials
Abstract
:1. Introduction
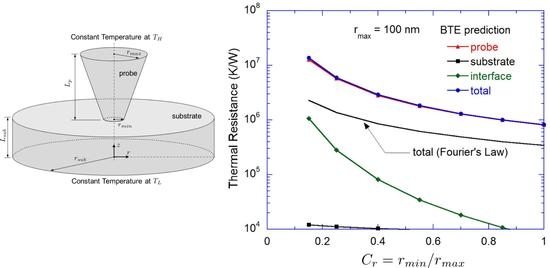
2. Results and Discussion
3. Summary
Acknowledgments
Author Contributions
Conflicts of Interest
References
- Cahill, D.G.; Ford, W.K.; Goodson, K.E.; Mahan, G.D.; Majumdar, A.; Maris, H.J.; Merlin, R.; Phillpot, S.R. Nanoscale Thermal Transport. J. Appl. Phys. 2003, 93, 793–818. [Google Scholar] [CrossRef]
- Cahill, D.G.; Braun, P.V.; Chen, G.; Clarke, D.R.; Fan, S.; Goodson, K.E.; Keblinski, P.; King, W.P.; Mahan, G.D.; Majumdar, A.; et al. Nanoscale Thermal Transport. II. 2003–2012. Appl. Phys. Rev. 2014, 1, 11305. [Google Scholar] [CrossRef]
- Shi, L.; Dames, C.; Lukes, J.R.; Reddy, P.; Duda, J.; Cahill, D.G.; Lee, J.; Marconnet, A.; Goodson, K.E.; Bahk, J.H.; et al. Evaluating Broader Impacts of Nanoscale Thermal Transport Research. Nanoscale Microscale Thermophys. Eng. 2015, 19, 127–165. [Google Scholar] [CrossRef]
- Yun, J.; Ahn, J.H.; Lee, B.J.; Moon, D.I.; Choi, Y.K.; Park, I. Temperature Measurement of Joule Heated Silicon Micro/Nanowires Using Selectively Decorated Quantum Dots. Nanotechnology 2016, 27, 505705. [Google Scholar] [CrossRef] [PubMed]
- Jeon, J.; Park, S.; Lee, B.J. Optical Property of Blended Plasmonic Nanofluid Based on Gold Nanorods. Opt. Express 2014, 22, A1101–A1111. [Google Scholar] [CrossRef] [PubMed]
- Han, S.; Shin, J.H.; Jung, P.H.; Lee, H.; Lee, B.J. Broadband Solar Thermal Absorber Based on Optical Metamaterials for High-Temperature Applications. Adv. Opt. Mater. 2016, 4, 1265–1273. [Google Scholar] [CrossRef]
- Zhai, Y.; Ma, Y.; David, S.N.; Zhao, D.; Lou, R.; Tan, G.; Yang, R.; Yin, X. Scalable-Manufactured Randomized Glass-Polymer Hybrid Metamaterial for Daytime Radiative Cooling. Science 2017, 355, 1062–1066. [Google Scholar] [CrossRef] [PubMed]
- Binnig, G.; Despont, M.; Drechsler, U.; Haeberle, W.; Lutwyche, M.; Vettiger, P.; Mamin, H.; Chui, B.; Kenny, T.W. Ultrahigh-Density Atomic Force Microscopy Data Storage with Erase Capability. Appl. Phys. Lett. 1999, 74, 1329–1331. [Google Scholar] [CrossRef]
- Poggi, M.A.; Lillehei, P.T.; Bottomley, L.A. Chemical Force Microscopy on Single-Walled Carbon Nanotube Paper. Chem. Mater. 2005, 17, 4289–4295. [Google Scholar] [CrossRef]
- King, W.P.; Bhatia, B.; Felts, J.R.; Kim, H.J.; Kwon, B.; Lee, B.; Somnath, S.; Rosenberger, M. Heated Atomic Force Microscope Cantilevers and Their Applications. Ann. Rev. Heat Transf. 2013, 16, 287–326. [Google Scholar] [CrossRef]
- Kim, K.; Song, B.; Fernández-Hurtado, V.; Lee, W.; Jeong, W.; Cui, L.; Thompson, D.; Feist, J.; Reid, M.H.; García-Vidal, F.J.; et al. Radiative Heat Transfer in the Extreme Near Field. Nature 2015, 528, 387–391. [Google Scholar] [CrossRef] [PubMed]
- Jean, V.; Fumeron, S.; Termentzidis, K.; Zianni, X.; Lacroix, D. Monte Carlo Simulations of Phonon Transport in Si Nanowires with Constrictions. Int. J. Heat Mass Transf. 2015, 86, 648–655. [Google Scholar] [CrossRef]
- Dulhani, J.; Lee, B.J. Effect of Constriction on Phonon Transport in Silicon Thin Films and Nanowires. Smart Sci. 2016, 4, 173–179. [Google Scholar] [CrossRef]
- Jean, V.; Randrianalisoa, J.; Trannoy, N. Monte Carlo Simulation of Phonon Transport Across Si-Si and SiO2 Interfaces. In Proceedings of the 21st International Workshop on Thermal Investigations of ICs and Systems (THERMINIC), Paris, France, 30 September–2 October 2015; pp. 1–6. [Google Scholar]
- Cooper, M.; Mikić, B.; Yovanovich, M. Thermal Contact Conductance. Int. J. Heat Mass Transf. 1969, 12, 279–300. [Google Scholar] [CrossRef]
- Mikić, B. Thermal Contact Conductance; Theoretical Considerations. Int. J. Heat Mass Transf. 1974, 17, 205–214. [Google Scholar] [CrossRef]
- Singh, D.; Murthy, J.Y.; Fisher, T.S. Phonon Transport Across Mesoscopic Constrictions. J. Heat Transf. 2011, 133, 42402. [Google Scholar] [CrossRef]
- Prasher, R. Predicting the Thermal Resistance of Nanosized Constrictions. Nano Lett. 2005, 5, 2155–2159. [Google Scholar] [CrossRef] [PubMed]
- Lemonnier, D. Solution of the Boltzmann Equation for Phonon Transport. In Microscale and Nanoscale Heat Transfer; Springer: Berlin, Germany, 2007; pp. 77–106. [Google Scholar]
- Chai, J.C.; Parthasarathy, G.; Lee, H.S.; Patankar, S.V. Finite Volume Radiative Heat Transfer Procedure for Irregular Geometries. J. Thermophys. Heat Transf. 1995, 9, 410–415. [Google Scholar] [CrossRef]
- Kim, M.Y.; Baek, S.W. Analysis of Radiative Transfer in Cylindrical Enclosures using the Finite Volume Method. J. Thermophys. Heat Transf. 1997, 11, 246–252. [Google Scholar] [CrossRef]
- Chen, G. Thermal Conductivity and Ballistic-Phonon Transport in the Cross-Plane Direction of Superlattices. Phys. Rev. B 1998, 57, 14958. [Google Scholar] [CrossRef]
- Cheney, D.A. Computational Modeling of Geometry Dependent Phonon Transport in Silicon Nanostructures. Ph.D. Thesis, University of Pennsylvania, Philadelphia, PA, USA, 2013. [Google Scholar]
- Muñoz, R.M.; Abad, B.; Manzano, C.; Torres, P.; Cartoixà, X.; Alvarez, F.; Martín, G.M. Thermal Conductivity of Bi2Te3 Nanowires: How Size Affects Phonon Scattering. Nanoscale 2017, 9, 6741–6747. [Google Scholar] [CrossRef] [PubMed]





© 2017 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Dulhani, J.; Lee, B.J. Phonon Transport through Nanoscale Contact in Tip-Based Thermal Analysis of Nanomaterials. Nanomaterials 2017, 7, 200. https://doi.org/10.3390/nano7080200
Dulhani J, Lee BJ. Phonon Transport through Nanoscale Contact in Tip-Based Thermal Analysis of Nanomaterials. Nanomaterials. 2017; 7(8):200. https://doi.org/10.3390/nano7080200
Chicago/Turabian StyleDulhani, Jay, and Bong Jae Lee. 2017. "Phonon Transport through Nanoscale Contact in Tip-Based Thermal Analysis of Nanomaterials" Nanomaterials 7, no. 8: 200. https://doi.org/10.3390/nano7080200
APA StyleDulhani, J., & Lee, B. J. (2017). Phonon Transport through Nanoscale Contact in Tip-Based Thermal Analysis of Nanomaterials. Nanomaterials, 7(8), 200. https://doi.org/10.3390/nano7080200