Influence of Sputtering Parameters on Structural, Electrical and Thermoelectric Properties of Mg–Si Coatings
Abstract
:1. Introduction
2. Materials and Methods
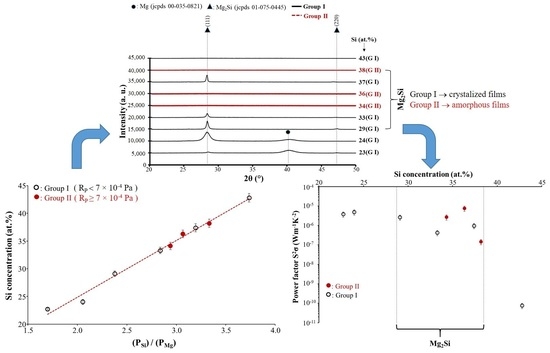
3. Results and Discussion
4. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Lopez, J.; Rios, R.; Bao, F.; Wang, G. Evolving privacy: From sensors to the Internet of Things. Future Gener. Comput. Syst. 2017, 75, 46–57. [Google Scholar] [CrossRef]
- Bello, O.; Zeadally, S. Toward efficient smartification of the Internet of Things (IoT) services. Future Gener. Comput. Syst. 2017, in press. [Google Scholar] [CrossRef]
- Guerrero-Ibanez, J.A.; Zeadally, S.; Contreras-Castillo, J. Integration challenges of intelligent transportation systems with connected vehicle, cloud computing, and internet of things technologies. IEEE Wirel. Commun. 2015, 22, 122–128. [Google Scholar] [CrossRef]
- Liu, Y.; Cui, J. Design and implementation of human health monitoring platform based on internet of things technology. In Proceedings of the 2017 IEEE International Conference on Computational Science and Engineering (CSE) and IEEE International Conference on Embedded and Ubiquitous Computing (EUC), Guangzhou, China, 21–24 July 2017; pp. 422–425. [Google Scholar]
- Iezzi, B.; Ankireddy, K.; Twiddy, J.; Losego, M.D.; Jur, J.S. Printed, metallic thermoelectric generators integrated with pipe insulation for powering wireless sensors. Appl. Energy 2017, 208, 758–765. [Google Scholar] [CrossRef]
- Selinger, M.; Sepulveda, A.; Buchan, J. Education and the Internet of Everything: How Ubiquitous Connectedness Can Help Transform Pedagogy; Cisco: San Jose, CA, USA, 2013. [Google Scholar]
- Iannacci, J. Microsystem based Energy Harvesting (EH-MEMS): Powering pervasivity of the Internet of Things (IoT)—A review with focus on mechanical vibrations. J. King Saud Univ. Sci. 2017, in press. [Google Scholar] [CrossRef]
- Nakagawa, K.; Suzuki, T. A High-Efficiency thermoelectric module with phase change material for IoT power supply. Proc. Eng. 2016, 168, 1630–1633. [Google Scholar] [CrossRef]
- He, W.; Zhang, G.; Zhang, X.; Ji, J.; Li, G.; Zhao, X. Recent development and application of thermoelectric generator and cooler. Appl. Energy 2015, 143, 1–25. [Google Scholar] [CrossRef]
- Hsu, K.F.; Loo, S.; Guo, F.; Chen, W.; Dyck, J.S.; Uher, C.; Hogan, T.; Polychroniadis, E.K.; Kanatzidis, M.G. Cubic AgPbmSbTe2+m: Bulk thermoelectric materials with figure of merit. Science 2004, 303, 818–821. [Google Scholar] [CrossRef] [PubMed]
- Biswas, K.; He, J.; Blum, I.D.; Wu, C.I.; Hogan, T.P.; Seidman, D.N.; Dravid, V.P.; Kanatzidis, M.G. High-performance bulk thermoelectrics with all-scale hierarchical architectures. Nature 2012, 489, 414–418. [Google Scholar] [CrossRef] [PubMed]
- Zhang, X.; Zhao, L. Thermoelectric materials: Energy conversion between heat and electricity. J. Materiomics 2015, 1, 92–105. [Google Scholar] [CrossRef]
- Poudel, B.; Hao, Q.; Ma, Y.; Lan, Y.; Minnich, A.; Yu, B.; Yan, X.; Wang, D.; Muto, A.; Vashaee, D.; Chen, X.; Liu, J.; Dresselhaus, M.S.; Chen, G.; Ren, Z. High-thermoelectric performance of nanostructured bismuth antimony telluride bulk alloys. Science 2008, 320, 634–638. [Google Scholar] [CrossRef] [PubMed]
- Shen, J.; Zhu, T.; Zhao, X.; Zhang, S.; Yang, S.; Yin, Z. Recrystallization induced in situ nanostructures in bulk bismuth antimony tellurides: A simple top down route and improved thermoelectric properties. Energy Environ. Sci. 2010, 3, 1519–1523. [Google Scholar] [CrossRef]
- Heremans, J.P.; Jovovic, V.; Toberer, E.S.; Saramat, A.; Kurosaki, K.; Charoenphakdee, A.; Yamanaka, S.; Snyder, G.J. Enhancement of thermoelectric efficiency in PbTe by distorsion of the electronic density of states. Science 2008, 321, 554–557. [Google Scholar] [CrossRef] [PubMed]
- Biswas, K.; He, J.; Zhang, Q.; Wang, G.; Uher, C.; Dravid, V.P.; Kanatzidis, M.G. Strained endotaxial nanostructures with high thermoelectric figure of merit. Nat. Chem. 2011, 3, 160–166. [Google Scholar] [CrossRef] [PubMed]
- Sun, X.; Gao, K.; Pang, X.; Yang, H.; Volinsky, A.A. Structure and composition effects on electrical and optical properties of sputtered PbSe thin films. Thin Solid Films 2015, 592, 59–68. [Google Scholar] [CrossRef]
- Daniel, M.V.; Friedemann, M.; Franke, J.; Albrecht, M. Thermal stability of thermoelectric CoSb3 skutterudite thin films. Thin Solid Films 2015, 589, 203–208. [Google Scholar] [CrossRef]
- Saiga, Y.; Du, B.; Deng, S.K.; Kajisa, K.; Takabatake, T. Thermoelectric properties of type-VIII clathrate Ba8Ga16Sn30 doped with Cu. J. Alloy. Compd. 2012, 537, 303–307. [Google Scholar] [CrossRef]
- Toberer, E.S.; Cox, C.A.; Brown, S.R.; Ikeda, T.; May, A.F.; Kauzlarich, S.M.; Snyder, G.J. Traversing the metal-insulator transition in a Zintl phase: Rational enhancement of thermoelectric efficiency in Yb14Mn1−xAlxSb11. Adv. Funct. Mater. 2008, 18, 2795–2800. [Google Scholar] [CrossRef]
- Yan, X.; Liu, W.; Wang, H.; Chen, S.; Shiomi, J.; Esfarjani, K.; Wang, H.; Wang, D.; Chen, G.; Ren, Z. Stronger phonon scattering by larger differences in atomic mass and size p-type half-Heuslers Hf1−xTixCoSb0.8Sn0.2. Energy Environ. Sci. 2012, 5, 7543–7548. [Google Scholar] [CrossRef]
- Chen, S.; Lukas, K.C.; Liu, W.; Opeil, C.P.; Chen, G.; Ren, Z. Effect of Hf concentration on thermoelectric properties of nanostructured n-type Half-Heusler materials HfxZr1-xNiSn0.99Sb0.01. Adv. Energy Mater. 2013, 3, 1210–1214. [Google Scholar] [CrossRef]
- Bashir, M.B.A.; Said, S.M.; Sabri, M.F.M.; Shnawah, D.A.; Elsheikh, M.H. Recent advances on Mg2Si1−xSnx materials for thermoelectric generation. Renew. Sustain. Energy Rev. 2014, 37, 569–584. [Google Scholar] [CrossRef]
- Prahoveanu, C.; Lacoste, A.; Bechu, S.; Vaulx, C.D.; Azzouz, K.; Laversenne, L. Investigation of Mg2(Si,Sn) thin films for integrated thermoelectric devices. J. Alloy. Compd. 2015, 649, 573–578. [Google Scholar] [CrossRef]
- Zhang, Q.; Zheng, Y.; Su, X.; Yin, K.; Tanga, X.; Uher, C. Enhanced power factor of Mg2Si0.3Sn0.7 synthesized by a non-equilibrium rapid solidification method. Scr. Mater. 2015, 96, 1–4. [Google Scholar] [CrossRef]
- Quoc, H.L.; Lacoste, A.; Béchu, S.; Bès, A.; Bourgault, D.; Fruchart, D. Deposition of thin films of Mg2Si1−xSnx solid solution by plasma-assisted co-sputtering. J. Alloy. Compd. 2012, 538, 73–78. [Google Scholar] [CrossRef]
- Murtaza, G.; Sajid, A.; Rizwan, M.; Takagiwa, Y.; Khachai, H.; Jibran, M.; Khenata, R.; Omran, S.B. First principles study of Mg2X (X = Si, Ge, Sn, Pb):Elastic, optoelectronic and thermoelectric properties. Mater. Sci. Semicond. Process. 2015, 40, 429–435. [Google Scholar] [CrossRef]
- Gao, H.; Zhu, T.; Zhao, X.; Deng, Y. Variations of thermoelectric properties of Mg2.2Si1-ySny-0.013Sb0.013 materials with different Si/Sn ratios. J. Solid State Chem. 2014, 220, 157–162. [Google Scholar] [CrossRef]
- Quoc, H.L.; Béchu, S.; Populoh, S.; Weidenkaff, A.; Lacoste, A. Thermoelectric properties of thin films of Sb doped Mg2Si1−xSnx solid solutions. J. Alloy. Compd. 2013, 546, 138–144. [Google Scholar] [CrossRef]
- Songa, R.B.; Aizawa, T.; Sun, J.Q. Synthesis of Mg2Si1−xSnx solid solutions as thermoelectric materials by bulk mechanical alloying and hot pressing. Mater. Sci. Eng. B Solid State Mater. 2007, 136, 111–117. [Google Scholar] [CrossRef]
- Hu, X.; Barnett, M.R.; Yamamoto, A. Synthesis of Al-doped Mg2Si1−xSnx compound using magnesium alloy for thermoelectric application. J. Alloy. Compd. 2015, 649, 1060–1065. [Google Scholar] [CrossRef]
- Liu, W.; Jie, Q.; Kim, H.S.; Ren, Z. Current progress and future challenges in thermoelectric power generation: From materials to devices. Acta Mater. 2015, 87, 357–376. [Google Scholar] [CrossRef] [Green Version]
- Kogut, I.; Record, M.C. Magnesium silicide thin film formation by reactive diffusion. Thin Solid Films 2012, 522, 149–158. [Google Scholar] [CrossRef]
- Serikawa, T.; Henmi, M.; Yamaguchi, T.; Oginuma, H.; Kondoh, K. Depositions and microstructures of Mg–Si thin film by ion beam sputtering. Surf. Coat. Technol. 2006, 200, 4233–4239. [Google Scholar] [CrossRef]
- Tani, J.I.; Kido, H. Electrical properties of Mg2Si thin films on flexible polyimide substrates fabricated by radio-frequency magnetron sputtering. J. Ceram. Soc. Jpn. 2015, 123, 298–301. [Google Scholar] [CrossRef]
- Prahoveanu, C.; Lacoste, A.; Vaulx, C.; Azzouz, K.; Salau, M.; Liu, Y.; Tainoff, D.; Bourgeois, O.; Laversenne, L. Effect of texture on the structural and transport properties of Sb-doped Mg2Si thin films. J. Alloy. Compd. 2016, 688, 195–201. [Google Scholar] [CrossRef]
- Ogawa, S.; Katagiri, A.T.; Shimizu, T.; Matsushima, M.; Akiyama, K.; Kimura, Y.; Uchida, H.; Funakubo, H. Electrical properties of (110)-oriented nondoped Mg2Si films with p-type conduction prepared by RF magnetron sputtering. J. Electron. Mater. 2014, 43, 2269–2273. [Google Scholar] [CrossRef]
- Creus, J.; Billard, A.; Sanchette, F. Corrosion behaviour of amorphous Al–Cr and Al–Cr–(N) coatings deposited by dc magnetron sputtering on mild steel substrate. Thin Solid Films. 2004, 466, 1–9. [Google Scholar] [CrossRef]
- Sanchette, F.; Loi, T.H.; Billard, A.; Frantz, C. Structure-properties relationship of metastable AI–Cr and AI–Ti alloys deposited by R.F. magnetron sputtering: Role of nitrogen. Surf. Coat. Technol. 1995, 74–75, 903–909. [Google Scholar] [CrossRef]
- Zhou, A.; Wang, W.; Yao, X.; Yang, B.; Li, J.; Zhao, Q.; Wang, C.; Xu, D.; Ziolkowski, P.; Mueller, E. Impact of the film thickness and substrate on the thermopower measurement of thermoelectric films by the potential-Seebeck microprobe (PSM). Appl. Therm. Eng. 2016, 107, 552–559. [Google Scholar] [CrossRef]
- Veprek, S.; Veprek-Heijman, M.G.J.; Karvankova, P.; Prochazka, J. Different approaches to superhard coatings and nanocomposites. Thin Solid Films 2005, 476, 1–29. [Google Scholar] [CrossRef]
- Yazdi, M.A.P.; Lomello, F.; Wang, J.; Sanchette, F.; Dong, Z.; White, T.; Wouters, Y.; Schuster, F.; Billard, A. Properties of TiSiN coatings deposited by hybrid HiPIMS and pulsed–DC magnetron co-sputtering. Vacuum 2014, 109, 43–51. [Google Scholar] [CrossRef]
- Kido, H. Thermoelectric properties of Bi-doped Mg2Si semiconductors. Phys. B Condens. Matter. 2005, 364, 218–224. [Google Scholar] [CrossRef]












| Target | Discharge Power (W) | Frequency (kHz) | Toff (µs) | Ar Flow Rate (sccm) | Working Pressure (Pa) | Run Duration (min) | Draw Distance (DT-S) (mm) | Residual Pressure, RP (Pa) | |
|---|---|---|---|---|---|---|---|---|---|
| Group I | Group II | ||||||||
| Si | 250–550 | 60 | 5 | 20 | 0.2 | 75 | 85 | RP < 7 × 10−4 | RP ≥ 7 × 10−4 |
| Mg | 146–150 | ||||||||
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Arab Pour Yazdi, M.; Martin, N.; Petitot, C.; Neffaa, K.; Palmino, F.; Cherioux, F.; Billard, A. Influence of Sputtering Parameters on Structural, Electrical and Thermoelectric Properties of Mg–Si Coatings. Coatings 2018, 8, 380. https://doi.org/10.3390/coatings8110380
Arab Pour Yazdi M, Martin N, Petitot C, Neffaa K, Palmino F, Cherioux F, Billard A. Influence of Sputtering Parameters on Structural, Electrical and Thermoelectric Properties of Mg–Si Coatings. Coatings. 2018; 8(11):380. https://doi.org/10.3390/coatings8110380
Chicago/Turabian StyleArab Pour Yazdi, Mohammad, Nicolas Martin, Christian Petitot, Khalid Neffaa, Frank Palmino, Frederic Cherioux, and Alain Billard. 2018. "Influence of Sputtering Parameters on Structural, Electrical and Thermoelectric Properties of Mg–Si Coatings" Coatings 8, no. 11: 380. https://doi.org/10.3390/coatings8110380
APA StyleArab Pour Yazdi, M., Martin, N., Petitot, C., Neffaa, K., Palmino, F., Cherioux, F., & Billard, A. (2018). Influence of Sputtering Parameters on Structural, Electrical and Thermoelectric Properties of Mg–Si Coatings. Coatings, 8(11), 380. https://doi.org/10.3390/coatings8110380