Research on the Sealing Mechanism of Split-Liner High-Pressure Hydrogen Storage Cylinders
Abstract
:1. Introduction
2. Experimental and Simulation Methods
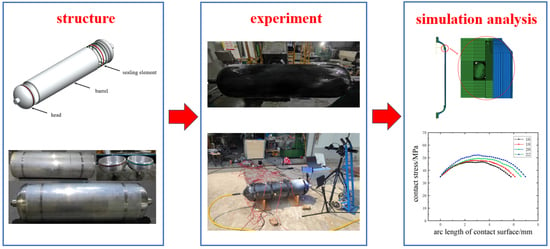
2.1. Designing the Structure
2.2. Preparation of Hydrogen Storage Cylinders
2.3. Experimental Methods
2.4. Finite Element Simulation
3. Results and Discussion
3.1. Accuracy of Finite Element Methods
3.2. Influence Analysis of Gas Cylinder Sealing Structure Parameters
3.2.1. Sealing of O-Rings at Different Compression Rates
3.2.2. Sealing of Different Distances from the Straight Edge Section to the Sealing Groove of the Head
3.2.3. Sealing of Different Head Thicknesses
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Aziz, G.; Sarwar, S.; Waheed, R.; Khan, M.S. Significance of Hydrogen Energy to Control the Environmental Gasses in Light of COP26: A Case of European Countries. Resour. Policy 2023, 80, 103240. [Google Scholar] [CrossRef]
- Lin, Y.; He, L.; Chen, T.; Zhou, D.; Wu, L.; Hou, X.; Zheng, C. Cost-Effective and Environmentally Friendly Synthesis of 3D Ni2P from Scrap Nickel for Highly Efficient Hydrogen Evolution in Both Acidic and Alkaline Media. J. Mater. Chem. A 2018, 6, 4088–4094. [Google Scholar] [CrossRef]
- Abdalla, A.M.; Hossain, S.; Nisfindy, O.B.; Azad, A.T.; Dawood, M.; Azad, A.K. Hydrogen Production, Storage, Transportation and Key Challenges with Applications: A Review. Energy Convers. Manag. 2018, 165, 602–627. [Google Scholar] [CrossRef]
- Muljadi, E.; Hossain, S. Powering Transportation with Hydrogen Fuel Cells [About This Issue]. IEEE Electrif. Mag. 2018, 6, 2–3. [Google Scholar] [CrossRef]
- Barthelemy, H.; Weber, M.; Barbier, F. Hydrogen Storage: Recent Improvements and Industrial Perspectives. Int. J. Hydrogen Energy 2017, 42, 7254–7262. [Google Scholar] [CrossRef]
- Moradi, R.; Groth, K.M. Hydrogen Storage and Delivery: Review of the State of the Art Technologies and Risk and Reliability Analysis. Int. J. Hydrogen Energy 2019, 44, 12254–12269. [Google Scholar] [CrossRef]
- Xia, Q.X.; Xie, S.W.; Huo, Y.L.; Ruan, F. Numerical Simulation and Experimental Research on the Multi-Pass Neck-Spinning of Non-Axisymmetric Offset Tube. J. Mater. Process. Technol. 2008, 206, 500–508. [Google Scholar] [CrossRef]
- Huang, C.-C.; Hung, J.-C.; Hung, C.; Lin, C.-R. Finite Element Analysis on Neck-Spinning Process of Tube at Elevated Temperature. Int. J. Adv. Manuf. Technol. 2011, 56, 1039–1048. [Google Scholar] [CrossRef]
- Pramod, R.; Krishnadasan, C.K.; Siva Shanmugam, N. Design and Finite Element Analysis of Metal-Elastomer Lined Composite over Wrapped Spherical Pressure Vessel. Compos. Struct. 2019, 224, 111028. [Google Scholar] [CrossRef]
- Zhou, C.; Zheng, J.; Gu, C.; Zhao, Y.; Liu, P. Sealing Performance Analysis of Rubber O-Ring in High-Pressure Gaseous Hydrogen Based on Finite Element Method. Int. J. Hydrogen Energy 2017, 42, 11996–12004. [Google Scholar] [CrossRef]
- Barboza Neto, E.S.; Chludzinski, M.; Roese, P.B.; Fonseca, J.S.O.; Amico, S.C.; Ferreira, C.A. Experimental and Numerical Analysis of a LLDPE/HDPE Liner for a Composite Pressure Vessel. Polym. Test. 2011, 30, 693–700. [Google Scholar] [CrossRef]
- Cho, S.-M.; Kim, K.-S.; Lee, S.-K.; Jung, G.-S.; Lee, S.-K.; Lyu, S.-K. Effect of Dome Curvature on Failure Mode of Type4 Composite Pressure Vessel. Int. J. Precis. Eng. Manuf. 2018, 19, 405–410. [Google Scholar] [CrossRef]
- Park, G.; Jang, H.; Kim, C. Design of Composite Layer and Liner for Structure Safety of Hydrogen Pressure Vessel (Type 4). J. Mech. Sci. Technol. 2021, 35, 3507–3517. [Google Scholar] [CrossRef]
- Zhu, J.; Li, Y.; Cao, W.; Li, Y.; Gao, Z. Failure Analysis of Novel BOSS Structures for Type IV Hydrogen Storage Vessels. Energies 2023, 16, 4005. [Google Scholar] [CrossRef]
- Zheng, Y.; Tan, Y.; Zhou, C.; Chen, G.; Li, J.; Liu, Y.; Liao, B.; Zhang, G. A Review on Effect of Hydrogen on Rubber Seals Used in the High-Pressure Hydrogen Infrastructure. Int. J. Hydrogen Energy 2020, 45, 23721–23738. [Google Scholar] [CrossRef]
- Zhou, C.; Chen, G.; Xiao, S.; Hua, Z.; Gu, C. Study on Fretting Behavior of Rubber O-Ring Seal in High-Pressure Gaseous Hydrogen. Int. J. Hydrogen Energy 2019, 44, 22569–22575. [Google Scholar] [CrossRef]
- Zhang, H.; Sun, Y.; Li, C.; Wang, H. Optimal Design of the Sealing Structure of a Hydraulic Cylinder on the Basis of a Surrogate Model. Adv. Mater. Sci. Eng. 2020, 2020, 1753964. [Google Scholar] [CrossRef]
- Li, Z. Study on the Sealing Performance of O-Ring under High-Pressure Environment. J. Phys. Conf. Ser. 2023, 2419, 012005. [Google Scholar] [CrossRef]
- Wu, E.; Zhao, Y.; Zhao, B.; Xu, W. Fatigue Life Prediction and Verification of High-Pressure Hydrogen Storage Vessel. Int. J. Hydrogen Energy 2021, 46, 30412–30422. [Google Scholar] [CrossRef]
- Son, D.-S.; Hong, J.-H.; Chang, S.-H. Determination of the Autofrettage Pressure and Estimation of Material Failures of a Type III Hydrogen Pressure Vessel by Using Finite Element Analysis. Int. J. Hydrogen Energy 2012, 37, 12771–12781. [Google Scholar] [CrossRef]
- Zu, L.; Xu, H.; Wang, H.; Zhang, B.; Zi, B. Design and Analysis of Filament-Wound Composite Pressure Vessels Based on Non-Geodesic Winding. Compos. Struct. 2019, 207, 41–52. [Google Scholar] [CrossRef]
- Hou, J.P.; Petrinic, N.; Ruiz, C.; Hallett, S.R. Prediction of Impact Damage in Composite Plates. Compos. Sci. Technol. 2000, 60, 273–281. [Google Scholar] [CrossRef]
- Hou, J.P.; Petrinic, N.; Ruiz, C. A Delamination Criterion for Laminated Composites under Low-Velocity Impact. Compos. Sci. Technol. 2001, 61, 2069–2074. [Google Scholar] [CrossRef]
- Liu, D.; Yun, F.; Jiao, K.; Wang, L.; Yan, Z.; Jia, P.; Wang, X.; Liu, W.; Hao, X.; Xu, X. Structural Analysis and Experimental Study on the Spherical Seal of a Subsea Connector Based on a Non-Standard O-Ring Seal. J. Mar. Sci. Eng. 2022, 10, 404. [Google Scholar] [CrossRef]
- Zhao, Y.; Feng, Z.; Li, Y.; Feng, L.; Li, Q.; Cui, W. Analysis of Contact Surface Wear Performance of O-Ring Dynamic Seal Based on Archard Model. Teh. Vjesn. 2022, 29, 281654. [Google Scholar] [CrossRef]
- He, S.; Peng, Y.; Qiu, S.; Du, X.; Jin, Y. Sealing Performance of Sealing Structure in a Sediment Sampler under Ultra-High Pressure. Sci. Rep. 2023, 13, 18548. [Google Scholar] [CrossRef]
- Windslow, R.J.; Busfield, J.J.C. Viscoelastic Modeling of Extrusion Damage in Elastomer Seals. Soft Mater. 2019, 17, 228–240. [Google Scholar] [CrossRef]



















| True Stress/MPa | Real Plastic Deformation |
|---|---|
| 296 | 0 |
| 330 | 0.0486 |
| Performance Parameters | Explanation | Numerical Value |
|---|---|---|
| E1/GPa | 1-direction (fiber direction) elastic modulus | 229 |
| E2&E3/GPa | 2-direction, 3-direction elastic modulus | 7.5 |
| u12&u13 | 12-direction, 13-direction Poisson’s ratio | 0.331 |
| u23 | 23-direction Poisson’s ratio | 0.36 |
| G12&G13/GPa | 12-direction, 13-direction shear modulus | 3.71 |
| G23/GPa | 23-direction shear modulus | 4.7 |
| XT/MPa | 1-direction tensile strength | 1345 |
| XC/MPa | 1-direction compressive strength | 1188 |
| YT&ZT/MPa | 2-, 3-direction tensile strength | 80 |
| YC&ZC/MPa | 2-, 3-direction compressive strength | 202 |
| S12&S13/MPa | 12-direction, 13-direction shear strength | 98 |
| S23/MPa | 23-direction shear strength | 76 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Tong, G.; Zhu, X.; Liu, Y.; Lv, F.; Lu, X. Research on the Sealing Mechanism of Split-Liner High-Pressure Hydrogen Storage Cylinders. Processes 2024, 12, 554. https://doi.org/10.3390/pr12030554
Tong G, Zhu X, Liu Y, Lv F, Lu X. Research on the Sealing Mechanism of Split-Liner High-Pressure Hydrogen Storage Cylinders. Processes. 2024; 12(3):554. https://doi.org/10.3390/pr12030554
Chicago/Turabian StyleTong, Guxing, Xiaolei Zhu, Yang Liu, Fuxiang Lv, and Xiaofeng Lu. 2024. "Research on the Sealing Mechanism of Split-Liner High-Pressure Hydrogen Storage Cylinders" Processes 12, no. 3: 554. https://doi.org/10.3390/pr12030554
APA StyleTong, G., Zhu, X., Liu, Y., Lv, F., & Lu, X. (2024). Research on the Sealing Mechanism of Split-Liner High-Pressure Hydrogen Storage Cylinders. Processes, 12(3), 554. https://doi.org/10.3390/pr12030554