Influence of β-Stabilizers on the α-Ti→ω-Ti Transformation in Ti-Based Alloys
Abstract
:1. Introduction
2. Materials and Methods
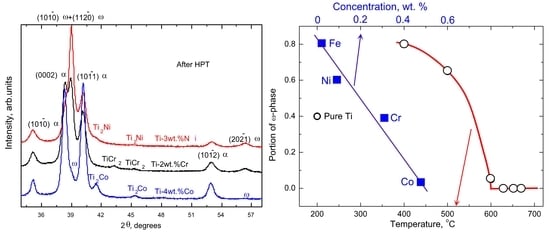
3. Results
4. Discussion
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Javanbakht, M.; Levitas, V.I. Phase field simulations of plastic strain-induced phase transformations under high pressure and large shear. Phys. Rev. B 2016, 94, 214104. [Google Scholar] [CrossRef] [Green Version]
- Feng, B.; Levitas, V.I. Plastic flows and strain-induced alpha to omega phase transformation in zirconium during compression in a diamond anvil cell: Finite element simulations. Mater. Sci. Eng. A 2017, 680, 130–140. [Google Scholar] [CrossRef] [Green Version]
- Pandey, K.K.; Levitas, V.I. In situ quantitative study of plastic strain-induced phase transformations under high pressure: Example for ultra-pure Zr. Acta Mater. 2020, 196, 338–346. [Google Scholar] [CrossRef]
- Usikov, M.P.; Zilberstein, V.A. The orientation relationship between the α- and ω- phases of titanium and zirconium. Phys. Stat. Sol. A 1973, 19, 53–58. [Google Scholar] [CrossRef]
- Trinkle, D.R.; Hennig, R.G.; Srinivasan, S.G.; Hatch, D.M.; Jones, M.D.; Stokes, H.T.; Albers, R.C.; Wilkins, J.W. New mechanism for the α to ω martensitic transformation in pure titanium. Phys. Rev. Lett. 2003, 91, 025701. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Jamieson, J.C. Crystal structures of titanium, zirconium, and hafnium at high pressures. Science 1963, 140, 72–73. [Google Scholar] [CrossRef] [PubMed]
- Sikka, S.K.; Vohra, Y.K.; Chidambaram, R. Omega phase in materials. Prog. Mater. Sci. 1982, 27, 245–310. [Google Scholar] [CrossRef]
- Feng, B.; Levitas, V.I.; Kamrani, M. Coupled strain-induced alpha to omega phase transformation and plastic flow in zirconium under high pressure torsion in a rotational diamond anvil cell. Mater. Sci. Eng. A 2018, 731, 623–633. [Google Scholar] [CrossRef] [Green Version]
- Zhang, J.; Zhao, Y.; Rigg, P.A.; Hixson, R.S.; Gray, G.T., III. Impurity effects on the phase transformations and equations of state of zirconium metals. J. Phys. Chem. Solids 2007, 68, 2297–2302. [Google Scholar] [CrossRef]
- Levitas, V.I. High pressure phase transformations revisited. J. Phys. Cond. Mat. 2018, 30, 163001. [Google Scholar] [CrossRef] [Green Version]
- Srinivasarao, B.; Zhilyaev, A.P.; Pérez-Prado, M.T. Orientation dependency of the α to Ω plus β transformation in commercially pure zirconium by high-pressure torsion. Scripta Mater. 2011, 65, 241–244. [Google Scholar] [CrossRef]
- Levitas, V.I.; Chen, H.; Xiong, L. Lattice instability during phase transformations under multiaxial stress: Modified transformation work criterion. Phys. Rev. B 2017, 96, 05411. [Google Scholar] [CrossRef] [Green Version]
- Zhang, J.; Zhao, Y.; Pantea, C.; Qian, J.; Rigg, P.; Hixson, R.; Gray, G.T.; Yang, Y.; Wang, L.; Wang, Y.; et al. Experimental constraints on the phase diagram of elemental zirconium. J. Phys. Chem. Solids 2005, 66, 1213–1219. [Google Scholar] [CrossRef]
- Kilmametov, A.R.; Khristoforov, A.V.; Wilde, G.; Valiev, R.Z. X-ray studies of nanostructured metals processed by severe plastic deformation. Zeits. Kryst. 2007, 2, 339–344. [Google Scholar] [CrossRef]
- Ivanisenko, Y.; Kilmametov, A.; Rösner, H.; Valiev, R.Z. Evidence of α to ω phase transition in titanium after high pressure torsion. Int. J. Mater. Res. 2008, 99, 36–41. [Google Scholar] [CrossRef]
- Mazilkin, A.; Straumal, B.; Kilmametov, A.; Straumal, P.; Baretzky, B. Phase transformations induced by severe plastic deformation. Mater. Trans. 2019, 60, 1489–1499. [Google Scholar] [CrossRef] [Green Version]
- Hennig, R.; Trinkle, D.R.; Bouchet, J.; Srinivasan, S.G.; Albers, R.C.; Wilkins, J.W. Impurities block the α to ω martensitic transformation in titanium. Nature Mater. 2005, 4, 129–133. [Google Scholar] [CrossRef] [Green Version]
- Afonikova, N.S.; Degtyareva, V.F.; Litvin, Y.A.; Rabinkin, A.G.; Skakov, Y.A. Formation of high-pressure ω-phase in titanium. Sov. Phys. Sol. State 1973, 15, 746–749. [Google Scholar]
- Dey, G.K.; Tewari, R.; Banerjee, S.; Jyoti, G.; Gupta, S.C.; Joshi, K.D.; Sikka, S.K. Formation of a shock deformation induced ω phase in Zr 20 Nb alloy. Acta Mater. 2004, 52, 5243–5254. [Google Scholar] [CrossRef]
- Kilmametov, A.; Ivanisenko, Yu.; Mazilkin, A.A.; Straumal, B.B.; Gornakova, A.S.; Fabrichnaya, O.B.; Kriegel, M.J.; Rafaja, D.; Hahn, H. The α→ω and β→ω phase transformations in Ti–Fe alloys under high-pressure torsion. Acta Mater. 2018, 144, 337–351. [Google Scholar] [CrossRef]
- Nash, P.; Choo, H.; Schwarz, R.B. Thermodynamic calculation of phase equilibria in the Ti–Co and Ni–Sn systems. J. Mater. Sci. 1998, 33, 4929–4936. [Google Scholar] [CrossRef]
- Murray, J. (Ed.) Phase Diagrams of Binary Titanium Alloys; ASM International: Metals Park, OH, USA, 1987; 59p. [Google Scholar]
- Murray, J. The Co-Ti (cobalt-titanium) system. Bull. Alloy Phase Diagr. 1982, 3, 74–86. [Google Scholar] [CrossRef]
- Wojdyr, M. Fityk: A general-purpose peak fitting program. J. Appl. Cryst. 2010, 43, 1126–1128. [Google Scholar] [CrossRef]
- Straumal, B.B.; Kilmametov, A.R.; Baretzky, B.; Kogtenkova, O.A.; Straumal, P.B.; Lityńska-Dobrzyńska, L.; Chulist, R.; Korneva, A.; Zięba, P. High pressure torsion of Cu–Ag and Cu–Sn alloys: Limits for solubility and dissolution. Acta Mater. 2020, 195, 184–198. [Google Scholar] [CrossRef]
- Straumal, B.B.; Korneva, A.; Kilmametov, A.R.; Lityńska-Dobrzyńska, L.; Gornakova, A.S.; Chulist, R.; Karpov, M.I.; Zięba, P. Structural and mechanical properties of Ti–Co alloys treated by the HPT. Materials 2019, 12, 426. [Google Scholar] [CrossRef] [Green Version]
- Korneva, A.; Straumal, B.; Kilmametov, A.; Gondek, Ł.; Wierzbicka-Miernik, A.; Lityńska-Dobrzyńska, L.; Chulist, R.; Cios, G.; Zięba, P. Thermal stability and microhardness of metastable ω-phase in the Ti-3.3at.%Co alloy subjected to HPT. J. Alloys Compd. 2020, 834, 155132. [Google Scholar] [CrossRef]
- Kilmametov, A.; Ivanisenko, Yu.; Straumal, B.B.; Mazilkin, A.A.; Gornakova, A.S.; Kriegel, M.J.; Fabrichnaya, O.B.; Rafaja, D.; Hahn, H. Transformations of α′ martensite in Ti–Fe alloys under HPT. Scr. Mater. 2017, 136, 46–49. [Google Scholar] [CrossRef]
- Pang, E.L.; Pickering, E.J.; Baik, S.I.; Seidman, D.N.; Jones, N.G. The effect of zirconium on the Ω phase in Ti-24Nb-[0–8.Zr] (at.%) alloys. Acta Mater. 2018, 153, 62–70. [Google Scholar] [CrossRef]
- Kolli, R.P.; Devaraj, A. A review of metastable beta titanium alloys. Metals 2018, 8, 506. [Google Scholar] [CrossRef] [Green Version]
- Xu, T.; Zhang, S.; Liang, S.; Cui, N.; Cao, L.; Wan, Y. Precipitation behaviour during the β → α/ω phase transformation and its effect on the mechanical performance of a Ti-15Mo-2.7Nb-3Al-0.2Si alloy. Sci. Rep. 2019, 9, 17628. [Google Scholar] [CrossRef]
- Babaei, H.; Levitas, V.I. Finite-strain scale-free phase-field approach to multivariant martensitic phase transformations with stress-dependent effective thresholds. J. Mech. Phys. Sol. 2020, 144, 104114. [Google Scholar] [CrossRef]
- Basak, A.; Levitas, V.I. An exact formulation for exponential-logarithmic transformation stretches in a multiphase phase field approach to martensitic transformations. Math. Mech. Sol. 2020, 25, 1219–1246. [Google Scholar] [CrossRef] [Green Version]
- Ehsan Esfahani, S.; Ghamarian, I.; Levitas, V.I. Strain-induced multivariant martensitic transformations: A scale-independent simulation of interaction between localized shear bands and microstructure. Acta Mater. 2020, 196, 430–443. [Google Scholar] [CrossRef]
- Basak, A.; Levitas, V.I. Matrix-precipitate interface-induced martensitic transformation within nanoscale phase field approach: Effect of energy and dimensionless interface width. Acta Mater. 2020, 189, 255–266. [Google Scholar] [CrossRef]
- Babaei, H.; Basak, A.; Levitas, V.I. Algorithmic aspects and finite element solutions for advanced phase field approach to martensitic phase transformation under large strains. Comput. Mech. 2019, 64, 1177–1197. [Google Scholar] [CrossRef]
- Basak, A.; Levitas, V.I. Nanoscale multiphase phase field approach for stress- and temperature-induced martensitic phase transformations with interfacial stresses at finite strains. J. Mech. Phys. Sol. 2018, 113, 162–196. [Google Scholar] [CrossRef] [Green Version]
- Basak, A.; Levitas, V.I. Finite element procedure and simulations for a multiphase phase field approach to martensitic phase transformations at large strains and with interfacial stresses. Comp. Meth. Appl. Mech. Eng. 2019, 343, 368–406. [Google Scholar] [CrossRef]
- Kilmametov, A.R.; Ivanisenko, Yu.; Straumal, B.B.; Gornakova, A.S.; Mazilkin, A.A.; Hahn, H. The α→ω transformation in titanium-cobalt alloys under high-pressure torsion. Metals 2018, 8, 1. [Google Scholar] [CrossRef] [Green Version]
- Straumal, B.; Kilmametov, A.; Gornakova, A.; Mazilkin, A.; Baretzky, B.; Korneva, A.; Zięba, P. Diffusive and displacive phase transformations in nanocomposites under high pressure torsion. Arch. Met. Mater. 2019, 64, 457–465. [Google Scholar]
- Babaei, H.; Levitas, V.I. Stress-measure dependence of phase transformation criterion under finite strains: Hierarchy of crystal lattice instabilities for homogeneous and heterogeneous transformations. Phys. Rev. Lett. 2020, 124, 075701. [Google Scholar] [CrossRef]
- Levitas, V.I. Phase field approach for stress- and temperature-induced phase transformations that satisfies lattice instability conditions. Part, I. General theory. Int. J. Plast. 2018, 106, 164–185. [Google Scholar] [CrossRef] [Green Version]
- Straumal, B.B.; Kilmametov, A.R.; Mazilkin, A.A.; Gornakova, A.S.; Fabrichnaya, O.B.; Kriegel, M.J.; Rafaja, D.; Bulatov, M.F.; Nekrasov, A.N.; Baretzky, B.; et al. The formation of the ω phase in the titanium-iron system under shear deformation. JETP Lett. 2020, 111, 568–574. [Google Scholar] [CrossRef]
- Levitas, V.I. High-pressure phase transformations under severe plastic deformation by torsion in rotational anvils. Mater. Trans. 2019, 60, 1294–1301. [Google Scholar] [CrossRef] [Green Version]
- Nakajima, H.; Ishioka, S.; Koiwa, M. Isotope effect for diffusion of cobalt in single-crystal α-titanium. Philos. Mag. A 1985, 52, 743–751. [Google Scholar] [CrossRef]



© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kilmametov, A.; Gornakova, A.; Karpov, M.; Afonikova, N.; Korneva, A.; Zięba, P.; Baretzky, B.; Straumal, B. Influence of β-Stabilizers on the α-Ti→ω-Ti Transformation in Ti-Based Alloys. Processes 2020, 8, 1135. https://doi.org/10.3390/pr8091135
Kilmametov A, Gornakova A, Karpov M, Afonikova N, Korneva A, Zięba P, Baretzky B, Straumal B. Influence of β-Stabilizers on the α-Ti→ω-Ti Transformation in Ti-Based Alloys. Processes. 2020; 8(9):1135. https://doi.org/10.3390/pr8091135
Chicago/Turabian StyleKilmametov, Askar, Alena Gornakova, Mikhail Karpov, Natalia Afonikova, Anna Korneva, Pawel Zięba, Brigitte Baretzky, and Boris Straumal. 2020. "Influence of β-Stabilizers on the α-Ti→ω-Ti Transformation in Ti-Based Alloys" Processes 8, no. 9: 1135. https://doi.org/10.3390/pr8091135
APA StyleKilmametov, A., Gornakova, A., Karpov, M., Afonikova, N., Korneva, A., Zięba, P., Baretzky, B., & Straumal, B. (2020). Influence of β-Stabilizers on the α-Ti→ω-Ti Transformation in Ti-Based Alloys. Processes, 8(9), 1135. https://doi.org/10.3390/pr8091135