Enhanced Methods to Estimate the Efficiency of Magnetic Nanoparticles in Imaging
Abstract
:1. Introduction
2. Results
2.1. Magnetic Characterization
2.1.1. Low-Field Susceptibility
2.1.2. Induced Magnetization
2.1.3. FORC Analysis
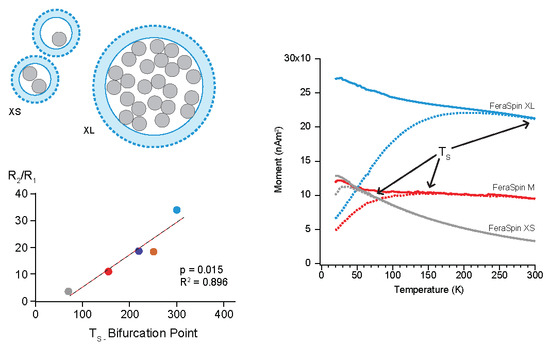
2.1.4. Zero-Field-Cooled and Field-Cooled Magnetization
2.1.5. AC Susceptibility
2.2. Imaging Performance
3. Discussion
4. Materials and Methods
5. Conclusions
Acknowledgments
Author Contributions
Conflicts of Interest
References
- Krishnan, K.M. Biomedical nanomagnetics: A spin through possibilities in imaging, diagnostics, and therapy. IEEE Trans. Magn. 2010, 46, 2523–2558. [Google Scholar] [CrossRef] [PubMed]
- Mornet, S.; Vasseur, S.; Grasset, F.; Duguet, E. Magnetic nanoparticle design for medical diagnosis and therapy. J. Mater. Chem. 2004, 14, 2161–2175. [Google Scholar] [CrossRef]
- Kolhatkar, A.G.; Jamison, A.C.; Litvinov, D.; Willson, R.C.; Lee, T.R. Tuning the magnetic properties of nanoparticles. Int. J. Mol. Sci. 2013, 14, 15977–16009. [Google Scholar] [CrossRef] [PubMed]
- Yoo, D.; Lee, J.H.; Shin, T.H.; Cheon, J. Theranostic magnetic nanoparticles. Acc. Chem. Res. 2011, 44, 863–874. [Google Scholar] [CrossRef] [PubMed]
- Brix, G.; Kolem, H.; Nitz, W.R.; Bock, M.; Huppertz, A.; Zech, C.J.; Dietrich, O. Basics of magnetic imaging and magnetic resonance spectroscopy. In Magnetic Resonance Tomography; Reiser, M.F., Semmler, W., Hricak, H., Eds.; Springer: Berlin, Germany, 2008; pp. 3–137. [Google Scholar]
- Ferguson, R.M.; Khandhar, A.P.; Arami, H.; Hua, L.; Hovorka, O.; Krishnan, K.M. Tailoring the magnetic and pharmacokinetic properties of iron oxide magnetic particle imaging tracers. Biomed. Tech./Biomed. Eng. 2013, 58, 493–507. [Google Scholar] [CrossRef] [PubMed]
- Ferguson, R.M.; Khandhar, A.P.; Kemp, S.J.; Arami, H.; Saritas, E.U.; Croft, L.R.; Konkle, J.; Goodwill, P.W.; Halkola, A.; Rahmer, J.; et al. Magnetic particle imaging with tailored iron oxide nanoparticle tracers. IEEE Trans. Med. Imaging 2015, 34, 1077–1084. [Google Scholar] [CrossRef] [PubMed]
- Hirt, A.M. Magneticmeasurements and characterization. In Iron Oxides: From Nature to Applications; Faivre, D., Ed.; Wiley: Weinheim, Germany, 2016; pp. 347–370. [Google Scholar]
- Heider, F.; Dunlop, D.J.; Sugiura, N. Magnetic properties of hydrothermally recrystallized magnetite crystals. Science 1987, 236, 1287–1290. [Google Scholar] [CrossRef] [PubMed]
- Maher, B.A. Magnetic properties of some synthetic sub-micron magnetites. Geophys. J. 1988, 94, 83–96. [Google Scholar] [CrossRef]
- Gundersen, H.G.; Bachgansmo, T.; Holtz, E.; Fahlvik, A.K.; Berg, A. Superparamagnetic contrast agents for magnetic-resonance-imaging. Investig. Radol. 1990, 25, S67–S68. [Google Scholar] [CrossRef]
- Ferguson, R.M.; Khandhar, A.P.; Krishnan, K.M. Tracer design for magnetic particle imaging (invited). J. Appl. Phys. 2012, 111, 07B318. [Google Scholar] [CrossRef] [PubMed]
- Khandhar, A.P.; Ferguson, R.M.; Arami, H.; Krishnan, K.M. Monodisperse magnetite nanoparticle tracers for in vivo magnetic particle imaging. Biomaterials 2013, 34, 3837–3845. [Google Scholar] [CrossRef] [PubMed]
- Lowa, N.; Knappe, P.; Wiekhorst, F.; Eberbeck, D.; Thunemann, A.F.; Trahms, L. Hydrodynamic and magnetic fractionation of superparamagnetic nanoparticles for magnetic particle imaging. J. Magn. Magn. Mater. 2015, 380, 266–270. [Google Scholar] [CrossRef]
- Panagiotopoulos, N.; Duschka, R.L.; Ahlborg, M.; Bringout, G.; Debbeler, C.; Graeser, M.; Kaethner, C.; Ludtke-Buzug, K.; Medimagh, H.; Stelzner, J.; et al. Magnetic particle imaging: Current developments and future directions. Int. J. Nanomed. 2015, 10, 3097. [Google Scholar] [CrossRef] [PubMed]
- Weizenecker, J.; Gleich, B.; Rahmer, J.; Borgert, J. Micro-magnetic simulation study on the magnetic particle imaging performance of anisotropic mono-domain particles. Phys. Med. Biol. 2012, 57, 7317–7327. [Google Scholar] [CrossRef] [PubMed]
- Ludwig, F.; Kuhlmann, C.; Wawrzik, T.; Dieckhoff, J.; Lak, A.; Kandhar, A.P.; Ferguson, R.M.; Kemp, S.J.; Krishnan, K.M. Dynamic magnetic properties of optimized magnetic nanoparticles for magnetic particle imaging. IEEE Trans. Magn. 2014, 50, 1–4. [Google Scholar] [CrossRef]
- Gehrke, N.; Heinke, D.; Eberbeck, D.; Ludwig, F.; Wawrzik, T.; Kuhlmann, C.; Briel, A. Magnetic characterization of clustered core magnetic nanoparticles for mpi. IEEE Trans. Magn. 2015, 51, 1–4. [Google Scholar] [CrossRef]
- Reimer, P.; Balzer, T. Ferucarbotran (resovist): A new clinically approved res-specific contrast agent for contrast-enhanced mri of the liver: Properties, clinical development, and applications. Eur. Radiol. 2003, 13, 1266–1276. [Google Scholar] [PubMed]
- Wetterskog, E.; Castro, A.; Zeng, L.; Petronis, S.; Heinke, D.; Olsson, E.; Nilsson, L.; Gehrke, N.; Svedlindh, P. Size and property bimodality in magnetic nanoparticle dispersions: Single domain particles vs. strongly coupled nanoclusters. Nanoscale 2017, 9, 4227–4235. [Google Scholar] [CrossRef] [PubMed]
- Ludwig, F.; Wawrzik, T.; Yoshida, T.; Gehrke, N.; Briel, A.; Eberbeck, D.; Schilling, M. Optimization of magnetic nanoparticles for magnetic particle imaging. IEEE Trans. Magn. 2012, 48, 3780–3783. [Google Scholar] [CrossRef]
- Gehrke, N.; Briel, A.; Ludwig, F.; Remmer, H.; Wawrzik, T.; Wellert, S. New perspectives for MPI: A toolbox for tracer research. In Magnetic Particle Imaging; Buzug, T.M., Borgert, J., Eds.; Springer: Berlin, Germany, 2012; Volume 140, pp. 99–103. [Google Scholar]
- Ludwig, F.; Remmer, H.; Kuhlmann, C.; Wawrzik, T.; Arami, H.; Ferguson, R.M.; Krishnan, K.M. Self-consistent magnetic properties of magnetite tracers optimized for magnetic particle imaging measured by ac susceptometry, magnetorelaxometry and magnetic particle spectroscopy. J. Magn. Magn. Mater. 2014, 360, 169–173. [Google Scholar] [CrossRef] [PubMed]
- Newell, A.J. A high-precsion model of first-order reversal curve (forc) functions for single-domain ferromagnetis with uniaxial anisotropy. Geochem. Geophys. Geosyst. 2005, 6. [Google Scholar] [CrossRef]
- Roberts, A.P.; Pike, C.R.; Verosub, K.L. First-order reversal curve diagrams: A new tool for characterizing the magnetic properties of natural samples. J. Geophys. Res. 2000, 105, 28461–28475. [Google Scholar] [CrossRef]
- Pike, C.R.; Roberts, A.P.; Dekkers, M.J.; Verosub, K.L. An investigation of multi-domain hysteresis mechanisms using forc diagrams. Phys. Earth Planet. Int. 2001, 126, 11–25. [Google Scholar] [CrossRef]
- Kumari, M.; Hirt, A.M.; Uebe, R.; Schuler, D.; Tompa, E.; Posfai, M.; Lorenz, W.; Ahrentorp, F.; Jonasson, C.; Johansson, C. Experimental mixtures of superparamagnetic and single-domain magnetite with respect to Day-Dunlop plots. Geochem. Geophys. Geosyst. 2015, 16, 1739–1752. [Google Scholar] [CrossRef]
- Egli, R.; Chen, A.P.; Winklhofer, M.; Kodama, K.P.; Horng, C.S. Detection of noninteracting single domain particles using first-order reversal curve diagrams. Geochem. Geophsy. Geosyst. 2010, 11. [Google Scholar] [CrossRef]
- Pike, C.R.; Roberts, A.P.; Verosub, K.L. Characterizing interactions in fine magnetic particle systems using first order reversal curves. J. Appl. Phys. 1999, 85, 6660–6667. [Google Scholar] [CrossRef]
- Hirt, A.M.; Sotiriou, G.A.; Kidambi, P.R.; Teleki, A. Effect of size, composition, and morphology on magnetic performance: First-order reversal curves evaluation of iron oxide nanoparticles. J. Appl. Phys. 2014, 115, 044314. [Google Scholar] [CrossRef]
- Muxworthy, A.; Williams, W. Magnetostatic interaction fields in first-order-reversal-curve diagrams. J. Appl. Phys. 2005, 97, 063905. [Google Scholar] [CrossRef] [Green Version]
- Morrish, A.H. Canted Antiferromagnetism: Hematite; World Scientific: Singapore, 1995. [Google Scholar]
- Morin, F.J. Magnetic susceptibility of α-Fe2O3 and α-Fe2O3 with added titanium. Phys. Rev. 1950, 78, 819–820. [Google Scholar] [CrossRef]
- Muench, G.J.; Arajs, S.; Matijevic, E. The Morin transition in small α-Fe2O3 particles. Phys. Status Solidi (A) 1985, 92, 187–192. [Google Scholar] [CrossRef]
- Kündig, W.; Bömmel, H.; Constabaris, G.; Lindquist, R.H. Some porperties of supported small α-Fe2O3 particles determined with Mössbauer effeect. Phys. Rev. 1966, 142, 327–333. [Google Scholar] [CrossRef]
- Özdemir, Ö.; Dunlop, D.J. The effect of oxidation on the verwey transition in magnetite. Geophys. Res. Lett. 1993, 20, 1671–1674. [Google Scholar] [CrossRef]
- Rochette, P.; Mathe, P.E.; Esteban, L.; Rakoto, H.; Bouchez, J.L.; Liu, Q.; Torrent, J. Non-saturation of the defect moment of goethite and fine-grained hematite up to 57 teslas. Geophys. Res. Lett. 2005, 32. [Google Scholar] [CrossRef]
- Khurshid, H.; Li, W.F.; Phan, M.H.; Mukherjee, P.; Hadjipanayis, G.C.; Srikanth, H. Surface spin disorder and exchange-bias in hollow maghemite nanoparticles. Appl. Phys. Lett. 2012, 101, 022403. [Google Scholar] [CrossRef]
- Yoshida, T.; Enpuku, K.; Ludwig, F.; Dieckhoff, J.; Wawrzik, T.; Lak, A.; Schilling, M. Characterization of resovist® nanoparticles for magnetic particle imaging. In Magnetic Particle Imaging; Buzug, T.M., Borgert, J., Eds.; Springer: Berlin, Germany, 2012; Volume 140, pp. 3–7. [Google Scholar]
- Kumari, M.; Widdrat, M.; Tompa, É.; Uebe, R.; Schueler, D.; Mihaly, P.; Faivre, D.; Hirt, A.M. Distinguishing magnetic particle size of iron oxide nanoparticles with first-order reversal curves. J. Appl. Phys. 2014, 116, 124304. [Google Scholar] [CrossRef]
- Shcherbakov, V.P.; Fabian, K. On the determination of magnetic grain-size distributions of superparamagnetic particle ensembles using the frequency dependence of susceptibility at different temperatures. Geophys. J. Int. 2005, 162, 736–746. [Google Scholar] [CrossRef]
- Worm, H.U.; Jackson, M. The superparamagnetism of yucca mountain tuff. J. Geophys. Res. 1999, 104, 25415–25425. [Google Scholar] [CrossRef]
- Schmidt, D.; Eberbeck, D.; Steinhoff, U.; Wiekhorst, F. Finding the magnetic size distribution of magnetic nanoparticles from magnetization measurements via the iterative Kaczmarz algorithm. J. Magn. Magn. Mater. 2017, 431, 33–37. [Google Scholar] [CrossRef]
- Ghasemi, A. Magnetic properties of substituted strontium ferrite nanoparticles and thin films. J. Magn. Magn. Mater. 2012, 324, 1375–1380. [Google Scholar] [CrossRef]
- Dormann, J.L.; Fiorani, D.; Tronc, E. On the models for interparticle interactions in nanoparticle assemblies: Comparison with experimental results. J. Magn. Magn. Mater. 1999, 202, 251–267. [Google Scholar] [CrossRef]
- Rohrer, M.; Bauer, H.; Mintorovitch, J.; Requardt, M.; Weinmann, H.-J. Comparison of magnetic properties of mri contrast media solutions at different magnetic field strengths. Investig. Radiol. 2005, 40, 715–724. [Google Scholar] [CrossRef]
- Ludwig, F.; Eberbeck, D.; Löwa, N.; Steinhoff, U.; Wawrzik, T.; Schilling, M.; Trahms, L. Characterization of magnetic nanoparticle systems with respect to their magnetic particle imaging performance. Biomed. Tech./Biomed. Eng. 2013, 58, 535–545. [Google Scholar] [CrossRef] [PubMed]
- Dearing, J.R.; Dann, R.J.L.; Hay, K.; Lees, J.A.; Loveland, P.J.; Maher, B.A.; O’Grady, K. Frequency-dependent susceptibility measurements of environmental materials. Geophys. J. Int. 1996, 124, 228–240. [Google Scholar] [CrossRef]
- Fidler, F.; Steinke, M.; Kraupner, A.; Gruttner, C.; Hiller, K.H.; Briel, A.; Westphal, F.; Walles, H.; Jakob, P.M. Stem cell vitality assessment using magnetic particle spectroscopy. IEEE Trans. Magn. 2015, 51, 1–4. [Google Scholar] [CrossRef]
- Reichel, V.; Kovacs, A.; Kumari, M.; Bereczk-Tompa, E.; Schneck, E.; Diehle, P.; Posfai, M.; Hirt, A.M.; Duchamp, M.; Dunin-Borkowski, R.E.; et al. Single crystalline superstructured stable single domain magnetite nanoparticles. Sci. Rep. 2017, 7, 45484. [Google Scholar] [CrossRef] [PubMed]
- Winklhofer, M.; Zimanyi, G.T. Extracting the intrinsic switching field distribution in perpendicular media: A comparative analysis. J. Appl. Phys. 2006, 99, 08E710. [Google Scholar] [CrossRef]
- Winklhofer, M.; Dumas, R.K.; Liu, K. Identifying reversible and irreversible magnetization changes in prototype patterned media using first- and second-order reversal curves. J. Appl. Phys. 2008, 103, 07C518. [Google Scholar] [CrossRef]
Sample Availability: Samples from the FeraSpinTMseries are available from nanoPET Pharma under www.viscover-online.de. |








| Sample Name | % Fraction of SP Particles (50 K) | % Fraction of SD Particles (50 K) | TS (K) | TB (K) |
|---|---|---|---|---|
| FeraSpin XS | - | - | 70 | 30 |
| FeraSpin M | 31 | 69 | 155 | 140 |
| FeraSpin XL | 25 | 75 | ca. 300 | 210 |
| FeraSpin R | 34 | 66 | 250 | 150 |
| Resovist | - | - | 220 | 90 |
| Sample Name | R1 1 | R2 1 | R2/R1 1 | MPI (Suspension) | MPI (Fixed) |
|---|---|---|---|---|---|
| FeraSpin XS | 13.0 | 49 | 3.8 | 4 | 4 |
| FeraSpin M | 9.9 | 117 | 11.2 | 3 | 1 |
| FeraSpin XL | 7.9 | 270 | 34.2 | 1 | 2 |
| FeraSpin R | 10.0 | 185 | 18.5 | 2 2 | 3 |
| Resovist | 9.3 | 174 | 18.7 | 2 2 | NA |
© 2017 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hirt, A.M.; Kumari, M.; Heinke, D.; Kraupner, A. Enhanced Methods to Estimate the Efficiency of Magnetic Nanoparticles in Imaging. Molecules 2017, 22, 2204. https://doi.org/10.3390/molecules22122204
Hirt AM, Kumari M, Heinke D, Kraupner A. Enhanced Methods to Estimate the Efficiency of Magnetic Nanoparticles in Imaging. Molecules. 2017; 22(12):2204. https://doi.org/10.3390/molecules22122204
Chicago/Turabian StyleHirt, Ann M., Monika Kumari, David Heinke, and Alexander Kraupner. 2017. "Enhanced Methods to Estimate the Efficiency of Magnetic Nanoparticles in Imaging" Molecules 22, no. 12: 2204. https://doi.org/10.3390/molecules22122204
APA StyleHirt, A. M., Kumari, M., Heinke, D., & Kraupner, A. (2017). Enhanced Methods to Estimate the Efficiency of Magnetic Nanoparticles in Imaging. Molecules, 22(12), 2204. https://doi.org/10.3390/molecules22122204