Activated Carbon from Agricultural Wastes for Adsorption of Organic Pollutants
Abstract
:1. Introduction
2. Methods and Calculation Procedures
2.1. Preparation of Activated Carbons
2.1.1. Preparation of Activated Carbon Using Chemical Activation
2.1.2. Preparation of Activated Carbon Using Physical Activation
2.1.3. Schematic Description of the Adsorbents Preparation Procedures
- Ac_Ar-strawberry seeds (precursor), chemical activation (acetic acid), pyrolysis process (600 °C, 2 h, argon atmosphere);
- CD_1 h-pistachio shells (precursor), pyrolysis process (400 °C, 1 h, and carbon dioxide atmosphere), physical activation (800 °C, 1 h, carbon dioxide atmosphere);
- CD_3 h-pistachio shells (precursor), pyrolysis process (400 °C, 1 h, and carbon dioxide atmosphere), physical activation (800 °C, 3 h, carbon dioxide atmosphere);
- ST_CD_1 h-pistachio shells (precursor), pyrolysis process (400 °C, 1 h, carbon dioxide atmosphere), physical activation (800 °C, 1 h, carbon dioxide, and water vapor atmosphere);
- ST_CD_3 h-pistachio shells (precursor), pyrolysis process (400 °C, 1 h, carbon dioxide atmosphere), physical activation (800 °C, 3 h, carbon dioxide, and water vapor atmosphere);
- CD_MV_1 h-pistachio shells (precursor), pyrolysis process (400 °C, 1 h, carbon dioxide atmosphere), first stage of physical activation (800 °C, 1 h, and carbon dioxide atmosphere), second stage of physical activation (microwave radiation, 1 h);
- CD_MV_1.5 h-pistachio shells (precursor), pyrolysis process (400 °C, 1 h, and carbon dioxide atmosphere), first stage of physical activation (800 °C, 1 h, and carbon dioxide atmosphere), second stage of physical activation (microwave radiation, 1.5 h).
2.2. Adsorbates
2.3. Methods of Investigations
2.3.1. Nitrogen Adsorption/Desorption Measurements
2.3.2. Small-Angle X-ray Scattering (SAXS)
2.3.3. Scanning Electron Microscopy/Transmission Electron Microscopy
2.3.4. Potentiometric Titration Measurements
2.3.5. X-ray Photoelectron Spectroscopy
2.3.6. Adsorption Equilibrium
2.3.7. Adsorption Kinetics
2.3.8. Thermal Analysis
3. Results and Discussion
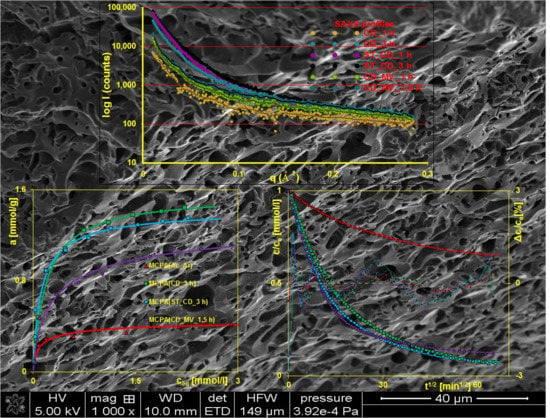
3.1. Characterization of the Adsorbents
3.2. Adsorption Equilibria
3.3. Adsorption Kinetics
3.4. Characterization of the Adsorbents after Adsorption
4. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Özsin, G.; Kılıç, M.; Apaydın-Varol, E.; Pütün, A.E. Chemically activated carbon production from agricultural waste of chickpea and its application for heavy metal adsorption: Equilibrium, kinetic, and thermodynamic studies. Appl. Water Sci. 2019, 9, 56. [Google Scholar] [CrossRef] [Green Version]
- Ghorbani, F.; Kamari, S.; Zamani, S.; Akbari, S.; Salehi, M. Optimization and modeling of aqueous Cr(VI) adsorption onto activated carbon prepared from sugar beet bagasse agricultural waste by application of response surface methodology. Surf. Interfaces 2020, 18, 100444. [Google Scholar] [CrossRef]
- Wan Ibrahim, W.M.H.; Mohamad Amini, M.H.; Sulaiman, N.S.; Kadir, W.R.A. Powdered activated carbon prepared from Leucaena leucocephala biomass for cadmium removal in water purification process. Arab. J. Basic Appl. Sci. 2019, 26, 30–40. [Google Scholar] [CrossRef] [Green Version]
- Borah, L.; Goswami, M.; Phukan, P. Adsorption of methylene blue and eosin yellow using porous carbon prepared from tea waste: Adsorption equilibrium, kinetics and thermodynamics study. J. Environ. Chem. Eng. 2015, 3, 1018–1028. [Google Scholar] [CrossRef]
- Abd-Elhamid, A.I.; Emran, M.; El-Sadek, M.H.; El-Shanshory, A.A.; Soliman, H.M.A.; Akl, M.A.; Rashad, M. Enhanced removal of cationic dye by eco-friendly activated biocharm derived from rice straw. Appl. Water Sci. 2020, 10, 45. [Google Scholar] [CrossRef] [Green Version]
- Lafi, R.; Montasser, I.; Hafiane, A. Adsorption of congo red dye from aqueous solutions by prepared activated carbon with oxygen-containing functional groups and its regeneration. Adsorpt. Sci. Technol. 2019, 37, 160–181. [Google Scholar] [CrossRef] [Green Version]
- Cansado, I.P.P.; Mourão, P.A.M.; Gomes, J.A.F.L.; Almodôvar, V. Adsorption of MCPA, 2,4-D and diuron onto activated carbons from wood composites. Ciencia e Tecnologia dos Materiais 2017, 29, e224–e228. [Google Scholar] [CrossRef]
- Ramamoorthy, M.; Ragupathy, S.; Sakthi, D.; Arun, V.; Kannadasan, N. Synthesis of SnO2 loaded on corn cob activated carbon for enhancing the photodegradation of methylene blue under sunlight irradiation. J. Environ. Chem. Eng. 2020, 8, 104331. [Google Scholar] [CrossRef]
- Muhammad, A.; ul Haq Ali Shah, A.; Bilal, S. Effective adsorption of hexavalent chromium and divalent nickel ions from water through polyaniline, iron oxide, and their composites. Appl. Sci. 2020, 10, 2882. [Google Scholar] [CrossRef] [Green Version]
- Ahmed, D.N.; Naji, L.A.; Faisal, A.A.H.; Al-Ansari, N.; Naushad, M. Waste foundry sand/MgFe-layered double hydroxides composite material for efficient removal of Congo red dye from aqueous solution. Sci. Rep. 2020, 10, 2042. [Google Scholar] [CrossRef]
- Naushad, M.; Sharma, G.; Alothman, Z.A. Photodegradation of toxic dye using Gum Arabic-crosslinked-poly(acrylamide)/Ni(OH)2/FeOOH nanocomposites hydrogel. J. Clean. Prod. 2019, 241, 118263. [Google Scholar] [CrossRef]
- Prasse, C.; Ford, B.; Nomura, D.K.; Sedlak, D.L. Unexpected transformation of dissolved phenols to toxic dicarbonyls by hydroxyl radicals and UV light. Proc. Natl. Acad. Sci. USA 2018, 115, 2311–2316. [Google Scholar] [CrossRef] [Green Version]
- Faisal, A.A.H.; Abdul-Kareem, M.B.; Mohammed, A.K.; Naushad, M.; Ghfar, A.A.; Ahamad, T. Humic acid coated sand as a novel sorbent in permeable reactive barrier for environmental remediation of groundwater polluted with copper and cadmium ions. J. Water Process Eng. 2020, 36, 101373. [Google Scholar] [CrossRef]
- Anitha, T.; Senthil Kumar, P.; Sathish Kumar, K. Synthesis of nano-sized chitosan blended polyvinyl alcohol for the removal of Eosin Yellow dye from aqueous solution. J. Water Process Eng. 2016, 13, 127–136. [Google Scholar] [CrossRef]
- Albadarin, A.B.; Collins, M.N.; Naushad, M.; Shirazian, S.; Walker, G.; Mangwandi, C. Activated lignin-chitosan extruded blends for efficient adsorption of methylene blue. Chem. Eng. J. 2017, 307, 264–272. [Google Scholar] [CrossRef] [Green Version]
- Al-Zaben, M.I.; Mekhamer, W.K. Removal of 4-chloro-2-methyl phenoxy acetic acid pesticide using coffee wastes from aqueous solution. Arabian, J. Chem. 2017, 10, S1523–S1529. [Google Scholar] [CrossRef] [Green Version]
- Açıkyıldız, M.; Gürses, A.; Karaca, S. Preparation and characterization of active carbon from plant wastes with chemical activation. Microporous Mesoporous Mater 2014, 198, 45–49. [Google Scholar] [CrossRef]
- Falco, C.; Marco-Lozar, J.P.; Salinas-Torres, D.; Morallón, E.; Cazorla-Amorós, D.; Titirici, M.M.; Lozano-Castelló, D. Tailoring the porosity of chemically active hydrothermal carbons: Influence of the precursor and hydrothermal carbonization temperature. Carbon 2013, 62, 46–55. [Google Scholar] [CrossRef] [Green Version]
- Arena, N.; Lee, J.; Clift, R. Life cycle assessment of active carbon production from coconut shells. J. Clean. Prod. 2016, 125, 68–77. [Google Scholar] [CrossRef] [Green Version]
- Demiral, I.; Atilgan, N.G.; Şensöz, S. Production of biofuel from soft shell of pistachio (Pistacia vera L.). Chem. Eng. Commun. 2009, 196, 104–115. [Google Scholar] [CrossRef]
- Çelik, I.; Demirer, G.N. Biogas production from pistachio (Pistacia vera L.) processing waste. Biocatal. Agric. Biotechnol. 2015, 4, 67–772. [Google Scholar] [CrossRef]
- Demirer, G.N. Biogas production from pistachio (Pistaciavera L.) de-hulling waste. Int. J. Green Energy 2016, 13, 1320–1324. [Google Scholar] [CrossRef]
- Taghizadeh-Alisaraei, A.; Assar, H.A.; Ghobadian, B.; Motevali, A. Potential of biofuel production from pistachio waste in Iran. Renew Sust. Energ. Rev. 2017, 72, 510–522. [Google Scholar] [CrossRef]
- Jaroniec, M.; Marczewski, A.W. Adsorption from solutions of nonelectrolytes on heterogeneous solid surfaces: A four-parameter equation for excess adsorption isotherm. Monatsh. Chem. 1984, 115, 541–550. [Google Scholar] [CrossRef]
- Marczewski, A.W.; Jaroniec, M. A new isotherm equation for single-solute adsorption from dilute solutions on energetically heterogeneous solids. Monatsh. Chem. 1983, 114, 711–715. [Google Scholar] [CrossRef]
- Blachnio, M.; Budnyak, T.M.; Derylo-Marczewska, A.; Marczewski, A.W.; Tertykh, V.A. Chitosan-silica hybrid composites for removal of sulfonated azo dyes from aqueous solutions. Langmuir 2018, 34, 2258–2273. [Google Scholar] [CrossRef] [PubMed]
- Derylo-Marczewska, A.; Blachnio, M.; Marczewski, A.W.; Seczkowska, M.; Tarasiuk, B. Phenoxyacid pesticide adsorption on activated carbon—Equilibrium and kinetics. Chemosphere 2019, 214, 349–360. [Google Scholar] [CrossRef]
- Derylo-Marczewska, A.; Miroslaw, K.; Marczewski, A.W.; Sternik, D. Studies of adsorption equilibria and kinetics of o-, m-, p-nitro- and chlorophenols on microporous carbons from aqueous solutions. Adsorption 2010, 16, 359–375. [Google Scholar] [CrossRef] [Green Version]
- Marczewski, A.W.; Derylo-Marczewska, A.; Slota, A. Adsorption and desorption kinetics of benzene derivatives on mesoporous carbons. Adsorption 2013, 19, 391–406. [Google Scholar] [CrossRef] [Green Version]
- Budnyak, T.M.; Błachnio, M.; Slabon, A.; Jaworski, A.; Tertykh, V.A.; Deryło-Marczewska, A.; Marczewski, A.W. Chitosan deposited onto fumed silica surface as sustainable hybrid biosorbent for Acid Orange 8 dye capture: Effect of temperature in adsorption equilibrium and kinetics. J. Phys. Chem. C 2020, 124, 15312–15323. [Google Scholar] [CrossRef]
- Marczewski, A.W. Analysis of kinetic Langmuir model. Part I: Integrated kinetic Langmuir equation (IKL): A new complete analytical solution of the Langmuir rate equation. Langmuir 2010, 26, 15229–15238. [Google Scholar] [CrossRef]
- Marczewski, A.W. Application of mixed order rate equations to adsorption of methylene blue on mesoporous carbons. Appl. Surf. Sci. 2010, 256, 5145–5152. [Google Scholar] [CrossRef]
- Derylo-Marczewska, A.; Blachnio, M.; Marczewski, A.W.; Swiatkowski, A.; Tarasiuk, B. Adsorption of selected herbicides from aqueous solutions on activated carbon. J. Therm. Anal. Calorim. 2010, 101, 785–794. [Google Scholar] [CrossRef] [Green Version]
- Derylo-Marczewska, A.; Marczewski, A.W.; Winter, S.; Sternik, D. Studies of adsorption equilibria and kinetics in the systems: Aqueous solution of dyes—Mesoporous carbons. Appl. Surf. Sci. 2010, 256, 5164–5170. [Google Scholar] [CrossRef]
- Liu, Y.; Shen, L. From Langmuir kinetics to first- and second-order rate equations for adsorption. Langmuir 2008, 24, 11625–11630. [Google Scholar] [CrossRef] [PubMed]
- Riahi, K.; Chaabane, S.; Thayer, B.B. A kinetic modeling study of phosphate adsorption onto Phoenix dactylifera L. date palm fibers in batch mode. J. Saudi Chem. Soc. 2017, 21, S143–S152. [Google Scholar] [CrossRef] [Green Version]
- Wu, F.-C.; Tseng, R.-L.; Juang, R.-S. Characteristics of Elovich equation used for the analysis of adsorption kinetics in dye-chitosan systems. Chem. Eng. J. 2009, 150, 366–373. [Google Scholar] [CrossRef]
- Brandt, A.; Bülow, M.; Derylo-Marczewska, A.; Goworek, J.; Schmeißer, J.; Schöps, W.; Unger, B. Novel zeolite composites and consequences for rapid sorption processes. Adsorption 2007, 13, 267–279. [Google Scholar] [CrossRef]
- Derylo-Marczewska, A.; Blachnio, M.; Marczewski, A.W.; Buczek, B.; Swiatkowski, A. Adsorption of chlorophenoxy pesticides on active carbon with gradually removed external particle layers. Chem. Eng. J. 2017, 308, 408–418. [Google Scholar] [CrossRef]
- Marczewski, A.W.; Seczkowska, M.; Derylo-Marczewska, A.; Blachnio, M. Adsorption equilibrium and kinetics of selected phenoxyacid pesticides on activated carbon—Effect of temperature. Adsorption 2016, 22, 777–790. [Google Scholar] [CrossRef] [Green Version]
- Puziy, A.M.; Poddubnaya, O.I.; Derylo Marczewska, A.; Marczewski, A.W.; Blachnio, M.; Tsyba, M.M.; Sapsay, V.I.; Klymchuk, D.O. Kinetics of protein adsorption by nanoporous carbons with different pore sizes. Adsorption 2016, 22, 541–552. [Google Scholar] [CrossRef]
- Porod, G. Die Röntgenkleinwinkelstreuung von dichtgepackten kolloiden Systemen. I. Teil Kolloid-Zeitschrift 1951, 124, 83–114. [Google Scholar] [CrossRef]
- Koberstein, J.T.; Morra, B.; Stein, R.S. The determination of diffuse-boundary thicknesses of polymers by small-angle X-ray scattering. J. Appl. Cryst. 1980, 13, 34–45. [Google Scholar] [CrossRef]
- Terzyk, A.P.; Wiśniewski, M.; Gauden, P.A.; Rychlicki, G.; Furmaniak, S. Carbon surface chemical composition in para-nitrophenol adsorption determined under real oxic and anoxic conditions. J. Colloid Interface Sci. 2008, 320, 40–51. [Google Scholar] [CrossRef]
- Czech, B.; Tyszczuk-Rotko, K. Visible-light-driven photocatalytic removal of acetaminophen from water using a novel MWCNT-TiO2-SiO2 photocatalysts. Sep. Purif. Technol. 2018, 206, 343–355. [Google Scholar] [CrossRef]
- Smith, M.W.; Garcia-Perez, M. Novel bio-char characterization strategies and their use to study the chemical and morphological evolution of material derived from xylan, cellulose and lignin. Engineering Conferences International Biochar: Production, Characterization and Applications Proceedings, University of Edinburgh, Edinburgh, UK Eds, ECI Symposium Series. 2017. Available online: http://dc.engconfintl.org/biochar/63 (accessed on 18 September 2020).
- Shafeeyan, M.S.; Daud, W.M.A.W.; Houshmand, A.; Shamiri, A. A review on surface modification of active carbon for carbon dioxide adsorption. J. Anal Appl. Pyrol. 2010, 89, 143–151. [Google Scholar] [CrossRef]
- Abdulrasheed, A.A.; Jalil, A.A.; Triwahyono, S.; Gambo, Y.; Ibrahim, M. Surface modification of activated carbon for adsorption of SO2 and NOX: A review of existing and emerging technologies. Renew Sust. Energ. Rev. 2018, 94, 1067–1085. [Google Scholar] [CrossRef]
- Szymański, G.S.; Karpiński, Z.; Biniak, S.; Świątkowski, A. The effect of the gradual thermal decomposition of surface oxygen species on the chemical and catalytic properties of oxidized activated carbon. Carbon 2002, 40, 2627–2639. [Google Scholar] [CrossRef]
- Nowicki, P.; Pietrzak, R. Activated carbons enriched with nitrogen—Preparation, properties and potential application. In Adsorbents and Catalysts, Selected Technologies and the Environment; Rzeszow University Publishing House: Rzeszów, Poland, 2012; pp. 129–144. [Google Scholar]
- Sternik, D.; Wiśniewska, M.; Nowicki, P. Thermal degradation of peat-based activated carbons covered with mixed adsorption layers of PAA polymer and SDS surfactant. Thermochim. Acta 2019, 676, 71–83. [Google Scholar] [CrossRef]


















| Adsorbate | Activated Carbon/Precursor | Monolayer Capacity1/Max. Sorption Capacity2 | Alternative Adsorbent | Monolayer Capacity1/Max. Sorption Capacity2 | Ref. |
|---|---|---|---|---|---|
| copper ions | activated carbon/chickpea waste | 56.2 mg/g2 | humic acid coated sand | 87.5 mg/g2 | [1,13] |
| chromium (VI) ions | activated carbon/sugar beet bagasse | 52.8 mg/g2 | Polyaniline/Fe3O4 composite | 174.09 mg/g2 | [2,9] |
| cadmium ions | activated carbon/Leucaena leucocephala biomass | 70.42 mg/g2 | humic acid coated sand | 18.9 mg/g2 | [3,13] |
| eosin yellow dye | activated carbon/tea waste | 400 mg/g2 | chitosan/PVA composite | 52.91 mg/g1 | [4,14] |
| methylene blue dye | activated biochar/rice straw | 90.91 mg/g2 | lignin/chitosan composite | 36.25 mg/g2 | [5,15] |
| congo red dye | activated carbon/coffee waste | 90.90 mg/g1 | sand/MgFe-layered double hydroxides composite | 9127.08 mg/g2 | [6,10] |
| MCPA | activated carbon/wood composites | 1.87 mmol/g2 | coffee waste | 0.34 g/g (1.69 mmol/g2) | [7,16] |
| methylene blue dye | activated carbon SnO2/corn cob | removal efficiency: 90.86% | Gum arabic-crosslinked-poly(acrylamide)/Ni(OH)2 /FeOOH | removal efficiency: 75% | [8,11] |
| Adsorbate Code | Chemical Formula | Molecular Weight [g/mol] | Ionization Constant pKa | Water Solubility [g/L] | Log Pow |
|---|---|---|---|---|---|
| MCPA |  | 200.6 | 3.07 1 | 0.825 2 | 2.32 3 |
| 2.4-D |  | 221.0 | 2.73 1 | 0.680 2 | 2.37 3 |
| CV |  | 407.9 | 0.8 4; 9.4 5 | 17 6 | 0.51 7 |
| Activated Carbon | SBET [m2/g] | Sext [m2/g] | Vt [cm3/g] | Vmic (T-Plot) [cm3/g] | Dmo (H-K) [nm] | pHpzc |
|---|---|---|---|---|---|---|
| Ac_Ar | 243 | 84 | 0.12 | 0.07 | 1.05 | 9.7 |
| CD_1 h | 599 | 114 | 0.27 | 0.20 | 0.50 | 11.4 |
| CD_3 h | 556 | 116 | 0.28 | 0.18 | 0.55 | 11.1 |
| ST_CD_1 h | 587 | 166 | 0.37 | 0.17 | 0.69 | 11 |
| ST_CD_3 h | 669 | 247 | 0.52 | 0.17 | 1.50 | 10.4 |
| CD_MV_1 h | 646 | 164 | 0.30 | 0.19 | 0.55 | 9.9 |
| CD_MV_1,5 h | 685 | 104 | 0.35 | 0.24 | 0.59 | 9.5 |
| Activated Carbon | kp | Porod Approximation | |
|---|---|---|---|
| , [Å−1] | SSAXS, [m2/g] | ||
| CD_1 h | 0.407 | 0.1302 | 620 |
| CD_3 h | 0.760 | 0.1344 | 592 |
| ST_CD_1 h | 0.939 | 0.1134 | 540 |
| ST_CD_3 h | 1.276 | 0.1155 | 550 |
| CD_MV_1 h | 0.525 | 0.1562 | 743 |
| CD_MV_1.5 h | 0.807 | 0.1621 | 771 |
| B.E. (eV) | Group | Ac_Ar | CD_3 h | ST_CD_3 h | CD_MV_1.5 h |
|---|---|---|---|---|---|
| C1s (%) | |||||
| 284.6–284.7 | C-C, C-H/sp3 | 27.4 | 30.9 | 31.0 | 26.3 |
| 284.2–284.3 | C=C/sp2 | 30.9 | 13.2 | 13.5 | 14.4 |
| 284.9–285 | C-C high | 16.4 | 28.2 | 28.6 | 24.7 |
| 285.5–285.7 | C-O-C, C-OH | 13.1 | 10.4 | 12.2 | 15.6 |
| 286.3–286.7 | C=O | 12.3 | 9.9 | 8.8 | 13.4 |
| 288–288.8 | COO | - | 7.4 | 5.9 | 4.4 |
| 290.2 | OCOO | - | - | - | 1.3 |
| O1s(%) | |||||
| 529.9–530.4 | O (oxides) | 4.2 | 5.4 | 1.3 | 8.7 |
| 531.3–532 | O=C | 23.3 | 29.9 | 30.4 | 42.0 |
| 532.3–533 | C-OH, C-O-C/aliph | 37.6 | 34.2 | 33.7 | 20.7 |
| 533.1–533.8 | C-OH, C-O-C/arom | 31.5 | 26.9 | 30 | 21.9 |
| 534.7–535.9 | H2O, O2/ads | 3.4 | 3.7 | 4.6 | 6.7 |
| Element (% at) | |||||
| carbon | 86.8 | 94.7 | 94.4 | 94.1 | |
| oxygen | 9.5 | 5.4 | 5.6 | 5.9 | |
| nitrogen | 2.5 | - | - | - | |
| phosphorous | 0.8 | - | - | - | |
| silicon | 0.4 | - | - | - | |
| System | Isotherm | am | m | n | Log K | R2 | SD(a) |
|---|---|---|---|---|---|---|---|
| MCPA (Ac_Ar) | T | 0.47 | 1 | 0.57 | 1.45 | 0.935 | 0.031 |
| MCPA (CD_3 h) | L | 1.56 | 1 | 1 | 0.72 | 0.992 | 0.046 |
| MCPA (ST_CD_3 h) | T | 1.44 | 1 | 0.85 | 1.01 | 0.961 | 0.103 |
| MCPA (CD_MV_1.5 h) | T | 1.43 | 1 | 0.58 | 0.91 | 0.976 | 0.062 |
| 2.4-D (CD_3 h)/ch | L | 1.57 | 1 | 1 | 0.83 | 0.992 | 0.050 |
| MCPA (CD_3 h)/ch | T/L | 1.56 | 1 | 0.99 | 0.76 | 0.994 | 0.044 |
| CV (ST_CD_3 h) | GF | 0.12 | 0.26 | 1 | 1.66 | 0.993 | 0.003 |
| Kinetic System | Fit | i | fi | log k* | t0.5 [min] | ueq | SD(c/co) | 1-R2 |
|---|---|---|---|---|---|---|---|---|
| MCPA (Ac_Ar) | Elovich | - | - | - | - | - | 0.35% | 1.1 × 10−3 |
| FOE | 2 | 0 | −2.73 | 371 | 0.31 | 1.91% | 3.4 × 10−2 | |
| SOE | 2 | 1 | −2.59 | 389 | 0.35 | 1.22% | 1.4 × 10−2 | |
| MOE | 2 | 0.99 | −7 | 389 | 0.35 | 1.24% | 1.4 × 10−2 | |
| m-exp | 1 | 0.11 | −1.02 | 7 | 0.36 | 0.21% | 3.4 × 10−4 | |
| 2 | 0.29 | −2.14 | 95 | |||||
| 3 | 0.60 | −3.16 | 1008 (av.342) | |||||
| MCPA (CD_3 h) | Elovich | - | - | - | - | - | 1.59% | 3.0 × 10−3 |
| FOE | 2 | 0 | −2.45 | 195 | 0.89 | 5.58% | 4 × 10−2 | |
| SOE | 2 | 1 | −2.25 | 179 | 0.97 | 2.97% | 1.1 × 10−2 | |
| MOE | 2 | 0.99 | −5.218 | 178 | 0.96 | 2.99% | 1.1 × 10−2 | |
| m-exp | 1 | 0.21 | −1.19 | 11 | 0.98 | 0.39% | 1.9 × 10−4 | |
| 2 | 0.39 | −2.18 | 104 | |||||
| 3 | 0.40 | −3.10 | 867 (av. 148) | |||||
| MCPA (ST_CD_3 h) | Elovich | - | - | - | - | - | 2.48% | 8.0 × 10−3 |
| FOE | 2 | 0 | −2.27 | 128 | 0.85 | 5.07% | 3.5 × 10−2 | |
| SOE | 2 | 1 | −2.07 | 117 | 0.91 | 2.59% | 8.9 × 10−3 | |
| MOE | 2 | 0.99 | −5.00 | 117 | 0.91 | 2.61% | 8.9 × 10−3 | |
| m-exp | 1 | 0.20 | −0.97 | 6 | 0.93 | 0.49% | 3.2 × 10−4 | |
| 2 | 0.44 | −2.01 | 72 | |||||
| 3 | 0.36 | −2.98 | 664 (av. 97) | |||||
| MCPA (CD_MV_1,5 h) | Elovich | - | - | - | - | - | 4.02% | 3.1 × 10−2 |
| FOE | 2 | 0 | −2.10 | 92 | 0.81 | 3.97% | 3 × 10−2 | |
| SOE | 2 | 1 | −1.90 | 79 | 0.87 | 1.35% | 3.4 × 10−3 | |
| MOE | 2 | 0.99 | −4.90 | 78 | 0.87 | 1.36% | 3.4 × 10−3 | |
| m-exp | 1 | 0.21 | −0.98 | 7 | 0.88 | 0.50% | 4.3 × 10−4 | |
| 2 | 0.55 | −2.02 | 72 | |||||
| 3 | 0.24 | −3.01 | 709 (av. 72) | |||||
| 2.4-D (CD_3 h)/ch | Elovich | - | - | - | - | - | 1.49% | 2.7 × 10−3 |
| FOE | 2 | 0 | −2.58 | 262 | 0.87 | 4.93% | 3.2 × 10−2 | |
| SOE | 2 | 1 | −2.39 | 247 | 0.95 | 2.82% | 1.0 × 10−2 | |
| MOE | 2 | 0.99 | −4.67 | 247 | 0.95 | 2.86% | 1.0 × 10−2 | |
| m-exp | 1 | 0.20 | −1.17 | 10 | 0.95 | 0.36% | 1.4 × 10−4 | |
| 2 | 0.37 | −2.26 | 126 | |||||
| 3 | 0.43 | −3.11 | 884 (av. 192) | |||||
| MCPA (CD_3 h)/ch | Elovich | - | - | - | - | - | 1.73% | 3.9 × 10−3 |
| FOE | 2 | 0 | −2.50 | 218 | 0.87 | 5.33% | 4.0 × 10−2 | |
| SOE | 2 | 1 | −2.27 | 188 | 0.93 | 2.94% | 1.1 × 10−2 | |
| MOE | 2 | 0.99 | −5.29 | 210 | 0.96 | 3.32% | 1.3 × 10−2 | |
| m-exp | 1 | 0.21 | −1.15 | 10 | 0.93 | 0.32% | 1.5 × 10−4 | |
| 2 | 0.38 | −2.15 | 98 | |||||
| 3 | 0.41 | −3.06 | 804 (av. 144) | |||||
| CV (ST_CD_3 h) | Elovich | - | - | - | - | - | 3.96% | 2.0 × 10−2 |
| FOE | 2 | 0 | −2.33 | 149 | 0.95 | 2.95% | 1.1 × 10−2 | |
| SOE | 2 | 1 | −2.09 | 122 | 1 | 2.63% | 9.6 × 10−3 | |
| MOE | 2 | 0.80 | −2.87 | 136 | 0.99 | 1.90% | 4.6 × 10−3 | |
| m-exp | 1 | 0.16 | 0.32 | 0.33 | 0.97 | 0.42% | 2.1 × 10−4 | |
| 2 | 0.20 | −1.66 | 32 | |||||
| 3 | 0.64 | −2.48 | 211 (av. 91) |
| ActivatedCarbon | SBET [m2/g] | Sext [m2/g] | Vt [cm3/g] | Vmic (t-plot) [cm3/g] | Dmo (H-K) [nm] |
|---|---|---|---|---|---|
| CD_3 h/before ads. | 556 | 116 | 0.28 | 0.18 | 0.55 |
| CD_3 h/after ads. | 115 | 67 | 0.12 | 0.02 | 4.40 |
| ST_CD_3 h/before ads. | 669 | 247 | 0.52 | 0.17 | 1.50 |
| ST_CD_3 h/after ads. | 230 | 209 | 0.25 | - | 8.49 |
Sample Availability: Samples of the activated carbons are available from the authors. | |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Blachnio, M.; Derylo-Marczewska, A.; Charmas, B.; Zienkiewicz-Strzalka, M.; Bogatyrov, V.; Galaburda, M. Activated Carbon from Agricultural Wastes for Adsorption of Organic Pollutants. Molecules 2020, 25, 5105. https://doi.org/10.3390/molecules25215105
Blachnio M, Derylo-Marczewska A, Charmas B, Zienkiewicz-Strzalka M, Bogatyrov V, Galaburda M. Activated Carbon from Agricultural Wastes for Adsorption of Organic Pollutants. Molecules. 2020; 25(21):5105. https://doi.org/10.3390/molecules25215105
Chicago/Turabian StyleBlachnio, Magdalena, Anna Derylo-Marczewska, Barbara Charmas, Malgorzata Zienkiewicz-Strzalka, Viktor Bogatyrov, and Mariia Galaburda. 2020. "Activated Carbon from Agricultural Wastes for Adsorption of Organic Pollutants" Molecules 25, no. 21: 5105. https://doi.org/10.3390/molecules25215105
APA StyleBlachnio, M., Derylo-Marczewska, A., Charmas, B., Zienkiewicz-Strzalka, M., Bogatyrov, V., & Galaburda, M. (2020). Activated Carbon from Agricultural Wastes for Adsorption of Organic Pollutants. Molecules, 25(21), 5105. https://doi.org/10.3390/molecules25215105