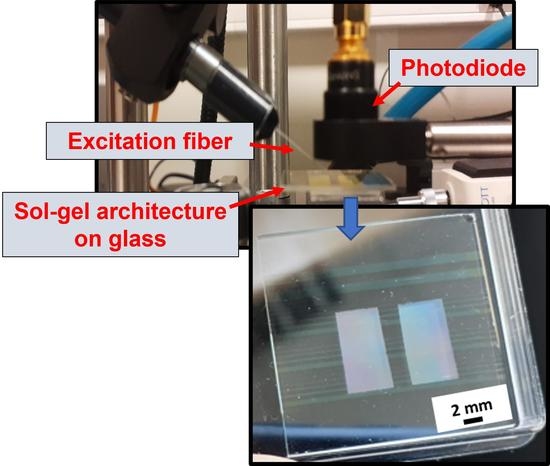
Study and Optimization of a Micro-Structured Waveguiding and Fluorescent Sol-Gel Architecture
Abstract
:1. Introduction
2. Results and Discussion
2.1. Optical Simulations
2.2. Channel Waveguide/Diffraction Grating Architecture Elaboration
2.2.1. Optimization of the Experimental Protocol
2.2.2. Complementary Optimization and Double Grating Architecture Elaboration
2.3. Double Grating Architecture Assessments
2.3.1. Preliminary Considerations
2.3.2. First Experimental Assessments
2.3.3. Fluorescence Measurements in Guided Configuration
2.3.4. Complementary Analyses and Fluorescence Measurements
3. Materials and Methods
3.1. Sol-Gel Processing
3.2. Micro-Structured Architecture Elaboration
3.3. Theoretical Analyses
3.4. Characterization
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Sample Availability
References
- Oubaha, M.; Gorin, A.; McDonagh, C.; Duffy, B.; Copperwhite, R. Development of a multianalyte optical sol-gel biosensor for medical diagnostic. Sens. Actuators 2015, 221, 96. [Google Scholar] [CrossRef]
- Liu, L.; Zhou, X.; Wilkinson, J.S.; Hua, P.; Song, B.; Shi, H. Integrated optical waveguide-based fluorescent immunosensor for fast and sensitive detection of microcystin-LR in lakes: Optimization and Analysis. Sci. Rep. 2017, 7, 3655. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Walter, J.-G.; Alwis, L.S.M.; Roth, B.; Bremer, K. All-optical planar waveguide-based biosensor chip designed for smartphone-assisted detection of vitamin D. Sensors 2020, 20, 6771. [Google Scholar] [CrossRef] [PubMed]
- Azuelos, P.; Girault, P.; Lorrain, N.; Poffo, L.; Guendouz, M.; Thual, M.; Lemaitre, J.; Pirasteh, P.; Hardy, I.; Charrier, J. High sensitivity optical sensor based on polymer materials and using the Vernier effect. Opt. Express 2017, 25, 30799. [Google Scholar] [CrossRef] [Green Version]
- Hajj-Hassan, M.; Gonzalez, T.; Ghafar-Zadeh, E.; Djeghelian, H.; Chodavarapu, V.; Andrews, M.; Therriault, D. Direct-dispense polymeric waveguides platform for optical chemical sensors. Sensors 2008, 8, 7636. [Google Scholar] [CrossRef] [Green Version]
- Ram, R.J.; Lee, K. Optical waveguides for microfluidic integration. In Proceedings of the 2009 IEEE LEOS Annual Meeting Conference Proceedings, Belek-Antalya, Turkey, 4–8 October 2009. [Google Scholar]
- Alberti, S.; Jagerska, J. Sol-gel thin film processing for integrated waveguide sensors. Front. Mater. 2021, 8, 629822. [Google Scholar] [CrossRef]
- Kazmierczak, A.; Butt, M.A.; Zieba, M.; Tyszkiewicz, C.; Karasinski, P.; Piramidowicz, R. Towards the most convenient configuration of integrated photonic sensor for implementation in SiO2:TiO2 sol-gel derived waveguide film technology. Proc. SPIE 2022, 12139, 1213908. [Google Scholar]
- Righini, G.C.; Armellini, C.; Ferrari, M.; Carlotto, A.; Carpentiero, A.; Chiappini, A.; Chiasera, A.; Lukowiak, A.; Tran, T.N.L.; Varas, S. Sol-Gel Photonic Glasses: From Material to Application. Materials 2023, 16, 2724. [Google Scholar] [CrossRef]
- Le, S.D.; Delcourt, E.; Girault, P.; Guttierez-Arroyo, A.; Azuelos, P.; Lorrain, N.; Bodiou, L.; Poffo, L.; Goujon, J.M.; Dumeige, Y.; et al. Study of optimized coupling based on micro-lensed fibers for fibers and photonic integrated circuits in the framework of telecommunication and sensing applications. Commun. Phys. 2016, 26, 325. [Google Scholar] [CrossRef] [Green Version]
- Dai, D.; Tang, Y.; Bowers, J.E. Mode conversion in tapered submicron silicon ridge optical waveguides. Opt. Express 2012, 20, 13425. [Google Scholar] [CrossRef]
- Cardenas, J.; Poitras, C.B.; Luke, K.; Luo, L.W.; Morton, P.A.; Lipson, M. High coupling efficiency etched facet tapers in silicon waveguides. IEEE Photonic Technol. Lett. 2014, 26, 2380. [Google Scholar] [CrossRef]
- Liu, J.; Raja, A.S.; Pfeiffer, M.H.P.; Herkommer, C.; Guo, H.; Zervas, M.; Geiselmann, M.; Kippenberg, T. Double inverse nanotapers for efficient light coupling to integrated photonic devices. Opt. Lett. 2018, 43, 3200. [Google Scholar] [CrossRef]
- Taillaert, D.; Van Laere, F.; Ayre, M.; Bogaerts, W.; Van Thourhout, D.; Bienstman, P.; Baets, R. Grating couplers for coupling between optical fibers. Jpn. J. Appl. Phys. 2006, 45, 6071. [Google Scholar] [CrossRef] [Green Version]
- Demeter, A.; Ruschin, S. Back-reflecting interferometric sensor based on grating coupler on a planar waveguide. J. Opt. 2016, 18, 015801. [Google Scholar] [CrossRef]
- Kuswandi, B.; Nuriman; Huskens, J.; Verboom, W. Optical sensing systems for microfluidic devices: A review. Anal. Chim. Acta 2007, 601, 141. [Google Scholar] [CrossRef] [PubMed]
- Lambeck, P.V. Integrated optical sensors for the chemical domain. Meas. Sci. Technol. 2006, 17, R93. [Google Scholar] [CrossRef]
- Mukundan, H.; Anderson, A.S.; Grace, W.K.; Grace, K.M.; Hartman, N.; Martinez, J.S.; Swanson, B.I. Waveguide-based biosensors for pathogen detection. Sensors 2009, 9, 5783. [Google Scholar] [CrossRef] [Green Version]
- Enami, Y. Fabricating 90 nm resolution structures in sol-gel silica optical waveguides for biosensor applications. J. Sens. 2017, 2017, 4198485. [Google Scholar] [CrossRef] [Green Version]
- Bonnel, M.; Riassetto, D.; Morand, A.; Bucci, D.; Langlet, M. Micro-structuration of a sol-gel architecture for channel waveguide/diffraction grating coupling. Opt. Mater. 2019, 92, 36. [Google Scholar] [CrossRef]
- Bonnel, M.; Marzouk, I.; Riassetto, D.; Morand, A.; Bucci, D.; Langlet, M. Setting up and assessing a new micro-structured waveguiding fluorescent architecture on glass entirely elaborated by sol–gel processing. Materials 2022, 15, 979. [Google Scholar] [CrossRef]
- Demuth, C.; Varonier, J.; Jossen, V.; Eibl, R.; Eibl, D. Novel probes for pH and dissolved oxygen measurements in cultivations from millilitre to benchtop scale. Appl. Microbiol. Biotechnol. 2016, 100, 3853. [Google Scholar] [CrossRef] [PubMed]
- Barczak, M.; McDonagh, C.; Wencel, D. Micro- and nanostructured sol-gel based materials for optical chemical sensing. Microchim. Acta 2016, 183, 2085. [Google Scholar] [CrossRef]
- Mills, A.; Graham, A.; O’Rourke, C. A novel titania sol-gel derived film for luminescence-based oxygen sensing. Sens. Actuators 2014, 190, 907. [Google Scholar] [CrossRef]
- Brinker, C.J.; Scherer, G.W. Sol-Gel Science—The Physics and Chemistry of Sol-Gel Processing; Academic Press Inc.: San Diego, CA, USA, 1990. [Google Scholar]
- Bornside, D.E.; Macosko, C.W.; Scriven, L.E. Modeling of spin coating. J. Imaging Technol. 1987, 13, 122. [Google Scholar]
- Hartmann, P.; Leiner, M.J.P.; Kohlbacher, P. Photobleaching of a ruthenium complex in polymers used for oxygen optodes and its inhibition by singlet oxygen quenchers. Sens. Actuators 1998, 51, 196. [Google Scholar] [CrossRef]
- Briche, S.; Tebby, Z.; Riassetto, D.; Messaoud, M.; Gamet, E.; Pernot, E.; Roussel, H.; Dellea, O.; Jourlin, Y.; Langlet, M. New insights in photo-patterned sol-gel derived TiO2 films. J. Mater. Sci. 2011, 46, 1474. [Google Scholar] [CrossRef]
- Tohge, N.; Shinmou, K.; Minami, T. Effects of UV-irradiation on the formation of oxide thin films from chemically modified metal-alkoxides. J. Sol.-Gel Sci. Technol. 1994, 2, 581. [Google Scholar] [CrossRef]
- Yariv, A.; Yeh, P. Photonics: Optical Electronic in Modern Communications, 6th ed.; Oxford University Press: New York, NY, USA, 2006. [Google Scholar]
- Hugonin, J.P.; Lalanne, P. Perfectly matched layers as nonlinear coordinate transforms: A generalized formalization. J. Opt. Soc. Am. A 2005, 22, 1844. [Google Scholar] [CrossRef]
- Bucci, D.; Martin, B.; Morand, A. Application of the three-dimensional aperiodic Fourier modal method using arc elements in curvilinear coordinates. J. Opt. Soc. Am. A 2012, 29, 367. [Google Scholar] [CrossRef]











| Rotation Duration (s) | Ti–BzAc Resist Thickness (nm)/Index | Waveguide Height (nm) | Grating Height (nm) |
|---|---|---|---|
| 1 | 250/1.75 | 250 | 250 |
| 30 | 160/1.75 | 160 | 160 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Marzouk, I.; Riassetto, D.; Morand, A.; Bucci, D.; Langlet, M. Study and Optimization of a Micro-Structured Waveguiding and Fluorescent Sol-Gel Architecture. Molecules 2023, 28, 4608. https://doi.org/10.3390/molecules28124608
Marzouk I, Riassetto D, Morand A, Bucci D, Langlet M. Study and Optimization of a Micro-Structured Waveguiding and Fluorescent Sol-Gel Architecture. Molecules. 2023; 28(12):4608. https://doi.org/10.3390/molecules28124608
Chicago/Turabian StyleMarzouk, Ibtihel, David Riassetto, Alain Morand, Davide Bucci, and Michel Langlet. 2023. "Study and Optimization of a Micro-Structured Waveguiding and Fluorescent Sol-Gel Architecture" Molecules 28, no. 12: 4608. https://doi.org/10.3390/molecules28124608
APA StyleMarzouk, I., Riassetto, D., Morand, A., Bucci, D., & Langlet, M. (2023). Study and Optimization of a Micro-Structured Waveguiding and Fluorescent Sol-Gel Architecture. Molecules, 28(12), 4608. https://doi.org/10.3390/molecules28124608