Strong Ferromagnetically-Coupled Spin Valve Sensor Devices for Droplet Magnetofluidics
Abstract
:1. Introduction
2. Experimental Section
2.1. Fabrication of Spin Valve Sensors
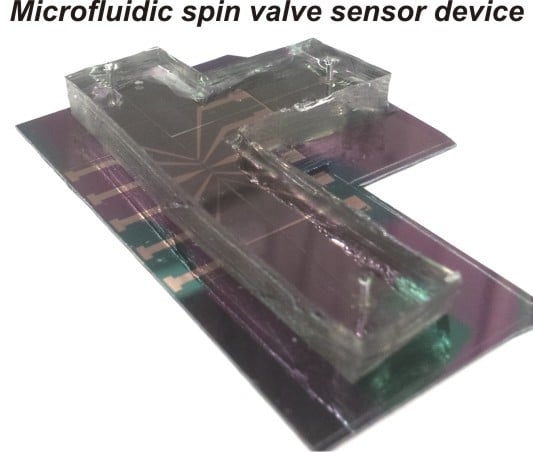
2.2. Assembly of Microfluidic Devices
2.3. On-Chip Formation of Ferrofluid Droplets
2.4. Magnetoelectrical Characterizations of Spin Valve Sensors
2.5. Real-Time Electrical Measurements of Droplets in Microfluidics
3. Results and Discussion
3.1. Optimization of Spin Valve Sensors


3.2. Dynamic Monitoring of Droplets in Microfluidics by Spin Valve Sensors


4. Conclusions
Acknowledgments
Author Contributions
Conflicts of Interest
References
- Mazutis, L.; Gilbert, J.; Ung, W.L.; Weitz, D.A.; Griffiths, A.D.; Heyman, J.A. Single-cell analysis and sorting using droplet-based microfluidics. Nat. Protoc. 2013, 8, 870–891. [Google Scholar] [CrossRef] [PubMed]
- Teste, B.; Ali-Cherif, A.; Viovy, J.L.; Malaquin, L. A low cost and high throughput magnetic bead-based immuno-agglutination assay in confined droplets. Lab Chip 2013, 13, 2344–2349. [Google Scholar] [CrossRef] [PubMed]
- Abate, A.R.; Hung, T.; Sperling, R.A.; Mary, P.; Rotem, A.; Agresti, J.J.; Weiner, M.A.; Weitz, D.A. DNA sequence analysis with droplet-based microfluidics. Lab Chip 2013, 13, 4864–4869. [Google Scholar] [CrossRef] [PubMed]
- Miller, O.J.; El Harrak, A.; Mangeat, T.; Baret, J.-C.; Frenz, L.; El Debs, B.; Mayot, E.; Samuels, M.L.; Rooney, E.K.; Dieu, P.; et al. High-resolution dose-response screening using droplet-based microfluidics. Proc. Natl. Acad. Sci. USA 2012, 109, 378–383. [Google Scholar] [CrossRef] [PubMed]
- Brouzes, E.; Medkova, M.; Savenelli, N.; Marran, D.; Twardowski, M.; Hutchison, J.B.; Rothberg, J.M.; Link, D.R.; Perrimon, N.; Samuels, M.L. Droplet microfluidic technology for single-cell high-throughput screening. Proc. Natl. Acad. Sci. USA 2009, 106, 14195–14200. [Google Scholar] [CrossRef] [PubMed]
- Nguyen, N.-T. Micro-magnetofluidics: Interactions between magnetism and fluid flow on the microscale. Microfluid. Nanofluidics 2011, 12, 1–16. [Google Scholar] [CrossRef]
- Misuk, V.; Mai, A.; Giannopoulos, K.; Alobaid, F.; Epple, B.; Loewe, H. Micro magnetofluidics: Droplet manipulation of double emulsions based on paramagnetic ionic liquids. Lab Chip 2013, 13, 4542–4548. [Google Scholar] [CrossRef] [PubMed]
- Sander, J.S.; Erb, R.M.; Denier, C.; Studart, A.R. Magnetic transport, mixing and release of cargo with tailored nanoliter droplets. Adv. Mater. 2012, 24, 2582–2587. [Google Scholar] [CrossRef] [PubMed]
- Nguyen, N.-T.; Ng, K.M.; Huang, X. Manipulation of ferrofluid droplets using planar coils. Appl. Phys. Lett. 2006, 89. [Google Scholar] [CrossRef]
- Kurtoğlu, E.; Bilgin, A.; Şeşen, M.; Mısırlıoğlu, B.; Yıldız, M.; Acar, H.F.Y.; Koşar, A. Ferrofluid actuation with varying magnetic fields for micropumping applications. Microfluid. Nanofluidics 2012, 13, 683–694. [Google Scholar] [CrossRef]
- Sabaté, R.; Barnadas-Rodríguez, R.; Callejas-Fernández, J.; Hidalgo-Alvarez, R.; Estelrich, J. Preparation and characterization of extruded magnetoliposomes. Int. J. Pharm. 2008, 347, 156–162. [Google Scholar] [CrossRef] [PubMed]
- Martina, M.-S.; Fortin, J.-P.; Ménager, C.; Clément, O.; Barratt, G.; Grabielle-Madelmont, C.; Gazeau, F.; Cabuil, V.; Lesieur, S. Generation of superparamagnetic liposomes revealed as highly efficient MRI contrast agents for in vivo imaging. J. Am. Chem. Soc. 2005, 127, 10676–10685. [Google Scholar] [CrossRef] [PubMed]
- Zhao, Y.; Xie, Z.; Gu, H.; Jin, L.; Zhao, X.; Wang, B.; Gu, Z. Multifunctional photonic crystal barcodes from microfluidics. NPG Asia Mater. 2012, 4. [Google Scholar] [CrossRef]
- Zhao, Y.; Shum, H.C.; Chen, H.; Adams, L.L.A.; Gu, Z.; Weitz, D.A. Microfluidic generation of multifunctional quantum dot barcode particles. J. Am. Chem. Soc. 2011, 133, 8790–8793. [Google Scholar] [CrossRef] [PubMed]
- Lin, G.; Makarov, D.; Medina-Sánchez, M.; Guix, M.; Baraban, L.; Cuniberti, G.; Schmidt, O.G. Magnetofluidic platform for multidimensional magnetic and optical barcoding of droplets. Lab Chip 2015, 15, 216–224. [Google Scholar] [CrossRef] [PubMed]
- Baselt, D.R.; Lee, G.U.; Natesan, M.; Metzger, S.W.; Sheehan, P.E.; Colton, R.J. A biosensor based on magnetoresistance technology. Biosens. Bioelectron. 1998, 13, 731–739. [Google Scholar] [CrossRef]
- Gaster, R.S.; Hall, D.A.; Wang, S.X. nanoLAB: An ultraportable, handheld diagnostic laboratory for global health. Lab Chip 2011, 11, 950–956. [Google Scholar] [CrossRef] [PubMed]
- Gaster, R.S.; Hall, D.A.; Nielsen, C.H.; Osterfeld, S.J.; Yu, H.; Mach, K.E.; Wilson, R.J.; Murmann, B.; Liao, J.C.; Gambhir, S.S.; et al. Matrix-insensitive protein assays push the limits of biosensors in medicine. Nat. Med. 2009, 15, 1327–1332. [Google Scholar] [CrossRef] [PubMed]
- Osterfeld, S.J.; Yu, H.; Gaster, R.S.; Caramuta, S.; Xu, L.; Han, S.-J.; Hall, D.A.; Wilson, R.J.; Sun, S.; White, R.L.; et al. Multiplex protein assays based on real-time magnetic nanotag sensing. Proc. Natl. Acad. Sci. USA 2008, 105, 20637–20640. [Google Scholar] [CrossRef] [PubMed]
- Loureiro, J.; Ferreira, R.; Cardoso, S.; Freitas, P.P.; Germano, J.; Fermon, C.; Arrias, G.; Pannetier-Lecoeur, M.; Rivadulla, F.; Rivas, J. Toward a magnetoresistive chip cytometer: Integrated detection of magnetic beads flowing at cm/s velocities in microfluidic channels. Appl. Phys. Lett. 2009, 95. [Google Scholar] [CrossRef]
- Fernandes, A.; Duarte, C.; Cardoso, F.; Bexiga, R.; Cardoso, S.; Freitas, P. Lab-on-Chip Cytometry Based on Magnetoresistive Sensors for Bacteria Detection in Milk. Sensors 2014, 14, 15496–15524. [Google Scholar] [CrossRef] [PubMed]
- Loureiro, J.; Andrade, P.Z.; Cardoso, S.; da Silva, C.L.; Cabral, J.M.; Freitas, P.P. Magnetoresistive chip cytometer. Lab Chip 2011, 11, 2255–2261. [Google Scholar] [CrossRef] [PubMed]
- Pekas, N.; Porter, M.D.; Tondra, M.; Popple, A.; Jander, A. Giant magnetoresistance monitoring of magnetic picodroplets in an integrated microfluidic system. Appl. Phys. Lett. 2004, 85. [Google Scholar] [CrossRef]
- Lin, G.; Makarov, D.; Melzer, M.; Si, W.; Yan, C.; Schmidt, O.G. A highly flexible and compact magnetoresistive analytic device. Lab Chip 2014, 14, 4050–4058. [Google Scholar] [CrossRef] [PubMed]
- Mönch, I.; Makarov, D.; Koseva, R.; Baraban, L.; Karnaushenko, D.; Kaiser, C.; Arndt, K.-F.; Schmidt, O.G. Rolled-up magnetic sensor: Nanomembrane architecture for in-flow detection of magnetic objects. ACS Nano 2011, 5, 7436–7442. [Google Scholar] [CrossRef] [PubMed]
- Fermon, C.; Pannetier-Lecoeur, M.; Biziere, N.; Cousin, B. Optimised GMR sensors for low and high frequencies applications. Sens. Actuators A Phys. 2006, 129, 203–206. [Google Scholar] [CrossRef]
- Buschow, K.H.J. Handbook of Magnetic Materials; Elsevier: Amsterdam, the Netherlands, 2003; Volume 15. [Google Scholar]
- Wang, S.X. Advances in Giant Magnetoresistance Biosensors With Magnetic Nanoparticle Tags: Review and Outlook. IEEE Trans. Magn. 2008, 44, 1687–1702. [Google Scholar] [CrossRef]
- Tamanaha, C.R.; Mulvaney, S.P.; Rife, J.C.; Whitman, L.J. Magnetic labeling, detection, and system integration. Biosens. Bioelectron. 2008, 24, 1–13. [Google Scholar] [CrossRef] [PubMed]
- Labrune, M.; Kools, J.C.S.; Thiaville, A. Magnetization rotation in spin-valve multilayers. J. Magn. Magn. Mater. 1997, 171, 1–15. [Google Scholar] [CrossRef]
- Parkin, S. Giant magnetoresistance in magnetic nanostructures. Annu. Rev. Mater. Sci. 1995, 25, 357–388. [Google Scholar] [CrossRef]
- Dieny, B. Giant magnetoresistance in spin-valve multilayers. J. Magn. Magn. Mater. 1994, 8853, 335–359. [Google Scholar] [CrossRef]
- Lee, C.-L.; Bain, J.A.; Chu, S.; McHenry, M.E. Separation of contributions to spin valve interlayer exchange coupling field by temperature dependent coupling field measurements. J. Appl. Phys. 2002, 91. [Google Scholar] [CrossRef]
- Teixeira, J.M.; Ventura, J.O.; Fermento, R.P.; Araujo, J.P.; Sousa, J.B.; Freitas, S.C.; Freitas, P.J. Interlayer Coupling and Magnetoresistance of MnIr-Based Spin Valves: Dependencies on Deposition Rate, Spacer Thickness, and Temperature. IEEE Trans. Magn. 2007, 43, 3143–3145. [Google Scholar] [CrossRef]
- Lin, G.; Baraban, L.; Han, L.; Karnaushenko, D.; Makarov, D.; Cuniberti, G.; Schmidt, O.G. Magnetoresistive emulsion analyzer. Sci. Rep. 2013, 3. [Google Scholar] [CrossRef] [PubMed]
- Lin, G.; Fomin, V.M.; Denys, M.; Schmidt, O.G. Supervised discriminant analysis for droplet-based micro-magnetofluidics. Microfluid. Nanofluidics 2015. [Google Scholar] [CrossRef]
- Zhu, G.-P.; Nguyen, N.-T.; Ramanujan, R.V.; Huang, X.-Y. Nonlinear deformation of a ferrofluid droplet in a uniform magnetic field. Langmuir 2011, 27, 14834–14841. [Google Scholar] [CrossRef] [PubMed]
© 2015 by the authors; licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lin, G.; Makarov, D.; Schmidt, O.G. Strong Ferromagnetically-Coupled Spin Valve Sensor Devices for Droplet Magnetofluidics. Sensors 2015, 15, 12526-12538. https://doi.org/10.3390/s150612526
Lin G, Makarov D, Schmidt OG. Strong Ferromagnetically-Coupled Spin Valve Sensor Devices for Droplet Magnetofluidics. Sensors. 2015; 15(6):12526-12538. https://doi.org/10.3390/s150612526
Chicago/Turabian StyleLin, Gungun, Denys Makarov, and Oliver G. Schmidt. 2015. "Strong Ferromagnetically-Coupled Spin Valve Sensor Devices for Droplet Magnetofluidics" Sensors 15, no. 6: 12526-12538. https://doi.org/10.3390/s150612526
APA StyleLin, G., Makarov, D., & Schmidt, O. G. (2015). Strong Ferromagnetically-Coupled Spin Valve Sensor Devices for Droplet Magnetofluidics. Sensors, 15(6), 12526-12538. https://doi.org/10.3390/s150612526