Relationship between Surface Properties and In Vitro Drug Release from a Compressed Matrix Containing an Amphiphilic Polymer Material
Abstract
:1. Introduction
2. Theoretical Considerations
2.1. Contact Angle and Wettability
2.2. Surface Free Energy
3. Materials and Methods
3.1. Materials
3.2. Obtaining and Characterization of PAM-18K Polymer
Preparation of Buffer Solutions
3.3. Methods
3.3.1. Granulometric Properties of Study Materials
3.3.2. Thermal Characterization of Polymer-Drug Blends
3.3.3. Preparation of the Compressed Matrices
3.3.4. Contact Angle Measurements
3.3.5. Determination of Surface Free Energy (SFE)
3.3.6. Water Absorption Rate
3.3.7. In vitro Dissolution Tests
3.3.8. Kinetic Study of the Model Drug Release
- (1)
- The zero order model: This model is widely used for pharmaceutical dosage systems that do not disintegrate and in which they have a very slow drug release. Furthermore, for this model it is assumed that the area of the tablet does not change significantly and material balance conditions are not formed. This model is expressed by the equation:where Qt is the amount of dissolved drug at time t, Q0 is the initial amount of drug in the solution (most of times Q0 = 0) and k0 corresponds to the constant of zero order release [57].
- (2)
- The first-order model: This model is commonly used to describe the absorption and release of water soluble drugs from porous matrices. However it is difficult to contextualize this mechanism to a theoretical basis. This model can be expressed by the equation:where Qt is the amount of dissolved drug at time t, Q0 is the initial amount of drug in the solution and k1 corresponds to the constant of first order release [56].
- (3)
- The Higuchi model: This model is widely used to describe the release of soluble and sparingly soluble drugs in aqueous media, from various semi-solid and/or solid matrices according to the equation:where kH is the Higuchi dissolution constant, while Qt and t correspond to the same parameters described previously [58].
- (4)
- The Korsmeyer–Peppas model: This is a generalized model of the Higuchi equation that allows one to explain drug delivery mechanisms where erosion and/or dissolution of the matrix occurs. This model has been widely used to describe the drug release from polymer systems. The related equation is:where Mt/M∞ corresponds to the fraction of drug released at time t; kr is the release constant which is characteristic for the polymer-drug interactions, while n is the diffusion exponent that is characteristic for the release mechanism. When n equals 0.5, the equation becomes equal to the Higuchi model, indicating that the release mechanism is of a Fickian type (case I), while values of n between 0.5 and 1.0 suggest that the release mechanism corresponds to an anomalous (non-Fickian) transport. Values of 1.0 indicate that the release mechanism is similar to a zero order release, while values of n greater than 1.0 (Super Case II transport), suggest a drug release process dependent of the relaxation of the polymer chains in the matrix, passing from a vitreous state (lower kinetic movement and increased potential energy) to a relaxed state rubber type (high kinetic movement and lower potential energy). For systems with a cylindrical matrix, the values of n are replaced by 0.45 instead of 0.5 and 0.89 instead of 1.0. The determination of n should be carried out only with a portion of drug release of 60% [56,59].
3.4. Data Processing and Analysis
4. Results and Discussion
4.1. Obtaining and Characterization of PAM-18K Polymer
4.2. Granulometric Properties of the Study Materials
4.3. Thermal Characterization of Polymer-Drug Mixtures
4.4. Preparation of the Compressed Matrices
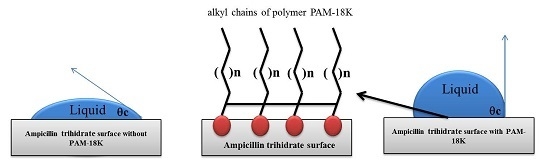
4.5. Contact Angle Measurements (θc)
4.6. Determination of Surface Free Energy (SFE)
4.7. Determination of Water Absorption Rate on the Tablets Surface
4.8. In vitro Dissolution Tests of the Model Drug
4.9. Kinetic Study of the Model Drug Release
5. Conclusions
Acknowledgments
Author Contributions
Conflicts of interest
References
- Allen, C.; Maysinger, D.; Eisenberg, A. Nano-engineering block copolymer aggregates for drug delivery. Colloids Surf. B Biointerfaces 1999, 16, 3–27. [Google Scholar] [CrossRef]
- Brudno, Y.; Mooney, D.J. On-demand drug delivery from local depots. J. Controll. Rel. 2015, 219, 8–17. [Google Scholar] [CrossRef] [PubMed]
- Peppas, N.A. Historical perspective on advanced drug delivery: How engineering design and mathematical modeling helped the field mature. Adv. Drug Deliv. Rev. 2013, 65, 5–9. [Google Scholar] [CrossRef] [PubMed]
- Zhang, H.; Zhai, Y.; Wang, J.; Zhai, G. New progress and prospects: The application of nanogel in drug delivery. Mater. Sci. Eng. C 2016, 60, 560–568. [Google Scholar] [CrossRef] [PubMed]
- Kassem, M.; Akbarieh, M.; Tawashi, R. Effect of fluid viscosity on the size and morphic features of salicylic acid during ball-mill grinding. Int. J. Pharm. 1988, 47, 179–183. [Google Scholar] [CrossRef]
- Mosig, J.; Kleinebudde, P. Evaluation of lubrication methods: How to generate a comparable lubrication for dry granules and powder material for tableting processes. Powder Technol. 2014, 266, 156–166. [Google Scholar] [CrossRef]
- Fürll, C.; Hoffmann, T. The influence of the granulometric condition on the flow characteristics of shredded grain products in their dependence on the duration of storage. Powder Technol. 2013, 235, 307–311. [Google Scholar] [CrossRef]
- Sander, A.; Kardum, J.P. Pentaerythritol crystallization—Influence of the process conditions on the granulometric properties of crystals. Adv. Powder Technol. 2012, 23, 191–198. [Google Scholar] [CrossRef]
- Zapata, C.; Frances, C.; Le Bolay, N.; Molina-Boisseau, S. Production of small composite particles by co-grinding in a media mill: Characterization of the granulometric and the mechanical properties. Chem. Eng. Res. Des. 2004, 82, 631–636. [Google Scholar] [CrossRef]
- Bacu, E.; Chitanu, G.C.; Couture, A.; Grandclaudon, P.; Singurel, G.; Carpov, A. Potential drug delivery systems from maleic anhydride copolymers and phenothiazine derivatives. Eur. Polym. J. 2002, 38, 1509–1513. [Google Scholar] [CrossRef]
- Bromberg, L. Chapter 7—Hydrophobically modified polyelectrolytes and polyelectrolyte block copolymers. In Handbook of Surfaces and Interfaces of Materials; Nalwa, H.S., Ed.; Academic Press: Burlington, MA, USA, 2001; pp. 369–404. [Google Scholar]
- Dhanya, S.; Bahadur, D.; Kundu, G.C.; Srivastava, R. Maleic acid incorporated poly-(n-isopropylacrylamide) polymer nanogels for dual-responsive delivery of doxorubicin hydrochloride. Eur. Polym. J. 2013, 49, 22–32. [Google Scholar] [CrossRef]
- Fredenberg, S.; Wahlgren, M.; Reslow, M.; Axelsson, A. The mechanisms of drug release in poly(lactic-co-glycolic acid)-based drug delivery systems—A review. Int. J. Pharm. 2011, 415, 34–52. [Google Scholar] [CrossRef] [PubMed]
- Kabanov, A.V.; Batrakova, E.V.; Miller, D.W. Pluronic® block copolymers as modulators of drug efflux transporter activity in the blood-brain barrier. Adv. Drug Deliv. Rev. 2003, 55, 151–164. [Google Scholar] [CrossRef]
- Lin, C.-C.; Metters, A.T. Hydrogels in controlled release formulations: Network design and mathematical modeling. Adv. Drug Deliv. Rev. 2006, 58, 1379–1408. [Google Scholar] [CrossRef] [PubMed]
- Tonge, S.R.; Tighe, B.J. Responsive hydrophobically associating polymers: A review of structure and properties. Adv. Drug Deliv. Rev. 2001, 53, 109–122. [Google Scholar] [CrossRef]
- Olea, A.F.; Martinez, A.B. Effect of hydrophobic bonding on the conformational transition and properties of intramolecular micelles formed by copolymers of maleic acid and styrene. J. Phys. Chem. B 1999, 103, 9306–9313. [Google Scholar] [CrossRef]
- Olea, A.F.; B, R.; Fuentes, I.; Aceve, B. Solubilization of phenols by intramolecular micelles formed by copolymers of maleic acid and olefins. Macromolecules 2002, 35, 1049–1053. [Google Scholar] [CrossRef]
- Angelova, N.; Yordanov, G. Nanoparticles of poly(styrene-co-maleic acid) as colloidal carriers for the anticancer drug epirubicin. Colloids Surf. A Physicochem. Eng. Asp. 2014, 452, 73–81. [Google Scholar] [CrossRef]
- Trivedi, B.C. Polymers and Copolymers of Maleic Anhydride; Chemical Abstracts: New York, NY, USA, 1982. [Google Scholar]
- Finne, U.; Kyyrönen, K.; Urtti, A. Drug release from monoisopropyl ester of poly(vinyl methyl ether-maleic anhydride) can be modified by basic salts in the polymer matrix. J. Controll. Rel. 1989, 10, 189–194. [Google Scholar] [CrossRef]
- Şen, M.; Uzun, C.; Güven, O. Controlled release of terbinafine hydrochloride from ph sensitive poly(acrylamide/maleic acid) hydrogels. Int. J. Pharm. 2000, 203, 149–157. [Google Scholar] [CrossRef]
- Nita, L.E.; Chiriac, A.P.; Mititelu-Tartau, L.; Stoleru, E.; Doroftei, F.; Diaconu, A. Patterning poly(maleic anhydride-co-3,9-divinyl-2,4,8,10-tetraoxaspiro (5.5) undecane) copolymer bioconjugates for controlled release of drugs. Int. J. Pharm. 2015, 493, 328–340. [Google Scholar] [CrossRef] [PubMed]
- Chiriac, A.P.; Nita, L.E.; Tudorachi, N.; Neamtu, I.; Balan, V.; Tartau, L. Upon synthesis of a polymeric matrix with ph and temperature responsiveness and antioxidant bioactivity based on poly(maleic anhydride-co-3,9-divinyl-2,4,8,10-tetraoxaspiro [5.5] undecane) derivatives. Mater. Sci. Eng. C 2015, 50, 348–357. [Google Scholar] [CrossRef] [PubMed]
- Salamanca, C.H.; Barraza, R.G.; Acevedo, B.; Olea, A.F. Hydrophobically modified polyelectrolytes as potential drugs reservoirs of n-alkyl-nitroimidazoles. J. Chil. Chem. Soc. 2007, 52. [Google Scholar] [CrossRef]
- Salamanca, C.; Quintero, A.; Pineda, D.; Andrade, A. Aggregates of alternate amphiphilc polyanion to carry zwitterionic drug in aqueous media. Int. J. Pharm. Sci. Res. 2015, 6, 2360–2366. [Google Scholar]
- Gamboa, C.; Olea, A.F. Association of cationic surfactants to humic acid: Effect on the surface activity. Colloids Surf. A Physicochem. Eng. Asp. 2006, 278, 241–245. [Google Scholar] [CrossRef]
- Olea, A.F.; Thomas, J.K. Fluorescence studies of the conformational changes of poly(methacrylic acid) with ph. Macromolecules 1989, 22, 1165–1169. [Google Scholar] [CrossRef]
- Olea, A.F.; Rosenbluth, H.; Thomas, J.K. Effect of themolecular weight on the dynamics of the conformationaltransition of poly(metahcrylic acid). Macromolecules 1999, 32, 8077–8083. [Google Scholar] [CrossRef]
- Saurí, J.; Suñé-Negre, J.M.; Díaz-Marcos, J.; Vilana, J.; Millán, D.; Ticó, J.R.; Miñarro, M.; Pérez-Lozano, P.; García-Montoya, E. Relationships between surface free energy, surface texture parameters and controlled drug release in hydrophilic matrices. Int. J. Pharm. 2015, 478, 328–340. [Google Scholar] [CrossRef] [PubMed]
- Lungan, M.-A.; Popa, M.; Racovita, S.; Hitruc, G.; Doroftei, F.; Desbrieres, J.; Vasiliu, S. Surface characterization and drug release from porous microparticles based on methacrylic monomers and xanthan. Carbohydr. Polym. 2015, 125, 323–333. [Google Scholar] [CrossRef] [PubMed]
- Kwok, D.Y.; Neumann, A.W. Contact angle measurement and contact angle interpretation. Adv. Colloid Interf. Sci. 1999, 81, 167–249. [Google Scholar] [CrossRef]
- Marmur, A. Equilibrium contact angles: Theory and measurement. Colloids Surf. A Physicochem. Eng. Asp. 1996, 116, 55–61. [Google Scholar] [CrossRef]
- Gennes, P.G.d. Wetting: Static and dynamics. Rev. Mod. Phys. 1985, 57, 827–863. [Google Scholar] [CrossRef]
- Hejazi, I.; Sadeghi, G.M.M.; Jafari, S.H.; Khonakdar, H.A.; Seyfi, J.; Holzschuh, M.; Simon, F. Transforming an intrinsically hydrophilic polymer to a robust self-cleaning superhydrophobic coating via carbon nanotube surface embedding. Mater. Des. 2015, 86, 338–346. [Google Scholar] [CrossRef]
- Lee, M.; Lee, S.; Yim, C.; Jeon, S. Surface wetting of superhydrophobic aluminum oxide nanostructures investigated using the fiber-optic spectrometer and quartz crystal microbalance. Sens. Actuators B Chem. 2015, 220, 799–804. [Google Scholar] [CrossRef]
- Nosonovsky, M.; Bhushan, B. Hierarchical roughness optimization for biomimetic superhydrophobic surfaces. Ultramicroscopy 2007, 107, 969–979. [Google Scholar] [CrossRef] [PubMed]
- Zhang, H.; Yang, J.; Chen, B.; Liu, C.; Zhang, M.; Li, C. Fabrication of superhydrophobic textured steel surface for anti-corrosion and tribological properties. Appl. Surf. Sci. 2015, 359, 905–910. [Google Scholar] [CrossRef]
- Lunkenheimer, K.; Wantke, K.D. On the applicability of the du nouy (ring) tensiometer method for the determination of surface tensions of surfactant solutions. J. Colloid Interf. Sci. 1978, 66, 579–581. [Google Scholar] [CrossRef]
- Nouy, P.L.D. A new apparatus for measuring surface tension. J. Gen. Physiol. 1919, 1, 521–524. [Google Scholar] [CrossRef] [PubMed]
- Zettlemoyer, A.C.; Subba Rao, V.V. Capabilities of the du noüy tensiometer. J. Colloid Interf. Sci. 1969, 29, 172. [Google Scholar] [CrossRef]
- Heertjes, P.M.; de Smet, E.C.; Witvoet, W.C. The determination of interfacial tensions with the wilhelmy plate method. Chem. Eng. Sci. 1971, 26, 1479–1480. [Google Scholar] [CrossRef]
- Pike, F.P.; Thakkar, C.R. Interfacial tension measurement by an improved wilhelmy technique. In Colloid and Interface Science; Kerker, M., Ed.; Academic Press: New York, NY, USA, 1976; pp. 375–402. [Google Scholar]
- Ferrero, F. Wettability measurements on plasma treated synthetic fabrics by capillary rise method. Polym. Test. 2003, 22, 571–578. [Google Scholar] [CrossRef]
- Susana, L.; Campaci, F.; Santomaso, A.C. Wettability of mineral and metallic powders: Applicability and limitations of sessile drop method and washburn’s technique. Powder Technol. 2012, 226, 68–77. [Google Scholar] [CrossRef]
- Amirfazli, A.; Neumann, A.W. Status of the three-phase line tension: A review. Adv. Colloid Interf. Sci. 2004, 110, 121–141. [Google Scholar] [CrossRef] [PubMed]
- Tavana, H.; Lam, C.N.C.; Grundke, K.; Friedel, P.; Kwok, D.Y.; Hair, M.L.; Neumann, A.W. Contact angle measurements with liquids consisting of bulky molecules. J. Colloid Interf. Sci. 2004, 279, 493–502. [Google Scholar] [CrossRef] [PubMed]
- Van Oss, C.J.; Busscher, I.H.J. Applied Surface Thermodynamics; Neumann, A.W., David, R., Zuo, Y., Eds.; CRC Press: Boca Raton, FL, USA, 1996. [Google Scholar]
- Owens, D.K.; Wendt, R.C. Estimation of the surface free energy of polymers. J. Appl. Polym. Sci. 1969, 13, 1741–1747. [Google Scholar] [CrossRef]
- Rudawska, A.; Jacniacka, E. Analysis for determining surface free energy uncertainty by the owen–wendt method. Int. J. Adhes. Adhes. 2009, 29, 451–457. [Google Scholar] [CrossRef]
- Wu, S. Calculation of interfacial tension in polymer systems. J. Polym. Sci. Part C Polym. Symp. 1971, 34, 19–30. [Google Scholar] [CrossRef]
- Rieke, P.C. Application of van oss-chaudhury-good theory of wettability to interpretation of interfacial free energies of heterogeneous nucleation. J. Cryst. Growth 1997, 182, 472–484. [Google Scholar] [CrossRef]
- Wu, S. Polar and nonpolar interactions in adhesion. J. Adhes. 1973, 5, 39–55. [Google Scholar] [CrossRef]
- Greiveldinger, M.; Shanahan, M.E.R. A critique of the mathematical coherence of acid/base interfacial free energy theory. J. Colloid Interf. Sci. 1999, 215, 170–178. [Google Scholar] [CrossRef] [PubMed]
- Mitchell, S.M.; Ullman, J.L.; Teel, A.L.; Watts, R.J. Ph and temperature effects on the hydrolysis of three β-lactam antibiotics: Ampicillin, cefalotin and cefoxitin. Sci. Total Environ. 2014, 466–467, 547–555. [Google Scholar] [CrossRef] [PubMed]
- Costa, P.; Sousa Lobo, J.M. Modeling and comparison of dissolution profiles. Eur. J. Pharm. Sci. 2001, 13, 123–133. [Google Scholar] [CrossRef]
- 5 mathematical models of drug release. In Strategies to Modify the Drug Release from Pharmaceutical Systems; Bruschi, M.L. (Ed.) Woodhead Publishing: Cambridge, England, 2015; pp. 63–86.
- Higuchi, W.I. Diffusional modelsuseful in biopharmaceutics drug releaserate processes. J. Pharm. Sci. 1967, 56, 315–324. [Google Scholar] [CrossRef]
- Korsmeyer, R.W.; Gurny, R.; Doelker, E.; Buri, P.; Peppas, N.A. Mechanisms of solute release from porous hydrophilic polymers. Int. J. Pharm. 1983, 15, 25–35. [Google Scholar] [CrossRef]
- Chang, M.-K. Mechanical properties and thermal stability of low-density polyethylene grafted maleic anhydride/montmorillonite nanocomposites. J. Ind. Eng. Chem. 2015, 27, 96–101. [Google Scholar] [CrossRef]
- Murillo, E.A.; López, B.L. Effect of the maleic anhydride content on the structural, thermal, rheological and film properties of the n-butyl methacrylate–maleic anhydride copolymers. Prog. Org. Coat. 2015, 78, 96–102. [Google Scholar] [CrossRef]
- Zuo, Y.; Gu, J.; Yang, L.; Qiao, Z.; Tan, H.; Zhang, Y. Synthesis and characterization of maleic anhydride esterified corn starch by the dry method. Int. J. Biol. Macromol. 2013, 62, 241–247. [Google Scholar] [CrossRef] [PubMed]
- Hapgood, K.P.; Litster, J.D.; Biggs, S.R.; Howes, T. Drop penetration into porous powder beds. J. Colloid Interf. Sci. 2002, 253, 353–366. [Google Scholar] [CrossRef] [PubMed]
- Birdi, K.S. Handbook of Surface and Colloid Chemistry; CRC Press: Boca Ratón, FL, USA, 1997. [Google Scholar]
- Ohm, A.; Lippold, B.C. Charakterisierung der benetzbarkeit von arzeistoffpulvern mit hilfe der sessipe-drop technik, teil 2: Oberflächenspannung und raindwinkel/oberflächenspannungskurven. Pharm. Ind. 1986, 48, 508–513. [Google Scholar]
- Li, X.; Zhang, C.; Du, Z.; Li, H. Preparation of hydrophilic/hydrophobic porous materials. J. Colloid Interf. Sci. 2008, 323, 120–125. [Google Scholar] [CrossRef] [PubMed]
- Siepmann, J.; Peppas, N.A. Modeling of drug release from delivery systems based on hydroxypropyl methylcellulose (HPMC). Adv. Drug Deliv. Rev. 2001, 48, 139–157. [Google Scholar] [CrossRef]
- Sugano, K.; Okazaki, A.; Sugimoto, S.; Tavornvipas, S.; Omura, A.; Mano, T. Solubility and dissolution profile assessment in drug discovery. Drug Metab. Pharmacokinet. 2007, 22, 225–254. [Google Scholar] [CrossRef] [PubMed]
- Brophy, M.R.; Deasy, P.B. Application of the Higuchi model for drug release from dispersed matrices to particles of general shape. Int. J. Pharm. 1987, 37, 41–47. [Google Scholar] [CrossRef]
- Siepmann, J.; Peppas, N.A. Higuchi equation: Derivation, applications, use and misuse. Int. J. Pharm. 2011, 418, 6–12. [Google Scholar] [CrossRef] [PubMed]








| % PAM-18K in the Tablet | Applied Pressure (psi) | Average Hardness (kp) | Disintegration Time (min:s) |
|---|---|---|---|
| 0 | 200 | 4.48 ± 0.24 | 3:45 |
| 300 | 7.86 ± 0.71 | 4:35 | |
| 400 | 9.51 ± 0.57 | 8:30 | |
| 10 | 200 | 6.15 ± 0.72 | 2:48 |
| 300 | 8.91 ± 0.71 | 3:26 | |
| 400 | 12.46 ± 1.45 | 3:28 | |
| 20 | 200 | 7.83 ± 0.69 | 3:32 |
| 300 | 10.17 ± 1.68 | 3:33 | |
| 400 | 12.99 ± 2.10 | 5:23 | |
| 30 | 200 | 12.54 ± 0.34 | 4:20 |
| 300 | 13.74 ± 0.52 | 7:12 | |
| 400 | 17.34 ± 0.36 | 9:00 | |
| 40 | 200 | 15.67± 1.13 | 9:50 |
| 300 | 18.75± 0.30 | 13:51 | |
| 400 | Undetermined | 17:30 |
| Physical Property | Water | Ethylene Glycol | Isopropanol | |
|---|---|---|---|---|
| * Surface tension (mN/m) | Total | 72.1 | 48.0 | 23.0 |
| Dispersion contribution | 19.9 | 29.0 | 19.5 | |
| Polar contribution | 52.2 | 19.0 | 3.5 | |
| ** Dielectric constant | 80.1 | 68.0 | 17.9 | |
| % PAM-18K within the Tablet | Surface Free Energy (mJ/m2) | ||||||
|---|---|---|---|---|---|---|---|
| Young-Dupre | Neuman (EdE) | Owens, Wendt, Rabel & Käelble (OWRK) | |||||
| (Wadh) | Total SFE | Dispersive | Polar | SFE (R2) | SFE (s) | ||
| 0% | 106.5 ± 2.8 | 46.4 ± 1.5 | 39.1 ± 2.4 | 5.1 | 33.9 | 0.970 | 4.1 |
| 10% | 99.6 ± 0.7 | 42.7 ± 0.4 | 34.3 ± 0.4 | 7.9 | 26.4 | 0.998 | 0.8 |
| 20% | 98.2 ± 1.6 | 41.9 ± 0.8 | 33.0 ± 1.1 | 8.1 | 24.9 | 0.994 | 1.6 |
| 30% | 86.2 ± 3.3 | 35.8 ± 1.7 | 26.5 ± 1.3 | 11.6 | 14.9 | 0.997 | 0.9 |
| 40% | 79.7 ± 3.1 | 32.5 ± 1.5 | 24.5 ± 0.6 | 14.3 | 10.3 | 0.999 | 0.4 |
| % PAM-18K within the Tablet | Initial θc (°) | Final θc (°) | Absorption Time (s) | Absorption Rate (°/s) |
|---|---|---|---|---|
| 0 | 61.9 | 10.0 | 16.9 | 3.2 |
| 10 | 72.7 | 10.3 | 5.9 | 10.6 |
| 20 | 86.2 | 10.7 | 8,8 | 8.5 |
| 30 | 86.4 | 25.5 | 9.9 | 6.1 |
| 40 | 80.5 | 10.3 | 15.8 | 4.4 |
| pH of the Medium | % Polymer in the Tablet | % of Dissolved Drug | Dissolution Time (min) | Dissolution Efficiency |
|---|---|---|---|---|
| 1.2 | 0 | 100 | 25 | 99.64 |
| 10 | 100 | 25 | 87.69 | |
| 20 | 100 | 25 | 86.49 | |
| 30 | 84 | 45 | 63.91 | |
| 40 | 84 | 45 | 57.29 | |
| 7.4 | 0 | 100 | 15 | 96.89 |
| 10 | 100 | 15 | 93.87 | |
| 20 | 100 | 20 | 84.84 | |
| 30 | 96.8 | 45 | 58.42 | |
| 40 * | 78.7 | 45 | 42.49 |
| pH of the Medium | % Polymer in the Tablet | Zero Order | First Order | Higuchi | Korsmeyer-Peppas | |||||
|---|---|---|---|---|---|---|---|---|---|---|
| k0 | R2 | k1 | R2 | kH | R2 | kr | N | R2 | ||
| 1.2 | 0 | 2.978 | 0.558 | 0.050 | 0.867 | 18.655 | 0.924 | - | - | - |
| 10 | 1.148 | 0.271 | 0.022 | 0.273 | 11.754 | 0.932 | - | - | - | |
| 20 | 3.642 | 0.767 | 0.082 | 0.992 | 23.110 | 0.978 | - | - | - | |
| 30 | 1.497 | 0.758 | 0.016 | 0.933 | 12.244 | 0.949 | 2.542 | 1.040 | 0.822 | |
| 40 | 1.868 | 0.933 | 0.020 | 0.991 | 14.006 | 0.982 | 1.399 | 1.340 | 0.932 | |
| 7.4 | 0 | 6.421 | 0.795 | 0.132 | 0.998 | 27.091 | 0.964 | - | - | - |
| 10 | 7.086 | 0.894 | 0.146 | 0.947 | 28.270 | 0.991 | - | - | - | |
| 20 | 5.444 | 0.959 | 0.099 | 0.919 | 24.300 | 0.933 | - | - | - | |
| 30 | 2.325 | 0.953 | 0.033 | 0.963 | 16.843 | 0.936 | 1.063 | 1.360 | 0.996 | |
| 40 | 1.826 | 0.993 | 0.015 | 0.981 | 12.902 | 0.928 | 1.135 | 1.184 | 0.993 | |
© 2016 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC-BY) license ( http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yarce, C.J.; Pineda, D.; Correa, C.E.; Salamanca, C.H. Relationship between Surface Properties and In Vitro Drug Release from a Compressed Matrix Containing an Amphiphilic Polymer Material. Pharmaceuticals 2016, 9, 34. https://doi.org/10.3390/ph9030034
Yarce CJ, Pineda D, Correa CE, Salamanca CH. Relationship between Surface Properties and In Vitro Drug Release from a Compressed Matrix Containing an Amphiphilic Polymer Material. Pharmaceuticals. 2016; 9(3):34. https://doi.org/10.3390/ph9030034
Chicago/Turabian StyleYarce, Cristhian J., Diego Pineda, Clara E. Correa, and Constain H. Salamanca. 2016. "Relationship between Surface Properties and In Vitro Drug Release from a Compressed Matrix Containing an Amphiphilic Polymer Material" Pharmaceuticals 9, no. 3: 34. https://doi.org/10.3390/ph9030034
APA StyleYarce, C. J., Pineda, D., Correa, C. E., & Salamanca, C. H. (2016). Relationship between Surface Properties and In Vitro Drug Release from a Compressed Matrix Containing an Amphiphilic Polymer Material. Pharmaceuticals, 9(3), 34. https://doi.org/10.3390/ph9030034