Differential Flatness Based-Control Strategy of a Two-Port Bidirectional Supercapacitor Converter for Hydrogen Mobility Applications
Abstract
:1. Introduction
2. Supercapacitor-Based Energy Storage for PEMFC Mobility Applications
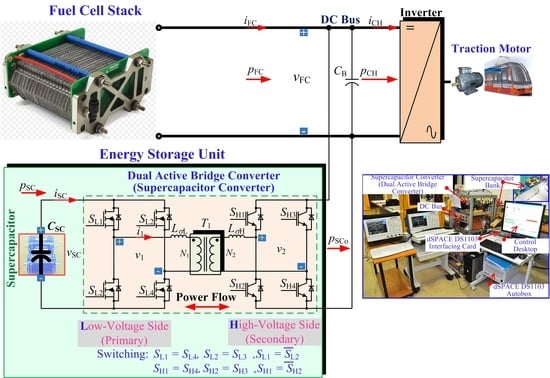
2.1. System Description
2.2. Proposed Two-Port Bidirectional DC-DC Converter
2.3. Reduced-Order Model of the System
3. Control Algorithm and Control Law
- (1)
- The FC voltage vFC is a relevant variable.
- (2)
- The SC voltage vSC is the second most essential.
3.1. Development of Flatness Control Applied to the Supercapacitor Energy Storage
3.2. Production of the FC Voltage Set Point and Charging Supercapacitor Strategy
3.3. FC Voltage Balance and Control Law
4. Performance Validation
- (1)
- The trajectory planning (9) generated a desired DC bus energy trajectory yd from 19.8 J to 15.8 J.
- (2)
- In effect, the FC voltage follows the reference trajectory yd.
- (3)
- The SC provided a major part of the power requested during the load step and went back to charge the SC storage device, given that VSCd (= VSCNom) > vSC.
- (4)
- Accordingly, the FC power increased, with a limited slope, to a final power of 400 W to meet the load requests and charge the SC storage device.
- (1)
- The trajectory planning (9) created a desiderate trajectory yd from 15.8 J to 15 J, which corresponds to the limited minimum FC voltage VFCMin of 80 V.
- (2)
- The SC unit, which provides the most important part of the power requested during each load step, remained in a discharged state as the result of the load step, since the steady-state load power (1 kW) is higher than the limited maximum power of the FC generator.
- (3)
- Concurrently, the FC power rises up by limiting its slope to its set maximum power of 420 W.
- (1)
- The FC source keeps providing a set maximum power of 420 W to respond to the load requests and charge the SC.
- (2)
- At t = 140.4 s, the SC is close to being fully charged—i.e., vSC = 21.5 V. Accordingly, the FC power is decreased with set power dynamics, corresponding to the energy reference trajectory yd.
- (3)
- Finally, at t = 190 s the SOC of SC reached 100%—i.e., vSC = vSCd = 22 V. After slowly decreasing, pSC = 0 W and pFC = pLoad.
- (1)
- Because the desired energy trajectory was too slow to limit the electrical stress of the FC source, the SC unit was charged by the FC and load.
- (2)
- At t = 35 s, the FC power decreased to zero; after that, the SC unit was charged only by regenerative braking. The FC voltage is always limited at 100 V. The SC was overloaded (VSCNom < vSC); nonetheless, the SC voltage was within the acceptable level (vSC < VSCMax). Refer to Figure 5.
- (1)
- The SC unit supplied the over-energy, stored from the last braking, to drive the load.
- (2)
- At t = 110 s, after discharging the SC module was fully charged (VSCd = vSC) and the FC supplied the energy to drive the load; pFC = pCH and pSC = 0.
5. Conclusions
Author Contributions
Funding
Conflicts of Interest
Appendix A. Principle of Nonlinear Control Based on the Differential Flatness

References
- Felseghi, R.-A.; Carcadea, E.; Raboaca, M.S.; TRUFIN, C.N.; Filote, C. Hydrogen Fuel Cell Technology for the Sustainable Future of Stationary Applications. Energies 2019, 12, 4593. [Google Scholar] [CrossRef] [Green Version]
- Guilbert, D.; N’Diaye, A.; Gaillard, A.; Djerdir, A. Reliability improvement of a floating interleaved DC/DC boost converter in a PV/fuel cell stand-alone power supply. EPE J. 2019, 29, 49–63. [Google Scholar] [CrossRef]
- Bizon, N.; Mazare, A.G.; Ionescu, L.M.; Thounthong, P.; Kurt, E.; Oproescu, M.; Serban, G.; Lita, I. Better Fuel Economy by Optimizing Airflow of the Fuel Cell Hybrid Power Systems Using Fuel Flow-Based Load-Following Control. Energies 2019, 12, 2792. [Google Scholar] [CrossRef] [Green Version]
- Hegazy, O.; Van Mierlo, J.; Lataire, P.; Coosemans, T.; Smenkens, J.; Monem, M.A.; Omar, N.; Van den Bossche, P. An Evaluation Study of Current and Future Fuel Cell Hybrid Electric Vehicles Powertrains. World Electr. Veh. J. 2013, 6, 476–483. [Google Scholar] [CrossRef] [Green Version]
- Bizon, N.; Lopez-Guede, J.M.; Kurt, E.; Thounthong, P.; Mazare, A.G.; Ionescu, L.M.; Iana, G. Hydrogen economy of the fuel cell hybrid power system optimized by air flow control to mitigate the effect of the uncertainty about available renewable power and load dynamics. Energy Convers. Manag. 2019, 179, 152–165. [Google Scholar] [CrossRef]
- Serpi, A.; Porru, M. Modelling and Design of Real-Time Energy Management Systems for Fuel Cell/Battery Electric Vehicles. Energies 2019, 12, 4260. [Google Scholar] [CrossRef] [Green Version]
- Sharer, P.; Rousseau, A. Benefits of Fuel Cell Range Extender for Medium-Duty Vehicle Applications. World Electr. Veh. J. 2013, 6, 452–463. [Google Scholar] [CrossRef] [Green Version]
- Lü, X.; Qu, Y.; Wang, Y.; Qin, C.; Liu, G. A comprehensive review on hybrid power system for PEMFC-HEV: Issues and strategies. Energy Convers. Manag. 2018, 171, 1273–1291. [Google Scholar] [CrossRef]
- Yao, G.; Du, C.; Ge, Q.; Jiang, H.; Wang, Y.; Ait-Ahmed, M.; Moreau, L. Traffic-Condition-Prediction-Based HMA-FIS Energy-Management Strategy for Fuel-Cell Electric Vehicles. Energies 2019, 12, 4426. [Google Scholar] [CrossRef] [Green Version]
- Sikkabut, S.; Mungporn, P.; Ekkaravarodome, C.; Bizon, N.; Tricoli, P.; Nahid-Mobarakeh, B.; Pierfederici, S.; Davat, B.; Thounthong, P. Control of high-energy high-power densities storage devices by li-ion battery and supercapacitor for fuel cell/photovoltaic hybrid power plant for autonomous system applications. IEEE Trans. Ind. Appl. 2016, 52, 4395–4407. [Google Scholar] [CrossRef] [Green Version]
- Do, T.C.; Truong, H.V.A.; Dao, H.V.; Ho, C.M.; To, X.D.; Dang, T.D.; Ahn, K.K. Energy Management Strategy of a PEM Fuel Cell Excavator with a Supercapacitor/Battery Hybrid Power Source. Energies 2019, 12, 4362. [Google Scholar] [CrossRef] [Green Version]
- Kasicheyanula, S.; John, V. Adaptive Control Strategy for Ultracapacitor Based Bidirectional DC–DC Converters. IEEE Trans. Ind. Appl. 2019, 55, 1717–1728. [Google Scholar] [CrossRef]
- Dey, S.; Mohon, S.; Ayalew, B.; Arunachalam, H.; Onori, S. A Novel Model-Based Estimation Scheme for Battery-Double-Layer Capacitor Hybrid Energy Storage Systems. IEEE Trans. Control Syst. Technol. 2019, 27, 689–702. [Google Scholar] [CrossRef]
- Mamun, A.; Liu, Z.; Rizzo, D.M.; Onori, S. An Integrated Design and Control Optimization Framework for Hybrid Military Vehicle Using Lithium-Ion Battery and Supercapacitor as Energy Storage Devices. IEEE Trans. Transp. Electrif. 2019, 5, 239–251. [Google Scholar] [CrossRef]
- Maher, B. Ultracapacitors Provide Cost and Energy Savings for Public Transportation Applications. Battery Power Mag. 2006, 10, 6. [Google Scholar]
- Godbersen, C. Energy storage system based on double layer capacitor technology—The gateway to high efficient improvement of mass transit power supply. In Proceedings of the 2nd UIC Railway Energy Efficiency Conference, Paris, France, 4–5 February 2004. [Google Scholar]
- Steiner, M.; Scholten, J. Energy Storage on board of railway vehicles. In Proceedings of the 11th European Conference on Power Electronics and Applications (EPE), Dresden, Germany, 11–14 September 2005. [Google Scholar]
- Kawashima, K.; Uchida, T.; Hori, Y. Development of a Novel Ultracapacitor Electric Vehicle and Methods to Cope with Voltage Variation. In Proceedings of the 5th IEEE Vehicle Power and Propulsion Conference (VPPC’09), Dearborn, MI, USA, 7–11 September 2009. [Google Scholar]
- Chakraborty, S.; Vu, H.-N.; Hasan, M.M.; Tran, D.-D.; Baghdadi, M.E.; Hegazy, O. DC-DC Converter Topologies for Electric Vehicles, Plug-in Hybrid Electric Vehicles and Fast Charging Stations: State of the Art and Future Trends. Energies 2019, 12, 1569. [Google Scholar] [CrossRef] [Green Version]
- Ibanez, F.M.; Beizama Florez, A.M.; Gutiérrez, S.; Echeverrría, J.M. Extending the Autonomy of a Battery for Electric Motorcycles. IEEE Trans. Veh. Technol. 2019, 68, 3294–3305. [Google Scholar] [CrossRef]
- Thammasiriroj, W.; Chunkag, V.; Phattanasak, M.; Pierfederici, S.; Davat, B.; Thounthong, P. Nonlinear model based single-loop control of interleaved converters for a hybrid source system. ECTI Trans. Electr. Eng. Electron. Commun. 2017, 15, 19–31. [Google Scholar]
- Shah, S.S.; Bhattacharya, S. A Simple Unified Model for Generic Operation of Dual Active Bridge Converter. IEEE Trans. Ind. Electron. 2019, 66, 3486–3495. [Google Scholar] [CrossRef]
- Guo, Z.; Sha, D. Dual-Active-Bridge Converter with Parallel-Connected Full Bridges in Low-Voltage Side for ZVS by Using Auxiliary Coupling Inductor. IEEE Trans. Ind. Electron. 2019, 66, 6856–6866. [Google Scholar] [CrossRef]
- Fliess, M.; Lévine, J.; Martin, P.; Rouchon, P. Flatness and defect of nonlinear systems: Introductory theory and examples. Int. J. Control 1995, 61, 1327–1361. [Google Scholar] [CrossRef] [Green Version]
- Ogunbodede, O.; Nandi, S.; Singh, T. Periodic Control of Unmanned Aerial Vehicles Based on Differential Flatness. ASME J. Dyn. Sys. Meas. Control 2019, 141, 071003. [Google Scholar] [CrossRef] [Green Version]
- Benaouadj, M.; Aboubou, A.; Ayad, M.; Becherif, M.; Bahri, M.; Tegani, I. Optimal/flatness based-control of stand-alone power systems using fuel cells, batteries and supercapacitors. J. Electr. Syst. 2017, 13, 43–54. [Google Scholar]
- Huangfu, Y.; Li, Q.; Xu, L.; Ma, R.; Gao, F. Extended state observer based flatness control for fuel cell output series interleaved boost converter. IEEE Trans. Ind. Appl. 2019, 55, 6427–6437. [Google Scholar] [CrossRef]
- Poonnoy, N.; Mungporn, P.; Thounthong, P.; Sikkabut, S.; Yodwong, B.; Boonseng, A.; Ekkaravarodome, C.; Kumam, P.; Bizon, N.; Nahid-Mobarakeh, B.; et al. Differential flatness based control of 3-phase AC/DC converter. In Proceedings of the IEEE 2017 European Conference on Electrical Engineering and Computer Science (EECS), Bern, Switzerland, 17–19 November 2017; pp. 136–141. [Google Scholar]
- Thounthong, P.; Sikkabut, S.; Poonnoy, N.; Mungporn, P.; Yodwong, B.; Kumam, P.; Bizon, N.; Nahid-Mobarakeh, B.; Pierfederici, S. Nonlinear differential flatness-based speed/torque control with state-observers of permanent magnet synchronous motor drives. IEEE Trans. Ind. Appl. 2018, 54, 2874–2884. [Google Scholar] [CrossRef]
- Sriprang, S.; Nahid-Mobarakeh, B.; Takorabet, N.; Pierfederici, S.; Bizon, N.; Kuman, P.; Thounthong, P. Permanent magnet synchronous motor dynamic modeling with state observer-based parameter estimation for AC servomotor drive application. Appl. Sci. Eng. Prog. 2019, 12, 286–297. [Google Scholar] [CrossRef]
- Thounthong, P.; Pierfederici, S.; Davat, B. Analysis of differential flatness-based control for a fuel cell hybrid power source. IEEE Trans. Energy Convers. 2010, 25, 909–920. [Google Scholar] [CrossRef] [Green Version]
- Yodwong, B.; Thounthong, P.; Guilbert, D.; Bizon, N. Differential Flatness-Based Cascade Energy/Current Control of Battery/Supercapacitor Hybrid Source for Modern e–Vehicle Applications. Mathematics 2020, 8, 704. [Google Scholar] [CrossRef]
- Thounthong, P.; Raël, S.; Davat, B. Energy management of fuel cell/battery/supercapacitor hybrid power source for vehicle applications. J. Power Sources 2009, 193, 376–385. [Google Scholar] [CrossRef]
- De Donker, R.W.; Divan, D.M.; Kheraluwala, M.H. A three-phase soft- switched high power density dc-dc converter for high power applications. IEEE Trans. Ind. Applicat. 1991, 27, 63–73. [Google Scholar] [CrossRef]
- Kheraluwala, M.H.; Gascoigne, R.W.; Divan, D.M.; Baumann, E.D. Performance characterization of a high-power dual active bridge dc-to-dc converter. IEEE Trans. Ind. Appl. 1992, 28, 1294–1301. [Google Scholar] [CrossRef]
- Wang, L.; Collins, E.G.; Li, H. Optimal Design and Real-Time Control for Energy Management in Electric Vehicles. IEEE Trans. Veh. Technol. 2011, 60, 1419–1429. [Google Scholar] [CrossRef]
- Tao, H.; Kotsopoulos, A.; Duarte, J.L.; Hendrix, M.A.M. Transformer-Coupled Multiport ZVS Bidirectional DC–DC Converter with Wide Input Range. IEEE Trans. Power Electron. 2008, 23, 771–781. [Google Scholar] [CrossRef] [Green Version]
- Jain, A.K.; Ayyanar, R. PWM Control of Dual Active Bridge: Comprehensive Analysis and Experimental Verification. IEEE Trans. Power Electron. 2008, 23, 771–781. [Google Scholar]
- Bai, H.; Mi, C. Eliminate Reactive Power and Increase System Efficiency of Isolated Bidirectional Dual-Active-Bridge DC–DC Converters Using Novel Dual-Phase-Shift Control. IEEE Trans. Power Electron. 2008, 23, 2905–2914. [Google Scholar] [CrossRef]
- Inoue, S.; Akagi, H. A Bidirectional DC–DC Converter for an Energy Storage System With Galvanic Isolation. IEEE Trans. Power Electron. 2007, 22, 2299–2306. [Google Scholar] [CrossRef]
- Wang, J.; Peng, F.Z.; Anderson, J.; Joseph, A.; Buffenbarger, R. Low Cost Fuel Cell Converter System for Residential Power Generation. IEEE Trans. Power Electron. 2004, 19, 1315–1322. [Google Scholar] [CrossRef]
- Huang, Y.; Wang, H.; Khajepour, A.; Li, B.; Ji, J.; Zhao, K.; Hu, C. A review of power management strategies and component sizing methods for hybrid vehicles. Renew. Sustain. Energy Rev. 2018, 96, 132–144. [Google Scholar] [CrossRef]
- Mungporn, P.; Thounthong, P.; Sikkabut, S.; Yodwong, B.; Ekkaravarodome, C.; Kumam, P.; Junkhiaw, S.T.; Bizon, N.; Nahid-Mobarakeh, B.; Pierfederici, S. Differential flatness-based control of current/voltage stabilization for a single-phase PFC with multiphase interleaved boost converters. In Proceedings of the 2017 IEEE European Conference on Electrical Engineering and Computer Science (EECS 2017), Bern, Switzerland, 17–19 November 2017; pp. 124–130. [Google Scholar]
- Sriprang, S.; Nahid-Mobarakeh, B.; Pierfederici, S.; Takorabet, N.; Bizon, N.; Kumam, P.; Mungporn, P.; Thounthong, P. Robust flatness-based control with state observer-based parameter estimation for PMSM drive. In Proceedings of the 2018 IEEE International Conference on Electrical Systems for Aircraft, Railway, Ship Propulsion and Road Vehicles and International Transportation Electrification Conference (ESARS-ITEC 2018), Nottingham, UK, 7–9 November 2018; pp. 1–6. [Google Scholar]
- Thammasiriroj, W.; Chunkag, V.; Phattanasak, M.; Pierfederici, S.; Davat, B.; Thounthong, P. Simplified single-loop full-flatness control of a hybrid power plant. In Proceedings of the 2016 IEEE SICE International Symposium on Control Systems (ISCS 2016), Nagoya, Japan, 7–10 March 2016. [Google Scholar]


















| Parameter | Value | Unit |
|---|---|---|
| VSCd | 22 | V |
| VFCMax | 100 | V |
| VFCmin | 80 | V |
| PFCMax | 420 | W |
| PFCMin | 0 | W |
| CB | 4700 | μF |
| CSC | 250 | F |
| rSC | 0.05 | Ω |
| PSCMax | +1000 | W |
| PSCMin | −1000 | W |
| VSCMax | 32 | V |
| VSCMin | 15 | V |
| ISCRated | 150 | A |
| KSC | 50 | pu. |
| ωS | 125,664 | rad/s |
| ωn1 | 50 | rad/s |
| ωn2 | 0.2 | rad/s |
| ζ1 | 0.707 | pu. |
| ζ2 | 1 | pu. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Thounthong, P.; Phattanasak, M.; Guilbert, D.; Takorabet, N.; Pierfederici, S.; Nahid-Mobarakeh, B.; Bizon, N.; Kumam, P. Differential Flatness Based-Control Strategy of a Two-Port Bidirectional Supercapacitor Converter for Hydrogen Mobility Applications. Energies 2020, 13, 2794. https://doi.org/10.3390/en13112794
Thounthong P, Phattanasak M, Guilbert D, Takorabet N, Pierfederici S, Nahid-Mobarakeh B, Bizon N, Kumam P. Differential Flatness Based-Control Strategy of a Two-Port Bidirectional Supercapacitor Converter for Hydrogen Mobility Applications. Energies. 2020; 13(11):2794. https://doi.org/10.3390/en13112794
Chicago/Turabian StyleThounthong, Phatiphat, Matheepot Phattanasak, Damien Guilbert, Noureddine Takorabet, Serge Pierfederici, Babak Nahid-Mobarakeh, Nicu Bizon, and Poom Kumam. 2020. "Differential Flatness Based-Control Strategy of a Two-Port Bidirectional Supercapacitor Converter for Hydrogen Mobility Applications" Energies 13, no. 11: 2794. https://doi.org/10.3390/en13112794
APA StyleThounthong, P., Phattanasak, M., Guilbert, D., Takorabet, N., Pierfederici, S., Nahid-Mobarakeh, B., Bizon, N., & Kumam, P. (2020). Differential Flatness Based-Control Strategy of a Two-Port Bidirectional Supercapacitor Converter for Hydrogen Mobility Applications. Energies, 13(11), 2794. https://doi.org/10.3390/en13112794