Experimental Evaluation of the Heat Balance of an Interactive Glass Wall in A Heating Season
Abstract
:1. Introduction
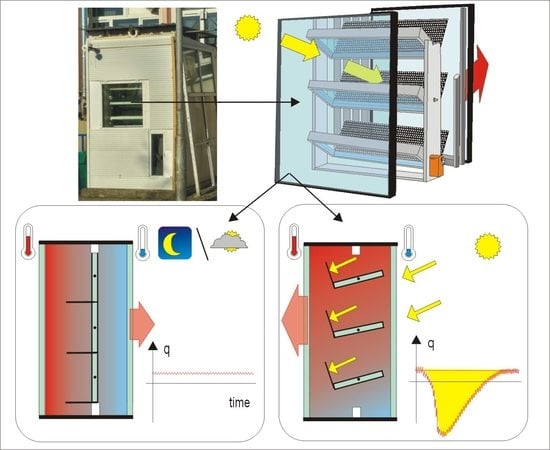
2. IGW Prototype Design and Test Method
2.1. Description of the Tested Prototype
2.2. Description of Test Stand
2.3. Description of the Method for the Evaluation of IGW Thermal Efficiency
- RIGW—total thermal resistance of IGW ((m2·K)/W),
- UIGW—heat transfer coefficient of IGW (W/(m2·K)),
- ΔT—temperature difference on the wall’s sides.
- A—total pane area (m2).
- Cg—ratio of the outer glazing visible area (not covered by the glazing bead) to the total glazing inner area IGW (Cg = 0.89),
- Sg—the sum of solar irradiance transferred through the outer glazing (Wh/m2).
3. Results and Discussion
3.1. Empirical Heat Transfer Coefficient UIGW
3.2. Determination of the Solar Heat Gain Utilisation Efficiency (ηSHGU)
- —solar irradiation transferred through the outer glazing in time interval (t1:t2).
3.3. Validation of IGW Heat Balance Calculation Mode
3.4. Prediction of IGW Heat Balance in a Heating Season Based on Climate Database
- A—surface of IGW (1 m2),
- UIGW—heat transfer coefficient (after Equation (7)),
- Ti—indoor air temperature (Ti = 20 °C)],
- Seh—hourly sum of solar irradiation recorded in front of the glazing,
- g—gain factor (g = 0.55 according to the manufacturer’s specifications of the glazing used).
4. Conclusions
- (1)
- The prediction of heat balance calculated for a heating season for a selected locality indicated the overbalance of heat gains over losses in all the months of the season when the IGW was oriented to the south, south-east and south-west. The western and eastern orientations in November and December resulted in the predominance of heat losses.
- (2)
- The tests indicated that, unlike conventional windows, apart from transparency, owing to the use of phase-change materials (PCM) in the IGW structure, it has the capacity of giving up heat gains even eight hours after the sunset.
- (3)
- The test results confirm the potential of the interactive designs, which apart from transparency, have the capacity to reduce the conventional energy demand and exert a favourable impact on the functionality of a building and occupants’ comfort. The use of cutting-edge technologies and their increasing availability for the shaping of the outer envelope of a building opens up new possibilities of construction engineering.
- (4)
- The validity of the method discussed in the paper indicated a difference in the heat balance calculated on the basis of the recorded heat flux density at the level of 15.53% of heat gains underestimation or its losses overestimation, which can be considered a result satisfactory with respect of calculations safety.
5. Patents
Funding
Acknowledgments
Conflicts of Interest
References
- Kim, S.-C.; Yoon, J.-H.; Lee, R.-D. Energy Performance Assessment of a 2nd-Generation Vacuum Double Glazing Depending on Vacuum Layer Position and Building Type in South Korea. Energies 2017, 10, 1240. [Google Scholar]
- Al Dakheel, J.; Tabet Aoul, K. Building Applications, Opportunities and Challenges of Active Shading Systems: A State-of-the-Art Review. Energies 2017, 10, 1672. [Google Scholar] [CrossRef] [Green Version]
- Biswas, K.; Jogineedi, R.; Desjarlais, A. Experimental and Numerical Examination of Naturally-Aged Foam-VIP Composites. Energies 2019, 12, 2539. [Google Scholar] [CrossRef] [Green Version]
- Buratti, C.; Moretti, E.; Zinzi, M. High Energy-Efficient Windows with Silica Aerogel for Building Refurbishment: Experimental Characterization and Preliminary Simulations in Different Climate Conditions. Buildings 2017, 7, 8. [Google Scholar] [CrossRef] [Green Version]
- Sohail, U.; Kwiatek, C.; Fung, A.S.; Joksimovic, D. Techno-Economic Feasibility of Wastewater Heat Recovery for A Large Hospital in Toronto, Canada. Multidiscip. Digit. Publ. Inst. Proc. 2019, 23, 1. [Google Scholar] [CrossRef] [Green Version]
- Saadatian, O.; Sopian, K.; Lim, C.H.; Asim, N.; Sulaiman, M.Y. Trombe walls: A review of opportunities and challenges in research and development. Renew. Sustain. Energy Rev. 2012, 16, 6340. [Google Scholar] [CrossRef]
- Zhongting, H.; Wei, H.; Jie, J.; Shengyao, Z. A review on the application of Trombe wall system in buildings. Renew. Sustain. Energy Rev. 2017, 70, 976–987. [Google Scholar]
- Zhang, H.; Shu, H.J. A Comprehensive Evaluation on Energy, Economic and Environmental Performance of the Trombe Wall during the Heating Season. J. Therm. Sci. 2019, 28, 1141–1149. [Google Scholar] [CrossRef]
- Bevilacqua, P.; Benevento, F.; Bruno, R.; Arcuri, N. Are Trombe walls suitable passive systems for the reduction of the yearly building energy requirements? Energy 2019, 185, 554–566. [Google Scholar] [CrossRef]
- Zalewski, L.; Chantant, M.; Lassue, S.; Duthoit, B. Experimental thermal study of a solar wall of composite type. Energy Build. 1997, 25, 7–18. [Google Scholar] [CrossRef]
- Shen, J.; Lassue, S.; Zalewski, L.; Huang, D. Numerical study on thermal behavior of classical or composite Trombe solar walls. Energy Build. 2007, 39, 962–974. [Google Scholar] [CrossRef]
- Ji, J.; Luo, C.; Sun, W.; Yu, H.; He, W.; Pei, G. An improved approach for the application of Trombe wall system to building construction with selective thermo-insulation façades. Chin. Sci. Bull. 2009, 54, 1949. [Google Scholar] [CrossRef]
- Wang, X.; Lei, B.; Bi, H.; Yu, T. Study on the Thermal Performance of a Hybrid Heat Collecting Facade Used for Passive Solar Buildings in Cold Region. Energies 2019, 12, 1038. [Google Scholar] [CrossRef] [Green Version]
- Hu, Z.; He, W.; Ji, J.; Hu, D.; Lv, S.; Chen, H.; Shen, Z. Comparative study on the annual performance of three types of building integrated photovoltaic (BIPV) Trombe wall system. Appl. Energy 2017, 194, 81–93. [Google Scholar] [CrossRef]
- Lin, Y.; Ji, J.; Zhou, F.; Ma, Y.; Luo, K.; Lu, X. Experimental and numerical study on the performance of a built-middle PV Trombe wall system. Energy Build. 2019, 200, 47–57. [Google Scholar] [CrossRef]
- Irshad, K.; Habib, K.; Thirumalaiswamy, N. Performance Evaluation of PV-trombe Wall for Sustainable Building Development. Procedia CIRP 2015, 26, 624–629. [Google Scholar] [CrossRef] [Green Version]
- Liu, X.; Zhou, Y.; Li, C.-Q.; Lin, Y.; Yang, W.; Zhang, G. Optimization of a New Phase Change Material Integrated Photovoltaic/Thermal Panel with The Active Cooling Technique Using Taguchi Method. Energies 2019, 12, 1022. [Google Scholar] [CrossRef] [Green Version]
- Yu, B.; Jiang, Q.; He, W.; Hu, Z.; Chen, H.; Ji, J.; Xu, G. The performance analysis of a novel TC-Trombe wall system in heating seasons. Energy Convers. Manag. 2018, 164, 242–261. [Google Scholar] [CrossRef]
- Nayak, J.K. Transwall versus trombe wall: Relative performance studies. Energy Convers. Manag. 1987, 27, 389–393. [Google Scholar] [CrossRef]
- Li, S.; Zhu, N.; Hu, P.; Lei, F.; Deng, R. Numerical study on thermal performance of PCM Trombe Wall. Energy Procedia 2019, 158, 2441–2447. [Google Scholar] [CrossRef]
- Corasaniti, S.; Manni, L.; Russo, F.; Gori, F. Numerical simulation of modified Trombe-Michel Walls with exergy and energy analysis. Int. Commun. Heat Mass Transf. 2017, 88, 269–276. [Google Scholar] [CrossRef]
- Leang, E.; Tittelein, P.; Zalewski, L.; Lassue, S. Numerical study of a composite Trombe solar wall integrating microencapsulated PCM. Energy Procedia 2017, 122, 1009–1014. [Google Scholar] [CrossRef]
- Simo-Tagne, M.; Ndukwu, M.C.; Zoulalian, A.; Bennamoun, L.; Kifani-Sahban, F.; Rogaume, Y. Numerical analysis and validation of a natural convection mix-mode solar dryer for drying redchilli under variable conditions. Renew. Energy 2019. [Google Scholar] [CrossRef]
- Simo-Tagne, M.; Ndukwu, M.C.; Rogaume, Y. Modelling and numerical simulation of hygrothermal transfer through a building wall for locations subjected to outdoor conditions in Sub-Saharan Africa. J. Build. Eng. 2019, 26, 100901. [Google Scholar] [CrossRef]
- Barone, G.; Buonomano, A.; Forzano, C.; Palombo, A. Building Energy Performance Analysis: An Experimental Validation of an In-House Dynamic Simulation Tool through a Real Test Room. Energies 2019, 12, 4107. [Google Scholar] [CrossRef] [Green Version]
- Briga-Sá, A.; Boaventura-Cunha, J.; Lanzinha, J.C.; Paiva, A. An experimental analysis of the Trombe wall temperature fluctuations for high range climate conditions: Influence of ventilation openings and shading devices. Energy Build. 2017. [Google Scholar] [CrossRef] [Green Version]
- Szyszka, J. Simulation of modified Trombe wall. E3S Web Conf. 2018, 49, 00114. [Google Scholar] [CrossRef] [Green Version]
- Website of the Ministry of Fundsand Regional Policy Republic of Poland, Data for A the Typical Meteorogical Year for Rzeszow. Available online: https://www.gov.pl/web/fundusze-regiony/dane-do-obliczen-energetycznych-budynkow (accessed on 22 June 2019).












| Kind of Sensor | Type of Sensor | Accuracy |
|---|---|---|
| Temperature sensor | PT1000 | A class (<0.2 °C) |
| Irradiaton sensor | DeltaOhm LP Pyra12 DeltaOhm LPPyra03 | <1 % (first class) <±0.2% (second class) |
| Heat flow density | ALMEMO FQ A020 C | <6% of measured value |
| Comet MS6D 16 Channel Data Acquisition Monitoring System | ||
| DC | 4 to 20 mA | ±0.1% (±0.02 mA) |
| DC | −10 V to +10 V | ±0.1% (±10 mV) |
| Temperature | PT1000 | ±0.2 °C (−200 °C to +100 °C) |
| Number of Day | |||
|---|---|---|---|
| 1 | 3.409 | 20.70 | 0.165 |
| 2 | 3.685 | 20.67 | 0.178 |
| 3 | 3.425 | 20.12 | 0.170 |
| 4 | 3.375 | 20.16 | 0.167 |
| 5 | 3.242 | 20.02 | 0.162 |
| Mean value | 0.168 | ||
| Standard deviation | 0.006 | ||
| Number of Day | |||
|---|---|---|---|
| 1 | 5.08 | 20.14 | 0.252 |
| 2 | 5.05 | 20.45 | 0.247 |
| 3 | 5.38 | 20.23 | 0.266 |
| Mean value | 0.255 | ||
| Standard deviation | 0.01 | ||
| Number of Day [n] | ηSHGU | ||
|---|---|---|---|
| 10 | 131.61 | 608.1 | 21.64 |
| 11 | 276.60 | 1440.8 | 19.20 |
| 12 | 289.75 | 1398.1 | 20.73 |
| 13 | 249.51 | 1190.6 | 20.96 |
| 14 | 236.32 | 1143.3 | 20.67 |
| 15 | 104.56 | 438.6 | 23.84 |
| Mean value | 21.17 | ||
| Standard deviation | 1.53 | ||
| QH−QS | ||||
|---|---|---|---|---|
| 575.89 [Wh] | 3084.79 [Wh] | 2419.46 [Wh] | 665.33 [Wh] | 15.53% |
| Month | Heat Balance [Wh/m2] | ||||
|---|---|---|---|---|---|
| E | SE | S | SW | W | |
| October | −2442.03 | −3526.65 | −4226.97 | −3592.03 | −2492.84 |
| November | 42.10 | −993.54 | −1691.16 | −1240.25 | −132.42 |
| December | 835.30 | −237.27 | −841.21 | −390.31 | 727.05 |
| January | 764.06 | −571.02 | −1160.47 | −421.21 | 870.04 |
| February | −884.79 | −2124.54 | −2690.94 | −1839.41 | −692.03 |
| March | −3260.19 | −4298.28 | −4679.78 | −3861.34 | −2921.9 |
| April | −6536.78 | −7243.21 | −7272.98 | −6953.11 | −6259.6 |
© 2020 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Szyszka, J. Experimental Evaluation of the Heat Balance of an Interactive Glass Wall in A Heating Season. Energies 2020, 13, 632. https://doi.org/10.3390/en13030632
Szyszka J. Experimental Evaluation of the Heat Balance of an Interactive Glass Wall in A Heating Season. Energies. 2020; 13(3):632. https://doi.org/10.3390/en13030632
Chicago/Turabian StyleSzyszka, Jerzy. 2020. "Experimental Evaluation of the Heat Balance of an Interactive Glass Wall in A Heating Season" Energies 13, no. 3: 632. https://doi.org/10.3390/en13030632
APA StyleSzyszka, J. (2020). Experimental Evaluation of the Heat Balance of an Interactive Glass Wall in A Heating Season. Energies, 13(3), 632. https://doi.org/10.3390/en13030632