Model Simulation and Prediction of Decadal Mountain Permafrost Distribution Based on Remote Sensing Data in the Qilian Mountains from the 1990s to the 2040s
Abstract
:1. Introduction
2. Study Area
3. Methodology
3.1. Data Acquisition
3.1.1. Bench-Mark Map
3.1.2. Topographic Parameters
3.1.3. Land Coverage Factor
3.1.4. MDAT Data
3.2. Model Construction
3.3. Model Validation Introduction
3.3.1. Referencing Results
3.3.2. Random Areas Selection
4. Results
4.1. Validation of the Model Simulation Results in Different Periods
4.1.1. Validation of the Model Simulation Results from the 1990s
4.1.2. Validation of the Model Simulation Result from the 2000s
4.1.3. Validation of the Model Simulation Result from the 2010s
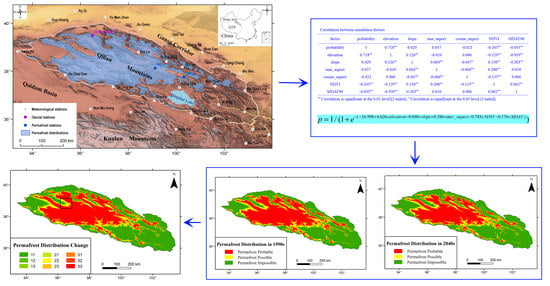
4.2. Model Simulation and Prediction Results of Mountain Permafrost Distribution from the 1990s to the 2040s
4.3. Distribution Change of Mountain Permafrost in the Qilian Mountains from the 1990s to the 2040s
5. Discussion
5.1. Model Effectiveness
5.2. Model Validation
5.3. Model Limitations
6. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Muller, S.W. Permafrost or Permanently Frozen Ground and Related Engineering Problems; Military Intelligence Division Office: Washington, DC, USA, 1943. [Google Scholar]
- Everdingen, V. Multi-Language Glossary of Permafrost and Related Ground-Ice Terms; National Snow and Ice Data Center/World Data Center for Glaciology: Boulder, CO, USA, 2005; Available online: http://nsidc.org/fgdc/glossary/ (accessed on 8 December 2016).
- Lewkowicz, A.G.; Bonnaventure, P.P. Equivalent Elevation: A New Method to Incorporate Variable Surface Lapse Rates into Mountain Permafrost Modelling. Permafr. Periglac. 2011, 22, 153–160. [Google Scholar] [CrossRef]
- Bergstedt, H.; Zwieback, S.; Bartsch, A.; Leibman, M. Dependence of C-Band Backscatter on Ground Temperature, Air Temperature and Snow Depth in Arctic Permafrost Regions. Remote Sens. 2018, 10, 142. [Google Scholar] [CrossRef]
- Wu, J.C.; Sheng, Y.; Wu, Q.B.; Wen, Z. Process and modes of permafrost degradation on the Qinghai-Tibet Plateau. Sci. China Ser. D Earth Sci. 2009, 39, 1570–1578. [Google Scholar] [CrossRef]
- Zhu, Y.; Zhang, Y.; Wen, H.; Lu, Z.; Jia, Z.; Li, Y.; Li, Q.; Liu, C.; Wang, P.; Guo, X. Gas hydrate in the Qilian Mountain permafrost, Qinghai, Northwest China. Acta Geol. Sin. 2009, 83, 1762–1771. [Google Scholar] [CrossRef]
- Nitze, I.; Grosse, G.; Jones, B.M.; Arp, C.D.; Ulrich, M.; Fedorov, A.; Veremeeva, A. Landsat-Based Trend Analysis of Lake Dynamics across Northern Permafrost Regions. Remote Sens. 2017, 9, 640. [Google Scholar] [CrossRef]
- Lara, M.J.; Nitze, I.; Grosse, G.; Martin, P.; McGuire, A.D. Reduced arctic tundra productivity linked with landform and climate change interactions. Sci. Rep. 2018, 8, 2345. [Google Scholar] [CrossRef] [PubMed]
- Yang, M.; Nelson, F.E.; Shiklomanov, N.I.; Guo, D.; Wan, G. Permafrost degradation and its environmental effects on the Tibetan Plateau: A review of past research. Earth-Sci. Rev. 2010, 103, 31–44. [Google Scholar] [CrossRef]
- Wang, G.; Li, Y.; Wang, Y.; Wu, Q. Effects of permafrost thawing on vegetation and soil carbon pool losses on the Qinghai–Tibet Plateau, China. Geoderma 2008, 143, 143–152. [Google Scholar] [CrossRef]
- Malevsky-Malevich, S.P.; Molkentin, E.K.; Nadyozhina, E.D.; Shklyarevich, O.B. Numerical simulation of permafrost parameters distribution in Russia. Cold Reg. Sci. Technol. 2001, 32, 1–11. [Google Scholar] [CrossRef]
- Imhof, M. Modelling and Verification of the Permafrost Distribution in the Bernese Alps (Western Switzerland). Permafr. Periglac. 1996, 7, 267–280. [Google Scholar] [CrossRef]
- Salzmann, N.; Frei, C.; Vidale, P.L.; Hoelzle, M. The application of Regional Climate Model output for the simulation of high-mountain permafrost scenarios. Glob. Planet. Chang. 2007, 56, 188–202. [Google Scholar] [CrossRef]
- Hoelzle, M. Permafrost occurrence from BTS measurements and climatic parameters in the eastern Swiss Alps. Permafr. Periglac. 1992, 3, 143–147. [Google Scholar] [CrossRef]
- Ishikawa, M.; Hirakawa, K. Mountain Permafrost Distribution Based on BTS Measurements and DC Resistivity Soundings in the Daisetsu Mountains, Hokkaido, Japan. Permafr. Periglac. 2000, 11, 109–123. [Google Scholar] [CrossRef]
- Mühll, D.V.; Hauck, C.; Gubler, R.; McDonald, R.; Russill, N. New geophysical methods of investigating the nature and distribution of mountain permafrost with special reference to radiometry techniques. Permafr. Periglac. 2001, 12, 27–38. [Google Scholar] [CrossRef]
- Lewkowicz, A.G.; Ednie, M. Probability mapping of mountain permafrost using the BTS method, Wolf Creek, Yukon Territory, Canada. Permafr. Periglac. 2004, 15, 67–80. [Google Scholar] [CrossRef]
- Janke, R.J. The occurrence of alpine permafrost in the Front Range of Colorado. Geomorphology 2005, 67, 375–389. [Google Scholar] [CrossRef]
- Etzelmüller, B.; Heggem, E.S.F.; Sharkhuu, N.; Frauenfelder, R.; Kääb1, A.; Goulden, C. Mountain permafrost distribution modelling using a multi-criteria approach in the Hövsgöl Area, Northern Mongoliay. Permafr. Periglac. 2006, 17, 91–104. [Google Scholar] [CrossRef]
- Li, J.; Sheng, Y.; Wu, J.; Chen, J.; Zhang, X. Probability distribution of permafrost along a transportation corridor in the northeastern Qinghai province of China. Cold Reg. Sci. Technol. 2009, 59, 12–18. [Google Scholar] [CrossRef]
- Li, S.D.; Cheng, G.D. Map of Frozen Ground on Qinghai—Xizang Plateau; Gansu Culture Press: Lanzhou, China, 1996. [Google Scholar]
- Etzelmüller, B.; Ødegård, R.S.; Berthling, I.; Solid, J.L. Terrain Parameters and Remote Sensing Data in the Analysis of Permafrost Distribution and Periglacial Processes: Principles and Examples from Southern Norway. Permafr. Periglac. 2001, 12, 79–92. [Google Scholar] [CrossRef]
- Wu, J.; Sheng, Y.; Li, J.; Wang, J. Permafrost in source areas of Shule River in Qilian Mountains. Acta Geogr. Sin. 2009, 64, 571–580. [Google Scholar]
- Zhou, C.; Cheng, W.; Qian, J. Remote Sensing Interpretation and Cartography to Digital Geomorphology; Science Press: Beijing, China, 2009; ISBN 9787030240668. [Google Scholar]
- Tang, G.; Liu, X.; Lu, G. Principles and Methods of Digital Elevation Model and Topographic Analysis; Science Press: Beijing, China, 2005; ISBN 9787030153210. [Google Scholar]
- Janke, R.J. Modelling past and future alpine permafrost distribution in the Colorado Front Range. Earth Surf. Proc. Land. 2005, 30, 1495–1508. [Google Scholar] [CrossRef]
- Song, Y.; Jin, L.; Wang, H. Vegetation Changes along the Qinghai-Tibet Plateau Engineering Corridor since 2000 Induced by Climate Change and Human Activities. Remote Sens. 2018, 10, 95. [Google Scholar] [CrossRef]
- Cheng, W.; Zhou, C.; Chai, H.; Zhao, S.; Liu, H.; Zhou, Z. Research and compilation of the Geomorphologic Atlas of the People’s Republic of China (1:1,000,000). J. Geogr. Sci. 2011, 21, 89–100. [Google Scholar] [CrossRef]
- Jin, R.; Li, X.; Che, T. A decision tree algorithm for surface soil freeze/thaw classification over China using SSM/I brightness temperature. Remote Sens. Environ. 2009, 113, 2651–2660. [Google Scholar] [CrossRef]
- Zhou, Y.; Guo, D.; Qiu, G.; Cheng, G.; Li, S. Geocryology in China; Science Press: Beijing, China, 2000; ISBN 7030082850. (In Chinese) [Google Scholar]
- Zhao, L.; Sheng, Y. Permafrost Map in Qinghai-Tibet Plateau from 2009 to 2013; Cold and Arid Regions Environmental and Engineering Research Institute Chinese Academy of Sciences: Lanzhou, China, 2015. [Google Scholar]
- Brenning, A.; Gruber, S.; Hoelzle, M. Sampling and Statistical Analyses of BTS Measurements. Permafr. Periglac. 2005, 16, 383–393. [Google Scholar] [CrossRef]
- Ekici, A.; Beer, C.; Hagemann, S.; Boike, J.; Langer, M.; Hauck, C. Simulating high-latitude permafrost regions by the JSBACH terrestrial ecosystem model. Geosci. Model. Dev. 2014, 7, 631–647. [Google Scholar] [CrossRef] [Green Version]
- Anisimov, O.A.; Shiklomanov, N.I.; Nelson, F.E. Variability of seasonal thaw depth in permafrost regions: A stochastic modeling approach. Ecol. Model. 2002, 153, 217–227. [Google Scholar] [CrossRef]
- Li, X.; Cheng, G.D. A GIS-aided response model of high-altitude permafrost to global change. Sci. China Ser. D Earth Sci. 1999, 42, 72–79. [Google Scholar] [CrossRef]
- Wu, Q.B.; Li, X.; Li, W.J. The Response Model of Permafrost along the Qinghai–Tibetan Highway under Climate Change. J. Glaciol. Geocryol. 2001, 23, 1–6. [Google Scholar]
- Nan, Z.T.; Li, S.X.; Cheng, G.D. Prediction of permafrost distribution on the Qinghai-Tibet Plateau in the next 50 and 100 years. Sci. China Ser. D Earth Sci. 2005, 48, 797–804. [Google Scholar] [CrossRef]
- Niu, F.; Yin, G.; Luo, J.; Lin, Z.; Liu, M. Permafrost Distribution along the Qinghai-Tibet Engineering Corridor, China Using High-Resolution Statistical Mapping and Modeling Integrated with Remote Sensing and GIS. Remote Sens. 2018, 10, 215. [Google Scholar] [CrossRef]
- Cheng, W.M.; Zhao, S.M.; Zhou, C.H.; Chen, X. Simulation of the Decadal Permafrost Distribution on the Qinghai-Tibet Plateau (China) over the Past 50 Years. Permafr. Periglac. Process. 2012, 23, 292–300. [Google Scholar] [CrossRef]
- Zhang, Z.; Wang, C.; Zhang, H.; Tang, Y.; Liu, X. Analysis of Permafrost Region Coherence Variation in the Qinghai–Tibet Plateau with a High-Resolution TerraSAR-X Image. Remote Sens. 2018, 10, 298. [Google Scholar] [CrossRef]
- Bonnaventure, P.P.; Lewkowicz, A.G. Modelling climate change effects on the spatial distribution of mountain permafrost at three sites in northwest Canada. Clim. Chang. 2010, 105, 293–312. [Google Scholar] [CrossRef]
- Zhao, S.M.; Cheng, W.M.; Zhou, C.H.; Chen, X.; Chen, J. Simulation of decadal alpine permafrost distributions in the Qilian Mountains over past 50 years by using Logistic Regression Model. Cold Reg. Sci. Technol. 2012, 73, 32–40. [Google Scholar] [CrossRef]
- Guan, Q.; Yang, L.; Pan, N.; Lin, J.; Xu, C.; Wang, F.; Liu, Z. Greening and Browning of the Hexi Corridor in Northwest China: Spatial Patterns and Responses to Climatic Variability and Anthropogenic Drivers. Remote Sens. 2018, 10, 1270. [Google Scholar] [CrossRef]
- Zhang, X.; Qin, X.; Xu, C.; Liu, Y. Simulation of Runoff and Glacier Mass Balance and Sensitivity Analysis in a Glacierized Basin, North-Eastern Qinhai-Tibetan Plateau, China. Water 2018, 10, 1259. [Google Scholar] [CrossRef]







| Decade | Linear Regression Model | R2 |
|---|---|---|
| 1990s | MDAT90=111.624 − 5.647 × [elevation]/1000 − 1.3016 × [latitude] − 0.4460 × [longitude] | 0.948 |
| 2000s | MDAT00=114.902 − 5.716 × [elevation]/1000 − 1.3180 × [latitude] − 0.4669 × [longitude] | 0.949 |
| 2010s | MDAT10=114.930 − 5.612 × [elevation]/1000 − 1.3109 × [latitude] − 0.4702 × [longitude] | 0.937 |
| 2020s | MDAT20=115.390 − 5.572 × [elevation]/1000 − 1.3053 × [latitude] − 0.4744 × [longitude] | 0.928 |
| 2030s | MDAT30=115.867 − 5.529 × [elevation]/1000 − 1.3003 × [latitude] − 0.4785 × [longitude] | 0.917 |
| 2040s | MDAT40= 116.305 − 5.481 × [elevation]/1000 − 1.2952 × [latitude] − 0.4822 × [longitude] | 0.903 |
| Decades | 1990s | 2000s | 2010s | 2020s | 2030s | 2040s | |
|---|---|---|---|---|---|---|---|
| Statistics | |||||||
| Maximum | 9.15 | 9.66 | 9.64 | 9.85 | 10.07 | 10.40 | |
| Minimum | −14.80 | −14.62 | −14.07 | −13.61 | −13.13 | −12.63 | |
| Mean | −2.04 | −1.69 | −1.34 | −0.95 | −0.54 | −0.12 | |
| Standard Deviation | 3.82 | 3.83 | 3.76 | 3.71 | 3.66 | 3.61 | |
| Factor | Probability | Elevation | Slope | Sine_Aspect | Cosine_Aspect | NDVI | MDAT90 |
|---|---|---|---|---|---|---|---|
| Probability | 1 | 0.718 ** | 0.029 | 0.037 | −0.021 | −0.103 ** | −0.693 ** |
| Elevation | 0.718 ** | 1 | 0.126 ** | −0.019 | 0.006 | −0.129 ** | −0.939 ** |
| Slope | 0.029 | 0.126 ** | 1 | 0.064 * | −0.047 | 0.150 ** | −0.203 ** |
| Sine_Aspect | 0.037 | −0.019 | 0.064 * | 1 | −0.060 * | 0.200 ** | 0.010 |
| Cosine_Aspect | −0.021 | 0.006 | −0.047 | −0.060 * | 1 | −0.135 ** | 0.006 |
| NDVI | −0.103 ** | −0.129 ** | 0.150 ** | 0.200 ** | −0.135 ** | 1 | 0.062 * |
| MDAT90 | −0.693 ** | −0.939 ** | −0.203 ** | 0.010 | 0.006 | 0.062 * | 1 |
| Type | 01 | 02 | 03 | 11 | 12 | 13 | |
|---|---|---|---|---|---|---|---|
| Period | |||||||
| 1990s | 81.3 | 17.4 | 11.7 | 8.8 | 13.3 | 61.8 | |
| 2000s | 77.1 | 11.8 | 4.1 | 14.2 | 19.0 | 68.2 | |
| 2010s | 73.2 | 18.6 | 3.1 | 2.5 | 12.1 | 67.9 | |
| Type | 01 | 02 | 03 | 11 | 12 | 13 | |
|---|---|---|---|---|---|---|---|
| Period | |||||||
| 1990s | 3485.5 | 666.9 | 542.5 | 348.9 | 426.7 | 2172.4 | |
| 2000s | 3007.7 | 392.8 | 166.4 | 881.2 | 699.5 | 2495.4 | |
| 2010s | 3137.6 | 684.6 | 147.8 | 110.8 | 406.9 | 2456.4 | |
| Decades | 1990s | 2000s | 2010s | 2020s | 2030s | 2040s | |
|---|---|---|---|---|---|---|---|
| Statistics | |||||||
| Mean | 0.456 | 0.450 | 0.444 | 0.438 | 0.430 | 0.423 | |
| Standard Deviation | 0.393 | 0.393 | 0.391 | 0.390 | 0.389 | 0.387 | |
| Decades | 1990s | 2000s | 2010s | 2020s | 2030s | 2040s | Change | % | |
|---|---|---|---|---|---|---|---|---|---|
| Statistics | |||||||||
| Permafrost impossible | 90.1 | 91.3 | 92.4 | 93.7 | 95.1 | 96.5 | 6.4 | 7.1 | |
| Permafrost possible | 30.7 | 30.8 | 30.9 | 31.0 | 31.2 | 31.3 | 0.6 | 2.0 | |
| Permafrost probable | 73.5 | 72.3 | 71.0 | 69.6 | 68.1 | 66.5 | −7.0 | −9.6 | |
| Type | 11 | 12 | 13 | 21 | 22 | 23 | 31 | 32 | 33 | |
|---|---|---|---|---|---|---|---|---|---|---|
| Period | ||||||||||
| From the 1990s to the 2000s | 89.9 | 0.2 | 0.0 | 1.4 | 29.1 | 0.2 | 0.0 | 1.4 | 72.0 | |
| From the 2000s to the 2010s | 91.1 | 0.2 | 0.0 | 1.3 | 29.2 | 0.3 | 0.0 | 1.5 | 70.8 | |
| From the 2010s to the 2020s | 92.1 | 0.3 | 0.0 | 1.5 | 29.1 | 0.3 | 0.0 | 1.7 | 69.3 | |
| From the 2020s to the 2030s | 93.4 | 0.3 | 0.0 | 1.6 | 29.1 | 0.3 | 0.0 | 1.8 | 67.8 | |
| From the 2030s to the 2040s | 94.8 | 0.3 | 0.0 | 1.7 | 29.2 | 0.3 | 0.0 | 1.9 | 66.2 | |
| From the 1990s to the 2040s | 90.0 | 0.2 | 0.0 | 6.5 | 24.0 | 0.2 | 0.0 | 7.2 | 66.3 | |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhao, S.; Zhang, S.; Cheng, W.; Zhou, C. Model Simulation and Prediction of Decadal Mountain Permafrost Distribution Based on Remote Sensing Data in the Qilian Mountains from the 1990s to the 2040s. Remote Sens. 2019, 11, 183. https://doi.org/10.3390/rs11020183
Zhao S, Zhang S, Cheng W, Zhou C. Model Simulation and Prediction of Decadal Mountain Permafrost Distribution Based on Remote Sensing Data in the Qilian Mountains from the 1990s to the 2040s. Remote Sensing. 2019; 11(2):183. https://doi.org/10.3390/rs11020183
Chicago/Turabian StyleZhao, Shangmin, Shifang Zhang, Weiming Cheng, and Chenghu Zhou. 2019. "Model Simulation and Prediction of Decadal Mountain Permafrost Distribution Based on Remote Sensing Data in the Qilian Mountains from the 1990s to the 2040s" Remote Sensing 11, no. 2: 183. https://doi.org/10.3390/rs11020183
APA StyleZhao, S., Zhang, S., Cheng, W., & Zhou, C. (2019). Model Simulation and Prediction of Decadal Mountain Permafrost Distribution Based on Remote Sensing Data in the Qilian Mountains from the 1990s to the 2040s. Remote Sensing, 11(2), 183. https://doi.org/10.3390/rs11020183