1. Introduction
The Sentinel-2A and Sentinel-2B satellites carry the Multi Spectral Instrument (MSI) that sense medium-resolution multi-spectral data designed for global land surface monitoring [
1]. Combined, the Sentinel-2A and -2B systems have a global median average revisit interval of five days [
2]. The MSI has 13 spectral bands ranging from the visible to the shortwave infrared (0.433 μm to 2.19 μm) with four 10 m visible and near-infrared bands, six 20 m red-edge, near-infrared (NIR), and short wave infrared bands, and three 60 m bands [
1]. The MSI red-edge bands are centered at 705 nm, 740 nm, and 783 nm, and are not present on the MSI heritage Landsat and Satellite Pour l’Observation de la Terre (SPOT) sensors. The red-edge encompasses the rapid change in vegetation reflectance from the red to the near infrared and is of interest for studies of chlorophyll and nitrogen content [
3,
4,
5], leaf area index [
6,
7,
8], and vegetation stress [
9], and also may be useful for certain classification applications such as for burned area mapping [
10,
11].
The Sentinel-2A and -2B MSI remote sensing systems are in sun-synchronous polar orbits and acquire images over a 20.6° field of view that results in directional reflectance effects over non-Lambertian surfaces. These effects, often described by the bidirectional reflectance distribution function (BRDF), can be non-trivial. In a recent study of 17 million pairs of forward and back scatter reflectance observations, pronounced visible, near-infrared, and short wave infrared BRDF effects were documented across the 290 km wide MSI swath [
12]. In this study, MSI red-edge BRDF effects are quantified using the same data that were extracted over 20° × 10° of southern Africa for two 10 day periods in January 2016 (acquired close to the solar principal plane when BRDF effects are maximal) and in April 2016 (acquired close to the orthogonal plane when BRDF effects are minimal).
The nadir BRDF-adjusted reflectance (NBAR), i.e., the reflectance at nadir (0° view zenith) for a specified solar zenith, nominally has no BRDF effects and therefore is well suited for applications that require consistent reflectance in space and time. The Moderate Resolution Imaging Spectral radiometer (MODIS) NBAR product is generated systematically on a global basis using BRDF model parameters that are derived by inversion against 16 days of MODIS observations [
13]. This approach will not work for Sentinel-2 MSI data because there are insufficient cloud-free MSI observations available every 16 days for BRDF model inversion [
12] and over longer periods the surface usually changes, precluding reliable BRDF model parameter estimations [
14,
15]. Recently, an empirical
c-factor approach was published to adjust Landsat reflectance to NBAR [
16]. The approach is based on multiplying the reflectance by the ratio of the reflectance modeled using MODIS BRDF spectral model parameters for the Landsat observed view zenith and for a nadir view. Global fixed BRDF model parameters were used and were defined by averaging, for each MODIS reflective wavelength band, the MODIS 500 m BRDF product (MCD43A1) spectral model parameters [
13] over the entire global land surface for 12 months [
16]. It was developed based on observations that BRDF shapes are sufficiently similar over the Landsat 15° field of view that a single global fixed set of MODIS BRDF spectral model parameters can be used regardless of the land cover or condition [
16]. Consequently, Landsat NBAR can be derived using the
c-factor approach for all the Landsat global long-term records and prior to the year 2000 availability of the MODIS BRDF product. The approach was subsequently demonstrated and used to correct Sentinel-2 MSI 10 m blue (490 nm), green (560 nm), red (665 nm), and near-infrared (NIR) (842 nm), in addition to the MSI 20 m short wave infrared (SWIR) (1610 nm and 2190 nm), reflectance to NBAR [
12]. The three MSI 20 m red-edge bands were not considered because there are no equivalent red-edge MODIS BRDF spectral model parameters.
In this study, the
c-factor approach is applied to the three MSI red-edge bands and used to adjust the red-edge reflectance to NBAR. MSI 705 nm, 740 nm, and 783 nm red-edge BRDF parameters are derived by linear wavelength-based interpolation of the red (645 nm) and NIR (858 nm) fixed global MODIS BRDF spectral model parameters. The efficacy of the interpolation is investigated by comparison with Polarization and Directionality of the Earth’s Reflectances (POLDER) red, red-edge and NIR BRDF spectral model parameters derived from a recently available POLDER BRDF database [
17]. The
c-factor NBAR approach is demonstrated considering the same MSI data used in [
12] and the view zenith variation in MSI red-edge reflectance quantified before (surface reflectance) and after BRDF correction (NBAR) are compared. The paper concludes with a discussion and implications of the study findings.
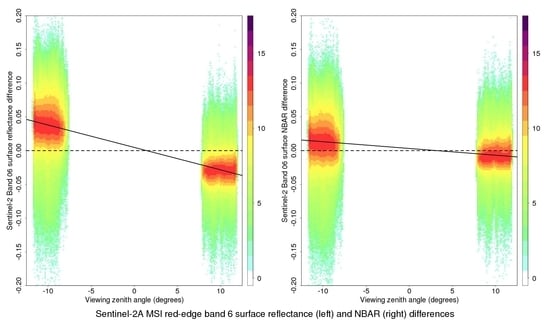
5. Discussion
BRDF effects are known to be present in most optical wavelength data including the red-edge [
33,
34,
35]. A previous study, undertaken using the same data and analytical techniques as this one, documented pronounced visible, near-infrared, and short wave infrared BRDF effects across the Sentinel-2A MSI field of view [
12]. In this study, we found similar BRDF effects in the three MSI red-edge bands, i.e., in MSI bands 5 (705 nm), 6 (740 nm), and 7 (783 nm). The B-F difference (that quantifies the average difference between surface reflectance in the forward and backward scatter directions at the MSI scan edges) varied among the three MSI red-edge bands from about 0.07 to 0.08 (January) and from about 0.02 to 0.03 (April). To provide context, the median red-edge surface reflectance for the Southern Africa study data was 0.22 (band 5), 0.25 (band 6), and 0.27 (band 7) for the January data and 0.18 (band 5), 0.25 (band 6), and 0.28 (band 7) for the April data. Further, the relative absolute percentage differences (
between the pairs of forward and backward scatter surface reflectance values varied among the red-edge bands from 13.3% to 14.9% (January) and 9.4% to 10.5% (April). The magnitude of these red-edge BRDF effects are not insignificant, and are comparable in magnitude to Landsat atmospheric correction errors [
44], and will likely be an issue for some Sentinel-2 MSI red-edge band applications. For example, the Sentinel-2 red-edge bands are of interest for studies of chlorophyll and nitrogen content, but statistical [
45,
46] and model inversion [
47] approaches will require minimization or incorporation of BRDF variations into the estimation approach.
For Sentinel-2 red-edge applications that consider subtle changes in reflectance, there is a need for the correction of BRDF effects. Recently, an empirical
c-factor approach was demonstrated to adjust MSI reflectance to a nadir view and specified solar zenith, i.e., to estimate nadir BRDF-adjusted reflectance (NBAR) [
12]. The approach was applied to the MSI visible, near-infrared, and short wave infrared reflectance and shown to reduce BRDF effects. It was developed based on observations that BRDF shapes are sufficiently similar over the narrow field of view that a single global fixed set of MODIS BRDF spectral model parameters can be used to provide a first-order BRDF correction with little sensitivity to the land cover type, condition, or surface disturbance [
16]. As there are no MODIS red-edge BRDF model parameters, a linear wavelength interpolation of the MODIS red and NIR global fixed BRDF parameters to the MSI red-edge band wavelengths was implemented. The developed method worked successfully. For all the MSI red-edge bands and for both months of data considered, the NBAR forward and backscatter differences were smaller than the corresponding surface reflectance differences. The approach can be applied in a computationally efficient manner globally. As noted in [
16], the approach should not be used to derive surface albedo, which would require a more accurate characterization of the surface BRDF than provided by fixed BRDF parameters.
The red-edge BRDF normalization to NBAR was not perfect because of factors including atmospheric correction and sensor calibration errors [
12]. In addition, a concern for this study is the appropriateness of the red-edge BRDF parameter generation by linear wavelength interpolation of the MODIS red and NIR BRDF parameters. To check that this simple interpolation approach was sufficient, an independent evaluation was undertaken using POLDER red, red-edge, and NIR fixed BRDF parameters. POLDER data were used because POLDER has a red-edge band with a multi-angular observation capability and the recent POLDER BRDF database makes access to POLDER data straightforward [
17]. The POLDER BRDF parameters derived from a year of globally distributed sites were used to compute global fixed red, red-edge, and NIR BRDF parameters. The linear wavelength interpolation approach was applied to the POLDER red and NIR fixed global BRDF parameters to derive interpolated POLDER red-edge BRDF parameters. Predicted reflectance values for a range of view zenith and solar zenith angles derived using the linearly interpolated and the fixed red-edge parameters were compared. The reflectances had similar BRDF shapes but quite different magnitudes, indicating that the POLDER wavelength dependence of the BRDF in the red-edge cannot be modelled reliably by the linear interpolation of red and NIR BRDF parameters. However, the corresponding POLDER derived
c-factors were linear and similar over the Sentinel-2 field of view, i.e., indicating that the interpolation approach is sufficient for the purposes of this research. More sophisticated interpolation approaches, for example, by fitting a spline or sigmoid curve to the MODIS visible, NIR, and SWIR BRDF parameters could be developed. However, in the absence of MODIS resolution multi-angular red-edge wavelength data required to test the interpolation, future research will need to proceed by modelling and/or by consideration of multi-spectral and multi-angular airborne and field measurements.
The majority of available medium resolution atmospheric correction algorithms assume a Lambertian surface and so the
c-factor approach can be meaningfully applied to the resulting atmospherically corrected data. We note, however, that surface BRDF and atmospheric corrections can be coupled but that this requires knowledge of the atmospheric constituents and/or sufficient multi-angular observations for model inversion [
37,
48,
49,
50], which are not available from Sentinel-2 data. Despite this, research on the use of more physically-based correction approaches, and ones that include corrections for topographic and adjacency effects, that are particularly evident in medium spatial resolution data [
51,
52], is recommended.
Finally, as observed in [
12,
16], the spatial scale difference between medium resolution Sentinel-2 or Landsat data and coarse resolution MODIS data means that the fixed global mean 12 month MODIS BRDF spectral model parameters provide an average representation of the local surface reflectance anisotropy. Certain geographic locations and times may have greater red-edge reflectance anisotropy than captured by the MODIS interpolated red-edge BRDF spectral model parameters. In addition, although more than 17 million pairs of forward and back scatter reflectance observations sensed close to the solar principal and orthogonal planes were considered, they did not include evergreen needleleaf and snow and ice land covers or observations sensed with solar zenith >50°. Therefore, the efficacy of the red-edge normalization described here may not be globally representative.