Numerical Investigation of Cell Encapsulation for Multiplexing Diagnostic Assays Using Novel Centrifugal Microfluidic Emulsification and Separation Platform
Abstract
:1. Introduction
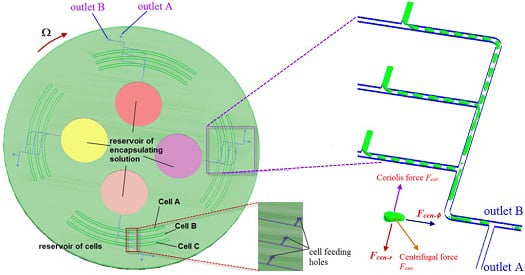
2. Design of the Centrifugal Microfluidic Device

3. Numerical Model
| Test Fluids | η (cP) | ρ (kg/m3) | σ (mN/m) (Silicon Oil and Water) |
|---|---|---|---|
| Silicon oil | 64.3 | 908.7 | 14.26 |
| Water | 1.003 | 997 |
4. Results and Discussion
4.1. Validation of Numerical Model
| Test Fluids | η (cP) | ρ (kg/m3) | σ (mN/m) (Sunflower Oil and Water) |
|---|---|---|---|
| Sunflower oil | 62.2 | 909 | 28.33 |
| Water | 1.09 | 1005 |

| Droplet Area (mm2) | #1 | #2 | #3 | #4 | #5 | #6 | #7 | Average | Standard Deviation | CV |
|---|---|---|---|---|---|---|---|---|---|---|
| Experiment | 0.078 | 0.081 | 0.078 | 0.086 | 0.078 | 0.085 | 0.076 | 0.080 | 0.004 | 0.046 |
| Simulation | 0.077 | 0.078 | 0.077 | 0.081 | 0.080 | 0.085 | 0.072 | 0.079 | 0.004 | 0.048 |
| Relative difference | 1.14% | 4.03% | 0.89% | 5.44% | 3.05% | 0.54% | 5.26% | 2.07% | 1.35% | 3.49% |

4.2. Encapsulation of Multiple Types of Cells



4.3. Droplet Sedimentation

5. Conclusions
Acknowledgments
Author Contributions
Conflicts of Interest
References
- Schramm, L.L. Emulsions, Foams, and Suspensions: Fundamentals and Applications, 1st ed.; Wiley-VCH: Weinheim, Germany, 2005. [Google Scholar]
- Song, H.; Chen, D.L.; Ismagilov, R.F. Reactions in droplets in microfluidic channels. Angew. Chem. Int. Ed. Engl. 2006, 45, 7336–7356. [Google Scholar] [CrossRef] [PubMed]
- Theberge, A.B.; Courtois, F.; Schaerli, Y.; Fischlechner, M.; Abell, C.; Hollfelder, F.; Huck, W.T. Microdroplets in microfluidics: An evolving platform for discoveries in chemistry and biology. Angew. Chem. Int. Ed. Engl. 2010, 49, 5846–5868. [Google Scholar] [CrossRef] [PubMed]
- Wang, J.T.; Wang, J.; Han, J.J. Fabrication of advanced particles and particle-based materials assisted by droplet-based microfluidics. Small 2011, 7, 1728–1754. [Google Scholar] [CrossRef] [PubMed]
- Li, W.; Greener, J.; Voicu, D.; Kumacheva, E. Multiple modular microfluidic (M3) reactors for the synthesis of polymer particles. Lab Chip 2009, 9, 2715–2721. [Google Scholar] [CrossRef] [PubMed]
- Shui, L.; Hayes, R.A.; Jin, M.; Zhang, X.; Bai, P.; van den Berg, A.; Zhou, G. Microfluidics for electronic paper-like displays. Lab Chip 2014, 14, 2374–2384. [Google Scholar] [CrossRef] [PubMed]
- Kim, S.H.; Lee, S.Y.; Yang, S.M.; Yi, G.R. Self-assembled colloidal structures for photonics. NPG Asia Mater. 2011, 3, 25–33. [Google Scholar] [CrossRef]
- Sacanna, S.; Pine, D.J. Shape-anisotropic colloids: Building blocks for complex assemblies. Curr. Opin. Colloid Interface Sci. 2011, 16, 96–105. [Google Scholar] [CrossRef]
- Stanway, R. Smart fluids: Current and future developments. Mater. Sci. Technol. 2004, 20, 931–939. [Google Scholar] [CrossRef]
- Chung, B.G.; Lee, K.H.; Khademhosseini, A.; Lee, S.H. Microfluidic fabrication of microengineered hydrogels and their application in tissue engineering. Lab Chip 2012, 12, 45–59. [Google Scholar] [CrossRef] [PubMed]
- Velasco, D.; Tumarkin, E.; Kumacheva, E. Microfluidic encapsulation of cells in polymer microgels. Small 2012, 8, 1633–1642. [Google Scholar] [CrossRef] [PubMed]
- Thangawng, A.L.; Howell, P.B.; Spillmann, C.M.; Naciri, J.; Ligler, F.S. UV polymerization of hydrodynamically shaped fibers. Lab Chip 2011, 11, 1157–1160. [Google Scholar] [CrossRef] [PubMed]
- Pardeike, J.; Hommoss, A.; Muller, R.H. Lipid nanoparticles (SLN, NLC) in cosmetic and pharmaceutical dermal products. Int. J. Pharm. 2009, 366, 170–184. [Google Scholar] [CrossRef] [PubMed]
- Duncanson, W.J.; Abbaspourrad, A.; Shum, H.C.; Kim, S.H.; Adams, L.L.A.; Weitz, D.A. Monodisperse gas-filled microparticles from reactions in double emulsions. Langmuir 2012, 28, 6742–6745. [Google Scholar] [CrossRef] [PubMed]
- Pessi, J.; Santos, H.A.; Miroshnyk, I.; Yliruusi, J.; Weitz, D.A.; Mirza, S. Microfluidics-assisted engineering of polymeric microcapsules with high encapsulation efficiency for protein drug delivery. Int. J. Pharm. 2014, 472, 82–87. [Google Scholar] [CrossRef] [PubMed]
- Gouin, S. Microencapsulation: industrial appraisal of existing technologies and trends. Trends Food Sci. Technol. 2004, 15, 330–347. [Google Scholar] [CrossRef]
- Chiu, D.T. Droplet nanolab for single-cell biology, single-organelle measurements, and single-molecules studies. Am. Chem. Soc. 2005, 230, U321. [Google Scholar]
- Rang, A.; Park, J.; Ju, J.; Jeong, G.S.; Lee, S.H. Cell encapsulation via microtechnologies. Biomaterials 2014, 35, 2651–2663. [Google Scholar]
- Abate, A.R.; Kutsovsky, M.; Seiffert, S.; Windbergs, M.; Pinto, L.F.V.; Rotem, A.; Utada, A.S.; Weitz, D.A. Synthesis of monodisperse microparticles from non-newtonian polymer solutions with microfluidic devices. Adv. Mater. 2011, 23, 1757–1760. [Google Scholar] [CrossRef] [PubMed]
- Whitesides, G.M. The origins and the future of microfluidics. Nature 2006, 442, 368–373. [Google Scholar] [CrossRef] [PubMed]
- Couture, O.; Faivre, M.; Pannacci, N.; Babataheri, A.; Servois, V.; Tabeling, P.; Tanter, M. Ultrasound internal tattooing. Med. Phys. 2011, 38, 1116–1123. [Google Scholar] [CrossRef] [PubMed]
- Ren, Y.; Liu, Z.; Shum, H.C. Breakup dynamics and dripping-to-jetting transition in a Newtonian/shear-thinning multiphase microsystem. Lab Chip 2015, 15, 121–134. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Roberts, C.C.; Rao, R.R.; Loewenberg, M.; Brooks, C.F.; Galambos, P.; Grillet, A.M.; Nemer, M.B. Comparison of monodisperse droplet generation in flow-focusing devices with hydrophilic and hydrophobic surfaces. Lab Chip 2012, 12, 1540–1547. [Google Scholar] [CrossRef] [PubMed]
- Wang, K.; Lu, Y.C.; Xu, J.H.; Tan, J.; Luo, G.S. Generation of micromonodispersed droplets and bubbles in the capillary embedded T-junction microfluidic devices. AICHE J. 2011, 57, 299–306. [Google Scholar] [CrossRef]
- Kobayashi, D.; Hiwatashi, R.; Asakura, Y.; Matsumoto, H.; Kuroda, C.; Shimada, Y.; Otake, K.; Shono, A. Preparation of oil-in-water emulsion by a two-step emulsification method. Kagaku Kogaku Ronbunshu 2015, 41, 241–245. [Google Scholar] [CrossRef]
- Li, Z.; Leshansky, A.M.; Metais, S.; Pismen, L.M.; Tabeling, P. Step-emulsification in a microfluidic device. Lab Chip 2015, 15, 1023–1031. [Google Scholar] [CrossRef] [PubMed]
- Utada, A.S.; Fernandez-Nieves, A.; Stone, H.A.; Weitz, D.A. Dripping to jetting transitions in coflowing liquid streams. Phys. Rev. Lett. 2007, 99, 094502. [Google Scholar] [CrossRef] [PubMed]
- Leung, W.W.F.; Ren, Y. Scale-up on mixing in rotating microchannel under subcritical and supercritical operating modes. Int. J. Heat Mass Transf. 2014, 77, 157–172. [Google Scholar] [CrossRef]
- Ren, Y.; Leung, W.W.F. Flow and mixing in rotating zigzag microchannel. Chem. Eng. J. 2013, 215, 561–578. [Google Scholar] [CrossRef]
- Ren, Y.; Leung, W.W.F. Numerical and experimental investigation on flow and mixing in batch-mode centrifugal microfluidics. Int. J. Heat Mass Transf. 2013, 60, 95–104. [Google Scholar] [CrossRef]
- Ren, Y.; Leung, W.W.F. Vortical flow and mixing in rotating milli- and micro-chambers. Comput. Fluids 2013, 79, 150–166. [Google Scholar] [CrossRef]
- Leung, W.W.F.; Ren, Y. Crossflow and mixing in obstructed and width-constricted rotating radial microchannel. Int. J. Heat Mass Transf. 2013, 64, 457–467. [Google Scholar] [CrossRef]
- Strohmeier, O.; Keller, M.; Schwemmer, F.; Zehnle, S.; Mark, D.; von Stetten, F.; Zengerle, R.; Paust, N. Centrifugal microfluidic platforms: Advanced unit operations and applications. Chem. Soc. Rev. 2015, 44, 6187–6229. [Google Scholar] [CrossRef] [PubMed]
- Gorkin, R.; Park, J.; Siegrist, J.; Amasia, M.; Lee, B.S.; Park, J.M.; Kim, J.; Kim, H.; Madou, M.; Cho, Y.K. Centrifugal microfluidics for biomedical applications. Lab Chip 2010, 10, 1758–1773. [Google Scholar]
- Ren, Y.; Chow, L.M.C.; Leung, W.W.F. Cell culture using centrifugal microfluidic platform with demonstration on Pichia pastoris. Biomed. Microdevices 2013, 15, 321–337. [Google Scholar] [CrossRef] [PubMed]
- Chakraborty, D.; Chakraborty, S. Controlled microbubble generation on a compact disk. Appl. Phys. Lett. 2010, 97, 234103. [Google Scholar] [CrossRef]
- Haeberle, S.; Zengerle, R.; Ducree, J. Centrifugal generation and manipulation of droplet emulsions. Microfluid. Nanofluid. 2007, 3, 65–75. [Google Scholar] [CrossRef]
- Wang, G.H.; Ho, H.P.; Chen, Q.L.; Yang, A.K.L.; Kwok, H.C.; Wu, S.Y.; Kong, S.K.; Kwan, Y.W.; Zhang, X.P. A lab-in-a-droplet bioassay strategy for centrifugal microfluidics with density difference pumping, power to disc and bidirectional flow control. Lab Chip 2013, 13, 3698–3706. [Google Scholar] [CrossRef] [PubMed]
- Abi-Samra, K.; Kim, T.H.; Park, D.K.; Kim, N.; Kim, J.; Kim, H.; Cho, Y.K.; Madou, M. Electrochemical velocimetry on centrifugal microfluidic platforms. Lab Chip 2013, 13, 3253–3260. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Hugo, S.; Land, K.; Madou, M.; Kido, H. A centrifugal microfluidic platform for point-of-care diagnostic applications. S. Afr. J. Sci. 2014, 110, 42–48. [Google Scholar] [CrossRef]
- Schuler, F.; Paust, N.; Zengerle, R.; von Stetten, F. Centrifugal step emulsification can produce water in oil emulsions with extremely high internal volume fractions. Micromachines 2015, 6, 1180–1188. [Google Scholar] [CrossRef]
- Schuler, F.; Schwemmer, F.; Trotter, M.; Wadle, S.; Zengerle, R.; von Stetten, F.; Paust, N. Centrifugal step emulsification applied for absolute quantification of nucleic acids by digital droplet RPA. Lab Chip 2015, 15, 2759–2766. [Google Scholar] [CrossRef] [PubMed]
- Pulko, I.; Krajnc, P. High internal phase emulsion templating—A path to hierarchically porous functional polymers. Macromol. Rapid Commun. 2012, 33, 1731–1746. [Google Scholar] [CrossRef] [PubMed]
- Haeberle, S.; Naegelel, L.; Burger, R.; Zengerle, R.; Ducree, J. Alginate micro-bead fabrication on a centrifugal microfluidics platform. In Proceedings of the IEEE Twentieth Annual International Conference on Micro Electro Mechanical Systems, Hyogo, Japan, 21–25 January 2007; pp. 614–617.
- Mark, D.; Haeberle, S.; Zengerle, R.; Ducree, J.; Vladisavljevic, G.T. Manufacture of chitosan microbeads using centrifugally driven flow of gel-forming solutions through a polymeric micronozzle. J. Colloid Interface Sci. 2009, 336, 634–641. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Maeda, K.; Onoe, H.; Takinoue, M.; Takeuchi, S. Controlled synthesis of 3D multi-compartmental particles with centrifuge-based microdroplet formation from a multi-barrelled capillary. Adv. Mater. 2012, 24, 1340–1346. [Google Scholar] [CrossRef] [PubMed]
- Haeberle, S.; Naegele, L.; Burger, R.; von Stetten, F.; Zengerle, R.; Ducree, J. Alginate bead fabrication and encapsulation of living cells under centrifugally induced artificial gravity conditions. J. Microencapsul. 2008, 25, 267–274. [Google Scholar] [CrossRef] [PubMed]
- Sang, L.; Hong, Y.P.; Wang, F.J. Investigation of viscosity effect on droplet formation in T-shaped microchannels by numerical and analytical methods. Microfluid. Nanofluid. 2009, 6, 621–635. [Google Scholar] [CrossRef]
- Ducree, J.; Haeberle, S.; Brenner, T.; Glatzel, T.; Zengerle, R. Patterning of flow and mixing in rotating radial microchannels. Microfluid. Nanofluid. 2006, 2, 97–105. [Google Scholar] [CrossRef]
- Meier, M.; Yadigaroglu, G.; Smith, B.L. A novel technique for including surface tension in PLIC-VOF methods. Eur. J. Mech. B Fluids 2002, 21, 61–73. [Google Scholar] [CrossRef]
- Madou, M.; Zoval, J.; Jia, G.Y.; Kido, H.; Kim, J.; Kim, N. Lab on a CD. Annu. Rev. Biomed. Eng. 2006, 8, 601–628. [Google Scholar] [CrossRef] [PubMed]
- Sugiura, S.; Oda, T.; Izumida, Y.; Aoyagi, Y.; Satake, M.; Ochiai, A.; Ohkohchi, N.; Nakajima, M. Size control of calcium alginate beads containing living cells using micro-nozzle array. Biomaterials 2005, 26, 3327–3331. [Google Scholar] [CrossRef] [PubMed]
- Jose, B.M.; Cubaud, T. Formation and dynamics of partially wetting droplets in square microchannels. RSC Adv. 2014, 4, 14962–14970. [Google Scholar] [CrossRef]
- Bhattacharya, S.; Datta, A.; Berg, J.M.; Gangopadhyay, S. Studies on surface wettability of poly(dimethyl) siloxane (PDMS) and glass under oxygen-plasma treatment and correlation with bond strength. J. Microelectromech. Syst. 2005, 14, 590–597. [Google Scholar] [CrossRef]
- Phaechamud, T.; Savedkairop, C. Contact angle and surface tension of some solvents used in pharmaceuticals. Res. J. Pharm. Biol. Chem. Sci. 2012, 3, 513–529. [Google Scholar]
© 2016 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons by Attribution (CC-BY) license ( http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ren, Y.; Leung, W.W.F. Numerical Investigation of Cell Encapsulation for Multiplexing Diagnostic Assays Using Novel Centrifugal Microfluidic Emulsification and Separation Platform. Micromachines 2016, 7, 17. https://doi.org/10.3390/mi7020017
Ren Y, Leung WWF. Numerical Investigation of Cell Encapsulation for Multiplexing Diagnostic Assays Using Novel Centrifugal Microfluidic Emulsification and Separation Platform. Micromachines. 2016; 7(2):17. https://doi.org/10.3390/mi7020017
Chicago/Turabian StyleRen, Yong, and Wallace Woon Fong Leung. 2016. "Numerical Investigation of Cell Encapsulation for Multiplexing Diagnostic Assays Using Novel Centrifugal Microfluidic Emulsification and Separation Platform" Micromachines 7, no. 2: 17. https://doi.org/10.3390/mi7020017
APA StyleRen, Y., & Leung, W. W. F. (2016). Numerical Investigation of Cell Encapsulation for Multiplexing Diagnostic Assays Using Novel Centrifugal Microfluidic Emulsification and Separation Platform. Micromachines, 7(2), 17. https://doi.org/10.3390/mi7020017