On-Surface Locomotion of Particle Based Microrobots Using Magnetically Induced Oscillation
Abstract
:1. Introduction
2. Materials and Methods
2.1. Fabrication
2.2. Motion Control Using Magnetic Fields
2.3. Oscillatory Swimming Motion
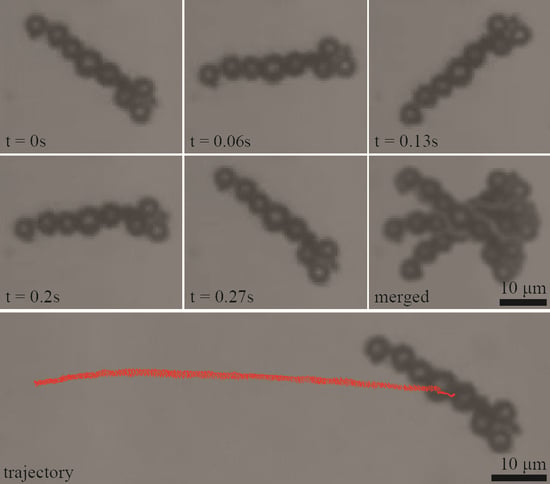
3. Results
3.1. Velocity Profile
3.2. Motion Control
4. Discussion
5. Conclusions
Supplementary Materials
Acknowledgments
Author Contributions
Conflicts of Interest
References
- Ferreira, A.; Agnus, J.; Chaillet, N.; Breguet, J.-M. A smart microrobot on chip: Design, identification, and control. Trans. Mechatron. 2004, 9, 508–519. [Google Scholar] [CrossRef]
- Zhang, H.; Hutmacher, D.W.; Chollet, F.; Poo, A.N.; Burdet, E. Microrobotics and MEMS-based fabrication techniques for scaffold-based tissue engineering. Macromol. Biosci. 2005, 5, 477–489. [Google Scholar] [CrossRef] [PubMed]
- Dogangil, G.; Ergeneman, O.; Abbott, J.J.; Pané, S.; Hall, H.; Muntwyler, S.; Nelson, B.J. Toward targeted retinal drug delivery with wireless magnetic microrobots. In Proceedings of the IEEE/RSJ International Conference on Intelligent Robots and Systems, Nice, France, 22–26 September 2008; pp. 1921–1926.
- Fusco, S.; Chatzipirpiridis, G.; Sivaraman, K.M.; Ergeneman, O.; Nelson, B.J.; Pané, S. Chitosan electrodeposition for microrobotic drug delivery. Adv. Healthc. Mater. 2013, 2, 1037–1044. [Google Scholar] [CrossRef] [PubMed]
- Kim, S.; Qiu, F.; Kim, S.; Ghanbari, A.; Moon, C.; Zhang, L.; Nelson, B.J.; Choi, H. Fabrication and characterization of magnetic microrobots for three-dimensional cell culture and targeted transportation. Adv. Mater. 2013, 25, 5863–5868. [Google Scholar] [CrossRef] [PubMed]
- Liu, X.; Kim, K.; Zhang, Y.; Sun, Y. Nanonewton force sensing and control in microrobotic cell manipulation. Int. J. Robot. Res. 2009, 28, 1065–1076. [Google Scholar]
- Martel, S.; Mathieu, J.-B.; Felfoul, O.; Chanu, A.; Aboussouan, E.; Tamaz, S.; Pouponneau, P.; Yahia, L.; Beaudoin, G.; Soulez, G. Automatic navigation of an untethered device in the artery of a living animal using a conventional clinical magnetic resonance imaging system. Appl. Phys. Lett. 2007, 90, 114105. [Google Scholar] [CrossRef]
- Grady, M.; Howard, M., III; Molloy, J.; Ritter, R.; Quate, E.; Gillies, G. Nonlinear magnetic stereotaxis: Three-dimensional, in vivo remote magnetic manipulation of a small object in canine brain. Med. Phys. 1990, 17, 405–415. [Google Scholar] [CrossRef] [PubMed]
- Mathieu, J.-B.; Beaudoin, G.; Martel, S. Method of propulsion of a ferromagnetic core in the cardiovascular system through magnetic gradients generated by an mri system. Trans. Biomed. Eng. 2006, 53, 292–299. [Google Scholar] [CrossRef] [PubMed]
- Purcell, E.M. Life at low reynolds number. Am. J. Phys. 1977, 45, 3–11. [Google Scholar] [CrossRef]
- Tottori, S.; Zhang, L.; Qiu, F.; Krawczyk, K.K.; Franco-Obregón, A.; Nelson, B.J. Magnetic helical micromachines: Fabrication, controlled swimming, and cargo transport. Adv. Mater. 2012, 24, 811–816. [Google Scholar] [CrossRef] [PubMed]
- Ghosh, A.; Fischer, P. Controlled propulsion of artificial magnetic nanostructured propellers. Nano Lett. 2009, 9, 2243–2245. [Google Scholar] [CrossRef] [PubMed]
- Cheang, U.K.; Roy, D.; Lee, J.H.; Kim, M.J. Fabrication and magnetic control of bacteria-inspired robotic microswimmers. Appl. Phys. Lett. 2010, 97, 213704. [Google Scholar] [CrossRef]
- Temel, F.Z.; Yesilyurt, S. Magnetically actuated micro swimming of bio-inspired robots in mini channels. In Proceedings of the IEEE International Conference on Mechatronics (ICM), Istanbul, Turkey, 13–15 April 2011; pp. 342–347.
- Peyer, K.E.; Zhang, L.; Nelson, B.J. Localized non-contact manipulation using artificial bacterial flagella. Appl. Phys. Lett. 2011, 99, 174101. [Google Scholar] [CrossRef]
- Gao, W.; Feng, X.; Pei, A.; Kane, C.R.; Tam, R.; Hennessy, C.; Wang, J. Bioinspired helical microswimmers based on vascular plants. Nano Lett. 2013, 14, 305–310. [Google Scholar] [CrossRef] [PubMed]
- Yan, X.; Zhou, Q.; Yu, J.; Xu, T.; Deng, Y.; Tang, T.; Feng, Q.; Bian, L.; Zhang, Y.; Ferreira, A. Magnetite nanostructured porous hollow helical microswimmers for targeted delivery. Adv. Funct. Mater. 2015, 25, 5333–5342. [Google Scholar] [CrossRef]
- Li, J.; Sattayasamitsathit, S.; Dong, R.; Gao, W.; Tam, R.; Feng, X.; Ai, S.; Wang, J. Template electrosynthesis of tailored-made helical nanoswimmers. Nanoscale 2014, 6, 9415–9420. [Google Scholar] [CrossRef] [PubMed]
- Barbot, A.; Decanini, D.; Hwang, G. On-chip microfluidic multimodal swimmer toward 3D navigation. Sci. Rep. 2016, 6, 19041. [Google Scholar] [CrossRef] [PubMed]
- Steager, E.B.; Sakar, M.S.; Kumar, V.; Pappas, G.J.; Kim, M.J. Electrokinetic and optical control of bacterial microrobots. J. Micromech. Microeng. 2011, 21, 035001. [Google Scholar] [CrossRef]
- Kim, H.; Cheang, U.K.; Julius, A.A.; Kim, M.J. Dynamic obstacle avoidance for bacteria-powered microrobots. In Proceedings of the IEEE/RSJ International Conference on Intelligent Robots and Systems, Hamburg, Germany, 28 September–2 October 2015; pp. 2000–2005.
- Kim, H.; Kim, M.J. Electric field control of bacteria-powered microrobots using a static obstacle avoidance algorithm. Trans. Robot. 2016, 32, 125–137. [Google Scholar] [CrossRef]
- Kim, D.H.; Cheang, U.K.; Kohidai, L.; Byun, D.; Kim, M.J. Artificial magnetotactic motion control of tetrahymena pyriformis using ferromagnetic nanoparticles: A tool for fabrication of microbiorobots. Appl. Phys. Lett. 2010, 97, 173702. [Google Scholar] [CrossRef]
- Diller, E.; Floyd, S.; Pawashe, C.; Sitti, M. Control of multiple heterogeneous magnetic microrobots in two dimensions on nonspecialized surfaces. IEEE Trans. Robot. 2012, 28, 172–182. [Google Scholar] [CrossRef]
- Leoni, M.; Kotar, J.; Bassetti, B.; Cicuta, P.; Lagomarsino, M.C. A basic swimmer at low reynolds number. Soft Matter 2009, 5, 472–476. [Google Scholar] [CrossRef]
- Cheang, U.K.; Kim, M. Self-assembly of robotic micro- and nanoswimmers using magnetic nanoparticles. J. Nanopart. Res. 2015, 17, 1–11. [Google Scholar] [CrossRef]
- Mori, N.; Kuribayashi, K.; Takeuchi, S. Artificial flagellates: Analysis of advancing motions of biflagellate micro-objects. Appl. Phys. Lett. 2010, 96, 083701. [Google Scholar] [CrossRef]
- Ismagilov, R.F.; Schwartz, A.; Bowden, N.; Whitesides, G.M. Autonomous movement and self-assembly. Angew. Chem. 2002, 114, 674–676. [Google Scholar] [CrossRef]
- Paxton, W.F.; Kistler, K.C.; Olmeda, C.C.; Sen, A.; St. Angelo, S.K.; Cao, Y.; Mallouk, T.E.; Lammert, P.E.; Crespi, V.H. Catalytic nanomotors: Autonomous movement of striped nanorods. J. Am. Chem. Soc. 2004, 126, 13424–13431. [Google Scholar] [CrossRef] [PubMed]
- Wang, Y.; Hernandez, R.M.; Bartlett, D.J.; Bingham, J.M.; Kline, T.R.; Sen, A.; Mallouk, T.E. Bipolar electrochemical mechanism for the propulsion of catalytic nanomotors in hydrogen peroxide solutions. Langmuir 2006, 22, 10451–10456. [Google Scholar] [CrossRef] [PubMed]
- Orozco, J.; García-Gradilla, V.; D’Agostino, M.; Gao, W.; Cortés, A.; Wang, J. Artificial enzyme-powered microfish for water-quality testing. ACS Nano 2012, 7, 818–824. [Google Scholar] [CrossRef] [PubMed]
- Manesh, K.M.; Cardona, M.; Yuan, R.; Clark, M.; Kagan, D.; Balasubramanian, S.; Wang, J. Template-assisted fabrication of salt-independent catalytic tubular microengines. ACS Nano 2010, 4, 1799–1804. [Google Scholar] [CrossRef] [PubMed]
- Solovev, A.A.; Mei, Y.; Bermúdez Ureña, E.; Huang, G.; Schmidt, O.G. Catalytic microtubular jet engines self-propelled by accumulated gas bubbles. Small 2009, 5, 1688–1692. [Google Scholar] [CrossRef] [PubMed]
- Gibbs, J.; Zhao, Y.-P. Autonomously motile catalytic nanomotors by bubble propulsion. Appl. Phys. Lett. 2009, 94, 163104. [Google Scholar] [CrossRef]
- Kline, T.R.; Paxton, W.F.; Mallouk, T.E.; Sen, A. Catalytic nanomotors: Remote-controlled autonomous movement of striped metallic nanorods. Angew. Chem. 2005, 117, 754–756. [Google Scholar] [CrossRef]
- Dreyfus, R.; Baudry, J.; Roper, M.L.; Fermigier, M.; Stone, H.A.; Bibette, J. Microscopic artificial swimmers. Nature 2005, 437, 862–865. [Google Scholar] [CrossRef] [PubMed]
- Gao, W.; Kagan, D.; Pak, O.S.; Clawson, C.; Campuzano, S.; Chuluun-Erdene, E.; Shipton, E.; Fullerton, E.E.; Zhang, L.; Lauga, E.; et al. Cargo-towing fuel-free magnetic nanoswimmers for targeted drug delivery. Small 2012, 8, 460–467. [Google Scholar] [CrossRef] [PubMed]
- Khalil, I.S.; Dijkslag, H.C.; Abelmann, L.; Misra, S. Magnetosperm: A microrobot that navigates using weak magnetic fields. Appl. Phys. Lett. 2014, 104, 223701. [Google Scholar] [CrossRef]
- Khalil, I.S.; Tabak, A.F.; Hosney, A.; Klingner, A.; Shalaby, M.; Abdel-Kader, R.M.; Serry, M.; Sitti, M. Targeting of cell mockups using sperm-shaped microrobots in vitro. In Proceedings of the 6th IEEE International Conference on Biomedical Robotics and Biomechatronics (BioRob), UTown, Singapore, 26–29 June 2016; pp. 495–501.
- Khalil, I.S.; Tabak, A.F.; Hosney, A.; Mohamed, A.; Klingner, A.; Ghoneima, M.; Sitti, M. Sperm-shaped magnetic microrobots: Fabrication using electrospinning, modeling, and characterization. In Proceedings of the IEEE International Conference on Robotics and Automation (ICRA), Stockholm, Sweden, 16–21 May 2016.
- Huang, H.-W.; Sakar, M.S.; Petruska, A.J.; Pané, S.; Nelson, B.J. Soft micromachines with programmable motility and morphology. Nat. Commun. 2016, 7, 12263. [Google Scholar] [CrossRef] [PubMed]
- Cheang, U.K.; Meshkati, F.; Fu, H.; Kim, M. Magnetic control of rigid achiral microswimmers. Bull. Am. Phys. Soc. 2013, 58, 18. [Google Scholar]
- Rodenborn, B.; Chen, C.-H.; Swinney, H.L.; Liu, B.; Zhang, H. Propulsion of microorganisms by a helical flagellum. Proc. Natl. Acad. Sci. USA 2013, 110, E338–E347. [Google Scholar] [CrossRef] [PubMed]
- Liu, B.; Breuer, K.S.; Powers, T.R. Propulsion by a helical flagellum in a capillary tube. Phys. Fluids 2014, 26, 011701. [Google Scholar] [CrossRef]
- Duprat, C.; Shore, H.A. Fluid-Structure Interactions In Low-Reynolds-Number Flows; Royal Society of Chemistry: London, UK, 2015; p. 483. [Google Scholar]
- Bray, D. Cell Movements: From Molecules to Motility; Garland Science: New York, NY, USA, 2001; p. 372. [Google Scholar]
- Cheang, U.K.; Lee, K.; Julius, A.A.; Kim, M.J. Multiple-robot drug delivery strategy through coordinated teams of microswimmers. Appl. Phys. Lett. 2014, 105, 083705. [Google Scholar] [CrossRef]
- Cheang, U.K.; Meshkati, F.; Kim, H.; Lee, K.; Fu, H.C.; Kim, M.J. Versatile microrobotics using simple modular subunits. Sci. Rep. 2016, 30472. [Google Scholar] [CrossRef] [PubMed]
- Wang, Y.; Gao, Y.; Wyss, H.; Anderson, P.; den Toonder, J. Out of the cleanroom, self-assembled magnetic artificial cilia. Lab Chip 2013, 13, 3360–3366. [Google Scholar] [CrossRef] [PubMed]
- Vilfan, M.; Potočnik, A.; Kavčič, B.; Osterman, N.; Poberaj, I.; Vilfan, A.; Babič, D. Self-assembled artificial cilia. Proc. Natl. Acad. Sci. USA 2010, 107, 1844–1847. [Google Scholar] [CrossRef] [PubMed]
- Babataheri, A.; Roper, M.; Fermigier, M.; Du Roure, O. Tethered fleximags as artificial cilia. J. Fluid Mech. 2011, 678, 5–13. [Google Scholar] [CrossRef]
- Coq, N.; Bricard, A.; Delapierre, F.-D.; Malaquin, L.; Du Roure, O.; Fermigier, M.; Bartolo, D. Collective beating of artificial microcilia. Phys. Rev. Lett. 2011, 107, 014501. [Google Scholar] [CrossRef] [PubMed]
- Abbott, J.J.; Peyer, K.E.; Lagomarsino, M.C.; Zhang, L.; Dong, L.; Kaliakatsos, I.K.; Nelson, B.J. How should microrobots swim? Int. J. Robot. Res. 2009, 28, 1434–1447. [Google Scholar] [CrossRef]
- Nelson, B.J.; Kaliakatsos, I.K.; Abbott, J.J. Microrobots for minimally invasive medicine. Annu. Rev. Biomed. Eng. 2010, 12, 55–85. [Google Scholar] [CrossRef] [PubMed]
- Griffiths, D.J.; College, R. Introduction to Electrodynamics; Prentice Hall: Upper Saddle River, NJ, USA, 1999; Volume 3. [Google Scholar]





| Frequency | Mean Velocity (µm/s) | Standard Deviation (µm/s) | Coefficient of Variations |
|---|---|---|---|
| 10 | 0.9284 | 0.4478 | 0.4824 |
| 20 | 2.3973 | 0.9301 | 0.3880 |
| 30 | 3.8580 | 0.9198 | 0.2384 |
© 2017 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license ( http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Cheang, U.K.; Ali, J.; Kim, H.; Rogowski, L.; Kim, M.J. On-Surface Locomotion of Particle Based Microrobots Using Magnetically Induced Oscillation. Micromachines 2017, 8, 46. https://doi.org/10.3390/mi8020046
Cheang UK, Ali J, Kim H, Rogowski L, Kim MJ. On-Surface Locomotion of Particle Based Microrobots Using Magnetically Induced Oscillation. Micromachines. 2017; 8(2):46. https://doi.org/10.3390/mi8020046
Chicago/Turabian StyleCheang, U Kei, Jamel Ali, Hoyeon Kim, Louis Rogowski, and Min Jun Kim. 2017. "On-Surface Locomotion of Particle Based Microrobots Using Magnetically Induced Oscillation" Micromachines 8, no. 2: 46. https://doi.org/10.3390/mi8020046
APA StyleCheang, U. K., Ali, J., Kim, H., Rogowski, L., & Kim, M. J. (2017). On-Surface Locomotion of Particle Based Microrobots Using Magnetically Induced Oscillation. Micromachines, 8(2), 46. https://doi.org/10.3390/mi8020046