Magnetocaloric Effect, Magnetoresistance of Sc_0.28Ti_0.72Fe₂, and Phase Diagrams of Sc_0.28Ti_0.72Fe_2−xT_x Alloys with T = Mn or Co

Abstract

(Sc,Ti)Fe₂ Laves phases present a relatively unique case of first-order ferro-ferromagnetic transition originating from an instability of the Fe moment. In addition to large magnetoelastic effects making them potential negative thermal expansion materials, here, we show that Sc_0.28Ti_0.72Fe₂ and related alloys also present sizable magnetocaloric and magnetoresistance effects. Both effects are found substantially larger at the ferro-ferromagnetic transition (T_t1) than near the Curie temperature T_C, yet they remain limited in comparison to other classes of giant magnetocaloric materials. We suggest a strategy to improve these properties by bringing the transition at T_t1 close to T_C, and test its possible realization by Co or Mn for Fe substitutions. The structural and magnetic phase diagrams of Sc_0.28Ti_0.72Fe_2−xT_x alloys with T = Mn or Co are explored. Substitutions for Fe by adjacent Mn or Co elements give rise to a breakdown of the long-range ferromagnetic order, as well as a swift disappearance of finite moment magnetism.

1. Introduction

Laves phases, AB₂, form a particularly large materials family exhibiting a vast array of intriguing properties, including those related to magnetism [1,2]. Transition-metal-based Laves phases AT₂ (with A an early 3, 4, or 5d transition metal and T a late 3d transition metal) are a typical example of this richness in properties since the crystal, electronic, and magnetic structures of binary and ternary alloys are highly sensitive to chemical composition. Hexagonal C14 transition-metal-based Laves phases such as Hf_1−xTa_xFe₂ or Hf_1−xNb_xFe₂ show a first-order ferromagnetic (FM) to antiferromagnetic (AFM) transition when increasing the temperature and have attracted particular interest in recent years for the specific mechanisms of this transition [3,4,5,6,7,8,9,10,11] and the associated magnetocaloric [4,12], giant negative expansion [9], or magnetoresistance effects [5]. On the other hand, Sc_1−xTi_xFe₂ Laves phases were intensively studied from the 1970s to 1990s for their complex magnetic phase diagram, in particular the compositions with 0.6 ≤ x ≤ 0.75 showing a ferromagnetic–paramagnetic transition around 300–400 K, and an additional, and quite unique, discontinuous ferromagnetic–ferromagnetic transition (T_t1) around 50–120 K with a change in amplitude of the magnetic moment [13,14,15,16]. This FM–FM transition is associated with a large magnetoelastic effect and corresponds to an increase upon cooling of the Fe magnetic moments from ~0.9 µ_B/Fe to ~1.3 µ_B/Fe, breaking down a local moment description. Local probes such as Mössbauer spectroscopy have shown that the Fe moment in the 2a position is highly dependent on the composition [14], and neutron diffraction experiments revealed that an instability of the Fe moment in the 2a position occurs as a function of temperature [17]. Interestingly, similar Fe moment instabilities are believed to be at the very heart of the exceptionally strong magnetoelastic first-order transitions and giant magnetocaloric effects observed in MnFe(P,Si) and La(Fe,Si)₁₃ materials systems [18,19].

In Sc_0.35Ti_0.65Fe_1.95, the low-temperature FM–FM first-order transition (T_t1) is accompanied by a large cell-volume contraction upon heating (ΔV/V ~ −1.1%) [15] and sizable transition entropy ΔS_tr estimated between 8 and 16 Jkg⁻¹K⁻¹ [20], which makes these materials interesting candidates for negative thermal expansion or magnetocaloric applications. It is, however, needed to find control parameters to adjust T_t1 and T_C transition temperatures. Ideally, one should aim to make both transitions coincide in order to create a synergy between the magnetic moment fluctuation at T_t1 and the change in magnetic order at T_C, which could potentially lead to a first-order magnetic transition with a large discontinuity in magnetization. Due to the large cell-volume contraction upon heating at T_t1, it could have been anticipated that negative chemical pressure such as that induced by Mn for Fe substitution would increase the T_t1 transition temperature. However, while the evolution of T_t1 and T_C due to alloying in Sc_1−zTi_zFe₂ ternaries [17,20], high pressure [21], or high magnetic fields [22] have been established, the effect of 3d electron count on the Fe sites remains unclear. In particular, AT₂ Laves phases are known to present complex structural or magnetic phase diagrams with competitions between different structural phases (C14/C15), different magnetic orders (FM, AFM, paramagnetic, or short-range magnetism, etc.), and different degrees of localization of the magnetic moment. Accurate predictions of the outcome of substitutions are thus virtually impossible at present. In this work, we explore the influence of Mn or Co for Fe substitutions in Sc_0.28Ti_0.72Fe_2−xT_x and establish structural and magnetic phase diagrams of these quaternary alloys.

2. Materials and Methods

Sc_0.28Ti_0.72Fe_2−xT_x with T = Mn and Co were prepared by arc-melting in a purified Ar atmosphere of commercial staring materials with purity greater than 99.9% (from the Baotou Research Institute of Rare Earths (Baotou, China) for Sc, and from Alfa Aesar (Haverhill, MA, USA) for the other transition metals). Each button is melted and stirred 5 times. For the parent composition Sc_0.28Ti_0.72Fe₂, the structural and magnetic properties were compared for samples: (i) as-cast, (ii) annealed at 1000 °C for 4 days and furnace cooled, and (iii) annealed at 1000 °C for 4 days and quenched. The three heat treatments led to comparable cell parameters, magnetic saturation, and transition temperatures. The first-order FM–FM transition T_t1 is, however, broader for (ii) furnace cooling than for (i) and (iii) as-cast and quenched samples, the latter two being on par with each other. Accordingly, for most compositions, only as-cast samples are reported, with a few exceptions indicated in the manuscript.

Powder X-ray diffraction experiments were carried out in reflection on an Empyrean diffractometer (Panalytical, Almelo, The Netherlands) using Cu Kα radiation. A TTK600 (Anton Paar, Graz, Austria) low-temperature chamber was used for temperature-dependent measurements. The powders used for XRD experiments were sieved below 36 μm in diameter. The diffraction patterns were analyzed using Fullprof software (version 7.20) [23], and VESTA software (version 3.4.6) was used for crystal structure representation [24]. Physical properties measurements were carried out using a Verslab system (Quantum Design, San Diego, CA, USA) equipped with a vibrating sample magnetometer or AC resistance options (with 4 points contacted with Cerasolzer^® (Kuroda Techno Co. Ltd., Yokohama, Japan) and ultrasonic iron), for magnetization and resistivity measurements, respectively.

3. Results and Discussion

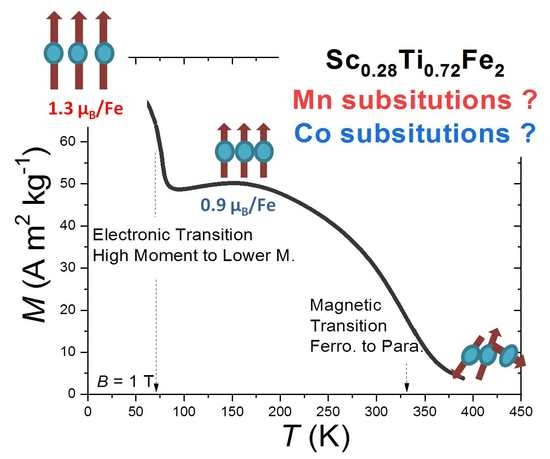
3.1. Magnetocaloric and Magnetoresitance of Sc_0.28Ti_0.72Fe₂ Ternary Alloy

Sc_0.28Ti_0.72Fe₂ was chosen as the starting composition for this study as, according to former reports [17,20], it offers a compromise between relatively high T_t1-transition temperature and sharpness of the transition. As illustrated in the next subsections, the cell parameters and basic magnetic properties of our Sc_0.28Ti_0.72Fe₂ sample are in line with former reports [17,20]. Figure 1 shows detailed magnetic data for Sc_0.28Ti_0.72Fe₂ around T_t1 and T_C. Though not particularly sharp, the T_t1 transition is a first-order magnetic transition (FOMT), as demonstrated by the relatively large thermal hysteresis of ~10 K. The shift of the transition due to the field dT_t1/dB is about +7.1(1) K/T, which is larger than the +4 K/T reported for Sc_0.25Ti_0.75Fe₂ [22] or +3.8 K/T Sc_0.35Ti_0.65Fe_1.95 [15]. Within Clausius–Clapeyron formalism, this results in a limited transition entropy ΔS_tr = −ΔM/(dT_t1/dB) ~2.8 Jkg⁻¹K⁻¹. Given that larger transition entropies were reported [22], this suggests that the strength of the FM–FM transition at T_t1 is highly sensitive to chemical composition, both the Sc/Ti ratio and Fe sub-stoichiometry. Limited transition entropy also results in a limited magnetocaloric effect as calculated from M_B(T) curves using the Maxwell relation [25], with ΔS = −1.4 and −2.2 Jkg⁻¹K⁻¹ at T_t1 for 1 and 2 T, respectively. The magnetocaloric effect is conventional, with a negative isothermal entropy change for a positive field change, both at T_t1 and T_C. Around T_C ≈ 325 K, the shape of the M_T(B) curves is typical of a second-order transition, and it corresponds to a magnetocaloric effect of ΔS = −0.4 and −0.8 Jkg⁻¹K⁻¹ for 1 and 2 T, respectively, spreading over a large temperature range. In comparison to other giant magnetocaloric materials [25], the magnetocaloric effect occurring at T_t1 and T_C is sizable, yet not among the best candidates known in each temperature range. For instance, in 1 T, a giant magnetocaloric effect of −10 up to −20 Jkg⁻¹K⁻¹ can be observed in MnFe(P,Si) and related materials [19,26,27], −18 Jkg⁻¹K⁻¹ in LaFe_11.44Si_1.56 [28], −13 Jkg⁻¹K⁻¹ in (Mn,Fe)(Fe,Ni)(Si,Ge,Al) alloys [29,30,31,32], or an inverse effect of +12 Jkg⁻¹K⁻¹ in FeRh [33] or +15 Jkg⁻¹K⁻¹ in Ni-Co-Mn-Ti alloys [34,35,36,37]. Part of the reason is that the magnetocaloric ΔS scales as dM/dT. At T_t1, the transition remains relatively sharp, about 15 K in width, but the change in magnetization is limited, approximately 0.3 μ_B/Fe. At T_C, the decrease in magnetization upon heating is larger (~0.8 μ_B/Fe) but spread over a much broader temperature range (nearly 150 K), leading to limited dM/dT. We thus believe that better magnetocaloric properties could be achieved in derivatives from (Sc,Ti)Fe₂ by bringing T_t1 and T_C close to each other, ideally by achieving a coupling between both transitions.

To illustrate the very different nature of the magnetic transitions at T_t1 and T_C, electrical resistivity (ρ) and magnetoresistance (MR) were measured and are presented in Figure 2. The temperature dependence of the resistivity is metallic, but a linear-like regime is not yet reached on the present data, suggesting a Debye temperature higher than 300 K. T_C does not correspond to any significant ρ anomaly; by contrast, a relatively discontinuous ρ increase of ~30% is observed at T_t1 with a thermal hysteresis in line with the first-order character of this transition. Due to the magnetic field sensitivity of T_t1, it results in a significant magnetoresistance whose maximum of −17% for ΔB = 3 T is reached near T_t1. In this low temperature range, the MR field dependence is also typical of that of a FOMT with a clear field-induced character and a finite magnetic hysteresis. By contrast, the magnetoresistance near T_C is limited, reaching a maximum of only −0.9%, as is usually found in metallic ferromagnets. The reduction in net magnetization at T_t1 is smaller than that at T_C, yet the MR is very significantly larger. This suggests that not only an evolution of magnon–electron interactions takes place at T_t1, but also that other contributions are involved, such as a change in magnon–phonon terms due to the large magnetoelastic effect occurring at T_t1.

3.2. Co for Fe Substitution in Sc_0.28Ti_0.72Fe₂

Figure 3 presents the powder diffraction patterns of Sc_0.28Ti_0.72Fe_2−xCo_x alloys and the corresponding cell volume and parameters. From x = 0 until x = 1, all XRD peaks can be indexed in the C14 hexagonal structure. The change from hexagonal to cubic C15 structure occurs around x = 1.5. This latter composition shows a coexistence of both phases (~35% hexagonal phase). After 3 days annealing at 1000 °C and quenching to avoid contamination from unreacted CoTi secondary phase, the fully substituted Sc_0.28Ti_0.72Co₂ sample is found to fully crystallize into the cubic C15 structure. Co substitution in Sc_0.28Ti_0.72Fe_2−xCo_x corresponds to a significant cell-volume contraction, in line with the smaller atomic radius of Co than that of Fe. The boundary between hexagonal and cubic structures marks out a large difference in volume (~1.2%). Within the hexagonal phase, the unit cell contraction appears relatively isotropic, as from x = 0 to 1 both a and c axes decrease, so that the lattice parameters ratio c/a experiences only a limited increase of about +0.07%.

Figure 4 presents the magnetic properties of Sc_0.28Ti_0.72Fe_2−xCo_x alloys. The unsubstituted sample x = 0 shows a Curie temperature near 325 K and the ferro-ferromagnetic transition T_t1 at 75 K in B = 1 T, in line with former reports on stoichiometric (Sc,Ti)Fe₂ or Fe-deficient (Sc,Ti)Fe_1.95 [13,14,15,16,20]. In addition, below ~150 K, one can notice a weak decrease in magnetization upon cooling before reaching T_t1. This leads to the appearance of a broad bump centered at T_f~150 K, also present in former studies, and which was ascribed to the development of a coexisting antiferromagnetic phase or of a canting [17,22]. In the range 0 < x ≤ 0.5, Co substitution leads to a rapid decrease in Curie temperature and a strong reduction in magnetization. Alloys with x > 0.5 no longer show signs of long-range ferromagnetic order on M(B) or M(T) curves, and no spontaneous magnetization could be observed on Arrott plots at 50 K (not shown). The change in crystal structure does not appear directly responsible for the loss of long-range magnetic order, as finite magnetic moments and ferromagnetism disappear within the compositional range of the C14 crystal structure. The fully substituted alloy Sc_0.28Ti_0.72Co₂ presents a very small magnetization (magnetization at room temperature is four orders of magnitude smaller than paramagnetic x = 0.5) and has only limited temperature dependence, recalling the Pauli paramagnetism of its TiCo₂ or ScCo₂ C15 parents [38,39]. The intermediate compositions with Co partially substituting Fe cannot be compared with closely related C14 ternaries such as TiFe_2−xCo_x, as Sc plays a critical role in stabilizing ferromagnetism in Sc_0.28Ti_0.72Fe_2−xCo_x with x ≤ 0.5. In addition, in contrast to TiFe_2−xCo_x with x ≤ 0.6 [40], no clear signature of long-range antiferromagnetic order is observed. The saturation magnetization at T = 50 K and B = 3 T is about 1.1 µ_B/Fe, 0.55, 0.43, and 0.32 µ_B per Fe or Co atom for x = 0, 0.1, 0.2, and 0.3, respectively, and the induced magnetization ~3 × 10⁻³ µ_B/Co for x = 2. The decrease in magnetization is faster than what could have been anticipated due to magnetic dilution of Fe by non-magnetic Co. In addition, the FM–FM transition at T_t1 is no longer observed in Co-substituted samples.

In (Sc,Ti)Fe₂ and (Sc,Ti)Fe_1.95, a typical signature of the Fe moment fluctuation at T_t1 was a large cell-volume contraction ΔV/V ~ −1.1% upon heating [15,16,17]. Powder X-ray diffraction experiments were carried out in Sc_0.28Fe_0.72Fe_1.9Co_0.1 between 100 and 480 K; see Figure 5. The absence of noticeable cell-volume anomaly further confirms the absence of the T_t1 transition in Co-substituted samples (in the investigated temperature range). The volume coefficient of thermal expansion ~ +15 ppm/K is nearly constant from 100 to 330 K, then increases in the paramagnetic phase +25 ppm/K, which is close to former reports on ternary alloys [15,16].

3.3. Mn for Fe Substitution in Sc_0.28Ti_0.72Fe₂

Figure 6 presents the crystal structure of Sc_0.28Ti_0.72Fe_2−yMn_y alloys determined from powder X-ray diffraction experiments. All alloys crystallize in the C14 crystal structure, so that Mn for Fe substitutions form a continuous solid solution. Some scatter on the cell-volume vs. composition curve is noticeable, most likely originating from two challenges met during the synthesis: (i) Mn evaporation occurs during the arc-melting process, which is only imperfectly compensated for by the addition of extra-manganese; (ii) Mn substitutions lead to the formation of a minor cubic impurity phase resembling FeTi alloys (up to ~4 w% in y = 1), on which annealing at 1000 °C has only minor effect. Nevertheless, Mn for Fe substitutions clearly show a cell-volume increase (+2.2%) due to an expansion of both a and c axes, but slightly anisotropic with an increase in the c/a ratio (+0.5%).

Figure 7 shows the magnetic properties of Sc_0.28Ti_0.72Fe_2−yMn_y alloys. Mn substitutions lead to a decrease in Curie temperature, in magnetization, and a disappearance of the T_t1 transition. Mn substitutions also drives the development of the coexisting antiferromagnetic-like phase at T_f toward lower temperatures, so that the magnetization in the finite magnetic field is found to increase around 100 K from y = 0 to y = 0.2. From y = 0.5 and above, the magnetization swiftly decreases; for Mn content larger than y ≥ 1, a long-range ferromagnetic order is no longer observed. In contrast to Ti(Fe,Mn)₂ alloys [40], no clear signature of bulk long-range antiferromagnetic order is found in the present Sc_0.28Ti_0.72Fe_2−yMn_y samples. The fully substituted sample Sc_0.28Ti_0.72Mn₂ does not exhibit a linear 1/χ Curie–Weiss behavior and is closer to a nearly temperature-independent paramagnet with χ₀ = 10⁻³ emu·mol⁻¹. This is in line with the “non-magnetic” Pauli paramagnetism reported for its TiMn₂ and ScMn₂ parents [41,42].

Figure 8 summarizes the magnetic data for Sc_0.28Ti_0.72Fe_2−xCo_x and Sc_0.28Ti_0.72Fe_2−yMn_y alloys. Both Co or Mn substitutions lead to a decrease in Curie temperature and a disappearance of the T_t1 transition. The magnetization at 50 K and 3 T as a function of Mn or Co substitutions is presented in Figure 8b in terms of average valence electrons per 2a and 6h sites. Substitutions for Fe lead to a swift reduction in magnetization. The negative chemical pressure due to Mn substitution on Fe does not lead to an increase in T_t1. Structural parameters such as cell volume are unlikely to be the primary driving force for the control of transition temperature or for the magnetization reduction, as Mn for Fe or Co for Fe substitutions should have resulted in opposite evolutions. An influence of the electron count on moment formation and ordering temperature is anticipated in such an itinerant system. The curve in Figure 8b vaguely resembles the Slater–Pauling curve for binary alloys of 3d elements; however, the slopes are much sharper than 1 or −1, preventing the description of the present alloys in a rigid band model. In other respects, the present results differ from that obtained in Sc_1−zTi_zFe₂ ternaries. In these, high Fe moments are observed with a titanium content varying from z = 0.75 to 0, with a salient T_t1 transition in the range 0.75 ≤ z ≤ 0.5 [17,20,22]. The d-electron count of the A atom in AFe₂ has a clear influence on the existence of T_t1 transition and on the transition temperature, with a finite range of possible compositions. By contrast, only 0.1 Mn or Co substitutions for Fe immediately led to the disappearance of the high moment state. The nonsimilar effect of Sc/Ti ratio or substitutions for Fe highlights that other mechanisms are involved in the occurrence of T_t1 than only the total electron count per f.u.

4. Conclusions

The magnetocaloric and magnetoresistance effects of Sc_0.28Ti_0.72Fe₂ Laves phase were investigated. Both effects were found to be substantially larger at the first-order ferro-ferromagnetic transition (T_t1) than near T_C. Though sizable, the magnetocaloric effect remains limited in comparison to other classes of giant magnetocaloric materials. We suggest a strategy to improve these properties by bringing the T_t1 transition temperature close to T_C and testing its possible realization by Co or Mn for Fe substitutions. Substitutions of Fe by adjacent elements Mn and Co, both of which do not carry a significant magnetic moment of their own in these Laves phases, give rise to a breakdown of the long-range ferromagnetic order, as well as a disappearance of the finite moment magnetism. Substitutions for Fe were found to be dissimilar to the effect of the Sc/Ti ratio, which indicates that other mechanisms than only the total electron count are involved in the occurrence of the ferro-ferromagnetic transition T_t1, keeping the door open for future independent control of the T_t1 and T_C transition temperatures.