X-ray Spectrum Reconstruction by Diamond Detectors with Linear Response to Dose Rate
Abstract
:1. Introduction
2. Materials and Methods
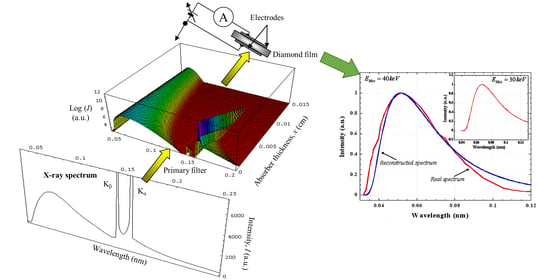
3. Results
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Sussmann, R.S. CVD Diamond for Electronic Devices and Sensors; John Wiley & Sons Ltd.: London, UK, 2009. [Google Scholar]
- Liu, Z.; Zhao, D.; Zhu, T.; Wang, J.; Yi, W.; Min, T.; Wang, H. Enhanced Responsivity of Diamond UV Detector Based on Regrown Lens Structure. IEEE Electron Device Lett. 2020, 41, 1829–1832. [Google Scholar] [CrossRef]
- Almaviva, S.; Marinelli, M.; Milani, E.; Prestopino, G.; Tucciarone, A.; Verona, C.; Verona-Rinati, G.; Angelone, M.; Pillon, M. Extreme UV single crystal diamond Schottky photodiode in planar and transverse configuration. Diam. Relat. Mater. 2010, 19, 78–82. [Google Scholar] [CrossRef]
- Liu, K.; Wang, W.; Dai, B.; Yang, L.; Zhao, J.; Xue, J.; Liu, B.; Lv, Z.; Bi, M.; Shu, G.; et al. Impact of UV spot position on forward and reverse photocurrent symmetry in a gold-diamond-gold detector. Appl. Phys. Lett. 2018, 113, 023501. [Google Scholar] [CrossRef]
- Bloomer, C.; Newton, M.E.; Rehm, G.; Salter, P.S. A single-crystal diamond X-ray pixel detector with embedded graphitic electrodes. J. Synchrotron Radiat. 2020, 27, 599–607. [Google Scholar] [CrossRef] [PubMed]
- Liu, L.Y.; Ouyang, X.P.; Zhang, J.F.; Jin, P.; Su, C.L. Properties comparison between nanosecond X-ray detectors of polycrystalline and single-crystal diamond. Diam. Relat. Mater. 2017, 73, 248–252. [Google Scholar] [CrossRef]
- Marinelli, M.; Milani, E.; Prestopino, G.; Verona, C.; Verona-Rinati, G.; Angelone, M.; Pillon, M.; Kachkanov, V.; Tartoni, N.; Benetti, M.; et al. X-ray beam monitor made by thin-film CVD single-crystal diamond. J. Synchrotron Radiat. 2012, 19, 1015–1020. [Google Scholar] [CrossRef] [Green Version]
- Tchouaso, M.T.; Kasiwattanawut, H.; Prelas, M.A. Energy response of diamond sensor to beta radiation. Appl. Radiat. Isot. 2018, 139, 66–69. [Google Scholar] [CrossRef]
- Chaudhuri, S.K.; Kleppinger, J.W.; Karadavut, O.; Mandal, K.C. Behavioral Contrast of Electron and Hole Transport in High-Resolution Diamond Detectors: A Biparametric Correlation Study. IEEE Electron Device Lett. 2021, 42, 200–203. [Google Scholar] [CrossRef]
- Bäni, L.; Alexopoulos, A.; Artuso, M.; Bachmair, F.; Bartosik, M.; Beck, H.; Bellini, V.; Belyaev, V.; Bentele, B.; Bes, A.; et al. A study of the radiation tolerance of poly-crystalline and single-crystalline CVD diamond to 800 MeV and 24 GeV protons. J. Phys. D Appl. Phys. 2019, 52, 465103. [Google Scholar] [CrossRef]
- Holmes, J.; Brown, J.; Koeck, F.A.; Johnson, H.; Benipal, M.K.; Kandlakunta, P.; Zaniewski, A.; Alarcon, R.; Cao, R.; Goodnick, S.M.; et al. Performance of 5-μm PIN diamond diodes as thermal neutron detectors. Nuclear Instrum. Methods Phys. Res. Sect. A Accel. Spectrom. Detect. Assoc. Equip. 2020, 961, 163601. [Google Scholar] [CrossRef]
- Muraro, A.; Giacomelli, L.; Nocente, M.; Rebai, M.; Rigamonti, D.; Belli, F.; Calvani, P.; Figueiredo, J.; Girolami, M.; Gorini, G.; et al. First neutron spectroscopy measurements with a pixelated diamond detector at JET. Rev. Sci. Instrum. 2016, 87, 11D833. [Google Scholar] [CrossRef] [Green Version]
- Girolami, M.; Bellucci, A.; Calvani, P.; Cazzaniga, C.; Rebai, M.; Rigamonti, D.; Tardocchi, M.; Pillon, M.; Trucchi, D.M. Mosaic diamond detectors for fast neutrons and large ionizing radiation fields. Phys. Status Solidi (A) 2015, 212, 2424–2430. [Google Scholar] [CrossRef]
- Angelone, M.; Pilotti, R.; Stacchi, F.; Pillon, M.; Klix, A.; Raj, P.; Loreti, S.; Pagano, G. Performance test of radiation detectors developed for ITER-TBM. Fusion Eng. Des. 2018, 136, 1386–1390. [Google Scholar] [CrossRef]
- Claps, G.; Murtas, F.; Foggetta, L.; Di Giulio, C.; Alozy, J.; Cavoto, G. Diamondpix: A CVD Diamond Detector With Timepix3 Chip Interface. IEEE Trans. Nuclear Sci. 2018, 65, 2743–2753. [Google Scholar] [CrossRef]
- Trucchi, D.M.; Allegrini, P.; Calvani, P.; Galbiati, A.; Oliver, K.; Conte, G. Very Fast and Priming-less Single-Crystal Diamond X-ray Dosimeters. IEEE Electron Device Lett. 2012, 33, 615–617. [Google Scholar] [CrossRef]
- Trucchi, D.M.; Allegrini, P.; Bellucci, A.; Calvani, P.; Galbiati, A.; Girolami, M. Resistant and sensitive single-crystal diamond dosimeters for ionizing radiation. Nuclear Instrum. Methods Phys. Res. Sect. A Accel. Spectrom. Detect. Assoc. Equip. 2013, 718, 373–375. [Google Scholar] [CrossRef]
- Pomorski, M.; Caylar, B.; Bergonzo, P. Super-thin single crystal diamond membrane radiation detectors. Appl. Phys. Lett. 2013, 103, 112106. [Google Scholar] [CrossRef] [Green Version]
- Bellucci, A.; Orlando, S.; Caputo, D.; Cappelli, E.; Trucchi, D.M. Dosimetric Performance of Single-Crystal Diamond X-Ray Schottky Photodiodes. IEEE Electron Device Lett. 2013, 34, 695–697. [Google Scholar] [CrossRef]
- Conte, G.; Spadaro, S.; Di Castro, E.; Carni, M. Zero-bias operation and beam energy dependence of diamond sensors. Key Eng. Mater. 2013, 543, 426–430. [Google Scholar] [CrossRef]
- Talamonti, C.; Kanxheri, K.; Pallotta, S.; Servoli, L. Diamond Detectors for Radiotherapy X-Ray Small Beam Dosimetry. Front. Phys. 2021, 9, 112. [Google Scholar] [CrossRef]
- Marinelli, M.; Prestopino, G.; Verona, C.; Verona-Rinati, G.; Ciocca, M.; Mirandola, A.; Mairani, A.; Raffaele, L.; Magro, G. Dosimetric characterization of a microDiamond detector in clinical scanned carbon ion beams. Med. Phys. 2015, 42, 2085. [Google Scholar] [CrossRef] [Green Version]
- Scaringella, M.; Zani, M.; Baldi, A.; Bucciolini, M.; Pace, E.; de Sio, A.; Talamonti, C.; Bruzzi, M. First dose-map measured with a polycrystalline diamond 2D dosimeter under an intensity modulated radiotherapy beam. Nuclear Instrum. Methods Phys. Res. Sect. A Accel. Spectrom. Detect. Assoc. Equip. 2015, 796, 89–92. [Google Scholar] [CrossRef]
- Ade, N. An investigation of the role of defect levels on the radiation response of synthetic diamond crystals when used as sensors for the detection of mammography X-rays. Appl. Radiat. Isot. 2017, 127, 237–244. [Google Scholar] [CrossRef] [PubMed]
- Sartori, A.F.; Orlando, S.; Bellucci, A.; Trucchi, D.M.; Abrahami, S.; Boehme, T.; Hantschel, T.; Vandervorst, W.; Buijnsters, J.G. Laser-Induced Periodic Surface Structures (LIPSS) on Heavily Boron-Doped Diamond for Electrode Applications. ACS Appl. Mater. Interfaces 2018, 10, 43236–43251. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Delfaure, C.; Pomorski, M.; de Sanoit, J.; Bergonzo, P.; Saada, S. Single crystal CVD diamond membranes for betavoltaic cells. Appl. Phys. Lett. 2016, 108, 252105. [Google Scholar] [CrossRef]
- Trucchi, D.M.; Cappelli, E.; Lisi, N.; Ascarelli, P. Feasibility of CVD diamond radiation energy conversion devices. Diam. Relat. Mater. 2006, 15, 1980–1985. [Google Scholar] [CrossRef]
- Bormashov, V.S.; Troschiev, S.Y.; Tarelkin, S.A.; Volkov, A.P.; Teteruk, D.V.; Golovanov, A.V.; Kuznetsov, M.S.; Kornilov, N.V.; Terentiev, S.A.; Blank, V.D. High power density nuclear battery prototype based on diamond Schottky diodes. Diam. Relat. Mater. 2018, 84, 41–47. [Google Scholar] [CrossRef]
- Mackenzie, G.R.; Kaluvan, S.; Martin, P.G.; Hutson, C.; Connolley, T.; Cattelan, M.; Dominguez-Andrade, H.; Martin, T.L.; Fox, N.A.; Scott, T.B. A diamond gammavoltaic cell utilizing surface conductivity and its response to different photon interaction mechanisms. Mater. Today Energy 2021, 21, 100688. [Google Scholar] [CrossRef]
- Trucchi, D.M.; Bellucci, A.; Girolami, M.; Calvani, P.; Cappelli, E.; Orlando, S.; Polini, R.; Silvestroni, L.; Sciti, D.; Kribus, A. Solar Thermionic-Thermoelectric Generator (ST2G): Concept, Materials Engineering, and Prototype Demonstration. Adv. Energy Mater. 2018, 8, 1802310. [Google Scholar] [CrossRef]
- Trucchi, D.M.; Scilletta, C.; Cappelli, E.; Merli, P.; Zoffoli, S.; Mattei, G.; Ascarelli, P. Optimization of the performance of CVD diamond electron multipliers. Diam. Relat. Mater. 2006, 15, 827–832. [Google Scholar] [CrossRef]
- Velardi, L.; Turco, V.; Monteduro, L.; Cicala, G.; Valentini, A.; Nassisi, V. Electron beams produced by innovative photocathodes based on nanodiamond layers. Phys. Rev. Accel. Beams 2019, 22, 093402. [Google Scholar] [CrossRef] [Green Version]
- Ukraintsev, E.; Kromka, A.; Janssen, W.; Haenen, K.; Takeuchi, D.; Bábor, P.; Rezek, B. Electron emission from H-terminated diamond enhanced by polypyrrole grafting. Carbon 2021, 176, 642–649. [Google Scholar] [CrossRef]
- Balmer, R.S.; Brandon, J.R.; Clewes, S.L.; Dhillon, H.K.; Dodson, J.M.; Friel, I.; Inglis, P.N.; Madgwick, T.D.; Markham, M.L.; Mollart, T.P.; et al. Chemical vapour deposition synthetic diamond: Materials, technology and applications. J. Phys. Condens. Matter Inst. Phys. J. 2009, 21, 364221. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Almaviva, S.; Ciancaglioni, I.; Consorti, R.; De Notaristefani, F.; Manfredotti, C.; Marinelli, M.; Milani, E.; Petrucci, A.; Prestopino, G.; Verona, C.; et al. Synthetic single crystal diamond dosimeters for Intensity Modulated Radiation Therapy applications. Nuclear Instrum. Methods Phys. Res. Sect. A Accel. Spectrom. Detect. Assoc. Equip. 2009, 608, 191–194. [Google Scholar] [CrossRef]
- Zani, M.; Bucciolini, M.; Casati, M.; Talamonti, C.; Marinelli, M.; Prestopino, G.; Tonnetti, A.; Verona-Rinati, G. A synthetic diamond diode in volumetric modulated arc therapy dosimetry. Med. Phys. 2013, 40, 092103. [Google Scholar] [CrossRef] [Green Version]
- Schreck, M. 3.11-Single Crystal Diamond Growth on Iridium. In Comprehensive Hard Materials; Sarin, V.K., Ed.; Elsevier: Oxford, UK, 2014; pp. 269–304. [Google Scholar]
- Berdermann, E.; Afanaciev, K.; Ciobanu, M.; Fischer, M.; Gsell, S.; Kiš, M.; Lagomarsino, S.; Lohmann, W.; Mayr, M.; Pomorski, M.; et al. Progress in detector properties of heteroepitaxial diamond grown by chemical vapor deposition on Ir/YSZ/Si(001) wafers. Diam. Relat. Mater. 2019, 97, 107420. [Google Scholar] [CrossRef]
- Li, R.; Li, L.; Chen, Z. Spectrum reconstruction method based on the detector response model calibrated by x-ray fluorescence. Phys. Med. Biol. 2017, 62, 1032–1045. [Google Scholar] [CrossRef]
- Michel, T.; Talla, P.T.; Firsching, M.; Durst, J.; Bohnel, M.; Anton, G. Reconstruction of X-ray spectra with the energy sensitive photon counting detector Medipix2. Nuclear Instrum. Methods Phys. Res. A 2009, 598, 510–514. [Google Scholar] [CrossRef]
- Ponchut, C. Correction of the charge sharing in photon-counting pixel detector data. Nuclear Instrum. Methods Phys. Res. Sect. A Accel. Spectrom. Detect. Assoc. Equip. 2008, 591, 311–313. [Google Scholar] [CrossRef]
- Cao, J.; Jiang, C.-Y.; Zhao, Y.-F.; Yang, Q.-W.; Yin, Z.-J. A novel X-ray tube spectra reconstruction method based on transmission measurements. Nuclear Sci. Tech. 2016, 27, 45. [Google Scholar] [CrossRef]
- Marsolat, F.; Tromson, D.; Tranchant, N.; Pomorski, M.; Bassinet, C.; Huet, C.; Derreumaux, S.; Chea, M.; Cristina, K.; Boisserie, G.; et al. Why diamond dimensions and electrode geometry are crucial for small photon beam dosimetry. J. Appl. Phys. 2015, 118, 234507. [Google Scholar] [CrossRef]
- Ade, N.; Nam, T.L. The influence of detector size relative to field size in small-field photon-beam dosimetry using synthetic diamond crystals as sensors. Radiat. Phys. Chem. 2015, 113, 6–13. [Google Scholar] [CrossRef]
- Hubbell, J.H.; Seltzer, S.M. X-Ray Mass Attenuation Coefficients. NISTIR 5632 2004. [Google Scholar] [CrossRef]
- Conte, G.; Rossi, M.C.; Salvatori, S.; Fabbri, F.; Loreti, S.; Ascarelli, P.; Cappelli, E.; Trucchi, D. Grain boundary transport in x-ray irradiated polycrystalline diamond. J. Appl. Phys. 2003, 93, 6078. [Google Scholar] [CrossRef]
- Bruzzi, M.; Menichelli, D.; Sciortino, S.; Lombardi, L. Deep levels and trapping mechanisms in chemical vapor deposited diamond. J. Appl. Phys. 2002, 91, 5765. [Google Scholar] [CrossRef] [Green Version]
- Behnke, T.; Oh, A.; Wagner, A.; Zeuner, W.; Bluhm, A.; Klages, C.P.; Paul, M.; Schafer, L. Development of diamond films for particle detector applications. Diam. Relat. Mater. 1998, 7, 1553–1557. [Google Scholar] [CrossRef]
- Shimaoka, T.; Kaneko, J.H.; Sato, Y.; Tsubota, M.; Shimmyo, H.; Chayahara, A.; Watanabe, H.; Umezawa, H.; Mokuno, Y. Fano factor evaluation of diamond detectors for alpha particles. Phys. Status Solidi (A) 2016, 213, 2629–2633. [Google Scholar] [CrossRef]
- Greening, J.R. The Determination of X-Ray Wavelength Distributions from Absorption Data. Proc. Phys. Soc. A 1950, 63, 1227–1234. [Google Scholar] [CrossRef]
- Fowler, J.F.; Attix, F.H. Solid state electrical conductivity dosimeters. In Radiation Dosimetry, 2nd ed.; Attix, F.H., Roesch, W.C., Eds.; Academic Press: New York, NY, USA, 1966. [Google Scholar]
- Macgillavry, C.H.; Rieck, G.D. International Tables For X-ray Crystallography; Volume 3: Physical and Chemical Tables; Kynoch Press for the International Union of Crystallography: Birmingham, UK, 1962; p. 362. [Google Scholar]




| Model/Fit Parameter | Units | Nichrome Absorbers | Aluminum Absorbers |
|---|---|---|---|
| Emax | keV | 40 | 30 |
| αNi(EKα) | cm−1 | 432.8 | 432.8 |
| αD(EKα) | cm−1 | 16.1 | 16.1 |
| αF(EKα) | cm−1 | 746.1 | 135.7 |
| αF(Emax) | cm−1 | 35.8 | 3.04 |
| x0 | cm | 2.13 × 10−3 | 5.11 × 10−2 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Trucchi, D.M.; Ascarelli, P. X-ray Spectrum Reconstruction by Diamond Detectors with Linear Response to Dose Rate. Crystals 2021, 11, 1258. https://doi.org/10.3390/cryst11101258
Trucchi DM, Ascarelli P. X-ray Spectrum Reconstruction by Diamond Detectors with Linear Response to Dose Rate. Crystals. 2021; 11(10):1258. https://doi.org/10.3390/cryst11101258
Chicago/Turabian StyleTrucchi, Daniele Maria, and Paolo Ascarelli. 2021. "X-ray Spectrum Reconstruction by Diamond Detectors with Linear Response to Dose Rate" Crystals 11, no. 10: 1258. https://doi.org/10.3390/cryst11101258
APA StyleTrucchi, D. M., & Ascarelli, P. (2021). X-ray Spectrum Reconstruction by Diamond Detectors with Linear Response to Dose Rate. Crystals, 11(10), 1258. https://doi.org/10.3390/cryst11101258