The Natural Breakup Length of a Steady Capillary Jet: Application to Serial Femtosecond Crystallography
Abstract
:1. Introduction
2. The Breakup Length of Both Ballistic and Flow-Focused Jets
Linear Global Instability vs. Nonlinear Breakup Length
3. Scaling Law
A Simplified Approach
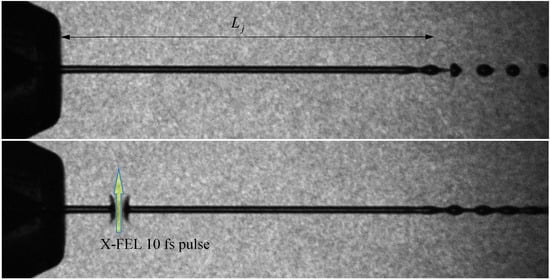
4. Experimental Validation
5. Concluding Remarks
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Plateau, J. Sur les figures d’equilibre d’une masse liquide sans pesanteur. Mem. Acad. R. Belg. Nouv. Ser. 1849, 23. [Google Scholar]
- Rayleigh, L. On the instability of jets. Proc. Lond. Math. Soc. 1878, s1-10, 4–13. [Google Scholar] [CrossRef] [Green Version]
- Eggers, J.; Villermaux, E. Physics of liquid jets. Rep. Prog. Phys. 2008, 71, 036601. [Google Scholar] [CrossRef]
- Chapman, H.N.; Fromme, P.; Barty, A.; White, T.A.; Kirian, R.A.; Aquila, A.; Hunter, M.S.; Schulz, J.; DePonte, D.P.; Weierstall, U.; et al. Femtosecond X-ray protein nanocrystallography. Nature 2011, 470, 73–79. [Google Scholar] [CrossRef]
- Boutet, S.; Lomb, L.; Williams, G.J.; Barends, T.R.; Aquila, A.; Doak, R.B.; Weierstall, U.; DePonte, D.P.; Steinbrener, J.; Shoeman, R.L.; et al. High-Resolution Protein Structure Determination by Serial Femtosecond Crystallography. Science 2012, 337, 362–364. [Google Scholar] [CrossRef] [Green Version]
- Schlichting, I. Serial femtosecond crystallography: The first five years. IUCrJ 2015, 2, 246–255. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Spence, J.C.H. XFELs for structure and dynamics in biology. IUCrJ 2017, 4, 322–339. [Google Scholar] [CrossRef] [PubMed]
- Aquila, A.; Hunter, M.S.; Doak, R.B.; Kirian, R.A.; Fromme, P.; White, T.A.; Andreasson, J.; Arnlund, D.; Bajt, S.; Barends, T.R.; et al. Time-resolved protein nanocrystallography using an X-ray free-electron laser. Opt. Express 2012, 20, 2706–2716. [Google Scholar] [CrossRef] [PubMed]
- Gati, C.; Oberthuer, D.; Yefanov, O.; Bunker, R.D.; Stellato, F.; Chiu, E.; Yeh, S.M.; Aquila, A.; Basu, S.; Bean, R.; et al. Atomic structure of granulin determined from native nanocrystalline granulovirus using an X-ray free-electron laser. Proc. Natl. Acad. Sci. USA 2017, 114, 2247–2252. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Seibert, M.M.; Ekeberg, T.; Maia, F.R.; Svenda, M.; Andreasson, J.; Jönsson, O.; Odić, D.; Iwan, B.; Rocker, A.; Westphal, D.; et al. Single mimivirus particles intercepted and imaged with an X-ray laser. Nature 2011, 470, 78–81. [Google Scholar] [CrossRef] [PubMed]
- Barends, T.R.; Foucar, L.; Ardevol, A.; Nass, K.; Aquila, A.; Botha, S.; Doak, R.B.; Falahati, K.; Hartmann, E.; Hilpert, M.; et al. Direct observation of ultrafast collective motions in CO myoglobin upon ligand dissociation. Science 2015, 350, 445–450. [Google Scholar] [CrossRef]
- Pande, K.; Hutchison, C.D.; Groenhof, G.; Aquila, A.; Robinson, J.S.; Tenboer, J.; Basu, S.; Boutet, S.; DePonte, D.P.; Liang, M.; et al. Femtosecond structural dynamics drives the trans/cis isomerization in photoactive yellow protein. Science 2016, 352, 725–729. [Google Scholar] [CrossRef] [Green Version]
- Stagno, J.R.; Liu, Y.; Bhandari, Y.R.; Conrad, C.E.; Panja, S.; Swain, M.; Fan, L.; Nelson, G.; Li, C.; Wendel, D.R.; et al. Structures of riboswitch RNA reaction states by mix-and-inject XFEL serial crystallography. Nature 2017, 541, 242–246. [Google Scholar] [CrossRef] [PubMed]
- Kupitz, C.; Olmos, J.L., Jr.; Holl, M.; Tremblay, L.; Pande, K.; Pandey, S.; Oberthür, D.; Hunter, M.; Liang, M.; Aquila, A.; et al. Structural enzymology using X-ray free electron lasers. Struct. Dyn. 2017, 4, 044003. [Google Scholar] [CrossRef] [PubMed]
- Wiedorn, M.O.; Awel, S.; Morgan, A.J.; Ayyer, K.; Gevorkov, Y.; Fleckenstein, H.; Roth, N.; Adriano, L.; Bean, R.; Beyerlein, K.R.; et al. Rapid sample delivery for megahertz serial crystallography at X-ray FELs. IUCrJ 2018, 5, 574–584. [Google Scholar] [CrossRef] [Green Version]
- Kalaaji, A.; Lopez, B.; Attane, P.; Soucemarianadin, A. Breakup length of forced liquid jets. Phys. Fluids 2003, 15, 2469–2479. [Google Scholar] [CrossRef]
- Garcia, F.; Gonzalez, H. Normal-mode linear analysis and initial conditions of capillary jets. J. Fluid Mech. 2008, 602, 81–117. [Google Scholar] [CrossRef]
- González, H.; García, F. The measurement of growth rates in capillary jets. J. Fluid Mech. 2009, 619, 179–212. [Google Scholar] [CrossRef]
- García, F.J.; González, H.; Castrejón-Pita, J.R.; Castrejón-Pita, A.A. The breakup length of harmonically stimulated capillary jets. App. Phys. Lett. 2014, 105, 094104. [Google Scholar] [CrossRef]
- Montanero, J.M.; Gañán-Calvo, A.M. Dripping, jetting and tip streaming. Rep. Prog. Phys. 2020, 83, 097001. [Google Scholar] [CrossRef]
- Gañán-Calvo, A.M. Generation of Steady Liquid Microthreads and Micron-Sized Monodisperse Sprays in Gas Streams. Phys. Rev. Lett. 1998, 80, 285–288. [Google Scholar] [CrossRef]
- Gordillo, J.M.; Pérez-Saborid, M.; Gañán-Calvo, A.M. Linear stability of co-flowing liquid-gas jets. J. Fluid Mech. 2001, 448, 23–51. [Google Scholar] [CrossRef]
- DePonte, D.P.; Weierstall, U.; Schmidt, K.; Warner, J.; Starodub, D.; Spence, J.C.H.; Doak, R.B. Gas dynamic virtual nozzle for generation of microscopic droplet streams. J. Phys. D Appl. Phys. 2008, 41, 195505. [Google Scholar] [CrossRef] [Green Version]
- Si, T.; Li, F.; Yin, X.Y.; Yin, X.Z. Modes in flow focusing and instability of coaxial liquid-gas jets. J. Fluid Mech. 2009, 629, 1–23. [Google Scholar] [CrossRef] [Green Version]
- Si, T.; Li, F.; Yin, X.Y.; Yin, X.Z. Spatial instability of coflowing liquid-gas jets in capillary flow focusing. Phys. Fluids 2010, 22, 112105. [Google Scholar] [CrossRef] [Green Version]
- Gañán-Calvo, A.M.; Herrada, M.A.; Montanero, J.M. How does a shear boundary layer affect the stability of a capillary jet? Phys. Fluids 2014, 26, 061701. [Google Scholar] [CrossRef]
- Zahoor, R.; Bajt, S.; Sarler, B. Influence of Gas Dynamic Virtual Nozzle Geometry on Micro-Jet Characteristics. Int. J. Multiph. Flow 2018, 104, 152–165. [Google Scholar] [CrossRef]
- McCarthy, M.J.; Molloy, N.A. Review of Stability of Liquid Jets and the Influence of Nozzle Design. Chem. Eng. J. 1974, 7, 1–20. [Google Scholar] [CrossRef]
- Sterling, A.; Sleicher, C.A. The instability of capillary jets. J. Fluid Mech. 1975, 68, 477–495. [Google Scholar] [CrossRef]
- Smith, S.W.J.; Moss, H. Experiments with mercury jets. Proc. R. Soc. Lond. A Math. Phys. Eng. 1917, 93, 373–393. [Google Scholar]
- Ismail, A.S.; Yao, J.; Xia, H.H.; Stark, J.P.W. Breakup Length of Electrified Liquid Jets: Scaling Laws and Applications. Phys. Rev. Appl. 2018, 10, 064010. [Google Scholar] [CrossRef]
- Umemura, A. Self-destabilising loop of a low-speed water jet emanating from an orifice in microgravity. J. Fluid Mech. 2016, 25, 146–180. [Google Scholar] [CrossRef]
- Rosell-Llompart, J.; Gañán-Calvo, A.M. Turbulence in pneumatic flow focusing and flow blurring regimes. Phys. Rev. E 2008, 77, 036321. [Google Scholar] [CrossRef]
- Popinet, S. Basilisk Flow Solver and PDE Library. Available online: http://basilisk.fr/ (accessed on 23 July 2018).
- Herrada, M.A.; Montanero, J.M. A numerical method to study the dynamics of capillary fluid systems. J. Comput. Phys. 2016, 306, 137–147. [Google Scholar] [CrossRef]
- Huerre, P.; Monkewitz, P.A. Local and Global Instabilites in Spatially Developing Flows. Annu. Rev. Fluid Mech. 1990, 22, 473–537. [Google Scholar] [CrossRef]
- Moseler, M.; Landman, U. Formation, Stability, and Breakup of Nanojets. Science 2000, 289, 1165–1169. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Beyerlein, K.R.; Adriano, L.; Heymann, M.; Kirian, R.; Knoska, J.; Wilde, F.; Chapman, H.N.; Bajt, S. Ceramic micro-injection molded nozzles for serial femtosecond crystallography sample delivery. Rev. Sci. Instrum. 2015, 86, 125104. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Leib, S.J.; Goldstein, M.E. Convective and absolute instability of a viscous liquid jet. Phys. Fluids 1986, 29, 952–954. [Google Scholar] [CrossRef]
- Gañán-Calvo, A.M.; Montanero, J.M. Revision of capillary cone-jet physics: Electrospray and flow focusing. Phys. Rev. E 2009, 79, 066305. [Google Scholar] [CrossRef]
- Wiedorn, M.O.; Oberthür, D.; Bean, R.; Schubert, R.; Werner, N.; Abbey, B.; Aepfelbacher, M.; Adriano, L.; Allahgholi, A.; Al-Qudami, N.; et al. Megahertz serial crystallography. Nat. Commun. 2018, 9, 4025. [Google Scholar] [CrossRef] [Green Version]








| Liquid | (kg·m) | (N·m) | (Pa·s) |
|---|---|---|---|
| water (22 C) | 1000 | 0.072 | 0.001 |
| water/ethanol (65/35 v/v %) (20 C) | 943 | 0.035 | 0.0026 |
| ethanol (22 C) | 795 | 0.023 | 0.00125 |
| water/glycerol (20/80 v/v %) (22 C) | 1217 | 0.065 | 0.0914 |
| Ejector | Orifice Shape | Dimensions (m) | (m) |
|---|---|---|---|
| 1 | slit | 15×45 | 30 |
| 2 | slit | 20×60 | 30 |
| 3 | round | 30 | 30 |
| 4 | round | 50 | 50 |
| 5 | round | 75 | 75 |
| 6 | round | 70 | 100 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gañán-Calvo, A.M.; Chapman, H.N.; Heymann, M.; Wiedorn, M.O.; Knoska, J.; Gañán-Riesco, B.; López-Herrera, J.M.; Cruz-Mazo, F.; Herrada, M.A.; Montanero, J.M.; et al. The Natural Breakup Length of a Steady Capillary Jet: Application to Serial Femtosecond Crystallography. Crystals 2021, 11, 990. https://doi.org/10.3390/cryst11080990
Gañán-Calvo AM, Chapman HN, Heymann M, Wiedorn MO, Knoska J, Gañán-Riesco B, López-Herrera JM, Cruz-Mazo F, Herrada MA, Montanero JM, et al. The Natural Breakup Length of a Steady Capillary Jet: Application to Serial Femtosecond Crystallography. Crystals. 2021; 11(8):990. https://doi.org/10.3390/cryst11080990
Chicago/Turabian StyleGañán-Calvo, Alfonso M., Henry N. Chapman, Michael Heymann, Max O. Wiedorn, Juraj Knoska, Braulio Gañán-Riesco, José M. López-Herrera, Francisco Cruz-Mazo, Miguel A. Herrada, José M. Montanero, and et al. 2021. "The Natural Breakup Length of a Steady Capillary Jet: Application to Serial Femtosecond Crystallography" Crystals 11, no. 8: 990. https://doi.org/10.3390/cryst11080990
APA StyleGañán-Calvo, A. M., Chapman, H. N., Heymann, M., Wiedorn, M. O., Knoska, J., Gañán-Riesco, B., López-Herrera, J. M., Cruz-Mazo, F., Herrada, M. A., Montanero, J. M., & Bajt, S. (2021). The Natural Breakup Length of a Steady Capillary Jet: Application to Serial Femtosecond Crystallography. Crystals, 11(8), 990. https://doi.org/10.3390/cryst11080990