Microstructural Origin of the Double Yield Points of the Metallocene Linear Low-Density Polyethylene (mLLDPE) Precursor Film under Uniaxial Tensile Deformation
Abstract
:1. Introduction
2. Experiments
2.1. Materials and Sample Preparation
2.2. Characterization
2.2.1. In Situ SAXS/WAXS
2.2.2. Scanning Electron Microscope (SEM) Measurements
3. Results
3.1. Crystal Morphologies
3.2. Mechanical Property
3.3. 2D SAXS/WAXS Patterns
3.4. Strain Dependent Long Period and Crystallinity
3.5. Influence of Different Orientations of mLLDPE Precursor Film
3.6. Micro-Strain and Lateral Size Evolution
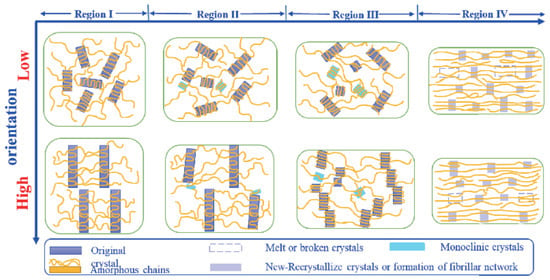
4. Discussion
5. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| mLLDPE | Metallocene linear low-density polyethylene |
| SAXS | Small-angle X-ray scattering |
| WAXS | Wide-angle X-ray scattering |
References
- Hyvärinen, M.; Jabeen, R.; Kärki, T. The Modelling of Extrusion Processes for Polymers—A Review. Polymers 2020, 12, 1306. [Google Scholar] [CrossRef] [PubMed]
- Sacco, F.D.; Gahleitner, M.; Wang, J.; Portale, G. Systematic Investigation on the Structure-Property Relationship in Isotactic Polypropylene Films Processed via Cast Film Extrusion. Polymers 2020, 12, 1636. [Google Scholar] [CrossRef] [PubMed]
- Peterlin, A. Drawing and Annealing of Fibrous Material. J. Appl. Phys. 1977, 48, 4099–4108. [Google Scholar] [CrossRef]
- Liu, H.L.; Lv, F.; Cao, T.; Wan, C.; Zhang, W.; Li, L.; Zheng, G.; Shen, C. Two-stage Drawing Process to Prepare High-Strength and Porous Ultrahigh-Molecular-Weight Polyethylene Fibers: Cold Drawing and Hot Drawing. J. Appl. Polym. Sci. 2015, 132, 42823. [Google Scholar] [CrossRef]
- Lv, F.; Chen, X.; Wan, C.; Su, F.; Ji, Y.; Lin, Y.; Li, X.; Li, L. Deformation of Ultrahigh Molecular Weight Polyethylene Precursor Fiber: Crystal Slip with or without Melting. Macromolecules 2017, 50, 6385–6395. [Google Scholar] [CrossRef]
- DeMeuse, M.T. Biaxial Stretching of Film: Principles and Applications; Elsevier: Amsterdam, The Netherlands, 2011. [Google Scholar]
- Lin, Y.; Chen, W.; Meng, L.; Wang, D.; Li, L. Recent Advances in Post-Stretching Processing of Polymer Films with in situ Synchrotron Radiation X-ray Scattering. Soft Matter 2020, 16, 3599–3612. [Google Scholar] [CrossRef]
- Arora, P.; Zhang, Z. Battery Separators. Chem. Rev. 2004, 104, 4419–4462. [Google Scholar] [CrossRef]
- Lin, Y.; Meng, L.; Wu, L.; Li, X.; Chen, X.; Zhang, Q.; Zhang, R.; Zhang, W.; Li, L. A Semi-Quantitative Deformation Model for Pore Formation in Isotactic Polypropylene Microporous Membrane. Polymer 2015, 80, 214–227. [Google Scholar] [CrossRef]
- Lin, Y.; Li, X.; Chen, X.; An, M.; Zhang, Q.; Wang, D.; Chen, W.; Sun, L.; Yin, P.; Meng, L.; et al. Deformation Mechanism of Hard Elastic Polyethylene Film During Uniaxial Stretching: Effect of Stretching Speed. Polymer 2019, 178, 121579. [Google Scholar] [CrossRef]
- Lin, Y.; Li, X.; Chen, X.; An, M.; Zhang, Q.; Wang, D.; Chen, W.; Sun, L.; Yin, P.; Meng, L.; et al. Structural Evolution of Hard-Elastic Polyethylene Cast Film in Temperature-Strain Space: An in situ SAXS and WAXS Study. Polymer 2019, 184, 121930. [Google Scholar] [CrossRef]
- Lin, Y.; Li, X.; Meng, L.; Chen, X.; Lv, F.; Zhang, Q.; Li, L. Stress-Induced Microphase Separation of Interlamellar Amorphous Phase in Hard-Elastic Isotactic Polypropylene Film. Polymer 2018, 148, 79–92. [Google Scholar] [CrossRef]
- Lin, Y.; Li, X.; Meng, L.; Chen, X.; Lv, F.; Zhang, Q.; Zhang, R.; Li, L. Structural Evolution of Hard-Elastic Isotactic Polypropylene Film during Uniaxial Tensile Deformation: The Effect of Temperature. Macromolecules 2018, 51, 2690–2705. [Google Scholar] [CrossRef]
- Li, L. In Situ Synchrotron Radiation Techniques: Watching Deformation Induced Structural Evolutions of Polymers. Chin. J. Polym. Sci. 2018, 36, 1093–1102. [Google Scholar] [CrossRef]
- Popli, R.; Mandelkern, L. Influence of Structural and Morphological Factors on the Mechanical Properties of the Polyethylenes. J. Polym. Sci. Part B 1987, 25, 441–483. [Google Scholar] [CrossRef]
- Seguela, R.; Rietsch, F. Double Yield Point in Polyethylene under Tensile Loading. J. Mater. Sci. Lett. 1990, 9, 46–47. [Google Scholar] [CrossRef]
- Séguéla, R.; Darras, O. Phenomenological Aspects of the Double Yield of Polyethylene and Related Copolymers under Tensile Loading. J. Mater. Sci. 1994, 29, 5342–5352. [Google Scholar] [CrossRef]
- Brooks, N.W.; Duckett, R.A.; Ward, I.M. Investigation into Double Yield Points in Polyethylene. Polymer 1992, 33, 1872–1880. [Google Scholar] [CrossRef]
- Brooks, N.W.; Duckett, R.A.; Ward, I.M. Modeling of Double Yield Points in Polyethylene: Temperature and Strain-Rate Dependence. J. Rheol. 1995, 39, 425. [Google Scholar] [CrossRef]
- Brooks, N.W.; Unwin, A.P.; Duckett, R.A.; Ward, I.M. Temperature and strain rate dependence of yield strain and deformation behavior in polyethylene. J. Polym. Sci. Part B 1997, 35, 545–552. [Google Scholar] [CrossRef]
- Lucas, J.C.; Failla, M.D.; Smith, F.L.; Mandelkern, L.; Peacock, A.J. The Double Yield in the Tensile Deformation of the Polyethylenes. Polym. Eng. Sci. 1995, 35, 1117–1123. [Google Scholar] [CrossRef]
- Plaza, A.R.; Ramos, E.; Manzur, A.; Olayo, R.; Escobar, A. Double yield points in triblends of LDPE, LLDPE and EPDM. J. Mater. Sci. 1997, 32, 549–554. [Google Scholar] [CrossRef]
- Balsamo, V.; Müller, A.J. The Phenomenon of Double Yielding under Tension in Low-Density Polyethylene, Linear Low-Density Polyethylene and Their Blends. J. Mater. Sci. Lett. 1993, 12, 1457–1459. [Google Scholar] [CrossRef]
- Feijoo, J.L.; Sánchez, J.J.; Müller, A.J. The Phenomenon of Double Yielding in Oriented High Density Polyethylene Films. J. Mater. Sci. Lett. 1997, 16, 1721–1724. [Google Scholar] [CrossRef]
- Gaucher-Miri, V.; Séguéla, R. Tensile Yield of Polyethylene and Related Copolymers: Mechanical and Structural Evidences of Two Thermally Activated Processes. Macromolecules 1997, 30, 1158–1167. [Google Scholar] [CrossRef]
- Razavi-Nouri, M.; Hay, L.N. Effect of Orientation on Mechanical Properties of Metallocene Polyethylenes. Iran. Polym. J. 2004, 13, 521–530. [Google Scholar]
- Manzur, A.; Rivas, J.I. Crystallinity Variations in the Double Yield Region of Polyethylene. J. Appl. Polym. Sci. 2007, 104, 3103–3111. [Google Scholar] [CrossRef]
- Manzur, A. Strain Rate Effect on Crystallinity Variations in the Double Yield Region of Polyethylene. J. Appl. Polym. Sci. 2008, 108, 1574–1581. [Google Scholar] [CrossRef]
- Romo-Uribe, A.; Manzur, A.; Olayo, R. Synchrotron Small-angle X-ray Scattering Study of Linear Low-Density Polyethylene under Uniaxial Deformation. J. Appl. Polym. Sci. 2012, 27, 1351–1359. [Google Scholar] [CrossRef]
- Galeski, A.; Bartczak, Z.; Vozniak, A.; Pawlak, A.; Walkenhorst, R. Morphology and Plastic Yielding of Ultrahigh Molecular Weight Polyethylene. Macromolecules 2020, 53, 6063–6077. [Google Scholar] [CrossRef]
- Razavi, M.; Wang, S.Q. Double Yielding in Deformation of Semicrystalline Polymers. Macromol. Chem. Phys. 2020, 221, 2000151. [Google Scholar] [CrossRef]
- Shan, G.-F.; Yang, W.; Xie, B.-H.; Li, Z.-M.; Chen, J.; Yang, M.-B. Double Yielding Behaviors of Polyamide 6 and Glass Bead Filled Polyamide 6 Composites. Polym. Test. 2005, 24, 704–711. [Google Scholar] [CrossRef]
- Shan, G.-F.; Yang, W.; Yang, M.-B.; Xie, B.-H.; Li, Z.-M.; Feng, J.-M. Effect of Crystallinity Level on the Double Yielding Behavior of Polyamide 6. Polym. Test. 2006, 25, 452–459. [Google Scholar] [CrossRef]
- Li, Z.-M.; Yang, W.; Yang, S.; Huang, R.; Yang, M.-B. Morphology-Tensile Behavior Relationship in Injection Molded Poly(ethylene terephthalate)/Polyethylene and Polycarbonate/Polyethylene Blends (I) Part I Skin-Core Structure. J. Mater. Sci. 2004, 39, 413–431. [Google Scholar] [CrossRef]
- Li, Z.-M.; Huang, C.-G.; Yang, W.; Yang, M.-B.; Huang, R. Morphology Dependent Double Yielding in Injection Molded Polycarbonate/Polyethylene Blend. Macromol. Mater. Eng. 2004, 289, 1004–1011. [Google Scholar] [CrossRef]
- Pan, J.L.; Zhong, G.; Ning, N.-Y.; Yang, S. Double Yielding in Injection-Molded Polycarbonate/Polyethylene Blends: Composition Dependence. Macromol. Mater. Eng. 2006, 291, 477–484. [Google Scholar] [CrossRef]
- Scogna, R.C.; Register, R.A. Plastic deformation of ethylene/methacrylic acid copolymers and ionomers. J. Polym. Sci. Part B 2009, 47, 1588–1598. [Google Scholar] [CrossRef]
- Wu, T.; Xiang, M.; Cao, Y.; Kang, J.; Yang, F. Influence of Lamellar Structure on Double Yield Behavior and Pore Size Distribution in β Nucleated Polypropylene Stretched Membranes. RSC Adv. 2014, 4, 43012–43023. [Google Scholar] [CrossRef]
- Wu, T.; Cao, Y.; Yang, F.; Xiang, M. Investigation on Double Yielding Behavior under Tensile Loading in Isotactic Polypropylene. Mater. Design 2014, 60, 153–163. [Google Scholar] [CrossRef]
- Hammersley, A.P. FIT2D: A Multi-Purpose Data Reduction, Analysis and Visualization Program. J. Appl. Cryst. 2016, 49, 646–652. [Google Scholar] [CrossRef]
- Olley, R.H.; Bassett, D.C. An Improved Permanganic Etchant for Polyolefine. Polymer 1982, 23, 1707–1710. [Google Scholar] [CrossRef]
- Jiang, Z.; Tang, Y.; Rieger, J.; Enderle, H.-F.; Lilge, D.; Roth, S.V.; Gehrke, R.; Heckmann, W.; Men, Y. Two Lamellar to Fibrillar Transitions in the Tensile Deformation of High-Density Polyethylene. Macromolecules 2010, 43, 4727–4732. [Google Scholar] [CrossRef]
- Tang, Y.; Jiang, Z.; Men, Y.; An, L.; Enderle, H.-F.; Lilge, D.; Roth, S.V.; Gehrke, R.; Rieger, J. Uniaxial Deformation of Overstretched Polyethylene: In situ Synchrotron Small Angle X-ray Scattering Study. Polymer 2007, 48, 5125–5132. [Google Scholar] [CrossRef]
- Xiong, B.; Lame, O.; Chenal, J.-M.; Rochas, C.; Seguela, R.; Vigier, G. Amorphous Phase Modulus and Micro-Macro Scale Relationship in Polyethylene via in situ SAXS and WAXS. Macromolecules 2015, 48, 2149–2160. [Google Scholar] [CrossRef]
- Bowden, P.B.; Young, R.J. Deformation Mechanisms in Crystalline Polymers. J. Mater. Sci. 1974, 9, 2034–2051. [Google Scholar] [CrossRef]
- Butler, M.F.; Donald, A.M.; Bras, W.; Mant, G.R.; Derbyshire, G.E.; Ryan, A. A Real-Time Simultaneous Small-and Wide-Angle X-ray Scattering Study of in situ Polyethylene Deformation at Elevated Temperatures. Macromolecules 1998, 31, 6234–6249. [Google Scholar] [CrossRef]
- Chen, X.; Lv, F.; Su, F.; Ji, Y.; Meng, L.; Wan, C.; Lin, Y.; Li, X.; Li, L. Deformation Mechanism of iPP under Uniaxial Stretching Over a Wide Temperature Range: An in situ Synchrotron Radiation SAXS/WAXS study. Polymer 2017, 118, 12–21. [Google Scholar] [CrossRef]
- Xiong, B.; Lame, O.; Chenal, J.-M.; Rochas, C.; Seguela, R.; Vigier, G. Temperature-Microstructure Mapping of the Initiation of the Plastic Deformation Processes in Polyethylene via In Situ WAXS and SAXS. Macromolecules 2015, 48, 5267–5275. [Google Scholar] [CrossRef]
- Chen, X.; Lv, F.; Lin, Y.; Wang, Z.; Meng, L.; Zhang, Q.; Zhang, W.; Li, L. Structure Evolution of Polyethylene-Plasticizer Film at Industrially Relevant Conditions Studied by in situ X-ray Scattering: The Role of Crystal Stress. Eur. Polym. J. 2018, 101, 358–367. [Google Scholar] [CrossRef]
- Men, Y.; Rieger, J.; Strobl, G. Role of the Entangled Amorphous Network in Tensile Deformation of Semicrystalline Polymers. Phys. Rev. Lett. 2003, 91, 095502. [Google Scholar] [CrossRef]







| Draw Ratio | (%) | (nm) | (nm) | Sample Name | |
|---|---|---|---|---|---|
| 20 | 0.61 | 41.5 | 20.56 | 20.76 | PE-61 |
| 80 | 0.72 | 49.9 | 17.94 | 19.82 | PE-72 |
| 120 | 0.78 | 49.1 | 16.63 | 19.59 | PE-78 |
| 240 | 0.87 | 44.9 | 17.32 | 18.00 | PE-87 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Iqbal, O.; Habumugisha, J.C.; Feng, S.; Lin, Y.; Chen, W.; Yu, W.; Li, L. Microstructural Origin of the Double Yield Points of the Metallocene Linear Low-Density Polyethylene (mLLDPE) Precursor Film under Uniaxial Tensile Deformation. Polymers 2021, 13, 126. https://doi.org/10.3390/polym13010126
Iqbal O, Habumugisha JC, Feng S, Lin Y, Chen W, Yu W, Li L. Microstructural Origin of the Double Yield Points of the Metallocene Linear Low-Density Polyethylene (mLLDPE) Precursor Film under Uniaxial Tensile Deformation. Polymers. 2021; 13(1):126. https://doi.org/10.3390/polym13010126
Chicago/Turabian StyleIqbal, Obaid, Jean Claude Habumugisha, Shengyao Feng, Yuanfei Lin, Wei Chen, Wancheng Yu, and Liangbin Li. 2021. "Microstructural Origin of the Double Yield Points of the Metallocene Linear Low-Density Polyethylene (mLLDPE) Precursor Film under Uniaxial Tensile Deformation" Polymers 13, no. 1: 126. https://doi.org/10.3390/polym13010126
APA StyleIqbal, O., Habumugisha, J. C., Feng, S., Lin, Y., Chen, W., Yu, W., & Li, L. (2021). Microstructural Origin of the Double Yield Points of the Metallocene Linear Low-Density Polyethylene (mLLDPE) Precursor Film under Uniaxial Tensile Deformation. Polymers, 13(1), 126. https://doi.org/10.3390/polym13010126