Mechanical Performance of Knitted Hollow Composites from Recycled Cotton and Glass Fibers for Packaging Applications
Abstract
:1. Introduction
2. Materials and Methods
2.1. Materials
2.2. Methods
2.2.1. Production of Composite Samples
2.2.2. Characterization
3. Results and Discussion
3.1. Surface Hardness
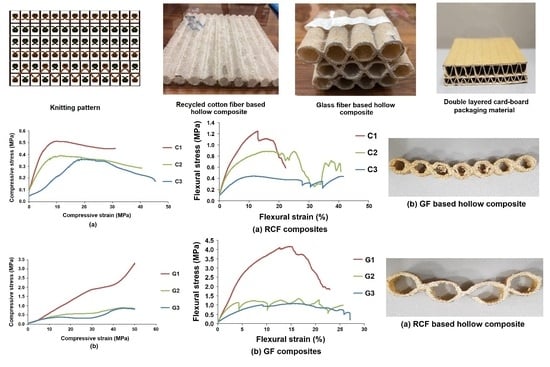
3.2. Compression Properties
3.3. Flexural Properties
3.4. Impact Properties
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Ramakrishna, H.; Priya, S.; Rai, S. Effect of fly ash content on impact, compression, and water absorption properties of epoxy toughened with epoxy phenol cashew nutshell liquid-fly ash composites. J. Reinf. Plast. Compos. 2006, 25, 455–462. [Google Scholar] [CrossRef]
- Jamshaid, H.; Mishra, R.; Pechociakova, M.; Noman, M.T. Mechanical, thermal and interfacial properties of green composites from basalt and hybrid woven fabrics. Fibers Polym. 2016, 17, 1675–1686. [Google Scholar] [CrossRef]
- Sargianis, J.; Kim, H.; Andres, E.; Suhr, J. Sound and vibration damping characteristics in natural material based sandwich composites. Compos. Struct. 2013, 96, 538–544. [Google Scholar] [CrossRef]
- Mohanty, A.; Misra, M.; Drzal, L. Natural Fibers, Biopolymers and Biocomposites; CRC Press: Boca Raton, FL, USA, 2005. [Google Scholar]
- Joserph, P.; Joseph, K.; Thomas, S. Effect of processing variables on the mechanical properties of sisal-fiber-reinforced polypropylene composites. Compos. Sci. Technol. 1999, 59, 1625–1640. [Google Scholar] [CrossRef]
- Sakthivel, S.; Kumar, S.; Melese, B.; Mekonnen, S.; Solomon, E.; Edae, A.; Abedom, F.; Gedilu, M. Development of nonwoven composites from recycled cotton/polyester apparel waste materials for sound absorbing and insulating properties. Appl. Acoust. 2021, 180, 108126. [Google Scholar] [CrossRef]
- Mishra, R.; Behera, B.K. Recycling of textile waste into green composites: Performance characterization. Polym. Compos. 2014, 35, 1960–1967. [Google Scholar] [CrossRef]
- Sezgin, H.; Kucukali-Ozturk, M.; Berkalp, O.B.; Yalcin-Enis, I. Design of composite insulation panels containing 100% recycled cotton fibers and polyethylene/polypropylene packaging wastes. J. Clean. Prod. 2021, 304, 127132. [Google Scholar] [CrossRef]
- Mishra, R.; Huang, J.; Kale, B.; Zhu, G.; Wang, Y. The production, characterization and applications of nanoparticles in the textile industry. Text. Prog. 2014, 46, 133–226. [Google Scholar] [CrossRef]
- Li, H.; Li, Z.; Liu, L. Flax/PP weft-knitted thermoplastic composites and its tensile properties. J. Reinf. Plast. Compos. 2010, 29, 1820–1825. [Google Scholar] [CrossRef]
- Hoffmann, G.; Diestel, O.; Cherif, O. Thermoplastic composite from innovative flat knitted 3D multi-layer spacer fabric using hybrid yarn and the study of 2D mechanical properties. Compos. Sci. Technol. 2010, 70, 363–370. [Google Scholar] [CrossRef]
- Harte, A.; Fleck, N. Deformation and failure mechanisms of braided composite tubes in compression and torsion. Acta Mater. 2000, 48, 1259–1271. [Google Scholar] [CrossRef]
- Mishra, R.; Gupta, N.; Pachauri, R.; Behera, B.K. Modelling and simulation of earthquake resistant 3D woven textile structural concrete composites. Compos. Part B Eng. 2015, 81, 91–97. [Google Scholar] [CrossRef]
- Beard, S.; Chang, F. Energy absorption of braided composite tubes. Int. J. Crashworthiness 2002, 7, 191–206. [Google Scholar] [CrossRef]
- Ziegmann, G.; Dickert, M.; Cristaldi, G. Properties and performances of various hybrid glass/natural fiber composites for curved pipes. Mater. Des. 2009, 30, 2538–2542. [Google Scholar] [CrossRef]
- Wu, X.; Zhang, Q.; Zhang, W. Axial compression deformation and damage of four-step 3-D circular braided composite tubes under various strain rates. J. Text. Inst. 2016, 107, 1584–1600. [Google Scholar] [CrossRef]
- Hu, D.; Luo, M.; Yang, J. Experimental study on crushing characteristics of brittle fiber/epoxy hybrid composite tubes. Int. J. Crashworthiness 2010, 15, 401–412. [Google Scholar] [CrossRef]
- Ahmed, M.; Hoa, S. Flexural stiffness of thick-walled composite tubes. Compos. Struct. 2016, 149, 125–133. [Google Scholar] [CrossRef]
- Yan, A.; Jospin, R.; Nguyen, D. An enhanced pipe elbow element application in plastic limit analysis of pipe structures. Int. J. Numer. Meth. Eng. 1999, 46, 409–431. [Google Scholar] [CrossRef]
- Bathe, K.; Almeida, C. A simple and effective pipe elbow element-linear analysis. J. Appl. Mech. 1980, 47, 93–100. [Google Scholar] [CrossRef]
- Qi, X.; Jiang, S. Design and analysis of a filament wound composite tube under general loadings with assistance of computer. In Proceedings of the 2nd International Conference on Education Technology and Computer (ICETC), Shanghai, China, 22–24 June 2010. [Google Scholar]
- Xu, D.; Derisi, B.; Hoa, S. Stress distributions of thermoplastic composite tubes subjected to four-point loading. In Proceedings of the 1st Joint Canadian-American International Conference, Delaware, DE, USA, 15–17 September 2009. [Google Scholar]
- Available online: https://www.shimaseiki.com/product/design/virtual_sampling/ (accessed on 19 July 2021).
- Almeida, J., Jr.; Ribeiro, M.L.; Tita, V.; Amico, S.C. Damage modeling for carbon fiber/epoxy filament wound composite tubes under radial compression. Compos. Struct. 2017, 160, 204–210. [Google Scholar] [CrossRef]
- Mishra, R.; Behera, B.K.; Mukherjee, S.; Petru, M.; Muller, M. Axial and radial compression behavior of composite rocket launcher developed by robotized filament winding: Simulation and experimental validation. Polymers 2021, 13, 517. [Google Scholar] [CrossRef]
- Hassan, T.; Jamshaid, H.; Mishra, R.; Khan, M.Q.; Petru, M.; Novak, J.; Choteborsky, R.; Hromasova, M. Acoustic, mechanical and thermal properties of green composites reinforced with natural fibers waste. Polymers 2020, 12, 654. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Sarr, M.M.; Inoue, H.; Kosaka, T. Study on the improvement of interfacial strength between glass fiber and matrix resin by grafting cellulose nanofibers. Compos. Sci. Technol. 2021, 211, 108853. [Google Scholar] [CrossRef]
- Halpin, A.J.C.; Kardos, J.L. The halpin-tsai equations: A review. Polym. Eng. Sci. 1976, 16, 344–352. [Google Scholar]
- Liu, Q.; Xu, X.; Ma, J. Lateral crushing and bending responses of CFRP square tube filled with aluminum honeycomb. Compos. Part B Eng. 2017, 118, 104–115. [Google Scholar] [CrossRef]
- Hamidon, M.; Sultan, M.; Ariffin, A. Investigation of Mechanical Testing on Hybrid Composite Materials. In Failure Analysis in Biocomposites, Fiber-Reinforced Composites and Hybrid Composites; Elsevier: Amsterdam, The Netherlands, 2019; pp. 133–156. [Google Scholar]
- Lu, Z.; Jing, X.; Sun, B.; Gu, B. Compressive behaviors of warp-knitted spacer fabrics impregnated with shear thickening fluid. Compos. Sci. Technol. 2013, 88, 184–189. [Google Scholar] [CrossRef]
- Asayesh, A.; Amini, M. The effect of fabric structure on the compression behavior of weft-knitted spacer fabrics for cushioning applications. J. Text. Inst. 2020, 1–12, Online First. [Google Scholar] [CrossRef]
- Liu, Y.; Hu, H.; Zhao, L.; Long, H. Compression behavior of warp-knitted spacer fabrics for cushioning applications. Text. Res. J. 2012, 82, 11–20. [Google Scholar] [CrossRef]
- Arumugam, V.; Mishra, R.; Tunak, M. In-plane shear behavior of 3D knitted spacer fabrics. J. Ind. Text. 2016, 46, 868–886. [Google Scholar] [CrossRef]
- Arumugam, V.; Mishra, R.; Tunak, M. In plane shear behavior of 3D warp-knitted spacer fabrics: Part-II: Effect of structural parameters. J. Ind. Text. 2018, 48, 772–801. [Google Scholar] [CrossRef]
- Arumugam, V.; Mishra, R.; Salacova, J. Investigation on thermo-physiological and compression characteristics of weft knitted 3D spacer fabrics. J. Text. Inst. 2017, 108, 1095–1105. [Google Scholar] [CrossRef]
- Ahmed, M.M.; Dhakal, H.N.; Zhang, Z.Y.; Barouni, A.; Zahari, R. Enhancement of impact toughness and damage behaviour of natural fibre reinforced composites and their hybrids through novel improvement techniques: A critical review. Compos. Struct. 2021, 259, 113496. [Google Scholar] [CrossRef]
- Li, F.S.; Gao, Y.B.; Jiang, W. Design of high impact thermal plastic polymer composites with balanced toughness and rigidity: Toughening with one phase modifier. Polymer 2019, 170, 101–106. [Google Scholar] [CrossRef]
- Liu, W.; Zhang, J.Q.; Hong, M.; Li, P.; Xue, Y.H.; Chen, Q.; Ji, X.L. Chain microstructure of two highly impact polypropylene resins with good balance between stiffness and toughness. Polymer 2020, 188, 122146. [Google Scholar] [CrossRef]
- Han, S.; Zhang, T.; Guo, Y.; Li, C.; Wu, H.; Guo, S. Brittle-ductile transition behavior of the polypropylene/ultra-high molecular weight polyethylene/olefin block copolymers ternary blends: Dispersion and interface design. Polymer 2019, 182, 121819. [Google Scholar] [CrossRef]
- Hajjari, M.; Nedoushan, R.J.; Dastan, T.; Sheikhzadeh, M.; Yu, W.R. Lightweight weft-knitted tubular lattice composite for energy absorption applications: An experimental and numerical study. Int. J. Solid Struct. 2021, 213, 77–92. [Google Scholar] [CrossRef]
- Khondker, O.A.; Leong, K.H.; Herszberg, I.; Hamada, H. Impact and compression-after-impact performance of weft-knitted glass textile composites. Compos. Part A Appl. Sci. Manuf. 2005, 36, 638–648. [Google Scholar] [CrossRef]













| Sample ID | Yarn Type | Linear Density of Yarn (Denier) | Plain Courses | Tube Courses | Wales (cm−1) | Courses (cm−1) | Stitch Length (cm) |
|---|---|---|---|---|---|---|---|
| C1 | Recycled Cotton (RC) | 1800 | 2 | 8 | 11 | 18 | 0.71 |
| C2 | Recycled Cotton (RC) | 1800 | 2 | 12 | 11 | 18 | 0.71 |
| C3 | Recycled Cotton (RC) | 1800 | 2 | 16 | 11 | 18 | 0.71 |
| G1 | Glass (G) | 1800 | 2 | 8 | 12 | 16 | 0.69 |
| G2 | Glass (G) | 1800 | 2 | 12 | 12 | 16 | 0.69 |
| G3 | Glass (G) | 1800 | 2 | 16 | 12 | 16 | 0.70 |
| Sample ID | Fiber Volume Fraction (Vf %) | Density (g/cm3) | Hardness (Barcol) Scale of (0–100) | Diameter of Tube | |
|---|---|---|---|---|---|
| Inner Dia (mm) | Outer Dia (mm) | ||||
| C1 | 40 ± 2 | 1.23 ± 0.02 | 82.7 ± 0.2 | 8.1 ± 0.1 | 11.8 ± 0.1 |
| C2 | 40 ± 2 | 1.21 ± 0.02 | 81.9 ± 0.2 | 10.2 ± 0.1 | 14.8 ± 0.1 |
| C3 | 40 ± 2 | 1.21 ± 0.02 | 80.1 ± 0.2 | 14.3 ± 0.1 | 18.5 ± 0.1 |
| G1 | 50 ± 2 | 1.41 ± 0.02 | 88.0 ± 0.2 | 8.2 ± 0.1 | 10.5 ± 0.1 |
| G2 | 50 ± 2 | 1.42 ± 0.02 | 87.7 ± 0.2 | 10.2 ± 0.1 | 12.5 ± 0.1 |
| G3 | 50 ± 2 | 1.39 ± 0.02 | 86.6 ± 0.2 | 14.2 ± 0.1 | 16.5 ± 0.1 |
| W | - | - | 11.5 ± 0.2 | - | 15.0 ± 0.1 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Jamshaid, H.; Mishra, R.; Zeeshan, M.; Zahid, B.; Basra, S.A.; Tichy, M.; Muller, M. Mechanical Performance of Knitted Hollow Composites from Recycled Cotton and Glass Fibers for Packaging Applications. Polymers 2021, 13, 2381. https://doi.org/10.3390/polym13142381
Jamshaid H, Mishra R, Zeeshan M, Zahid B, Basra SA, Tichy M, Muller M. Mechanical Performance of Knitted Hollow Composites from Recycled Cotton and Glass Fibers for Packaging Applications. Polymers. 2021; 13(14):2381. https://doi.org/10.3390/polym13142381
Chicago/Turabian StyleJamshaid, Hafsa, Rajesh Mishra, Muhammad Zeeshan, Bilal Zahid, Sikandar Abbas Basra, Martin Tichy, and Miroslav Muller. 2021. "Mechanical Performance of Knitted Hollow Composites from Recycled Cotton and Glass Fibers for Packaging Applications" Polymers 13, no. 14: 2381. https://doi.org/10.3390/polym13142381
APA StyleJamshaid, H., Mishra, R., Zeeshan, M., Zahid, B., Basra, S. A., Tichy, M., & Muller, M. (2021). Mechanical Performance of Knitted Hollow Composites from Recycled Cotton and Glass Fibers for Packaging Applications. Polymers, 13(14), 2381. https://doi.org/10.3390/polym13142381