Linear-Nonlinear Stiffness Responses of Carbon Fiber-Reinforced Polymer Composite Materials and Structures: A Numerical Study
Abstract
:1. Introduction
2. Damage Model of FRP Composite Material
2.1. Damage Initiation
2.2. Post-Damage Initiation
2.3. Damage Propagation
3. Mechanical Properties and Damage Model Parameters
4. Finite Element Model and Simulation Process
4.1. Finite Element Simulation
4.2. Validation of the Finite Element Model
5. Results and Discussion
5.1. Stiffness Response with Respect to the Variation of Elastic Properties
5.2. Stiffness Response with Respect to Variation of Damage Parameters
5.2.1. CFRP Composite Beam under Tension
5.2.2. Thin Composite Plate under Three-Point Bending
5.2.3. Thick Composite Plate under Three-Point Bending
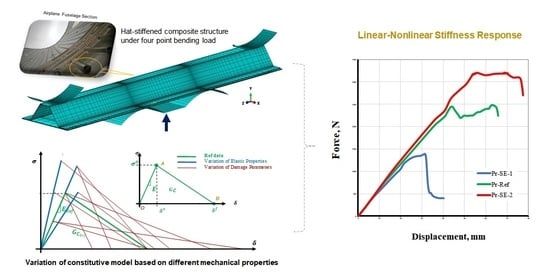
5.2.4. Composite Profile under Flexural Loading
6. Concluding Remarks
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Koloor, S.S.R.; Khosravani, M.R.; Hamzah, R.; Tamin, M. FE model-based construction and progressive damage processes of FRP composite laminates with different manufacturing processes. Int. J. Mech. Sci. 2018, 141, 223–235. [Google Scholar] [CrossRef]
- Rangappa, S.M.; Parameswaranpillai, J.; Siengchin, S.; Kroll, L. Lightweight Polymer Composite Structure: Design and Manufacturing Techniques; CRC Press: Boca Raton, FL, USA, 2020. [Google Scholar]
- Kaddour, A.; Hinton, M.; Smith, P.; Li, S. The background to the third world-wide failure exercise. J. Compos. Mater. 2013, 47, 2417–2426. [Google Scholar] [CrossRef]
- Hinton, M.J.; Kaddour, A.S.; Soden, P.D. Failure Criteria in Fibre Reinforced Polymer Composites: The World-Wide Failure Exercise; Elsevier: Amsterdam, The Netherlands, 2004. [Google Scholar]
- Pidaparti, R.M. Engineering Finite Element Analysis; Morgan & Claypool Publishers: San Rafael, CA, USA, 2017. [Google Scholar]
- Schuecker, C.; Pettermann, H.E. A continuum damage model for fiber reinforced laminates based on ply failure mechanisms. Compos. Struct. 2006, 76, 162–173. [Google Scholar] [CrossRef]
- Hallett, S.R.; Jiang, W.-G.; Khan, B.; Wisnom, M.R. Modelling the interaction between matrix cracks and delamination damage in scaled quasi-isotropic specimens. Compos. Sci. Technol. 2008, 68, 80–89. [Google Scholar] [CrossRef]
- Koloor, S.S.R.; Tamin, M. Mode-II interlaminar fracture and crack-jump phenomenon in CFRP composite laminate materials. Compos. Struct. 2018, 204, 594–606. [Google Scholar] [CrossRef]
- Lopes, B.; Arruda, M.R.T.; Almeida-Fernandes, L.; Castro, L.; Silvestre, N.; Correia, J.R. Assessment of mesh dependency in the numerical simulation of compact tension tests for orthotropic materials. Compos. Part C Open Access 2020, 1, 100006. [Google Scholar] [CrossRef]
- Ng, T.P.; Koloor, R.S.S.; Djuansjah, J.R.P.; Abdul, K.M.R. Assessment of compressive failure process of cortical bone materials using damage-based model. J. Mech. Behav. Biomed. Mater. 2017, 66, 1–11. [Google Scholar] [CrossRef]
- Karimzadeh, A.; Koloor, S.S.R.; Ayatollahi, M.R.; Bushroa, A.R.; Yahya, M.Y. Assessment of nano-indentation method in mechanical characterization of heterogeneous nanocomposite materials using experimental and computational approaches. Sci. Rep. 2019, 9, 1–14. [Google Scholar] [CrossRef] [Green Version]
- Rahimian, K.S.S.; Karimzadeh, A.; Tamin, M.N.; Abd, S.M.H. Effects of sample and indenter configurations of nanoindentation experiment on the mechanical behavior and properties of ductile materials. Metals 2018, 8, 421. [Google Scholar] [CrossRef] [Green Version]
- Koloor, S.S.R.; Rahimian-Koloor, S.M.; Karimzadeh, A.; Hamdi, M.; Petrů, M.; Tamin, M. Nano-level damage characterization of graphene/polymer cohesive interface under tensile separation. Polymers 2019, 11, 1435. [Google Scholar] [CrossRef] [Green Version]
- Bassiri, N.A.; Farokhi, N.A.; Xin, L.; Ayob, A.; Yahya, M.Y.; R. Koloor, S.S.; Petrů, M.; Abu, H.S. Dynamic response of aluminium sheet 2024-T3 subjected to close-range shock wave: Experimental and numerical studies. J. Mater. Res. Technol. 2021, 10, 349–362. [Google Scholar] [CrossRef]
- Khan, M.S.; Abdul-Latif, A.; Koloor, S.S.R.; Petrů, M.; Tamin, M.N. Representative Cell Analysis for Damage-Based Failure Model of Polymer Hexagonal Honeycomb Structure under the Out-of-Plane Loadings. Polymers 2020, 13, 52. [Google Scholar] [CrossRef] [PubMed]
- Congro, M.; Roehl, D.; Mejia, C. Mesoscale computational modeling of the mechanical behavior of cement composite materials. Compos. Struct. 2021, 257, 113137. [Google Scholar] [CrossRef]
- Koloor, S.S.R.; Ayatollahi, M.R.; Tamin, M.N. Elastic-damage deformation response of fiber-reinforced polymer composite laminates with lamina interfaces. J. Reinf. Plast. Compos. 2017, 36, 832–849. [Google Scholar] [CrossRef]
- Kolanu, N.R.; Raju, G.; Ramji, M. A unified numerical approach for the simulation of intra and inter laminar damage evolution in stiffened CFRP panels under compression. Compos. Part B Eng. 2020, 190, 107931. [Google Scholar] [CrossRef]
- Lapczyk, I.; Hurtado, J.A. Progressive damage modeling in fiber-reinforced materials. Compos. Part A Appl. Sci. Manuf. 2007, 38, 2333–2341. [Google Scholar] [CrossRef]
- Eyer, G.; Montagnier, O.; Hochard, C.; Charles, J.P. Effect of matrix damage on compressive strength in the fiber direction for laminated composites. Compos. Part A Appl. Sci. Manuf. 2017, 94, 86–92. [Google Scholar] [CrossRef]
- Higuchi, R.; Okabe, T.; Nagashima, T. Numerical simulation of progressive damage and failure in composite laminates using XFEM/CZM coupled approach. Compos. Part A Appl. Sci. Manuf. 2017, 95, 197–207. [Google Scholar] [CrossRef]
- Chang, F.-K.; Lessard, L.B. Damage tolerance of laminated composites containing an open hole and subjected to compressive loadings: Part I—Analysis. J. Compos. Mater. 1991, 25, 2–43. [Google Scholar] [CrossRef]
- Greszczuk, L. Microbuckling of lamina-reinforced composites. In Proceeding of the Composite Materials: Testing and Design (Third Conference), Williamsburg, VA, USA, 21–22 March 1973; ASTM International: West Conshohocken, PA, USA, 1974. [Google Scholar]
- Puck, A.; Schürmann, H. Failure analysis of FRP laminates by means of physically based phenomenological models. Compos. Sci. Technol. 2002, 62, 1633–1662. [Google Scholar] [CrossRef]
- Lee, J.D. Three dimensional finite element analysis of damage accumulation in composite laminate. Comput. Struct. 1982, 15, 335–350. [Google Scholar] [CrossRef]
- Hashin, Z.; Rotem, A. A fatigue failure criterion for fiber reinforced materials. J. Compos. Mater. 1973, 7, 448–464. [Google Scholar] [CrossRef] [Green Version]
- Shahid, I.; Chang, F.-K. An accumulative damage model for tensile and shear failures of laminated composite plates. J. Compos. Mater. 1995, 29, 926–981. [Google Scholar] [CrossRef]
- Maimí, P.; Camanho, P.P.; Mayugo, J.; Dávila, C. A continuum damage model for composite laminates: Part I–Constitutive model. Mech. Mater. 2007, 39, 897–908. [Google Scholar] [CrossRef]
- Murakami, S. Continuum Damage Mechanics: A Continuum Mechanics Approach to the Analysis of Damage and Fracture; Springer Science & Business Media: Berlin/Heidelberg, Germany, 2012; Volume 185. [Google Scholar]
- Kachanov, L. Introduction to Continuum Damage Mechanics; Springer Science & Business Media: Berlin/Heidelberg, Germany, 2013; Volume 10. [Google Scholar]
- Xue, J.; Kirane, K. Effect of the Shape of the Softening Damage Law on the Predicted Fracturing Behavior of Composites. In Proceedings of the American Society for Composites—Thirty-Fifth Technical Conference, Hoboken, NJ, USA, 14–17 September 2020. [Google Scholar]
- Kaw, A.K. Mechanics of Composite Materials; CRC Press: Boca Raton, FL, USA, 2005. [Google Scholar]
- ASTM. D4762–08, Standard Guide for Testing Polymer Matrix Composite Materials. 2008. Available online: https://www.astm.org/Standards/D4762.htm (accessed on 10 December 2020).
- Koloor, R.S.S. Simulation Methodology for Fracture Processes of Composite Laminates Using Damage-Based Models. Ph.D. Degree, Universiti Teknologi Malaysia, Johor, Malaysia, 2016. [Google Scholar]
- Hashin, Z. Failure criteria for unidirectional fiber composites. J. Appl. Mech. 1980, 47, 329–334. [Google Scholar] [CrossRef]
- Orifici, A.; Herszberg, I.; Thomson, R. Review of methodologies for composite material modelling incorporating failure. Compos. Struct. 2008, 86, 194–210. [Google Scholar] [CrossRef]
- ABAQUS-6.9EF. Theory Manual. 2010. Available online: http://130.149.89.49:2080/v6.9ef/index.html (accessed on 21 January 2021).
- Hinton, M.; Kaddour, A. The background to the Second World-Wide Failure Exercise. J. Compos. Mater. 2012, 46, 2283–2294. [Google Scholar] [CrossRef]
- Chaboche, J. The Concept of Effective Stress Applied Elasticity and to Viscoplasticity in Presence of Anisotropic Damage; Royal Aircraft Establishment: Farnborough, UK, 1979; pp. 737–760. [Google Scholar]
- Pinho, S.T. Modelling Failure of Laminated Composites Using Physically-Based Failure Models. Ph.D. Thesis, Imperial College, London, UK, 2005. [Google Scholar]
- Camanho, P.P.; Dávila, C.G. Mixed-mode decohesion finite elements for the simulation of delamination in composite materials. NASA Tech. Pap. 2002, 211737, 33. [Google Scholar]
- Matzenmiller, A.; Lubliner, J.; Taylor, R. A constitutive model for anisotropic damage in fiber-composites. Mech. Mater. 1995, 20, 125–152. [Google Scholar] [CrossRef]
- Kaddour, A.; Hinton, M.; Smith, P.; Li, S. A comparison between the predictive capability of matrix cracking, damage and failure criteria for fibre reinforced composite laminates: Part A of the third world-wide failure exercise. J. Compos. Mater. 2013, 47, 2749–2779. [Google Scholar] [CrossRef]
- Koloor, R.S.S.; Tamin, M. Effects of lamina damages on flexural stiffness of CFRP composites. In Proceedings of the 8th Asian-Australasian Conference on Composite Materials 2012, ACCM 2012—Composites: Enabling Tomorrow’s Industry Today, Kuala Lumpur, Malaysia, 6–8 November 2012; Asian Australasian Association for Composite Materials (AACM): Red Hook, NY, USA, 2013; Volume 1, pp. 237–243. [Google Scholar]
- Koloor, R.S.S.; Karimzadeh, A.; Yidris, N.; Petrů, M.; Ayatollahi, M.R.; Tamin, M.N. An energy-based concept for yielding of multidirectional FRP composite structures using a mesoscale lamina damage model. Polymers 2020, 12, 157. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Varvani-Farahani, A.; Haftchenari, H.; Panbechi, M. An energy-based fatigue damage parameter for off-axis unidirectional FRP composites. Compos. Struct. 2007, 79, 381–389. [Google Scholar] [CrossRef]
- Wang, Y.; Wong, P.; Kodur, V. An experimental study of the mechanical properties of fibre reinforced polymer (FRP) and steel reinforcing bars at elevated temperatures. Compos. Struct. 2007, 80, 131–140. [Google Scholar] [CrossRef]
- Vermeulen, B.; Van Tooren, M. Design case study for a comparative performance analysis of aerospace materials. Mater. Des. 2006, 27, 10–20. [Google Scholar] [CrossRef]
- Camanho, P.P.; Fink, A.; Obst, A.; Pimenta, S. Hybrid titanium–CFRP laminates for high-performance bolted joints. Compos. Part A Appl. Sci. Manuf. 2009, 40, 1826–1837. [Google Scholar] [CrossRef] [Green Version]
- Oya, N.; Hamada, H. Mechanical properties and failure mechanisms of carbon fibre reinforced thermoplastic laminates. Compos. Part A Appl. Sci. Manuf. 1997, 28, 823–832. [Google Scholar] [CrossRef]
- Kumar, S.B.; Sridhar, I.; Sivashanker, S.; Osiyemi, S.; Bag, A. Tensile failure of adhesively bonded CFRP composite scarf joints. Mater. Sci. Eng. B 2006, 132, 113–120. [Google Scholar] [CrossRef]
- Wang, S.-X.; Wu, L.-Z.; Ma, L. Low-velocity impact and residual tensile strength analysis to carbon fiber composite laminates. Mater. Des. 2010, 31, 118–125. [Google Scholar] [CrossRef]
- Fan, J.; Guan, Z.; Cantwell, W. Numerical modelling of perforation failure in fibre metal laminates subjected to low velocity impact loading. Compos. Struct. 2011, 93, 2430–2436. [Google Scholar] [CrossRef]
- Koloor, R.S.S.; Abdul-Latif, A.; Tamin, M.N. Mechanics of Composite Delamination under Flexural Loading. Key Eng. Mater. 2011, 462, 726–731. [Google Scholar] [CrossRef]
- Koloor, R.S.S.; Redzuan, N.; Tamin, M.N. Shear-dominated Interlaminar Fracture Process in CFRP Composite Laminates. In Proceedings of the 9th International Conference on Fracture & Strength of Solids, Jeju Island, Korea, 9–13 June 2013. [Google Scholar]












| Elastic Properties | Damage Model Parameters | |||||||
|---|---|---|---|---|---|---|---|---|
Sets  | 1/2Ref | Ref | 3/2Ref | 1/2Ref | Ref | 3/2Ref | ||
| E11, GPa | 52.75 | 105.5 | 158.25 | Longitudinal tensile strength, MPa | XT | 670 | 1340 | 2010 |
| E22, GPa | 3.6 | 7.2 | 10.8 | Longitudinal compressive strength, MPa | XC | 596 | 1192 | 1788 |
| E33, GPa | 3.6 | 7.2 | 10.8 | Transverse tensile strength, MPa | YT | 9.8 | 19.6 | 29.4 |
| G12, GPa | 1.7 | 3.4 | 5.1 | Transverse compressive strength, MPa | YC | 46.15 | 92.3 | 138.45 |
| G13, GPa | 1.7 | 3.4 | 5.1 | Longitudinal shear strength, MPa | SL | 25.5 | 51 | 76.5 |
| G23, GPa | 1.26 | 2.52 | 3.78 | Transverse shear strength, MPa | ST | 11.5 | 23 | 34.5 |
| ν12 | 0.34 | 0.34 | 0.34 | Longitudinal tensile fracture energy, N/mm | GXT | 24.2 | 48.4 | 72.6 |
| ν13 | 0.34 | 0.34 | 0.34 | Longitudinal compressive fracture energy, N/mm | GXC | 30.15 | 60.3 | 90.45 |
| ν23 | 0.378 | 0.378 | 0.378 | Transverse tensile fracture energy, N/mm | GYT | 2.25 | 4.5 | 6.75 |
| Transverse compressive fracture energy, N/mm | GYC | 4.25 | 8.5 | 12.75 | ||||
| No. | Levels | Composite Case | Specification | Load | Dimension |
|---|---|---|---|---|---|
| 1 | Material | Beam | [45]5 | Tensile | 200 × 20 × 1 mm3 |
| 2 | Structure | ThinPlate 1 | [60/45/90/−45/30] | Three-point bending | 150 × 60 × 1 mm3 |
| ThickPlate 2 | [453/903/03/−453/453] | Three-point bending | 150 × 60 × 3 mm3 | ||
| 3 | Super-structure | Profile | Hat structure: [45/−45/90/45/90] Plate structure: [45/−45/45/−45/45] | Four-point bending | Refer to Figure 3 |
| No. | Properties | Cases | Property Code | FE Model | Case Code |
|---|---|---|---|---|---|
| 1 | Elastic Properties (Figure 2a) | 1 | Ep-1 | Beam | Be-Ep-1 |
| Plate1 | P1-Ep-1 | ||||
| Plate2 | P2-Ep-1 | ||||
| Profile | Pr-Ep-1 | ||||
| Ref | Ep-Ref | Beam | Be-Ep-Ref * | ||
| Plate1 | P1- Ep-Ref ** | ||||
| Plate2 | P2- Ep-Ref *** | ||||
| Profile | Pr-Ep-Ref **** | ||||
| 2 | Ep-2 | Beam | Be-Ep-2 | ||
| Plate1 | P1-Ep-2 | ||||
| Plate2 | P2-Ep-2 | ||||
| Profile | Pr-Ep-2 | ||||
| 2 | Strength values (Figure 2b) | 1 | S-1 | Beam | Be-S-1 |
| Plate1 | P1-S-1 | ||||
| Plate2 | P2-S-1 | ||||
| Profile | Pr-S-1 | ||||
| Ref | S-Ref | Beam | Be-S-Ref * | ||
| Plate1 | P1-S-Ref ** | ||||
| Plate2 | P2-S-Ref *** | ||||
| Profile | Pr-S-Ref **** | ||||
| 2 | S-2 | Beam | Be-S-2 | ||
| Plate1 | P1-S-2 | ||||
| Plate2 | P2-S-2 | ||||
| Profile | Pr-S-2 | ||||
| 3 | Energy values (Figure 2c) | 1 | E-1 | Beam | Be-E-1 |
| Plate1 | P1-E-1 | ||||
| Plate2 | P2-E-1 | ||||
| Profile | Pr-E-1 | ||||
| Ref | E-Ref | Beam | Be-E-Ref * | ||
| Plate1 | P1-E-Ref ** | ||||
| Plate2 | P2-E-Ref *** | ||||
| Profile | Pr-E-Ref **** | ||||
| 2 | E-2 | Beam | Be-E-2 | ||
| Plate1 | P1-E-2 | ||||
| Plate2 | P2-E-2 | ||||
| Profile | Pr-E-2 | ||||
| 4 | Strength and Energy values (Figure 2d) | 1 | SE-1 | Beam | Be-SE-1 |
| Plate1 | P1-SE-1 | ||||
| Plate2 | P2-SE-1 | ||||
| Profile | Pr-SE-1 | ||||
| Ref | SE-Ref | Beam | Be-SE-Ref * | ||
| Plate1 | P1-SE-Ref ** | ||||
| Plate2 | P2-SE-Ref *** | ||||
| Profile | Pr-SE-Ref **** | ||||
| 2 | SE-2 | Beam | Be-SE-2 | ||
| Plate1 | P1-SE-2 | ||||
| Plate2 | P2-SE-2 | ||||
| Profile | Pr-SE-2 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
R. Koloor, S.S.; Karimzadeh, A.; Abdullah, M.R.; Petrů, M.; Yidris, N.; Sapuan, S.M.; Tamin, M.N. Linear-Nonlinear Stiffness Responses of Carbon Fiber-Reinforced Polymer Composite Materials and Structures: A Numerical Study. Polymers 2021, 13, 344. https://doi.org/10.3390/polym13030344
R. Koloor SS, Karimzadeh A, Abdullah MR, Petrů M, Yidris N, Sapuan SM, Tamin MN. Linear-Nonlinear Stiffness Responses of Carbon Fiber-Reinforced Polymer Composite Materials and Structures: A Numerical Study. Polymers. 2021; 13(3):344. https://doi.org/10.3390/polym13030344
Chicago/Turabian StyleR. Koloor, S. S., A. Karimzadeh, M. R. Abdullah, M. Petrů, N. Yidris, S. M. Sapuan, and M. N. Tamin. 2021. "Linear-Nonlinear Stiffness Responses of Carbon Fiber-Reinforced Polymer Composite Materials and Structures: A Numerical Study" Polymers 13, no. 3: 344. https://doi.org/10.3390/polym13030344
APA StyleR. Koloor, S. S., Karimzadeh, A., Abdullah, M. R., Petrů, M., Yidris, N., Sapuan, S. M., & Tamin, M. N. (2021). Linear-Nonlinear Stiffness Responses of Carbon Fiber-Reinforced Polymer Composite Materials and Structures: A Numerical Study. Polymers, 13(3), 344. https://doi.org/10.3390/polym13030344