Next Article in Journal
Impact of Damaging Geo-Hydrological Events and Population Development in Calabria, Southern Italy
Previous Article in Journal
Selecting Sustainable Drainage Structures Based on Ecosystem Service Variables Estimated by Different Stakeholder Groups
 
 
Search for Articles:
Title / Keyword
Author / Affiliation / Email
Journal
Article Type
 
 
Advanced Search
 
Section
Special Issue
Volume
Issue
Number
Page
 
Journals
Water
Volume 5
Issue 4
10.3390/w5041760
water-logo
Submit to this Journal Review for this Journal Propose a Special Issue
► Article Menu

Article Menu

share Share announcement Help format_quote Cite question_answer Discuss in SciProfiles thumb_up
...
Endorse textsms
...
Comment
first_page
settings
Order Article Reprints
Font Type:
Arial Georgia Verdana
Font Size:
Aa Aa Aa
Line Spacing:
Column Width:
Background:
Article

How Much for Water? Economic Assessment and Mapping of Floodplain Water Storage as a Catchment-Scale Ecosystem Service of Wetlands

by
Mateusz Grygoruk
1,2,*,
Dorota Mirosław-Świątek
1,
Weronika Chrzanowska
1 and
Stefan Ignar
1
1
Department of Hydraulic Engineering, Warsaw University of Life Sciences—SGGW, ul. Nowoursynowska 159, Warsaw 02-776, Poland
2
Biebrza National Park, Osowiec-Twierdza 8, Goniądz 19-110, Poland
*
Author to whom correspondence should be addressed.
Water 2013, 5(4), 1760-1779; https://doi.org/10.3390/w5041760
Submission received: 26 August 2013 / Revised: 11 October 2013 / Accepted: 16 October 2013 / Published: 1 November 2013
Browse Figures
Versions Notes

Abstract

:
The integration of water management goals in protected wetland areas agriculturally managed in an intensive manner recalls the comparison of apples (ecological values) and oranges (economic dimension of agriculture). Sustainable wetland management frequently fails if environmental features are not referred to as ecosystem services and quantified in economic terms. In our hydrological-economical study on floodplain wetlands located in the Lower Basin of the Biebrza Valley, we attempt to quantify the monetary value of water storage in the floodplain during flood phenomena as an important ecosystem service. The unit monetary value of water storage in the catchment of Biebrza Valley was assessed on the basis of small artificial water reservoirs, constructed in recent years and located in the area of research, and reached 0.53 EUR·m−3·year−1. In a GIS-based study on hydrological floodplain processes in the years 1995–2011, we assessed the average annual volume of active water storage in the floodplain which reached 10.36 M m3 year−1, giving a monetary value of EUR 5.49 million per annum. We propose that the methodology presented in our analysis could be applied as water storage subsidies in valuable floodplains, to prevent their deterioration originating from agriculture intensification.

Graphical Abstract

1. Introduction

The water storage role of wetlands has become one of the hot topics in the contemporary worldwide discussion on possible measurable benefits which come along with an appropriate status and function of those ecosystems [1,2,3]. As ecology-based messages rarely find positive feedback from stakeholders, the economic valuation of wetland functions must be implemented in environmental management to enhance the efficiency of nature conservation [4,5]. It is otherwise likely that economic gains from extensive agriculture will take over wetland management, and that increasing human pressures aimed at intensive use of wetlands will influence decision-making and possibly deteriorate wetland ecosystems. So far, the result of human-enforced adaptation of wetland ecosystems for the purpose of agriculture, industry, and urbanization has resulted in degradation for the majority of European wetlands [6]. This has occurred mainly due to drainage. Despite numerous environmental conservation policies implemented throughout the continent (Natura 2000, National Parks, Habitat-Directive and Bird-Directive sites), drainage of well-preserved and near-natural wetlands still remains a vast challenge for their conservation in the face of increasing intensity of agriculture.
Agricultural development supported by EU funds in Central Europe (agro-environmental schemes and direct payments that reflect the implementation of the common agricultural policy) has expanded over the last decade, underpinning a serious threat to the sustainability of wetlands. Formerly abandoned wetland meadows, frequently of high ecological value, suddenly became valuable in economic terms as well—species-oriented agricultural payment schemes (e.g., for the Aquatic warbler Acrocephalus paludicola or the Corncrake Crex crex) brought these wetlands back to active management. In certain areas, such as those in northeastern Poland where the valuable wetland ecosystems (e.g., Figure 1A) cover a vast area, tens of thousands of hectares of floodplain meadows are again maintained and mowed in order to receive funds from EU agro-environmental schemes. Management policies that support the sustainable use of wetlands in general oblige payment-benefited land users to mow wetland meadows. However, this is the only prerequisite for the agro-environmental payment to be fulfilled. If the meadow is not mown, then the subsidies are limited. Such a mechanism forces land users to mow their meadows regardless of physical conditions and climatic variability. Though, flood phenomena which naturally and regularly occur in natural lowland floodplains, become a limiting factor for agriculture. Hence, as an obstacle to “regional development”, floodplains in many regions are challenged—especially in wetter years—by increasing pressure on drainage.
In the most recent decade, Central Europe faced frequent summer floods due to climatic variability, which occurred more often than in the previous five decades [7,8]. As observed in Poland, high water levels in managed wetlands during the summers of 2010 and 2011 created a series of conflicts [9]. Even though the assumptions of the EU agro-environmental schemes are oriented towards species conservation and habitat preservation, this climatic variability caused pressures to increase drainage and undertake intensive river dredging. The result: a broad-scale degradation of aquatic ecosystems [10]. To “dry up” the land remained a dogmatic and policy-supported goal of regional water management authorities. Despite protests by local NGOs and the start of an adaptive-management-related stakeholder dialogue, numerous (sometimes illegal) river training and drainage projects were started (Figure 1B). Support for this process had an economic origin: floods on maintained meadows (of water levels seldom exceeding 0.5 m) limit accessibility to the land and prohibit mowing, thus limiting income.
Water 05 01760 g001 1024
Figure 1. (A) Natural floodplain wetlands in the Lower Biebrza Basin; (B) Freshly (2011) trained river Klimaszewnica—a tributary of the Biebrza in the Lower Biebrza Basin.
Figure 1. (A) Natural floodplain wetlands in the Lower Biebrza Basin; (B) Freshly (2011) trained river Klimaszewnica—a tributary of the Biebrza in the Lower Biebrza Basin.
Water 05 01760 g001
At the same time, as increasing water storage capacity in a catchment scale is required by the Water Framework Directive and numerous regional programs [11] implemented by member states of the EU and aimed at implementation of sustainable agriculture, the construction of water storage reservoirs has recently intensified. These small-volume reservoirs (Figure 2B) are constructed (which also qualify for EU subsidies) with a volume seldom exceeding 0.5 M m3 (normally reaching less than 100,000 m3). The ultimate goal of these reservoirs is water storage, according to the assumptions of financing bodies, designers and investors, for agriculture.
Integrated water resources management requires considering water as a benefit in a catchment scale [12,13]. Therefore, agricultural water management aimed in one particular catchment at increased water storage on one hand, and on the other at increased intensity of the drainage and drying of agriculturally maintained wetland meadows, can be considered as internally incoherent. Moreover, if drainage activities on lands receiving EU funds (Figure 1B) for biodiversity and species abundance (as is the case for most wetlands in NE Poland) are the result of farmers’ pressures, and at the same time those farmers claim the need of construction of storage reservoirs, further efforts should be taken to present this incoherence and show the true economic dimension of meadow flooding (i.e., water storage), which is profitable for a much broader audience than it appears negative for farmers.
The main goal of our study is to evaluate and assess the economic context of water storage in a lowland catchment. Our approach is as follows: (i) we evaluate the unit cost of water storage in artificial reservoirs constructed in years 2000–2010 and planned for construction in the near future within the catchment of the Biebrza River (NE Poland); (ii) we evaluate floodplain storage in the river valley on the basis of GIS analysis and hydrological data on water levels and lastly; (iii) we assess the annual monetary value of water stored in the floodplain during flood phenomena recorded in years 1995–2011. In the latter part of our paper we discuss the practical possibilities of implementing our approach in water management policies for protected floodplains in order to prevent their further ecologic deterioration by drainage, and through highlighting their role on a catchment scale by putting water storage in the floodplain in economic terms.

2. Materials and Methods

2.1. Wetlands of the Lower Biebrza Basin—Nature and Agriculture

Catchment of the Biebrza River and the Biebrza Valley are located in NE Poland (Figure 2A). The Biebrza is a tributary of the Narew River. Wetlands of the Biebrza Valley remain one of the best preserved and the broadest in coherent extent within the European Lowlands [14]. Ecological relations in ecosystems of the Biebrza Valley, and the Lower Biebrza Basin in particular, are considered as natural and related to hydrological processes, of which the seasonal flooding (in riparian wetlands) and constantly high groundwater levels (in groundwater fed fens) remain the most important processes that sustain the biodiversity [15,16].
Water 05 01760 g002 1024
Figure 2. (A) Study area—catchment of the Biebrza (NE Poland) and the Lower Biebrza Basin; (B) Bobra Wielka—construction of the artificial storage reservoir; (C) Floodplain of the Lower Biebrza Basin during the spring flood event in March 2007. 1 major towns; 2 artificial storage reservoirs (created and planned); 3 drainage network; 4 lakes/flood extent in the Lower Biebrza Basin; 5 watershed of the Biebrza; 6 Biebrza National Park; 7 Natura 2000 sites.
Figure 2. (A) Study area—catchment of the Biebrza (NE Poland) and the Lower Biebrza Basin; (B) Bobra Wielka—construction of the artificial storage reservoir; (C) Floodplain of the Lower Biebrza Basin during the spring flood event in March 2007. 1 major towns; 2 artificial storage reservoirs (created and planned); 3 drainage network; 4 lakes/flood extent in the Lower Biebrza Basin; 5 watershed of the Biebrza; 6 Biebrza National Park; 7 Natura 2000 sites.
Water 05 01760 g002
Riparian wetland meadows located in the Lower Biebrza Basin (Figure 2C) are flooded each year as a result of the spring snowmelt floods, which occur from late February up to May [7,17]. These floods are most likely enhanced by controlled discharge from lakes located upstream in the catchment. In wet years, summer flooding also occurs. Average momentary discharge of the Biebrza reaches 22.4 m3·s−1 in the upper-most profile of the Lower Biebrza Basin (Osowiec) and 33.5 m3·s−1 in the lower-most profile of the Lower Biebrza Basin (Burzyn). The bankful discharge reaches 26.7 m3·s−1 and 40.5 m3·s−1 respectively [7,17]. Such a small difference between the average momentary discharge and the bankful discharge shows that flooding is still a relatively frequent phenomenon in the Lower Biebrza Basin, which determines intensive water and nutrient exchange within the floodplain, and is at the same time an obstacle for mechanic agricultural measures.
The Biebrza Valley is well known as one of the most important European stopover sites for migratory birds [18]. In order to manage and protect the unique wetlands of the Biebrza Valley, the Biebrza National Park was established in 1993. Today, conservation activities are aimed at preservation and the increase of suitable habitats for rare species of flora and fauna. The majority of actions are oriented at the reduction/prevention of secondary succession of shrubs to open areas, which are considered a threat to protected birds (e.g., Aquatic Warbler Acrocephalus paludicola and the Greater Spotted Eagle Aquila clanga). Tens of hectares of wetland meadows in the Biebrza Valley are subsidized with EU funds and so must be mown on a regular basis; mostly mechanical methods of mowing are used (tractors, adjusted mowing rattracks, mechanic mowers). As approximately 40% of the Biebrza National Park’s area remains private, many conflicts arise regarding the flooding of wetland meadows [9].

2.2. Economic Assessment of Water Storage Cost in the Catchment of the Biebrza

The average unit cost of storage of 1 m3 of water (the Scost) in the Biebrza catchment was calculated on the basis of available data on expenses of local authorities on design, construction, and maintenance of storage reservoirs in recent years. Only those reservoirs whose sole function is “water storage” (indicated in technical documentation) were taken into consideration. Due to the limited availability of data on technical properties, budgets, and financing of such investments, only nine objects located in the catchment of Biebrza could be described in the required data configuration (Figure 2A, Table 1). The Scost in the catchment of Biebrza was calculated as a weighted mean of unit costs of water storage in each of the analyzed objects, according to the Equation (1):
Water 05 01760 i001
where Scost is the average, weighted unit cost of storage of 1 m3 of water per year in a particular artificial storage reservoir, expressed in EUR·m−3·yea−1; Rc is the total sum of expenses spent on design and construction of a particular artificial storage reservoir, expressed in EUR; M is the estimated cost of reservoir maintenance in the Dr−1 time; Dr is the depreciation rate per annum (dimensionless); and Rv is the volume of a particular artificial storage reservoir, expressed in m3. Data on Rc and Rv were assessed on the basis of official information given in public procurement procedures announced in a public bid. Therefore, we assume that the calculated Scost remains the market-based economic estimate of the water storage cost, representative of current social and economic demand in a catchment scale, and balanced by possible “water storage service” supply. According to Polish legislation, the Dr value—amortization (depreciation) rate of permanent assets, such as hydrotechnic investments including storage ponds, weirs, and drainage systems—equals 4.5% per annum. Hence, permanent assets depreciate totally after approximately 22 years. Therefore, we assumed that the analyzed storage investments were strategically planned for 22 years. In our analysis we neglect any costs of reservoir maintenance after it was constructed, as no precise data in this matter could be retrieved from the maintaining authorities. Thus, in our calculations, M = 0 despite the fact, that this assumption can entail significant underestimation of unit costs of water storage. For six objects, located in the southern part of the catchment (Bobra Wielka, Janów-Sitawka, Jasionówka, Karpowicze, Korycin and Szumowo-Olszynka), detailed data on expenses and hydrological parameters of the reservoirs were obtained directly from local authorities. The remaining three objects, located in the northern part of the Biebrza catchment (two storage ponds in Borki Forest District and one storage pond in Szczebra Forest District), have become relatively little ponds constructed by the State Forests of Poland. For these objects only the data on water storage volume could be obtained. The price of water storage in these three reservoirs was assessed on the basis of values given by Tyszka [19] and equaled 5.83 PLN·m−3 (1.3 EUR·m−3 approximately) per average object of this kind in Poland. However, in the approach of Tyszka [19], the depreciation rate was not included and the unit cost was only calculated as Rc/Rv [refer to Equation (1)]. In order to provide comprehensive data on water storage cost in these reservoirs per annum, we divided this value by 22 (similar to the other values), which represents depreciation at the same rate (4.5%) as the other objects analyzed.
Table
Table 1. Artificial storage ponds considered in the economic assessment of unit cost of waterstorage in the catchment of Biebrza. * Currency exchange rate used in the analysis 1 EUR =4 PLN; ** approximate value calculated on the basis of Tyszka [19]. Explanation in text.
Table 1. Artificial storage ponds considered in the economic assessment of unit cost of waterstorage in the catchment of Biebrza. * Currency exchange rate used in the analysis 1 EUR =4 PLN; ** approximate value calculated on the basis of Tyszka [19]. Explanation in text.
ReservoirYearVolume [M m3]Approx. cost of design and construction [PLN]Approx. cost of design and construction [EUR] *Unit cost of water storage [EUR·m−3·year−1]
Korycin20020.4811,500,000375,0000.04
Karpowicze20090.0775,489,1501,372,2880.81
Janów-Sitawka20060.0871,300,000325,0000.17
Bobra Wielka20120.06314,700,0003,675,0002.67
Jasionówka20010.067581,328145,3320.10
Szumowo–Olszynka20120.0805,500,0001,375,0000.78
Storage Pond–Borki Forest Districtn.a.0.020n.a.n.a.0.07 **
Storage Pond–Borki Forest Districtn.a.0.024n.a.n.a.0.07 **
Storage Pond-Szczebra Forest Districtn.a.0.001n.a.n.a.0.07 **
Statistics-Ʃ = 0.90Ʃ = 29,070,478Ʃ = 7,267,620avg = 0.53

2.3. Floodplain Storage Volume Assessment

The stretch of Biebrza Valley between the Osowiec and Burzyn (the Lower Biebrza Basin) was the focus of the analysis. Flood extents in this zone were comprehensively studied as to their spatial and temporal dynamics in both field monitoring [7,15,16,17] and modeling manners [20,21,22]. The research on flood dynamics and floodplain water storage was based on water levels and discharges of the Biebrza River, recorded in the period 1995–2011 by the National Institute of Meteorology and Water Management (IMGW). Basing upon previous research, and due to some constraints resulting from the uncertain accuracy of a rating curve in Burzyn (floodplain width reaches 4–8 km and lack of discharge measurements during high water level conditions), we assumed the classic approach to storage calculation expressed as the sum of momentary differences between the inflow and outflow to the floodplain will not represent reality in the analyzed case. In our approach, we analyzed flood extent in the Lower Biebrza Basin as a function of water level on the Burzyn water gauge (Figure 3 and Figure 4). On the basis of field observations, we observed that the water level of bankful flow in Lower Biebrza Basin is represented by the approximate value of 250 cm on the Burzyn gauge. Although this water level does not represent the overbank flow on the Burzyn gauge itself, it represents the threshold when the flood starts in the floodplain between the gauges of Burzyn and Osowiec [23].
Water 05 01760 g003 1024
Figure 3. Example assessment of average water level for particular flood phenomenon on the basis of water level hydrograph measured on the Burzyn water gauge. Source of data: Institute of Meteorology and Water Management (IMGW). 1 water level hydrograph in exemplary dry years (01/11/1995–31/10/1997); 2 average water levels during flood phenomena; 3 water level hydrograph in exemplary wet years (01/11/2009–31/10/2011); 4 average water levels during flood phenomena; 5 flood threshold.
Figure 3. Example assessment of average water level for particular flood phenomenon on the basis of water level hydrograph measured on the Burzyn water gauge. Source of data: Institute of Meteorology and Water Management (IMGW). 1 water level hydrograph in exemplary dry years (01/11/1995–31/10/1997); 2 average water levels during flood phenomena; 3 water level hydrograph in exemplary wet years (01/11/2009–31/10/2011); 4 average water levels during flood phenomena; 5 flood threshold.
Water 05 01760 g003
The main aim of our approach in the floodplain storage calculation was to derive the relation between average water levels of a particular flood phenomenon and the corresponding flood extents, and so establish floodplain water storage volumes (later on referred to as H-Fvol relation). Delineation of flood extents was done in a five-step approach. Firstly, in order to cover the broadest possible range of water levels in a H-Fvol relation, we calculated values of the discharges of particular probable exceedance frequencies (FQp%) with the classic quantile-based statistical approach with the Pearson 3rd type distribution applied [24,25]. FQp%s were assessed for Osowiec and Burzyn gauges for 1, 2, 5, 10, 20 and 50 percent theoretical exceedance frequencies. Secondly, on the basis of long term data on discharges and water levels in Osowiec and Burzyn, we assessed water levels that corresponded to particular values of calculated FQp%s. Thirdly, the corresponding values of water levels for particular FQp%s were interpolated along the course of the Biebrza River between Osowiec and Burzyn. The distribution of longitudinal slopes of the water table on this river stretch was verified during numerous field measurement campaigns conducted during the spring and summer floods in 2007–2013. Fourthly, interpolated values were assigned to the cross sections distributed through the valley, downstream from Osowiec and towards Burzyn. Cross sections representing changing elevations across the valley were derived from DEM (elaborated by Świątek and Chormański [20], verified by Szporak et al. [26], and improved with data from additional topographic field measurements) every kilometer of the valley. As the analyzed floods were represented by the average water levels measured during each of the flood events (Figure 3), we assumed that the constant water levels along each of the cross sections, equal to the water levels in the river in a particular stretch, could have been assigned. Next, the automatic interpolation was applied in order to delineate the water level in the valley for particular conditions of FQp% in between the cross sections. In the fifth step, the interpolated plain of the water table was intersected with the DEM of the Lower Biebrza Basin, which allowed the delineation of the flood extents (Figure 4A,B).
Water 05 01760 g004 1024
Figure 4. Flood extent delineation in the Lower Biebrza Basin. (A) Flood of 1% exceedance probability; (B) flood of 50% exceedance frequency; (C) flood extent delineated for August 2011. 1 water gauge; 2 drainage network; 3 boundaries of Biebrza National Park; 4 flood extent.
Figure 4. Flood extent delineation in the Lower Biebrza Basin. (A) Flood of 1% exceedance probability; (B) flood of 50% exceedance frequency; (C) flood extent delineated for August 2011. 1 water gauge; 2 drainage network; 3 boundaries of Biebrza National Park; 4 flood extent.
Water 05 01760 g004
In order to get additional flood extents in the H-Fvol analysis, some field measured water elevations were interpolated and the flood extents for particular measured conditions were delineated (e.g., Figure 4C). As presented, flood extents reflect water levels derived from the river only and neglect the cross-sectional variability of the slope of the water table (on the edges of the valley water levels might be higher/lower than in the river). One can conclude that flood volumes and extents assessed for particular water levels with the presented methodology represent values close to the average conditions. The analysis of water levels, flood extents, and flood volumes allowed empirical relationships between these variables to be derived (Figure 5A,B). The authors are aware that in summer and autumn, when the floodplain vegetation develops intensively, the total volume of flood should be reduced by the volume of vegetation. However, due to a lack of precise data we decided to calculate both winter/spring and summer/autumn flood volumes with the same regression, and recommend the analysis of the influence of vegetation on flood volume in the summer/autumn periods for future research.
Water 05 01760 g005 1024
Figure 5. (A) Relation between water level of Biebrza (Burzyn gauge) and the volume of flood in the Lower Biebrza Basin; (B) Relation between water level of Biebrza (Burzyn gauge) and the area of flood in the Lower Biebrza Basin (stretch of the valley between Osowiec water gauge and Burzyn water gauge).
Figure 5. (A) Relation between water level of Biebrza (Burzyn gauge) and the volume of flood in the Lower Biebrza Basin; (B) Relation between water level of Biebrza (Burzyn gauge) and the area of flood in the Lower Biebrza Basin (stretch of the valley between Osowiec water gauge and Burzyn water gauge).
Water 05 01760 g005
In total “active” storage calculations, in addition to the surface water storage (Fv) derived for the particular average water levels in flood conditions, we took into consideration the shallow groundwater stored in the peat up to the critical depth which determines the possibility of using mechanical equipment (tractors, rattracks) in agricultural practices on floodplain meadows due to too high saturation of the soil. Therefore, the total water storage capacity of the Biebrza floodplain between the profiles of Osowiec and Burzyn (StWet) was calculated as shown in the Equation (2):
Water 05 01760 i002
where StWet is the storage volume of floodplain, expressed in m3; Fv is the volume of flood calculated on the basis of the derived relation between the GIS-estimated valley storage and water level (Figure 5A), expressed in m3; 𝜙 is the dimensionless value of the porosity of a superficial layer of soil; Fa is the area of flood assessed on the basis of GIS analysis and water level modeling in the Lower Biebrza Basin, expressed in m2 and representative for particular water levels (Figure 5B); Cd is the critical water table depth, above which the implementation of agricultural practices (mowing) is impossible due to the high saturation of the soil.
It is assumed, that once the water table remains shallower than 0.15 m, then mechanical mowing is impossible because of high saturation of the peat soil. Normally above this water level, farmers claim that the drainage should be enhanced. The porosity of flooded peat soils was assessed as high as 0.85. Tf is the dimensionless index expressing the fraction of the year when the floodplain was flooded (in our approach, when the water level in Burzyn was higher than 250 cm). The term Tf in Equation (2). Represents in fact the time when active water storage in the floodplain was occurring. It reflects the duration curve, meaning that Tf = 1 represents the hypothetical situation of a flood that lasted 365 days (throughout the whole year water level in Burzyn was above 250 cm) and Tf = 0 characterizes the situation when at any day of the year the water level in Burzyn did not exceed 250 cm. In our approach, we neglect the amount of water stored in the 0.15 m depth of soil outside of the flooded range. We state that in areas not reached by the flood waters there are factors other than the flood entailing hydrological processes (e.g., groundwater discharge). Although these processes can somehow keep in the feedback with the flood, we do not consider them in our analysis. Hence, our calculations of the floodplain volume are likely to be underestimated in the catchment-scale. In our research we do not consider water stored in peat below the critical depth of 0.15 m either. This water is not mobile and in most cases does not participate in the short-term water exchange in the analyzed system. Finally, the annual floodplain storage economic value was calculated as:
Water 05 01760 i003
where FloVal is the economic value of floodplain storage in a particular year; StWet is the storage volume of the floodplain, expressed in m3 [Equation (2)]; Scost is the average, weighted unit cost of storage of 1 m3 of water per year in the catchment of Biebrza [Equation (1)].
This comprehensive methodology of the floodplain water storage volume assessment reflects the actual and dynamic volume of water taking part in active water exchange during floods. The proposed algorithm includes only water which is somehow problematic for farmers and land owners. Hence we conclude, that in the analyzed example of a near-natural floodplain, the calculated storage volumes can be considered as active and exchangeable water, which—although not directly maintained in a reservoir-manner—theoretically becomes an equivalent of water stored in artificial reservoirs when considered in a catchment scale.

2.5. Economic Assumptions of the Analysis

In order to compare water storage of artificial reservoirs and the floodplain during flood events in one and the same catchment, we assume that the caeteris paribus rule covers any storage within one catchment. Practically, if one is willing to pay for any design, construction, or maintenance of an artificial storage reservoir whose only goal is water storage, and the unit cost of water storage can be assessed according to Equation (1), then the floodplain storage, as is, is worth the same amount of capital. Also, if the storage-related issues are analyzed in a catchment scale (e.g., flood protection for areas located downstream of the analyzed catchment) then both the storage in artificial reservoirs and the floodplain storage during floods in one catchment provide the same output, which is reduction of the discharge to the reaches of the river located downstream from the analyzed catchment.

3. Results

3.1. Unit Cost of Water Storage in Artificial Reservoirs in the Catchment of Biebrza

Data on costs of design and construction of particular minor reservoirs aimed at water storage in the catchment of Biebrza led to an estimate of the average unit cost of water storage in those reservoirs as high as 0.53 EUR·m−3·year−1 (Table 1).
The unit cost of water storage in particular reservoirs varied from 0.04 EUR·m−3·year−1 in the case of the Korycin pond up to 2.67 EUR·m−3·year−1 in the case of the Bobra Wielka reservoir (Table 1, Figure 6). One can observe that in general the reservoirs constructed in the early 2000s store water “cheaper” than the ponds constructed later. This can be related to the accession of Poland to the EU, when additional sources of financing such as investments in storage ponds were started, and local authorities as well as regional boards of irrigation and drainage could apply for additional funding. The analysis indicated that water storage in reservoirs supervised by the State Forests of Poland (storage ponds in Borki and Szczebra Forest Districts) is more efficient in terms of economy than in the remaining reservoirs analyzed, excluding the Korycin Pond (Figure 6). Despite the differences in unit cost of water storage in the particular reservoirs analyzed, one can conclude that the given values are of a similar order of magnitude.
Water 05 01760 g006 1024
Figure 6. Location, volume, and design/construction costs of selected water storage investments, undertaken in years 2000–2012 within the catchment of the Biebrza River. Calculated unit cost of water storage is given by each location. 1 storage ponds; 2 major towns; 3 rivers and canals; 4 lakes; 5 watershed of the Biebrza catchment; 6 boundaries of the Biebrza National Park; 7 Natura 2000 sites.
Figure 6. Location, volume, and design/construction costs of selected water storage investments, undertaken in years 2000–2012 within the catchment of the Biebrza River. Calculated unit cost of water storage is given by each location. 1 storage ponds; 2 major towns; 3 rivers and canals; 4 lakes; 5 watershed of the Biebrza catchment; 6 boundaries of the Biebrza National Park; 7 Natura 2000 sites.
Water 05 01760 g006
The total amount of water stored in the analyzed reservoirs reached nearly 1 M m3, which can be considered a relatively small value if compared to the regular size of reservoirs constructed in order to prevent floods. Moreover, the storage capacity of all analyzed reservoirs is much smaller than the capacity of the Lower Biebrza’s floodplain storage derived on the basis of algorithms and GIS analysis [Equations (1), (2) and Figure 5A].

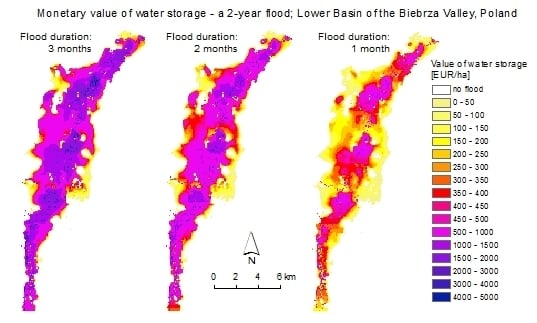
3.2. Hydroeconomy of Water Storage in the Floodplainof the Biebrza

On the basis of the water level hydrograph for the Burzyn gauge, we selected all the flood events above the assumed threshold of 250 cm and the average water level for each of the flood events was calculated. Using the assumptions presented in Equation (2) and Figure 5, we evaluated the floodplain storage volume for each flood phenomenon (Table 2) and the annual active water storage in the Lower Biebrza Basin (Figure 7A).
In the last step of our analysis, the total calculated floodplain water storage was multiplied by the unit cost of water storage assessed on the basis of economic evaluation of artificial reservoirs [Equation (3), Table 1], so the monetary value of water storage in the Lower Biebrza Basin for particular years between 1995 and 2011 (Figure 7B) could be estimated.
Table
Table 2. Calculations of the total active water storage volume in the floodplain of the Lower Biebrza Basin. Hydrological data obtained from the Institute of Meteorology and Water Management (IMGW).
Table 2. Calculations of the total active water storage volume in the floodplain of the Lower Biebrza Basin. Hydrological data obtained from the Institute of Meteorology and Water Management (IMGW).
Date of flood startAverage water levelFlood duration [days]TfSeasonFv [mln m3]Fa [km2]StWet [mln m3]
3 Nov. 1995270850.23winter/spring10.236.992.59
2 Mar. 19963191180.32winter/spring36.7635.1913.34
17 Dec. 199625440.01winter/spring2.831.100.03
14 Feb. 1997270400.11winter/spring10.146.891.21
4 May 1997256320.09winter/spring3.800.000.33
5 Aug. 199725890.02summer/autumn2.360.960.06
5 Jun. 19982961930.53summer/autumn14.2821.048.97
19 Jul. 19993022580.71summer/autumn16.5824.8613.96
11 May 20002961550.42winter/spring23.3521.0511.06
5 Jan. 2001262100.03winter/spring6.573.020.19
7 Feb. 2001266170.05winter/spring8.184.770.41
19 Feb. 2001260100.03winter/spring5.662.030.16
5 Mar. 200126470.02winter/spring7.594.130.16
15 May 2001269650.18winter/spring9.636.351.86
11 May 20022932170.59winter/spring21.6319.2214.32
5 May 20032711060.29winter/spring10.917.723.45
9 Jan. 2004271210.06winter/spring10.837.650.68
7 Jun. 20042911470.40summer/autumn12.5318.125.98
15 Oct. 2004254450.12summer/autumn1.221.000.17
29 Jun. 20053052360.65summer/autumn17.6426.6113.60
17 Jan. 2006272210.06winter/spring11.047.870.69
14 May 2006279770.21winter/spring14.4411.533.36
28 Oct. 2006259500.14summer/autumn2.691.510.39
20 May 20073061920.53winter/spring29.1527.1917.16
23 Aug. 2007253120.03summer/autumn0.861.600.03
3 Jun. 20083001970.54summer/autumn15.7223.4310.10
9 May 20092921420.39winter/spring21.5719.169.34
7 Jul. 2009254140.04summer/autumn1.211.000.05
31 Jul. 200925140.01summer/autumn0.432.400.01
9 Aug. 20103072750.75summer/autumn18.3027.6916.44
5 Jun. 20113272760.76summer/autumn26.1540.5723.68
8 Jul. 2011284670.18summer/autumn10.4314.612.26
Water 05 01760 g007 1024
Figure 7. (A) Volume of water stored within the floodplain of the Lower Biebrza Basin in particular years of the period 1995–2011; and (B) The annual monetary value of water stored within the floodplain of the Lower Biebrza Basin, in particular years of the period 1995–2011.
Figure 7. (A) Volume of water stored within the floodplain of the Lower Biebrza Basin in particular years of the period 1995–2011; and (B) The annual monetary value of water stored within the floodplain of the Lower Biebrza Basin, in particular years of the period 1995–2011.
Water 05 01760 g007
Water 05 01760 g008 1024
Figure 8. Maps of the monetary values of stored water [EUR/ha] in the Lower Biebrza Basin in conditions of flood of 50% exceedance probability, assuming that the area was flooded (A) 3 months; (B) 2 months; and (C) 1 month.
Figure 8. Maps of the monetary values of stored water [EUR/ha] in the Lower Biebrza Basin in conditions of flood of 50% exceedance probability, assuming that the area was flooded (A) 3 months; (B) 2 months; and (C) 1 month.
Water 05 01760 g008
Once the unit monetary value of water storage was estimated, it was possible to assess the approximate value of the flood water storage that occurs during the FQp50% (a so-called normal flood or a 2-year flood). This phenomenon can be considered as regular and therefore representative for the analysis of the interface of flood and affected agriculture in the Biebrza Valley. With use of the FQp50% flood extent (Figure 4B) and the DEM, we could assess approximate depths of the flood and—referring to the assumption of Equations (2) and (3)—the monetary values of water storage in the analyzed floodplain. To cover the most frequent and most problematic situations for agriculture we assessed a FQp50% flood, that hypothetically lasted for 3, 2 and 1 month(s) (Figure 8A–C respectively). As the flood extent was in each case the same, only the parameter Tf [Equation (2)] determined the floodplain storage monetary value calculation.
We estimated, that during the analyzed hypothetical 2-year flood of assumed duration 1, 2 and 3 months, the average monetary values of water storage in each of the cases reached 311.58, 623.06 and 951.18 EUR·ha−1 respectively, and the total value of water stored in the analyzed stretch of the valley reached 2.52, 5.04 and 7.73 million EUR respectively (Table 3).
Table
Table 3. Selected monetary values of floodplain water storage.
Table 3. Selected monetary values of floodplain water storage.
Qp50% Flood duration [months]123
Average storage value [EUR·ha−1]311.58623.06951.18
Total value of water storage [M EUR]2.525.047.73

4. Discussion

As shown above, water storage volume of the floodplain is greater in magnitude than all of the reservoirs summed together in our approach. Also, in terms of economy, water storage on the floodplain remains much more efficient and potentially profitable than any of the analyzed reservoirs. Moreover, as in the hydrological and GIS study presented in this paper, we focused only on a certain portion of the Biebrza Valley, neglecting the larger part of it as well as the other floodplains located within the analyzed catchment. One can conclude that numbers (cubic meters and Euros) presented are in fact underestimated in the catchment scale. It is likely that the natural flood regime and the floodplain itself, left as an area for a natural flood processes, would provide much more valuable ecosystem services, i.e., serving as a refuge to rare birds [18] (bringing tourists and thus income), biotope for protected plant species (biodiversity) and productivity [27], serving as carbon sinks (reduction of CO2 & CH4 emissions), increasing self-purification of river waters and nutrient removal from the floodplain, and acting as a desirable habitat for fish spawning [28]—in general a diverse portfolio of ecosystem-based profits [29]. Therefore, we consider the role of flooded floodplain broader and much more positive than just agricultural damages (that can be calculated on the basis of regional economic indicators for agricultural production [30], which is claimed by the local farmers and authorities as the ultimate factor resulting from the floodplain function and negatively inducing regional development).
In cases of more dynamic floodplain systems than the analyzed Biebrza Valley, it is likely that other possibly more sophisticated methods of active storage capacity calculation (e.g. based on the temporal differentiation of inflow and outflow to/from the floodplain) could be applied. However, the simplified approach of floodplain water storage assessment presented herein on the basis of average water level of the flood event, seems robust enough for cases when the storage-generating flood duration exceeds some weeks (in the presented case the average duration of an overbank flow reached approximately 97 days per year, Table 2), being not very dynamic in time. The presented approach to hydrologic aspects of floodplain water storage volume can also be improved, if the relation between water levels and flood volumes/extents (Figure 5A,B) distinguished vary from seasonal conditions of vegetation development. This is because during the peak growth period (July–August) the same flood discharge can result in a much broader flood extent than within dormant periods. Consequently, the same flood extent in summer has a lower water storage capacity than in winter/spring conditions. Therefore, we state that in order to improve the presented approach, field research on the floodplain vegetation’s volume should be developed, possibly incorporating remote sensing and hydraulic modeling techniques.
The presented approach could be improved if the precise costs of analyzed artificial reservoir post-construction maintenance were known [parameter M in the Equation (1)]. Then, undoubtedly, unit value of water storage would increase. Either way, the results of our research bring us to the conclusion that once the monetary value of water storage is even preliminarily assessed within a certain region (preferably one hydrologic system of the river basin), it can serve as an index to calculate the value of water stored on a particular share of land, i.e., a plot of land possessed by one party. On the basis of our study and through the modification of algorithm Equation (3), where water storage in the whole floodplain FloVal was substituted with the volume of water stored on one particular plot (calculated as an area of the parcel multiplied by the average depth of water + consideration of water in the superficial layer of soil) in the given time Tf, one can robustly calculate the monetary value of water storage within a particular zone (e.g., on 1 ha of the floodplain meadow). If assumed, that a particular meadow of 1 ha of area was flooded for 1 month and the average water depth reached 0.1 m (conditions similar to the flood that occurred in summer 2011), and the remaining parameters such as the soil porosity and the critical depth remain constant to the ones presented herein, then the presented algorithms [Equations (2) and (3)] allow us to assess that the value of water stored in this meadow is 100.48 EUR. This value could be used in the calculation of economic donations for land users/owners, whose lands were flooded and are temporarily impossible to be maintained in an agricultural manner. Monetary value for water storage remains on a similar order of magnitude as other EU subsidies for meadows in protected areas (mentioned agro-environmental schemes). One can suspect that if the contemporarily implemented agro-environmental schemes include nowadays subsidies for sustaining biodiversity by means of active protection (mowing, shrub removal), it is likely that in the near future (sketched by the financing perspectives of any political or decision-making body) floodplain storage on the scale of one plot could also eventually be funded. In this context, the presented methodology appears useful in comprehensive water storage assessment in a catchment scale.
We stress that the given monetary value of water storage on unit area of land should not be considered as a substitute for flood-related losses. Presented values should rather become a side in the balanced calculations of whether to drain the area (which can only temporarily improve the productivity of a meadow) or to keep it as a sustainable and living floodplain. It is likely that even the vast drainage would never totally prevent the flooding of such broad and flat floodplains as the analyzed Biebrza Valley, as the amounts and dynamics of water remain in such systems a catchment-related phenomenon. Therefore, if water storage subsidies were implemented and the level of subsidies was calculated in relation to the catchment (and henceforth vary from catchment to catchment being adapted to local hydrological and economic conditions), they could (i) prevent further deterioration of ecosystems imposed by the pressure of drainage; (ii) improve the perception of floodplains, i.e., as an income-generating system, by local stakeholders.
The relatively easy calculation algorithm provided in our analysis can successfully be applied in other areas as nearly all of the data we use in our approach can be easily obtained. Such an analysis, resulting in comparative water storage valuation on the international scale, would likely become a starting point in an international discussion on funding the key ecosystem service of floodplain wetlands, which is water storage. The applied GIS and hydrological tools are robust enough to map the economic dimension of floodplain storage. The results of our approach can be transferred to the optimization of the valuation of lands in terms of ecology and economy by indicating the areas of the most significance for water storage. This way, the presented results can remain an important aspect of the decision-making process (e.g., in setting up protection goals in protected areas or by delineating the most problematic lands because of regular flooding or high income potential, if water storage is conceived in economic terms). Such an approach is required nowadays in ecologic-economic assessments of land and processes, and remains a solid base for sustainable regional development policies [31,32,33,34] or any modern environmental management at the country level [35].
The authors are aware that the presented approach does not distinguish floodplain water storage and reservoirs on the level of functionality (water storage in artificial reservoirs can be regulated efficiently and remains adjustable to the current hydrologic situation; floodplains cannot). However, it is unlikely that the storage ponds of the kind analyzed here can play any important role in flood risk mitigation, as their total volume is relatively small. In this regard, the floodplain acts as one homogeneous active storage reservoir, despite the fact that the inflow/outflow to/from the floodplain is controlled mainly by natural processes. Therefore, the role of the floodplain in flood mitigation and water storage of this natural system is much more significant than the small storage reservoirs randomly distributed within a catchment.
The importance of floodplain dynamics and its interface with ecosystems and agriculture has increased recently due to the changing climate [36,37]. It is to be expected that the variability of floods in the Biebrza Valley is likely to increase, and summer floods are projected to be more frequent than at any time before [8]. This trend, observed over the last 60 years [7], results from increasing polarization of summer precipitation—longer periods of drought are interrupted by short and intensive (~30 mm·day−1) rainfalls. This process, resulting from the changing climate is considered as a driver of increasing social pressure aimed at the drainage. In this context, proposed subsidies for flooded meadows can remain the ultimate measure of management adaptation to a changing climate, and also an easy to apply activity aimed at mitigation of negative climate-induced pressures to the regional economy.
The presented results, if built up on the whole sequence of other services provisioned by floods within the semi-natural floodplains, can also be useful in studies on areas with heavily modified flood regimes (e.g., downstream large dams which flatten the discharge hydrograph). The unraveled economic benefits that come along with floods in near-natural, extensively used floodplains highlight the importance of floodplain dynamics and water storage for regional societies [38]. One can suspect that the dispersion of knowledge on more than just the ecological benefits that come along with well-preserved floodplain ecosystems and flood phenomena, including economic assessment of the floodplain storage, can bridge the economic development and environmental conservation of valuable ecosystems by sustainable water management and preservation of those unique habitats [4,5]. Certainly the enhancement of positive social perception of floods and the development of so-called “flood benefits” for farmers that maintain regularly flooded meadows can make water management goals claimed by the agriculture and environmental conservation feed back again through the economy. Hopefully, once the economic dimension of water stored during floods within the agriculturally maintained floodplains is unraveled by stakeholders, the threat of drainage of unique wetland habitats will be reduced. Hence, it is likely that the continuation of research on economic aspects of floodplain water storage in agricultural landscapes of high natural value can entail real integration in water management, making this concept—hopefully—to work better than at any time before [39].

5. Conclusions

The applied algorithm of economic assessment of water storage in a catchment scale, based on the economic analysis of water storage in artificial reservoirs, allows us to quantify the unit monetary value of water storage in the Biebrza River Basin that reached 0.53 EUR·m−3·year−1. Concerning the presented assumptions as to the hydrological processes, the average annual active water storage capacity of the floodplain in the Lower Biebrza Basin in years 1995–2011 equaled 10.36 M m3·year−1. The average annual monetary value of water stored in the analyzed floodplain, calculated according to the presented assumptions, reached EUR 5.49 million year−1 and varied from approximatelyEUR 1 million year−1 up to nearly EUR 14 million year−1, depending on hydrological conditions. Average annual water storage capacity of the floodplain is of the same order of magnitude as the biggest artificial reservoirs in this part of the country. We conclude that the active storage capacity of the floodplain plays a key role in flood mitigation, remaining much more important than the small reservoirs constructed to increase the storage capacity in a catchment scale considered in our analysis. If economic and hydrological criteria were considered in the efficiency assessment of water storage in a catchment scale of the presented river system, then conservation of floodplain wetlands appears to be much more efficient than construction of small water storage ponds in terms of economy, flood protection, and environmental conservation.
The presented methodology, although simplified from the point of view of dynamic flood processes and the economic aspects of water storage in a catchment scale, has proven to be robust enough in lowland systems and could be considered as a starting point for the discussions on possible implementation of EU-funds-supported “water storage subsidies” for ecologically valuable areas of floodplains (e.g., covered by the Natura 2000 program and areas of National Parks). If the water storage process on agriculturally managed areas of meadows was granted in terms of economy by the proposed subsidies, than (i) the conservation of floodplain ecosystems would benefit from the positive feedback of local stakeholders and (ii) the ultimate deterioration of remaining natural floodplains can be slowed. Either way, it seems that the presentation of water storage on wetlands in a strong economic context allows for the integration of goals of environmental conservation, water management, and agriculture. Bearing in mind the limitations of the water trade-off approaches, one can conclude that the herein presented integrated-colored water footprint [40] will at least focus the attention of stakeholders on water storage of agriculturally maintained semi-natural lowland floodplains, as an economically quantifiable service.

Acknowledgments

Field validation of modeled flood extents was done in the framework of the HABIT-CHANGE 2CE168P3 Project, implemented by the Biebrza National Park through the CENTRAL EUROPE Programme, co-financed by the European Regional Development Fund. Michał Fabiszewski, Grzegorz Kwiatkowski and Adam Szarnecki from the Biebrza National Park are acknowledged for help in field research. We thank editors and two anonymous reviewers for their useful comments.

Conflicts of Interest

The authors declare no conflict of interest.

References

  1. Acreman, M.C. Wetlands and Water Storage: Current and Future Trends and Issues; Ramsar Convention Secretariat: Gland, Switzerland, 2012. [Google Scholar]
  2. Blackwell, M.S.A.; Maltby, E. Ecoflood Guidelines: How to Use Floodplains for Flood Risk Reduction; 4 European Commission D.G. Research: Brussels, Belgium, 2006. [Google Scholar]
  3. Maltby, E. Functional Assessment of Wetlands: Towards Evaluation of Ecosystem Services; Woodhead Publishing Ltd.: Cambridge, UK, 2009; p. 672. [Google Scholar]
  4. Turner, R.K.; van den Bergh, J.C.J.M.; Soderqvist, T.; Barendregt, A.; van der Straaten, J.; Maltby, E.; van Ierland, E.C. Ecological-economic analysis of wetlands: Scientific integration for management and policy. Ecol. Econ. 2000, 35, 7–23. [Google Scholar] [CrossRef]
  5. Webler, T.; Kastenholz, H.; Renn, O. Public participation in impact assessment: A social learning perspective. Environ. Impact Assess. 1995, 15, 443–463. [Google Scholar] [CrossRef]
  6. Joosten, H.; Clarke, D. Wise Use of Mires and Peatlands—Background and Principles Including a Framework for Decision Making; IMCG Cooperation, International Peat Society (IPS): Saarijarvi, Finland, 2002. [Google Scholar]
  7. Grygoruk, M.; Mirosław-Świątek, D.; Kardel, I.; Okruszko, T.; Michałowski, R.; Kwiatkowski, G. Analysis of Past-Present Hydrological Phenomena of the Biebrza Valley; Report 4.4.2. for HABIT-CHANGE Project 2CE168P3; Biebrza National Park: Osowiec-Twierdza, Poland, 5 December 2011; p. 29. Available online: http://www2.ioer.de/download/habit-change/HABIT-CHANGE_4_4_2%20hydrologic_phenomena.pdf (accessed on 14 July 2013).
  8. Grygoruk, M.; Biereżnoj-Bazille, U.; Mazgajski, M.; Sienkiewicz, J. Climate-Induced Challenges for Wetlands: Revealing the Background for Adaptive Management of Ecosystems in the Biebrza Valley, Poland. In 58th Advances in Global Change Research, 58, Managing Protected Areas in Central And Eastern Europe Under Climate Change; Rannow, S., Neubert, M., Eds.; Springer: Berlin-Heidelberg, Germany, 2014; ISBN ISBN 978-94-007-7959-4. (in press) [Google Scholar]
  9. Mioduszewski, W.; Okruszko, T. Protection of natural wetlands—The examples of conflicts. J. Water Land Dev. 2012, 16, 35–42. [Google Scholar]
  10. Jabłońska, E.; Kotkowicz, M.; Manewicz, M.; Nawrocki, P.; Pawlaczyk, P. Inventory and Environmental Assessment of River Training in Łódzkie, Podkarpackie, Małopolskie, Świętokrzyskie, Warmińsko-Mazurskie, Zachodniopomorskie, Opolskie, Wielkopolskie, Mazowieckie i Podlaskie Regions in 2010–2012…; (in Polish). WWF Poland report on River Conservation in Poland: Warsaw, Poland, 17 April 2013; p. 124. Available online: http://awsassets.wwfpl.panda.org/downloads/raport_wwf_polska_prace_utrzymaniowe.pdf (accessed on 16 July 2013).
  11. Regional board for drainage and irrigation in Białystok (Wojewódzki zarząd melioracji i urządzeń wodnych w Białymstoku). In Programme of Irrigation for Podlaskie Voivodeship for Years 2007–2013; (in Polish). Wojewódzki Zarząd Melioracji i Urządzeń Wodnych: Białystok, Polish, 2008; p. 76.
  12. Biswas, A.K.; Tortajada, C. Appraising the Concept of Sustainable Development: Water Management and Related Environmental Challenges; Oxford University Press: Oxford, UK, 2004; p. 223. [Google Scholar]
  13. McDonnell, R. Challenges for integrated water resources management: How do we provide the knowledge to support truly integrated thinking? Int. J. Water Resour. Dev. 2008, 24, 131–143. [Google Scholar] [CrossRef]
  14. Wassen, M.J.; Okruszko, T.; Kardel, I.; Chormański, J.; Świątek, D.; Mioduszewski, W.; Bleuten, W.; Querner, E.P.; El Kahloun, M.; Batelaan, O.; et al. Eco-Hydrological Functioning of the Biebrza Wetlands: Lessons for the Conservation and Restoration of Deteriorated Wetlands. In Wetlands: Functioning, Biodiversity Conservation and Restoration; Bobbink, R., Beltman, B., Verhoeven, J.T.A., Wigham, D.F., Eds.; Springer-Verlag: Berlin, Germany, 2006; pp. 285–310. [Google Scholar]
  15. Ignar, S.; Maksymiuk-Dziuban, A.; Mirosław-Świątek, D.; Chormański, J.; Okruszko, T.; Wysocki, P. Temporal variability of selected flood parameters in the Biebrza River valley. Ann. Warsaw Univ. Life Sci. SGGW 2011, 43, 135–142. [Google Scholar]
  16. Chormański, J.; Okruszko, T.; Ignar, S.; Batelaan, O.; Rebel, K.T.; Wassen, M.J. Flood mapping with remote sensing and hydrochemistry: A new method to distinguish the origin of flood water during floods. Ecol. Eng. 2011, 37, 1334–1349. [Google Scholar] [CrossRef]
  17. Byczkowski, A.; Kiciński, T. Frequency and duration of floods in the Biebrza river valley. Zesz. Probl. Post. Nauk Roln. 1983, 255, 75–87. (in Polish). [Google Scholar]
  18. Polakowski, M.; Broniszewska, M.; Jankowiak, Ł.; Ławicki, Ł.; Siuchno, M. Numbers and dynamics of spring migration of geese in the Biebrza Basin. Ornis Pol. 2011, 52, 169–180. [Google Scholar]
  19. Tyszka, J. Estimation and economic valuation of the forest retention capacities. J. Water Land Dev. 2009, 13, 149–159. [Google Scholar]
  20. Świątek, D.; Chormański, J. The Verification of the Numerical River Flow Model by Use of Remote Sensing. In Wetlands: Monitoring, Modelling and Management; Okruszko, T., Maltby, E., Szatyłowicz, J., Świątek, D., Kotowski, W., Eds.; Taylor & Francis Group: London, UK, 2007. [Google Scholar]
  21. Świątek, D.; Szporak, S.; Chormański, J.; Okruszko, T. Hydrodynamic model of the Lower Biebrza River flow—A tool for assessing the hydrologic vulnerability of a floodplain to management practices. Ecohydrol. Hydrobiol. 2008, 2–4, 331–337. [Google Scholar]
  22. Chormański, J.; Mirosław-Świątek, D.; Michałowski, R. A hydrodynamic model coupled with GIS for flood characteristics in the Biebrza riparian wetland. Oceanol. Hydrobiol. St. 2009, 38, 65–73. [Google Scholar]
  23. Mirosław-Świątek, D.; Utratna, M. Automatic system of registration of water level on floodplains in the lower basin of Biebrza river. Sci. Rev. Eng. Environ. Sci. 2012, 21, 20–32. [Google Scholar]
  24. Kaczmarek, Z.; Trykozko, E. Application of the method of quantiles of estimation of the Pearson distribution. Acta Geoph. Polon. 1964, 12, 5–12. [Google Scholar]
  25. Chrzanowska, W. Evaluation of the Surface Water Storage in the Lower Biebrza Basin. Ph.D. Thesis, Warsaw University of Life Sciences—SGGW, Warsaw, Poland, 15 February 2013. [Google Scholar]
  26. Szporak, S.; Mirosław-Świątek, D.; Chormański, J. The flood extent in the Lower Biebrza Basin calculated by the 1D flow model for different land use scenarios. Ann. Warsaw Univ. Life Sci. SGGW 2008, 40, 45–54. [Google Scholar]
  27. Gerard, M.; El Kahloun, M.; Mertens, W.; Verhagen, B.; Meire, P. Impact of flooding on potential and realised grassland species richness. Plant Ecol. 2008, 194, 85–98. [Google Scholar]
  28. Junk, W.J.; Bayley, P.B.; Sparks, R.E. The flood pulse concept in river-floodplain systems. Can. Spec. Publ. Fish. Aquat. Sci. 1989, 106, 110–127. [Google Scholar]
  29. Okruszko, T.; Duel, H.; Acreman, M.; Grygoruk, M.; Flörke, M.; Schneider, C. Broad scale ecosystem services of European wetlands—Overview of the current situation and future perspectives under different climate and water management scenarios. Hydrol. Sci. J. 2011, 53, 1501–1517. [Google Scholar]
  30. Rychłowski, A. Analysis of Variable Costs of Production. (in Polish). Available online: http://ksow.pl/fileadmin/user_upload/podlaskie/pliki/Analiza_zmiennych_koszt%C3%B3w_produkcji.pdf (accessed on 25 May 2013).
  31. Burkhard, B.; Crossman, N.; Nedkov, S.; Petz, K.; Alkemade, R. Mapping and modelling ecosystem services for science, policy and practice. Ecosyst. Serv. 2013, 2, 1–3. [Google Scholar]
  32. Maes, J.; Egoh, B.; Willemen, L.; Liquete, C.; Vihervaara, P.; Schagner, J.P.; Grizzetti, B.; Drakou, E.G.; Notte, A.L.; Zulian, G.; et al. Mapping ecosystem services for policy support and decision making in the European Union. Ecosyst. Serv. 2012, 1, 31–39. [Google Scholar] [CrossRef]
  33. Sullivan, T.J. Combining ecosystem service and critical load concepts for resources management and public policy. Water 2012, 4, 905–913. [Google Scholar] [CrossRef]
  34. Bateman, I.J.; Harwood, A.R.; Mace, G.M.; Watson, R.T.; Abson, D.J.; Andrews, B.; Binner, A.; Crowe, A.; Day, B.H.; Dugdale, S.; et al. Bringing ecosystem services into economic decision-making: Land use in the United Kingdom. Science 2013, 341, 45–50. [Google Scholar] [CrossRef]
  35. Lawton, R.N.; Rudd, M.A. Strange bedfellows: Ecosystem services, conservation science, and central government in the United Kingdom. Resources 2013, 2, 114–127. [Google Scholar] [CrossRef]
  36. Karamouz, M.; Noori, N.; Moridi, A.; Ahmadi, A. Evaluation of floodplain variability, considering impacts of climate change. Hydrol. Process. 2010, 25, 90–103. [Google Scholar] [CrossRef]
  37. Schneider, C.; Flörke, M.; Gerling, G.; Duel, H.; Grygoruk, M.; Okruszko, T. The future of European floodplain wetlands under a changing climate. J. Water Clim. Chang. 2011, 2–3, 106–122. [Google Scholar]
  38. Richter, B.D.; Thomas, G.A. Restoring environmental flows by modifying dam operations. Ecol. Soc. 2007, 12. Article 12. [Google Scholar]
  39. Biswas, A.K. Integrated water resources management—Is it working? Int. J. Water Resour. Dev. 2008, 1, 5–22. [Google Scholar] [CrossRef]
  40. Hoekstra, A.Y.; Chapagain, A.K.; Aldaya, M.M.; Mekonnen, M.M. The Water Footprint Assessment Manual: Setting the Global Standard; Water Footprint Network, Earthscan Ltd.: London, UK; Washington, USA, 2011; p. 228. Available online: http://www.waterfootprint. org/downloads/TheWaterFootprintAssessmentManual.pdf (accessed on 20 July 2013).

Share and Cite

MDPI and ACS Style

Grygoruk, M.; Mirosław-Świątek, D.; Chrzanowska, W.; Ignar, S. How Much for Water? Economic Assessment and Mapping of Floodplain Water Storage as a Catchment-Scale Ecosystem Service of Wetlands. Water 2013, 5, 1760-1779. https://doi.org/10.3390/w5041760

AMA Style

Grygoruk M, Mirosław-Świątek D, Chrzanowska W, Ignar S. How Much for Water? Economic Assessment and Mapping of Floodplain Water Storage as a Catchment-Scale Ecosystem Service of Wetlands. Water. 2013; 5(4):1760-1779. https://doi.org/10.3390/w5041760

Chicago/Turabian Style

Grygoruk, Mateusz, Dorota Mirosław-Świątek, Weronika Chrzanowska, and Stefan Ignar. 2013. "How Much for Water? Economic Assessment and Mapping of Floodplain Water Storage as a Catchment-Scale Ecosystem Service of Wetlands" Water 5, no. 4: 1760-1779. https://doi.org/10.3390/w5041760

APA Style

Grygoruk, M., Mirosław-Świątek, D., Chrzanowska, W., & Ignar, S. (2013). How Much for Water? Economic Assessment and Mapping of Floodplain Water Storage as a Catchment-Scale Ecosystem Service of Wetlands. Water, 5(4), 1760-1779. https://doi.org/10.3390/w5041760

Article Metrics

Back to TopTop