Multilayer Substrate Configuration Enhances Removal Efficiency of Pollutants in Constructed Wetlands
Abstract
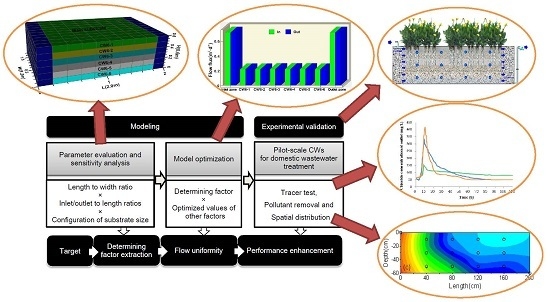
:1. Introduction
2. Materials and Methods
2.1. Modeling
2.1.1. Model Domain Setup and Calculation
2.1.2. Model Evaluation
2.1.3. Model Optimization
2.2. Experimental Validation
2.2.1. Experimental System Setup
2.2.2. Water Sampling and Measurement
2.2.3. Tracer Test
2.3. Statistical Analyses
3. Results and Discussion
3.1. Effects of Design Parameters on Water Flow Distribution
3.2. Model Optimization
3.3. Experimental Validation
3.3.1. Hydraulic Performance
3.3.2. Overall Pollutant Removal Efficiencies
3.3.3. Water Quality and Spatial Distribution of Pollutants
4. Conclusions
Acknowledgments
Author Contributions
Conflicts of Interest
Appendix A
| Models | Length to Width Ratios | Inlet/Outlet to Length Ratios | Configurations of Substrate Size, K (m/Day) (Listed in Order of Surface to Bottom Layers) |
|---|---|---|---|
| Model 1 | 1:1 | 1:5 | monolayer, K = 200 |
| Model 2 | 1:1 | 1:5 | three-layers, K = 50, 100 and 200 |
| Model 3 | 1:1 | 1:5 | three-layers, K = 200, 100 and 50 |
| Model 4 | 1:1 | 1:10 | monolayer, K = 200 |
| Model 5 | 1:1 | 1:10 | three-layers, K = 50, 100 and 200 |
| Model 6 | 1:1 | 1:10 | three-layers, K = 200, 100 and 50 |
| Model 7 | 1:1 | 1:20 | monolayer, K = 200 |
| Model 8 | 1:1 | 1:20 | three-layers, K = 50, 100 and 200 |
| Model 9 | 1:1 | 1:20 | three-layers, K = 200, 100 and 50 |
| Model 10 | 2:1 | 1:5 | monolayer, K = 200 |
| Model 11 | 2:1 | 1:5 | three-layers, K = 50, 100 and 200 |
| Model 12 | 2:1 | 1:5 | three-layers, K = 200, 100 and 50 |
| Model 13 | 2:1 | 1:10 | monolayer, K = 200 |
| Model 14 | 2:1 | 1:10 | three-layers, K = 50, 100 and 200 |
| Model 15 | 2:1 | 1:10 | three-layers, K = 200, 100 and 50 |
| Model 16 | 2:1 | 1:20 | monolayers, K = 200 |
| Model 17 | 2:1 | 1:20 | three-layers, K = 50, 100 and 200 |
| Model 18 | 2:1 | 1:20 | three-layers, K = 200, 100 and 50 |
| Model 19 | 3:1 | 1:5 | monolayer, K = 200 |
| Model 20 | 3:1 | 1:5 | three-layers, K = 50, 100 and 200 |
| Model 21 | 3:1 | 1:5 | three-layers, K = 200, 100 and 50 |
| Model 22 | 3:1 | 1:10 | monolayer, K = 200 |
| Model 23 | 3:1 | 1:10 | three-layers, K = 50, 100 and 200 |
| Model 24 | 3:1 | 1:10 | three-layers, K = 200, 100 and 50 |
| Model 25 | 3:1 | 1:20 | monolayer, K = 200 |
| Model 26 | 3:1 | 1:20 | three-layers, K = 50, 100, and 200 |
| Model 27 | 3:1 | 1:20 | three-layers, K = 200, 100 and 50 |
References
- Brix, H.; Schierup, H.H.; Arias, C.A. Twenty years experience with constructed wetland systems in Denmark—What did we learn? Water Sci. Technol. 2007, 56, 63–68. [Google Scholar] [CrossRef] [PubMed]
- Vymazal, J. The use of sub-surface constructed wetlands for wastewater treatment in the Czech Republic: 10 years experience. Ecol. Eng. 2002, 18, 633–646. [Google Scholar] [CrossRef]
- Ríosa, D.A.; Vélez, F.T.A.; Pena, M.R.; Parra, C.A.M. Changes of flow patterns in a horizontal subsurface flow constructed wetland treating domestic wastewater in tropical regions. Ecol. Eng. 2009, 35, 274–280. [Google Scholar] [CrossRef]
- Brovelli, A.; Carranza-Diaz, O.; Rossi, L.; Barry, D.A. Design methodology accounting for the effects of porous medium heterogeneity on hydraulic residence time and biodegradation in horizontal subsurface flow constructed wetlands. Ecol. Eng. 2011, 37, 758–770. [Google Scholar] [CrossRef]
- Knowles, P.R.; Griffin, P.; Davies, P.A. Complementary methods to investigate the development of clogging within a horizontal sub-surface flow tertiary treatment wetland. Water Res. 2010, 44, 320–330. [Google Scholar] [CrossRef] [PubMed]
- Knowles, P.; Dotro, G.; Nivala, J.; García, J. Clogging in subsurface-flow treatment wetlands: Occurrence and contributing factors. Ecol. Eng. 2011, 37, 99–112. [Google Scholar] [CrossRef]
- Pedescoll, A.; Sidrach-Cardona, R.; Sa’nchez, J.C.; Carretero, J.; Garfi, M.; Be’cares, E. Design configurations affecting flow pattern and solids accumulation in horizontal free water and subsurface flow constructed wetlands. Water Res. 2013, 47, 1448–1458. [Google Scholar] [CrossRef] [PubMed]
- Morales, L.P.; Franco, M.; Garvi, D.; Lebrato, J. Influence of the stone organization to avoid clogging in horizontal subsurface-flow treatment wetlands. Ecol. Eng. 2013, 54, 136–144. [Google Scholar] [CrossRef]
- García, J.; Aguirre, P.; Barragán, J.; Mujeriego, R.; Matamoros, V.; Bayona, J.M. Effect of key design parameters on the efficiency of horizontal subsurface flow constructed wetlands. Ecol. Eng. 2005, 25, 405–418. [Google Scholar] [CrossRef]
- García, J.; Chiva, J.; Aguirre, P.; lvarez, E.A.; Sierra, J.P.; Mujeriego, R. Hydraulic behaviour of horizontal subsurface flow constructed wetlands with different aspect ratio and granular medium size. Ecol. Eng. 2004, 23, 177–187. [Google Scholar] [CrossRef]
- Kadlec, R.H.; Wallace, S. Treatment Wetlands; CRC Lewis Regional Relevance: Boca Raton, FL, USA, 2008. [Google Scholar]
- Suliman, F.; Futsaether, C.; Oxaal, U.; Haugen, L.E.; Jenssen, P. Effect of the inlet–outlet positions on the hydraulic performance of horizontal subsurface-flow wetlands constructed with heterogeneous porous media. J. Contam. Hydrol. 2006, 87, 22–36. [Google Scholar] [CrossRef] [PubMed]
- Liolios, K.A.; Moutsopoulos, K.N.; Tsihrintzis, V.A. Modeling of flow and bod fate in horizontal subsurface flow constructed wetlands. Chem. Eng. J. 2012, 200–202, 681–693. [Google Scholar] [CrossRef]
- Ronkanen, A.K.; Kløve, B. Hydraulics and flow modelling of water treatment wetlands constructed on peatlands in Northern Finland. Water Res. 2008, 42, 3826–3836. [Google Scholar] [CrossRef] [PubMed]
- Harbaugh, A. Modflow-2005, the US geological survey modular ground-water model—The ground-water flow process. In US Geological Survey Techniques of Water Resources Investigation Book; U.S. Department of the Interior, U.S. Geological Survey: Washington, DC, USA, 2005. [Google Scholar]
- Persson, J.; Somes, N.L.G.; Wong, T.H.F. Hydraulics efficiency of constructed wetlands and ponds. Water Sci. Technol. 1999, 40, 291–300. [Google Scholar] [CrossRef]
- Alcocer, D.J.R.; Vallejos, G.G.; Champagne, P. Assessment of the plug flow and dead volume ratios in a sub-surface horizontal-flow packed-bed reactor as a representative model of a sub-surface horizontal constructed wetland. Ecol. Eng. 2012, 40, 18–26. [Google Scholar] [CrossRef]
- Pedescoll, A.; Uggetti, E.; Llorens, E.; Granés, F.; García, D.; García, J. Practical method based on saturated hydraulic conductivity used to assess clogging in subsurface flow constructed wetlands. Ecol. Eng. 2009, 35, 1216–1224. [Google Scholar] [CrossRef]
- Schincariol, R.A.; Schwartz, F.W. An experimental investigation of variable density flow mixing in homogeneous and heterogeneous media. Water Resour. Res. 1990, 26, 2317–2329. [Google Scholar] [CrossRef]
- Lightbody, A.F.; Nepf, H.M.; Baysb, J.S. Modeling the hydraulic effect of transverse deep zones on the performance of short-circuiting constructed treatment wetlands. Ecol. Eng. 2009, 35, 754–768. [Google Scholar] [CrossRef]
- Suliman, F.; French, H.K.; Haugen, L.E.; Søvik, A.K. Change in flow and transport patterns in horizontal subsurface flow constructed wetlands as a result of biological growth. Ecol. Eng. 2006, 27, 124–133. [Google Scholar] [CrossRef]
- Jiang, C.; Jia, L.; He, Y.; Zhang, B.; Kirumba, G.; Xie, J. Adsorptive removal of phosphorus from aqueous solution using sponge iron and zeolite. J. Colloid Interface Sci. 2013, 402, 246–252. [Google Scholar] [CrossRef] [PubMed]
- Tuszynska, A.; Kolecka, K.; Quant, B. The influence of phosphorus fractions in bottom sediments on phosphate removal in semi-natural systems as the 3rd stage of biological wastewater treatment. Ecol. Eng. 2013, 53, 321–328. [Google Scholar] [CrossRef]
- Vohla, C.; Kõiv, M.; Bavor, H.J.; Chazarenc, F.; Mander, Ü. Filter materials for phosphorus removal from wastewater in treatment wetlands—A review. Ecol. Eng. 2011, 37, 70–89. [Google Scholar] [CrossRef]
- Dunne, E.J.; Coveney, M.F.; Marzolf, E.R.; Hoge, V.R.; Conrow, R.; Naleway, R.; Lowe, E.F.; Battoe, L.E. Efficacy of a large-scale constructed wetland to remove phosphorus and suspended solids from Lake Apopka, Florida. Ecol. Eng. 2012, 42, 90–100. [Google Scholar] [CrossRef]









| Variable | L | I | S | L × I | L × S | I × S |
|---|---|---|---|---|---|---|
| Layer 1 | 109 * | 378 * | 430 * | 0.5 | 0.7 | 3 |
| Layer 2 | 42 * | 507 ** | 183 * | 1 * | 16 * | 8 * |
| Layer 3 | 215 * | 248 * | 300 * | 22 * | 15 * | 14 * |
| Layer 4 | 163 * | 56 * | 173 * | 10 * | 8 * | 8 * |
| Layer 5 | 202 * | 17 * | 394 * | 6 * | 26 * | 3 |
| Layer 6 | 2526 * | 29 * | 3376 * | 4 | 315 * | 5 * |
© 2016 by the authors; licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC-BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Bai, S.; Lv, T.; Ding, Y.; Li, X.; You, S.; Xie, Q.; Brix, H. Multilayer Substrate Configuration Enhances Removal Efficiency of Pollutants in Constructed Wetlands. Water 2016, 8, 556. https://doi.org/10.3390/w8120556
Bai S, Lv T, Ding Y, Li X, You S, Xie Q, Brix H. Multilayer Substrate Configuration Enhances Removal Efficiency of Pollutants in Constructed Wetlands. Water. 2016; 8(12):556. https://doi.org/10.3390/w8120556
Chicago/Turabian StyleBai, Shaoyuan, Tao Lv, Yanli Ding, Xuefen Li, Shaohong You, Qinglin Xie, and Hans Brix. 2016. "Multilayer Substrate Configuration Enhances Removal Efficiency of Pollutants in Constructed Wetlands" Water 8, no. 12: 556. https://doi.org/10.3390/w8120556
APA StyleBai, S., Lv, T., Ding, Y., Li, X., You, S., Xie, Q., & Brix, H. (2016). Multilayer Substrate Configuration Enhances Removal Efficiency of Pollutants in Constructed Wetlands. Water, 8(12), 556. https://doi.org/10.3390/w8120556