Friction and Temperature Behavior of Lubricated Thermoplastic Polymer Contacts
Abstract
:1. Introduction
2. Experimental Setup
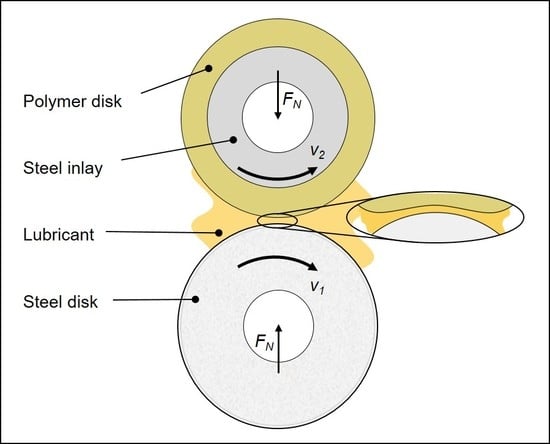
2.1. Twin-Disk Test Rig and Specimen
2.2. Operating Conditions and Experimental Procedure
3. Results and Discussion
3.1. Temperature Behavior in Load Sequences
3.2. Friction and Temperature Behavior in Slip Curves
3.2.1. Influence of the Matrix Material
3.2.2. Influence of the Lubricant
3.2.3. Influence of the Oil Temperature
3.2.4. Influence of the Load
3.2.5. Influence of the Disk Setup
3.2.6. Influence of Glass Fiber Reinforcement
3.2.7. Surface Impressions
4. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Nomenclature
| E | Tensile modulus, N/mm2 |
| Friction force, N | |
| Normal force, N | |
| Minimal film thickness, µm | |
| Measuring distance of the roughness measurement, mm | |
| PA66 | Polyamide 66 |
| PA66+GF30 | Polyamide 66 with 30 wt.-% glass fibers |
| PEEK | Polyetheretherketone |
| PEEK+GF30 | Polyetheretherketone with 30 wt.-% glass fibers |
| Volume flow, l/min | |
| Arithmetic mean roughness, µm | |
| Maximum height, µm | |
| s | Slip rate, % |
| Sliding velocity, m/s | |
| Sum velocity, m/s | |
| Surface velocity (lower disk), m/s | |
| Surface velocity (upper disk), m/s | |
| Glass transition temperature, °C | |
| Initial bulk temperature, °C | |
| Bulk temperature, °C | |
| Oil temperature, °C | |
| Coefficient of friction | |
| Oil kinematic viscosity, | |
| Cut-off wavelength, mm | |
| Relative film thickness |
References
- Erhard, G. Konstruieren mit Kunststoffen, 4th ed.; Carl Hanser Verlag: München, Wien, Germany, 2004. [Google Scholar]
- Ehrenstein, G.W. Mit Kunststoffen konstruieren: Eine Einführung, 3rd ed.; Carl Hanser Verlag: München, Germany, 2007. [Google Scholar]
- Hasl, C.; Illenberger, C.; Oster, P.; Tobie, T.; Stahl, K. Potential of oil-lubricated cylindrical plastic gears. J. Adv. Mech. Des. Syst. Manuf. 2018, 12, 1–9. [Google Scholar] [CrossRef] [Green Version]
- Illenberger, C.; Tobie, T.; Stahl, K. Flank load carrying capacity of oil-lubricated high performance plastic gears—Analysis of the pitting development in back-to-back tests. In Proceedings of the International Conference on Gears, Garching, Germany, 18–20 September 2019. [Google Scholar]
- Bartel, D. Simulation von Tribosystemen: Grundlagen und Anwendungen; Vieweg+Teubner: Wiesbaden, Germany, 2010. [Google Scholar]
- Maier, E.; Ziegltrum, A.; Lohner, T.; Stahl, K. Characterization of TEHL contacts of thermoplastic gears. Forsch. Ing. 2017, 81, 317–324. [Google Scholar] [CrossRef]
- Vincente, J.; Stokes, J.R.; Spikes, H.A. The frictional properties of Newtonian fluids in rolling–sliding soft-EHL contact. Tribol. Lett. 2005, 20, 273–286. [Google Scholar] [CrossRef]
- Myers, T.G.; Hall, R.W.; Savage, M.D.; Gaskell, P. The Transition Region of Elastohydrodynamic Lubrication. Proc. Math. Phys. Sci. 1991, 432, 469–479. [Google Scholar]
- Dearn, K.D.; Hoskins, T.J.; Andrei, L.; Walton, D. Lubrication Regimes in High-Performance Polymer Spur Gears. Adv. Tribol. 2013, 2013, 1–9. [Google Scholar] [CrossRef] [Green Version]
- Hamrock, B.J.; Dowson, D. Elastohydrodynamic Lubrication of Elliptical Contacts for Materials of Low Elastic Modulus I - Fully Flooded Conjunction. Trans. ASME 1978, 100, 236–245. [Google Scholar] [CrossRef]
- Myant, C.; Spikes, H.A.; Stokes, J.R. Influence of load and elastic properties on the rolling and sliding friction of lubricated compliant contacts. Tribol. Int. 2010, 43, 55–63. [Google Scholar] [CrossRef]
- Stupkiewicz, S.; Lengiewicz, J.; Sadowski, P.; Kucharski, S. Finite deformation effects in soft elastohydrodynamic lubrication problems. Tribol. Int. 2016, 93, 511–522. [Google Scholar] [CrossRef]
- Lin, W.; Kampf, N.; Klein, J. Designer Nanoparticles as Robust Superlubrication Vectors. ACS Nano 2020, 14, 7008–7017. [Google Scholar] [CrossRef]
- Yaniv, R.; Koren, E. Robust Superlubricity of Gold–Graphite Heterointerfaces. Adv. Funct. Mater. 2019, 30, 1901138. [Google Scholar] [CrossRef]
- Baykara, M.Z.; Vazirisereshk, M.R.; Martini, A. Emerging superlubricity: A review of the state of the art and perspectives on future research. Appl. Phys. Rev. 2018, 5, 041102. [Google Scholar] [CrossRef] [Green Version]
- Persson, B.N.J.; Scaraggi, M. On the transition from boundary lubrication to hydrodynamic lubrication in soft contacts. J. Phys. Condens. Matter 2009, 21, 1–22. [Google Scholar] [CrossRef]
- Duan, Y.; Liu, Y.; Li, J.; Feng, S.; Wen, S. AFM Study on Superlubricity between Ti6Al4V/Polymer Surfaces Achieved with Liposomes. Biomacromolecules 2019, 20, 1522–1529. [Google Scholar] [CrossRef] [PubMed]
- Lindner, M.; Sextro, W.; Popp, K. Hysteretic Friction of a Sliding Rubber Element. Proc. Appl. Math. Mech. 2004, 4, 101–102. [Google Scholar] [CrossRef]
- Putignano, C.; Reddyhoff, T.; Dini, D. The influence of temperature on viscoelastic friction properties. Tribol. Int. 2016, 100, 338–343. [Google Scholar] [CrossRef] [Green Version]
- Hooke, C.J.; Huang, P. Elastohydrodynamic lubrication of soft viscoelastic materials in line contact. Proc. Inst. Mech. Eng. 1997, 221, 185–194. [Google Scholar] [CrossRef]
- Putignano, C.; Reddyhoff, T.; Carbone, G.; Dini, D. Experimental Investigation of Viscoelastic Rolling Contacts: A Comparison with Theory. Tribol. Lett. 2013, 51, 105–113. [Google Scholar] [CrossRef]
- Snoeijer, J.H.; Eggers, J.; Venner, C.H. Similarity theory of lubricated Hertzian contacts. Phys. Fluids 2013, 25, 101705. [Google Scholar] [CrossRef] [Green Version]
- Putignano, C.; Dini, D. Soft Matter Lubrication: Does Solid Viscoelasticity Matter? Appl. Mater. Interfaces 2017, 9, 42287–42295. [Google Scholar] [CrossRef] [Green Version]
- Lohner, T.; Merz, R.; Mayer, J.; Michaelis, K.; Kopnarski, M.; Stahl, K. On the Effect of Plastic Deformation (PD) Additives in Lubricants. Tribol. + Schmier. 2015, 62, 13–24. [Google Scholar]
- Doll, N.; Verdesca, A.; Bastos, E.; Osswald, T.; Kleiss, R. Methodology for quasi-viscoelastic simulation of polymer gears made from PEEK using Ansys. In Proceedings of the ANTEC, Orlando, FL, USA, 23–25 March 2015. [Google Scholar]
- Verein Deutscher Ingenieure e.V. Thermoplastische Zahnräder: Werkstoffe, Werkstoffauswahl, Herstellverfahren, Herstellgenauigkeit (VDI 2736–Blatt 1); Beuth-Verlag: Berlin, Germany, 2014. [Google Scholar]
- Martienssen, W.; Warlimont, H. Handbook of Condensed Matter and Materials Data; Springer: Berlin/Heidelberg, Germany, 2005. [Google Scholar]
- Davim, J.P.; Reis, P. Machinability study on composite (polyetheretherketone reinforced with 30% glass fibre–PEEK GF 30) using polycrystalline diamond (PCD) and cemented carbide (K20) tools. Int. J. Adv. Manuf. Technol. 2004, 23, 412–418. [Google Scholar] [CrossRef]
- CAMPUS—A Material Information System for the Plastics Industry Computer Aided Material Preselection by Uniform Standards. Available online: https://www.campusplastics.com/ (accessed on 2 June 2020).
- Deutsche Edelstahlwerke (2016) Werkstoffdatenblatt 16MnCr5/16MnCrS5. Available online: https://www.dew-stahl.com/fileadmin/files/dew-stahl.com/documents/Publikationen/Werkstoffdatenblaetter/Baustahl/1.7131_1.7139_de.pdf (accessed on 2 June 2020).
- Data Sheet: VESTAKEEP® L4000G; Evonik Resource Efficiency GmbH: Darmstadt, Germany, 2019.
- Data Sheet: VESTAKEEP® 2000 GF30; Evonik Resource Efficiency GmbH: Darmstadt, Germany, 2019.
- Data Sheet: CREAMID-A4H9*800; Plastic Technology Service: Rothenburg ob der Tauber, Germany, 2014.
- Data Sheet: V-CREAMID-A3H9-1G6*M9000A; Plastic Technology Service: Rothenburg ob der Tauber, Germany, 2016.
- Deutsches Institut für Normung e.V. Geometrical Product Specifications (GPS)–Surface texture: Profile Method–Rules and Procedures for the Assessment of Surface Texture (DIN EN ISO 4288); Beuth-Verlag: Berlin, Germany, 1998. [Google Scholar]
- Laukotka, E.M. Referenzöle Datensammlung. Reference Lubricants Data Collection; FVA-Nr. 660; Forschungsvereinigung Antriebstechnik e.V.: Frankfurt/Main, Germany, 2007. [Google Scholar]
- Niemann, G.; Winter, H.; Höhn, B.R.; Stahl, K. Maschinenelemente–Band 1: Konstruktion und Berechnung von Verbindungen, Lagern, Wellen, 5th ed.; Springer: Berlin, Germany, 2019. [Google Scholar]
- Ziegltrum, A.; Maier, E.; Lohner, T.; Stahl, K. A Numerical Study on Thermal Elastohydrodynamic Lubrication of Coated Polymers. Tribol. Lett. 2020, 68, 1–13. [Google Scholar] [CrossRef]












| PA66 | PEEK | PA66 + GF30 | PEEK + GF30 | 16MnCr5 | |
|---|---|---|---|---|---|
| Young’s modulus E in MPa | 3100 | 3600 | 10,500 | 11,000 | 210,000 |
| Poisson number | 0.33 | 0.37 | 0.35 | 0.37 | 0.30 |
| Thermal conductivity in | 0.23 | 0.29 | 0.35 | 0.43 | 44.0 |
| Glass transition temperature in °C | 66 | 143 | 64 | 143 | - |
| FVA3 | PAO | WAT | |
|---|---|---|---|
| Kinematic viscosity | 95 | 100 | 90 |
| Kinematic viscosity | 40 | 40 | 40 |
| Kinematic viscosity | 11 | 14 | 15 |
| Density in | 0.896 (15°C) | 0.894 (20°C) | 1.090 (20°C) |
| Load Sequences (Temperature Behavior) | Slip Curves (Friction and Temperature Behavior) | |
|---|---|---|
| Normal force in N | >1000 | 1000; 1500 |
| Sum velocity in m/s | 1; 4; 16 | |
| Slip ratio s in % | 0 | 0/5/10/20/30/40/50/60/70 |
| Oil temperature in °C | 60 | 40; 60; 100 |
| Lubricants | FVA3 | FVA3; PAO; WAT |
| Materials | PA66; PA66+GF30; PEEK; PEEK+GF30 | |
| Setup of disks | polymer-steel | polymer-steel/polymer-polymer |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Reitschuster, S.; Maier, E.; Lohner, T.; Stahl, K. Friction and Temperature Behavior of Lubricated Thermoplastic Polymer Contacts. Lubricants 2020, 8, 67. https://doi.org/10.3390/lubricants8060067
Reitschuster S, Maier E, Lohner T, Stahl K. Friction and Temperature Behavior of Lubricated Thermoplastic Polymer Contacts. Lubricants. 2020; 8(6):67. https://doi.org/10.3390/lubricants8060067
Chicago/Turabian StyleReitschuster, Stefan, Enzo Maier, Thomas Lohner, and Karsten Stahl. 2020. "Friction and Temperature Behavior of Lubricated Thermoplastic Polymer Contacts" Lubricants 8, no. 6: 67. https://doi.org/10.3390/lubricants8060067
APA StyleReitschuster, S., Maier, E., Lohner, T., & Stahl, K. (2020). Friction and Temperature Behavior of Lubricated Thermoplastic Polymer Contacts. Lubricants, 8(6), 67. https://doi.org/10.3390/lubricants8060067