1. Introduction
Current processes for the extraction of metals from their respective ores are characterized by high energy consumption and the release of environmentally undesirable by-products, including large quantities of fine particulate, SO
2, CO
x and NO
x [
1]. In particular, ore reduction processes are based on the use of fossil fuels, with consequent high CO
2 emissions, which make primary metallurgy critical with reference to the international effort in CO
2 reduction plans. Moreover, normally these technologies require a complex raw material preparation, to fulfill process requirements, including additional plant units, with their relevant consumptions, emissions and investments.
To overcome these limits, the use of microwave energy in materials processing has been studied for nearly sixty years [
2,
3,
4], addressing the possible selective, rapid and volumetric heating offered by this technique. Researchers in the last few decades started suggesting microwave heating as an alternative way for rapid and uniform heating of pellets [
5]. Its implementation, both coupled with conventional heating techniques or as pure microwave heating, is expected to lead to further advantages in terms of faster throughput [
6]. For these reasons, microwave heating has been extensively explored in various fields of materials processing [
7], for both chemical and metallurgical industries [
2,
3,
5] but also in energy and the environmental field [
8].
Microwave heating is an energy transfer to electrically nonconductive materials, converting such energy into heat dependently on their electric, dielectric and magnetic properties [
9,
10]. It thus provides an alternative to the burning of fossil fuels and can be proficiently used to perform the initial heating which is functional to other electro-heat techniques, like induction heating or electric arc heating [
1]
In contrast to all other commonly used methods [
2], microwaves exhibits unique characteristics such as volumetric and selective heating [
7]. The main benefits of exploiting microwave energy in thermally activated processes stem from the specificity of microwave energy absorption, which can be used also against unfavorable temperature gradients [
3]. When applied to low thermal conductivity loads or relatively large solid loads, microwave heating can lead to many exceptional advantages over conventional processing methods, including generation of peculiar temperature profiles, reduction in process time [
3], saving of pre-treatment units with faster processing and both energy and cost savings and greater sustainability (CO
2 emission reduction). As a matter of fact, the heat generation inside the load eliminates the need for consuming energy on heating the walls of the furnace or reactor, its massive components and heat carriers. As a result, the use of microwave heating is expected to allow to significantly lower energy consumption, especially in high-temperature processes, since heat losses grow dramatically with an increase in the process temperature. Moreover, it was reported in literature the possible existence of the so-called ”non-thermal effect,” though its nature is still debated [
8], which becomes relevant when solid state diffusion is involved, like during sintering. In many cases microwave processing is capable of improving the product quality or it leads to results that cannot be achieved conventionally [
2]. An idea of the energy saving potential of microwave processing can be inferred from the results of a number of comparative studies regarding sintering or synthesis of specific alloys [
2]. For instance, the reactive sintering of high entropy alloys by microwave synthetic route, starting from metallic powders, allows the formation of a minimal fraction of liquid phase, leading to the possibility of achieving microwave assisted near-net shape processing [
11]. By this way the limits of current melting technologies (defects formation) or conventional solid state ones (time demanding) was overcome but with the drawback of some residual porosity ascribable to the pressure-less conditions used [
12,
13]. Comparison to other thermal (heating in furnace) and not thermal techniques (mechanical alloying) and to literature results (arc melting) allowed to evaluate the specific energy consumption of the different synthetic route. Microwave heating resulted the less energy intensive one, in the experimental conditions investigated. The small dimensions of the microwave applicator used and the short synthesis time makes this synthetic route interesting from a process intensification point of view and the benefits become particularly relevant in case of manufacturing of near net shape parts is required [
14].
The application of microwave heating to the metallurgical field, where large quantities of low thermal conductivity oxides or sulphides are used, is the natural consequence of this kind of heat generation process, but, as explained later, with some possible issues regarding the processing of loads which undergo phase transformations. Concerning conductive loads, generally, it is known that a bulk metal reflects microwaves; however, metal particles can be heated [
15] and in case of ferromagnetic powders, the surface heating rate achievable can result even higher, indicating that magnetism is related to the heating mechanism. However, many processes of the primary metallurgy start from non-conductive loads but can end with conductive ones, possibly even in the molten state.
The earliest application of microwave energy to metallurgy can be traced back to 1960s when Ford et. al. began with a study of the high temperature processing of certain oxides and sulfides using a resonant cavity [
16]. The direct reduction of metal oxides using microwave energy has been extensively explored since the early 1990s [
7]. Roy reported full sintering of metallic materials in 1999 [
17]. More recently, pioneering applications of microwave assisted combustion synthesis of pure metal powders as reactants has been used by some of the authors to prepare intermetallics [
18], functionally graded materials [
19] or to join dissimilar materials [
20]. During last decades, microwave heating has been extended to various fields of metallurgy, especially ferrous pyrometallurgy and nonferrous hydrometallurgy. The distinguishing advantages of microwave heating described above (such as volumetric and selective heating) lead to rapid chemical reactions (extraction of metals) with much less environmental pollution. Most studies found improved metal productivity and/or extraction rates by applying microwave irradiation as the energy source, compared to conventional heating [
5]. Application of microwave energy to extractive metallurgy, from ore extraction, to concentration, roasting and reduction is still in the early stages of development, although it has been studied for over half a century [
7].
In recent years, there have been significant advancements and achievements in both investigations of microwave fundamentals-associated metallurgical processes for extraction of a number of metals and microwave applications to pretreatment of metal sources, reduction of metal oxides/ores, treatment of relevant wastes and synthesis of metal powders [
21]. In particular, microwave ironmaking and steelmaking were found successful in numerous laboratory-scale studies exhibiting a significant reduction in energy costs and CO
2 emissions. Over the past 10 years, researchers also made significant progress in the scale-up of this novel metallurgical technology with successful construction of large microwave heating systems equipped with power up to 225 kW [
7,
22]. Microwave heating of iron ore was investigated as an alternative to conventional reduction processes to solve the problem of slow heat transfer [
3]. Minerals such as magnetite and carbon have high losses (i.e., are good microwave absorbers) and can be heated selectively. Heat transfer, thus, can become independent of the gas stream and high-velocity gas flows are not required, which mitigates potential dust problems and allows for significant savings in fan energy [
3,
23]. In this framework, the use of pelletization can lead to even higher advantages. For preparation of carbon-containing pellets, most of literature studies focused on the use of pulverized coal and coke, still causing large emissions of SO
2, NO
x and CO
2 in the ironmaking process [
24]. Early attempts [
23] at combining iron ore and carbon into composite pellets and then reducing them in a high-temperature furnace were not economically successful. Although the intimate mixing of ore and carbon did accelerate the reduction process by reducing the distance the gases had to travel, it did not yield the expected benefit in the conventional-heating system. The reason is that the conventional-heating system does not have the ability to supply heat to the interior of the composite pellets at a rate fast enough to compensate for the heat consumed by the gasification of carbon. Consequently, carbothermic reduction using conventional heating results in composite pellets with “cold centers” and, consequently, low reduction rates. Hence, the application of microwave energy can be a way to subvert the heat-transfer problem entirely, by generating the energy needed to drive gasification inside the pellet [
3]. The reduction rate results then faster in microwave heating than conventional one. Other possible successful applications of microwave heating to the metallurgical field is the recovery of iron from red muds [
25]. Microwaves offer selective volumetric heating of ferrous minerals and the presence of hematite as well as of titanium phases in red mud results in high microwave susceptibility. In addition, the microwave assisted carbothermal reduction offers a faster and cleaner reduction process. It is largely documented [
7] that microwave reduction of many metal-bearing minerals could be achieved rapidly, which is attributed to the volumetric and selective heating characteristics of microwave heating [
7,
24].
The wide availability of microwave power sources at ISM frequencies (i.e., allocated for Industrial, Scientific and Medical use) of 915 MHz and 2.45 GHz and good microwave absorption properties of many materials have led to the emergence of industrial facilities for various applications with hundreds of megawatts in total of installed microwave power [
2]. Also, attempts to apply microwave heating for metallurgy at higher frequencies, like 28 and 30 GHz, were widely reported [
7]. However, it should be noted that in most studies reported thus far, experiments were performed on a small scale, using microwave power lower than 3 kW.
However, from studies over the past half century, it was recognized that there are still difficulties that hinder the advancement of microwave-assisted metallurgy and more broad applications of this technology to materials processing, deriving mostly form the lack of homogenous heating of static loads and the process control when reactions occur, making the load nature changing dramatically (i.e., from dielectric to conductive, from solid to liquid). Moreover, the limited power capacity of microwave generators is an issue in the scaling-up to industrial capacity, due to economic factors and plant design constraints. Many challenges were confronted in the commercialization and industrialization of microwave assisted metallurgy [
7,
21]. As a matter of fact, microwave heating of materials has a two main limitations: non-uniform heating and thermal runaway [
2,
5]. The former has been observed in many experiments where ‘hot spots’ are generated. This is primary caused by non-uniform microwave distribution as a result of improper applicator design but is also affected by various microwave absorption behaviors of materials [
23]. The intrinsic characteristic of microwave energy, especially volumetric heating and the non-uniform distribution, in moderate-scale industrial applicator cause inhomogeneous temperature distribution. The inhomogeneous temperature distribution is more serious during large scale microwave heating, being the microwave power penetration depth a kind of limiting factor for processing large static loads but also due to large load surface exposed to the furnace colder environment, leading to heat losses. To overcome this limit both thermal insulation arrangements for reducing heat losses and hybrid heating system might be implemented. In addition, due to the strong temperature dependences of permittivity and permeability [
26], thermal runaway usually makes the metallurgical heating process difficult to control. In principle, according to some authors, non-uniform heating and thermal runaway may be simultaneously associated with nonthermal effects, which affect the heating process by accelerating reaction rates [
6].
As mentioned, the proper microwave applicator design is mandatory. In this framework, both in the research and application development areas a crucial role is played by the modelling and simulation of microwave processes [
2]. Combining efforts of numerical simulation and experimental attempts, based on further understanding of microwave–material interactions, would not only contribute to successful design of efficient microwave furnaces for commercial and industrial uses in the field of metallurgy but also benefit from the exploration of many relevant material processing applications for recognizing the full potential of microwave heating.
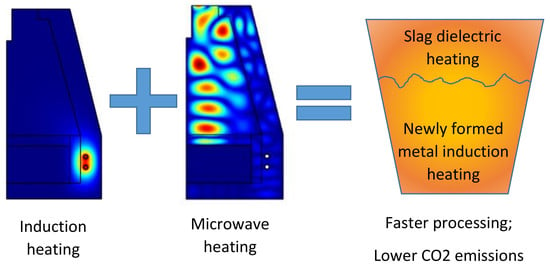
In summary, microwave energy has been broadly used for metallurgy because of the unique and distinguishing advantages of microwave heating. Although there is still much work ahead for advancing the understanding and applications of microwave-assisted metallurgy. One possible solution would be the coupling of different heating techniques, exploiting the advantages of each one of them in processing a special class of materials. For instance, when it comes to molten metal, induction heating is known to provide extremely rapid (and again, volumetric) heating, besides acting as stirring system, for some class of melts. On the other hand, induction heating is not as effective in heating dielectric materials, like the slag which is usually present as a byproduct of the reduction process. Hence, where microwave efficiency drops, induction heating could supply a valuable help and vice versa.
Based on these premises, a new microwave applicator designed to selectively heat the dielectric portion of a heterogeneous and layered load (slag over a molten metal) has been developed using numerical simulation. The applicator is designed in order to be coupled with an induction heating module, to process the newly formed metal. The novelty of this combined approach lies also in the possibility of realizing a complete reduction equipment in the small scale. This would allow to process mineral ores also at the small scale, for instance to serve as auxiliary plants to manufacture “on demand” intermediates for the steelmaking process, like ferroalloys. Ferroalloys are widely used to modify the chemical composition of iron based alloys but in many cases their addition amounts only to a limited percentage of the alloy weight. They possess a high value, especially the low carbon variants, and thus they could benefit of the use of smaller, electrical-powered units, where reduction is performed by metallothermal reactions or using non-carbon based reductants, possibly under vacuum or controlled atmosphere.
3. Prototype Testing and Results
Figure 10 shows the applicator, comprehensive of the induction heating section (bottom part, including an ingot casting station), the microwave heating part and auxiliaries for loading/unloading and gases treatment. The microwave applicator is fed by a new solid state source, 7.5 kW RF power, at 915 ± 15 GHz supplied by MKS Alter, through a coaxial to waveguide transition, a 3-stub tuner and a quartz pressure window. The equipment, as a matter of fact, can be operated both in vacuum and in inert gas overpressure up to 5 atm.
In order to perform a simple and rapid validation of the modelling results, considering the existence in the applicator of multiple loads (the load itself and the refractory materials and crucible), calorimetric testing with a tap water load have been performed, changing the operating frequency of the microwave source. Results, summarized in
Figure 11, confirm that, operating on water load and in a limited bandwidth, the applicator provides the highest energy efficiency at 915 MHz.
Despite a good qualitative agreement, the measured efficiency results lower. This can be ascribed to multiple factors, neglected during simulation, ranging from the hypothesis of using perfect electrical conductors for the applicator walls and neglecting heat transfer. As a matter of fact, the here presented numerical simulation addressed only electromagnetic field distribution and power density, not the temperature increase of the load. Such temperature is affected also by heat dissipation towards the surrounding environment, which remains substantially colder. Moreover, no temperature dependence of permittivity of water has been considered in the simplified model, while large variation can occur as temperature increases. This has been experimentally observed also using the “autotuning” function of the solid state generator, with the optimum operating frequency shifting from the initial 915 MHz as heating proceeds.
The applicator was then reverted to the heating of metallurgical products and to test the capabilities of performing a rapid and efficient dielectric heating, some reference loads have been used, amounting to 2500 g of product to be processed. The metallurgical products tested were—iron oxide pellets used for blast furnace (main composition 90% Fe
2O
3, 6.5% SiO
2 + other elements; 3% bentonite as a binder, particle size below 20 mm), electrical arc furnace dust from Rubiera Special Steel S.p.A. (main composition 3.2% C, 32% Fe, 22.1% Zn, 5% CaO, 3% SiO
2 + other elements and oxygen, in powder shape unmilled) and the metallurgical slag comes from the production of lead in K.S.S. plant (main composition 19.4% Fe, 15% Zn, 25% CaO, 25% SiO
2 + other elements and oxygen, in granules).
Table 5 summarizes the results in terms of achievable maximum temperature, measured by a contact thermocouple after 60 s from the turning off of the microwave power, without induction heating. Frequency has been kept fixed during the whole process, using one of the two best frequencies obtained by modelling (see
Figure 5).
Results show that using the frequency control option of the solid state generator, extremely low reflected power as measured by the directional coupler of the generator can be achieved, lower than 0.3%. In case of using of the autotuning option, it automatically selected an operating frequency which further minimizes reflections. This is not necessarily the best way to conduct the reduction process, as for some frequencies the refractories dissipate large amounts of power, instead of having it transferred to the load.
For instance, referring to data of
Table 5, the EAF dust has been heated with similar input power but in case of frequency of 920 MHz, the average reflected power results slightly higher but the final temperature is 200 °C higher than operating with higher power at 925 MHz. Moreover, as expected for loads experiencing phase transformations and heat losses through the crucible walls and open top, there is a strong nonlinearity between emitted power and final average temperature. This is evident in the case of slag heating, where an almost 2000 W increase of the power output, for a given frequency of 925 MHz, provides only 150 °C increase. These preliminary results suggest that temperature control of the load during heating is mandatory for this kind of equipment and that the coupling of the experimental activity with modelling—tough simplified—can provide a better insight to the process and help explaining some apparent anomalies. This approach will be followed on the next developments with repeated metal-making tests and acquisition of the relevant parameters to evaluate the specific energy consumption and yields.