Optimization of Squeeze Casting Parameters for 2017 A Wrought Al Alloy Using Taguchi Method

Abstract

This study applies the Taguchi method to investigate the relationship between the ultimate tensile strength, hardness and process variables in a squeeze casting 2017 A wrought aluminium alloy. The effects of various casting parameters including squeeze pressure, melt temperature and die temperature were studied. Therefore, the objectives of the Taguchi method for the squeeze casting process are to establish the optimal combination of process parameters and to reduce the variation in quality between only a few experiments. The experimental results show that the squeeze pressure significantly affects the microstructure and the mechanical properties of 2017 A Al alloy.

1. Introduction

Recently, great attention has been focused on aluminium and its alloys due to their high technological value and wide range of industrial applications, thanks to their various advantages such as lower density, good formability, high thermal conductivity, high specific rigidity, excellent corrosion resistance, high castability and attractive tensile strength [1,2]. For this reason, aluminium alloys are widespread, used especially in the most important industrial material of foundry. On the other hand, they offer important opportunities for applications in a diversity of areas particularly in mechanical automotive and aerospace industry [3].

In recent years, a new casting technology called squeeze casting has been developed to make better use of aluminium alloys [4]. Squeeze casting (as liquid metal forging) is a casting process which solidifies the molten metal under pressure on the closed die positioned between the plates of hydraulic press [5,6,7,8]. Compared with conventional casting methods, squeeze casting possesses many pronounced advantages, such as free shrinkage and gas porosity, to provide components with high integrity with improved mechanical properties. Yue et al. [9] found that the squeeze casting process was an ideal process to produce high quality light metal components with near net shape. Kim et al. [10] stated that squeeze casting accounted for a 15%–40% improvement in mechanical properties from the gravity die casting process. Vijian et al. [7] reported that squeeze casting exhibited remarkable grain refinement and substantial improvement in mechanical properties.

Many research works on squeeze casting parameters of aluminium alloys [11,12,13] and magnesium alloys [14,15] as well as their composites [16,17,18] have been reported in the literature. The intensity of applied pressure, the melt temperature and the die temperature have been shown to be among the most important parameters affecting the quality of squeeze cast components [7,19]. An understanding of the effects of process parameters is particularly important since the mechanical properties of components are related to the microstructure and the casting variables to a large extent [20]. In this regard, Malki et al. [11,12] have investigated effects of squeeze casting parameters on the macrostructure, microstructure, density and hardness of LM13 aluminium alloy. The results indicated that an increase in applied pressure decreased the grain size and SDAS (Secondary Dendrite Arm Spacing) of the primary α-phase (Al), as well as modifying the eutectic silicon particles and improving hardness. A decrease in the die or melt temperature rendered similar effects on the microstructure, macrostructure and hardness of the as-cast samples [11,12]. Optimizing these parameters is particularly important. However, Taguchi statistical design is a powerful method to understand the effect of these processing factors by running only a few experiments.

The present investigation aims, essentially, to determine a good combination of applied pressure, melt temperature and the die temperature for squeeze casting 2017 A wrought Al alloy. Ultimate tensile strength (UTS) and hardness tests of the liquid forged samples at different squeeze casting parameters were characterized and the optimal condition is found by the Taguchi method.

2. Statistical Analysis and Discussion

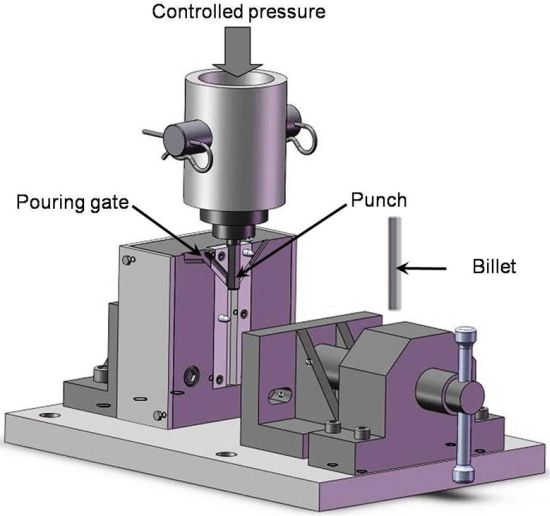
The squeeze casting process parameters namely squeeze pressure (A), melt temperature (B) and die temperature (C) at three levels are listed in Table 1. To ensure the accuracy of the results, three samples were fabricated for each of the parameter combinations. The averages were computed for ultimate tensile strength (UTS) and hardness in each of the nine experimental conditions. In the latter, main effect, variance analysis (ANOVA) and signal-to-noise (S/N) ratio are analyzed to find ranking and optimum levels of the process parameters.

Table 1. Results of L₉ orthogonal array experiments.

No	A	B	C	UTS (MPa)				Hardness (HV)
				Y1	Y2	Y3	Average	Y1	Y2	Y3	Average
1	30	700	200	176	178	170	174.667	65	69	64	66.000
2	30	750	250	159	162	167	162.667	56	62	59	59.000
3	30	800	300	154	157	148	153.667	54	58	56	56.000
4	60	700	250	178	198	189	188.333	77	65	79	73.666
5	60	750	300	178	172	175	175.000	74	65	68	69.000
6	60	800	200	175	180	185	180.000	76	65	78	73.000
7	90	700	300	208	213	216	212.333	82	80	86	82.666
8	90	750	200	202	204	209	205.000	80	77	84	80.333
9	90	800	250	198	203	196	199.000	78	70	74	74.000

Table 1. Results of L₉ orthogonal array experiments.

No	A	B	C	UTS (MPa)				Hardness (HV)
				Y1	Y2	Y3	Average	Y1	Y2	Y3	Average
1	30	700	200	176	178	170	174.667	65	69	64	66.000
2	30	750	250	159	162	167	162.667	56	62	59	59.000
3	30	800	300	154	157	148	153.667	54	58	56	56.000
4	60	700	250	178	198	189	188.333	77	65	79	73.666
5	60	750	300	178	172	175	175.000	74	65	68	69.000
6	60	800	200	175	180	185	180.000	76	65	78	73.000
7	90	700	300	208	213	216	212.333	82	80	86	82.666
8	90	750	200	202	204	209	205.000	80	77	84	80.333
9	90	800	250	198	203	196	199.000	78	70	74	74.000

2.1. Main Effects

The average value of UTS and hardness for each parameter A, B and C at level 1, 2 and 3 are grouped in Table 2. The response graphs of the main effects and their variation between levels of the parameters on the UTS and hardness are shown in Figure 1 and Figure 2, respectively. The average values of the response at each parameter level are obtained by adding the results of all trails conditions at the level considered, and then dividing by the number of data points added. The main purpose of this work is to find the “larger is better” which is the experiment goal obtained by setting the process parameters. It is clear from Table 2 and from Figure 1 and Figure 2 that the UTS and hardness have maximum values at the third level of parameter A (90 MPa) and at the first level of parameters B (700 °C) and C (200 °C). Hence, it can be concluded that the optimum levels were A3 B1 C1. The increase in the UTS, and hardness with increasing squeeze pressure could be attributed essentially to the refinement of the microstructure. In general, the applied pressure has the advantage of increasing the density and suppressing the shrinkage during the solidification of metals [12,21,22]. In addition, decreasing both melting and die temperatures can cause the increase of UTS and hardness. This has been attributed to the sudden increase of cooling rate which leads to the decrease of the alloy grain size. It can also be seen from Table 3 that the above combination of factor levels (3, 1, 1) are not among the nine combinations tested for the experiment. The reason for this difference could be the multifactor nature of the experimental design employed (nine from 27 possible combinations).

Table 2. Levels average for main effects.

Level (L)	Average UTS (MPa)			Average Hardness (HV)
	A	B	C	A	B	C
L1	163.7	191.8	186.6	60.33	74.11	73.11
L2	181.1	180.9	183.3	71.89	69.44	68.89
L3	205.4	177.6	180.3	79	67.67	69.22
Max-Min	41.8	14.2	6.2	18.67	6.44	4.22
Rank	1	2	3	1	2	3

Table 2. Levels average for main effects.

Level (L)	Average UTS (MPa)			Average Hardness (HV)
	A	B	C	A	B	C
L1	163.7	191.8	186.6	60.33	74.11	73.11
L2	181.1	180.9	183.3	71.89	69.44	68.89
L3	205.4	177.6	180.3	79	67.67	69.22
Max-Min	41.8	14.2	6.2	18.67	6.44	4.22
Rank	1	2	3	1	2	3

Table 3. Experimental layout using L₉ standard orthogonal array.

Test number	A	B	C
1	1	1	1
2	1	2	2
3	1	3	3
4	2	1	2
5	2	2	3
6	2	3	1
7	3	1	3
8	3	2	1
9	3	3	2

Table 3. Experimental layout using L₉ standard orthogonal array.

Test number	A	B	C
1	1	1	1
2	1	2	2
3	1	3	3
4	2	1	2
5	2	2	3
6	2	3	1
7	3	1	3
8	3	2	1
9	3	3	2

Figure 1. Main effects graph for ultimate tensile strength (UTS).

Figure 1. Main effects graph for ultimate tensile strength (UTS).

Figure 2. Main effects graph for hardness.

Figure 2. Main effects graph for hardness.

2.2. Analysis of Variance (ANOVA)

The analysis of variance (ANOVA) was used to investigate which parameters significantly affected the quality characteristic and to determine the percentage contribution of the parameters at 95% confidence level. The F ratio value named Fisher test was used to see which process parameters have a significant effect. Usually, when the F-values of experimental trials are higher than 5.32 (from the F table), it means that the change in the process parameter has significant effect on the quality characteristic. In addition, the percentage contribution expresses the importance of the process parameters for the response.

ANOVA analysis for UTS and hardness was carried out using Equations (2)–(6) and the resulting data is given in Table 4 and Table 5, respectively. The significance and importance of the parameters were determined by the F-value and percentage contribution, respectively. The F-value in Table 4 and Table 5 shows that the considered process parameters are highly significant factors affecting the mechanical properties of 2017A Al alloy in the order of squeeze pressure (parameter A), melt temperature (parameter B) and die temperature (parameter C). However, squeeze pressure has the most significant effect on UTS and hardness as shown by much higher F-value (i.e., 197.74 and 122.33) and also percent contribution (i.e., 85.93 and 83.06). The percentage contribution of significant factors on the quality characteristic is shown in Figure 3.

Table 4. Variance (ANOVA) Table for ultimate tensile strength (UTS).

Source	Degrees of freedom (DOF)	Sum of squares (SS)	Variance (V)	F ratio	Percent contribution (P)
A	2	5.9391	2.9695	197.74	85.93
B	2	0.7591	0.3795	25.28	10.98
C	2	0.1831	0.0915	6.10	2.65
Error	2	0.0300	0.0150		0.44
Total	8	6.9114			100.00

Table 4. Variance (ANOVA) Table for ultimate tensile strength (UTS).

Source	Degrees of freedom (DOF)	Sum of squares (SS)	Variance (V)	F ratio	Percent contribution (P)
A	2	5.9391	2.9695	197.74	85.93
B	2	0.7591	0.3795	25.28	10.98
C	2	0.1831	0.0915	6.10	2.65
Error	2	0.0300	0.0150		0.44
Total	8	6.9114			100.00

Table 5. Variance (ANOVA) Table for hardness.

Source	Degrees of freedom (DOF)	Sum of squares (SS)	Variance (V)	F ratio	Percent contribution (P)
A	2	8.4730	4.2364	122.33	83.06
B	2	1.0712	0.5356	15.47	10.50
C	2	0.5875	0.2937	8.48	5.76
Error	2	0.0693	0.0346		0.68
Total	8	10.2009			100.00

Table 5. Variance (ANOVA) Table for hardness.

Source	Degrees of freedom (DOF)	Sum of squares (SS)	Variance (V)	F ratio	Percent contribution (P)
A	2	8.4730	4.2364	122.33	83.06
B	2	1.0712	0.5356	15.47	10.50
C	2	0.5875	0.2937	8.48	5.76
Error	2	0.0693	0.0346		0.68
Total	8	10.2009			100.00

Figure 3. Percentage contribution of significant control factors.

Figure 3. Percentage contribution of significant control factors.

2.3. Signal to Noise Ratio (S/N)

The next analysis was investigated by using analysis of signal-to-noise ratio (S/N). According to the data presented in Table 1 and Equation (6), the average response and S/N ratio of UTS and hardness for the nine experiments were calculated on the basis of the following procedure. Typically, the average effect for level 1 of the squeeze pressure is computed using data from experiments 1–3 of Table 6. Similarly, the average effects for levels 2 and 3 of squeeze pressure were computed using data from experiments 4–6 and 7–9, respectively. The level 3 for squeeze pressure (90 MPa, see No.7–9) has the highest S/N ratio value, which indicates that the casting performance at such level produces the minimum variation of the UTS and hardness due to uncontrollable factors. However, a maximum of S/N ratio corresponds to better quality characteristics [23]. It can also be seen from Table 6 that experiment number 7 possesses the largest S/N ratio when squeeze pressure at 90 MPa, melt temperature at 700 °C and die temperature at 300 °C; therefore, the combination of parameters and their levels is A3 B1 C3 as shown in Table 3.

Table 6. Computation of S/N ratio for ultimate tensile strength (UTS) and hardness.

No	Average UTS	Average hardness	S/N ratio of UTS	S/N ratio of hardness
1	174.667	66.000	44.839	36.377
2	162.667	59.000	44.220	35.394
3	153.667	56.000	43.727	34.952
4	188.333	73.666	45.473	37.246
5	175.000	69.000	44.858	36.739
6	180.000	73.000	45.098	37.180
7	212.333	82.666	46.537	38.334
8	205.000	80.333	46.232	38.081
9	199.000	74.000	45.974	37.359
Mean	Y1=183.407	Y2=70.407

Table 6. Computation of S/N ratio for ultimate tensile strength (UTS) and hardness.

No	Average UTS	Average hardness	S/N ratio of UTS	S/N ratio of hardness
1	174.667	66.000	44.839	36.377
2	162.667	59.000	44.220	35.394
3	153.667	56.000	43.727	34.952
4	188.333	73.666	45.473	37.246
5	175.000	69.000	44.858	36.739
6	180.000	73.000	45.098	37.180
7	212.333	82.666	46.537	38.334
8	205.000	80.333	46.232	38.081
9	199.000	74.000	45.974	37.359
Mean	Y1=183.407	Y2=70.407

The combination shown above differs from the previously mentioned one in main effects. It sheds light on the optimum combination of parameters and their levels. However, it shows that, in the present case study, the combination of parameters and their levels A3 B1 C3 yield optimum mechanical properties with minimum variance from the target value.

2.4. Estimation of Predicted Mean and Confirmation Test

The purpose of estimation of predicted means is to validate the squeeze casting condition at the optimal levels of parameters, which is A3 B1 C1 for mechanical properties. The predicted mean (μ) for UTS and hardness was estimated using the following two equations [24]:

μ_UTS = Y1 + (A3 − Y1) + (B1 − Y1) + (C3 − Y1)

(1)

μ_hardness = Y2 + (A3 − Y2) + (B2 − Y2) + (C3 − Y2)

(2)

where, A3 is the average UTS and hardness at third level of squeeze pressure (Table 2), B1 is the average UTS and hardness at first level of melt temperature (Table 2), C1 is the average UTS and hardness at first level of die temperature (Table 2), Y1 and Y2 are the means of UTS and hardness (Table 6). Substituting the values of various terms in Equations (1) and (2), then

µ_UTS = 183.407 + (205.4 − 183.407) + (191.8 − 183.407) + (186.6 − 183.407) = 216.986 MPa

(3)

µ_hardness = 70.407 + (79 − 70.407) + (74.11 − 70.407) + (73.11 − 70.407) = 85.406 HV

(4)

Three confirmation tests are conducted at the optimum settings of squeeze casting parameters recommended by the investigation. The average values of UTS and hardness obtained at the optimum settings of the process parameters are 219.333 MPa and 86.666 HV, respectively. We notice that the difference between the estimated results and the experimental results is negligible. Therefore, the experimental values are within the confidence interval of the predicted optimal of mechanical properties.

2.5.Effect of the Squeeze Pressure on Microstructure and Mechanical Properties

The influence of squeeze pressure (the most significant factor) on the microstructure and the mechanical properties has been analyzed on the basis of the statistical analysis developed in Section 2.1 (at pouring and die temperatures of 700 °C and 200 °C, respectively).

Figure 4a–d illustrates the microstructure of the 2017 A Al alloy squeeze cast under various pressure levels.

Figure 4. Optical micrographs of the squeeze cast sample (a) 15 MPa; (b) 30 MPa; (c) 60 MPa; and (d) 90 MPa applied pressure.

Figure 4. Optical micrographs of the squeeze cast sample (a) 15 MPa; (b) 30 MPa; (c) 60 MPa; and (d) 90 MPa applied pressure.

These micrographs show that the microstructures prepared under higher applied pressures are much finer and smaller α-primary dendrites. It is clear that the squeezing pressure has significant influence on the microstructure of the alloy [7]. Furthermore, the inter-metallic phases in the alloy with no applied pressure are coarser than those under high squeezing pressure. This effect is a result of the change in phase diagram according to the Clausius-Clapeyron Equation [6]:

(5)

where T_f is the equilibrium freezing temperature, P is the applied pressure, V_l and V_s are the specific volumes of the liquid and solid, respectively, and ΔH_f is the latent heat of fusion. During the solidification process, both ΔH_f and (V_l − V_s) are normally negative due to the heat release and shrinkage of metals, respectively. Thus, dT_f/dP is positive, which indicates that the applied pressure will increase the melting point of a metal having a volume decrease tendency during solidification. Increasing the freezing point causes undercooling in the alloy that is already superheated. However, such change in freezing temperature with the increasing pressure is expected due to the reduction in interatomic distance and thus the restriction of atomic movement [6]. The higher freezing point brings about the larger undercooling in the initially superheated alloy and thus elevates the nucleation frequency, resulting in a more fine-grained structure. Apart from the changes in undercooling of the molten alloy caused by applied pressure, greater cooling rates for the solidifying alloy can be realized due to reduction in the air gap between the alloy and the die wall and thus larger effective contact area. Obviously, the increase of cooling rate and heat-transfer coefficient will result in the refinement of the grain size of squeeze casting alloy.

The mechanical properties of squeeze cast specimens such as ultimate tensile strength (UTS) and hardness (HV) are compiled in Figure 5. It shows that an increase in squeeze pressure from 15–90 MPa enhances the UTS with 46% increment from 150 MPa (15 MPa) to 219.66 MPa (90 MPa). Also, there is a 58% increase in hardness over the 15 MPa squeeze pressure.

Evidently the improvement of mechanical properties by increasing the pressure up to 90 MPa seems to be attributed, in part, to the refinement of the α-primary dendrites and, in part, to material densification.

Figure 5. Ultimate tensile strength (UTS) and hardness of 2017 A Al alloy manufactured in various conditions.

Figure 5. Ultimate tensile strength (UTS) and hardness of 2017 A Al alloy manufactured in various conditions.

3. Experimental Procedure

3.1. Design of Experiments

The traditional experimental techniques, i.e., varying one parameter at a time while keeping others constant is complex and further suffers from the major drawback of a large number of experiments which in turn increases the cost of experiments to achieve superior-quality products. The Taguchi method is one of the solution tools that helps decrease the number of experiments [25] and to achieve a high quality system without increasing costs [26]. This technique that combines the quality loss function concept and experimental design theory has been applied for solving several complex problems in manufacturing industries.

In this study, the Taguchi method has been adopted to observe the influencing process parameters in the squeeze casting process. Taguchi statistical design is adopted to understand the effect of these processing parameters by running only a few experiments while achieving strong mechanical properties. The casting parameters each at three levels considered in this study and the details are presented in Table 7.

Table 7. Squeeze casting parameters and their levels.

Notation	Parameters	Level 1	Level 2	Level 3
A	Squeeze pressure (Mpa)	30	60	90
B	Melt temperature (°C)	700	750	800
C	Die temperature (°C)	200	250	300

Table 7. Squeeze casting parameters and their levels.

Notation	Parameters	Level 1	Level 2	Level 3
A	Squeeze pressure (Mpa)	30	60	90
B	Melt temperature (°C)	700	750	800
C	Die temperature (°C)	200	250	300

The Taguchi technique employs a generic signal-to-noise (S/N) ratio to quantify the present variation. Broadly speaking, the (S/N) ratio is the ratio of the mean (signal) to the standard deviation (noise). Depending on the particular type of characteristics involved, three types of S/N ratios are applicable, including “higher is better” (HB), “lower is better” (LB) and “nominal is best” (NB). Because the target of this work is to maximize the mechanical properties (UTS and hardness), the S/N ratio with HB characteristics is required, which is given by:

(6)

where n is the number of measurements in a trial under the same design conditions, (here n = 3), Y represents the results of measuring and subscript i indicates the number of simulation design parameters in the orthogonal array (OA) table.

A statistical analysis of variance (ANOVA) can be performed in order to see which process parameter (factor) is statistically significant for each quality characteristic (see Equations (7)–(11) [27,28]).

(7)

(8)

DOF = N − 1

(9)

V_factor = SS_factor/DOF

(10)

F_factor = V_factor/V_error

(11)

where SS_total is the total sum of squares, N is the total number of experiments, SS_A the factorial sum of squares due to factor A, K_A represents the number of levels for factor A, A_i stands for the sum of the total ith level of the factor A, n_Ai the number of samples for ith level of factor A, T the sum of total (S/N) ratio of the experiments, DOF the number of degrees of freedom, V_factor the variance of the factor, SS_factor represents the sum of squares of the factor and F_factor is the F ratio of the factor.

3.2. Material

The experiments were carried out using a 2017 A wrought aluminium alloy. The material provides average tensile strength but good machinability. It is widely used in mechanical applications [29,30]. The alloy is received as extruded bar of diameter 80 mm. Its chemical compositions are Cu 4.47, Mg 0.45, Si 0.86, Fe 0.49, Mn 0.36, Ni 0.1, Pd 0.03, Zn 0.25, Cr 0.1 (mass fraction, %) and Al balance. The material was melted in an electric resistance furnace using a steel crucible.

3.3. Squeeze Casting Method

The squeeze casting experiments were performed on a hydraulic press (see Figure 6 and Figure 7) consisting of steel mould. The device allows the molding of vertical specimens under a pressure of up to 90 MPa applied by the punch until material solidification. The device is also equipped with a thermocouple which provides temperature regulation of the mold. The punch-and-die set were made of hot-die steel and the cast billet is a rod shaped with a circular cross-section of 23 mm diameter and a length of 110 mm. The die was coated with a graphite suspension before each experiment.

Figure 6. Experimental setup of squeeze casting process.

Figure 6. Experimental setup of squeeze casting process.

Figure 7. Schematic representation of squeeze casting process.

Figure 7. Schematic representation of squeeze casting process.

3.4. Tensile and Hardness Testing

The tensile specimens were machined to evaluate the ultimate tensile strength (UTS). For each experimental condition, three specimen samples were prepared. INSTRON (ENSIL, Limoges, France) universal testing machine was used for performing tensile tests on the specimens. The tests were performed under displacement control with a strain rate start at 1 mm·min⁻¹. An extensometer (gage length of 14.3 mm) is attached with two rubber bands to the central part of the specimen.

Hardness analysis HV was performed on a transverse section of the specimen. Measurements were performed employing a MEKTON Vickers Hardness Tester with a diamond pyramidal indenter. Three measurements were taken at randomly selected points with a load of 300 g applied for 30 s.

3.5. Selection of Orthogonal Array (OA)

In Taguchi technique, experimental analysis is based on orthogonal array (OA). It is the shortest possible matrix of combinations in which all the parameters vary at the same time and their effect and performance interactions are studied simultaneously. The selection of an appropriate orthogonal array depends on the total degrees of freedom (DOF) required [31]. In this study, an L₉ (3³) standard orthogonal array is considered in determining the effect of three process parameters. Thus, the array has three columns and nine rows of three levels. The number of experiments required can be reduced to nine, which in classical combination method using full factorial experimentation would require 3³ = 27 experiments to capture the influencing parameters. An L₉ standard OA is shown in Table 3 was employed for present investigation.

4. Conclusions

In this study, the optimal squeeze casting parameters of 2017 A wrought aluminium alloy have been specified through the Taguchi method, and the obtained results are acceptable for the ranges of squeeze parameters that have been selected in the present investigation. According to the results, the following conclusions can be drawn:

• The combination A3 B1 C1 that means squeeze pressure 90 MPa, melt temperature 700 °C and die temperature 200 °C are recommended to obtain higher mechanical properties in squeeze casting of 2017 A Al alloy.
• Squeeze pressure, melt temperature and die temperature were identified as significant process parameters from ANOVA. It is noted that the contribution of squeeze pressure is a larger for the UTS and hardness.
• From the S/N ratio, it was evident that the combination of parameters and their levels A3 B1 C3 yield the optimum mechanical properties with minimum distinction about the target value.
• The refinement of microstructure was the main reason for increasing the mechanical properties of the squeeze cast specimens.