On the PLC Effect in a Particle Reinforced AA2017 Alloy
Abstract
:1. Introduction
2. Materials and Methods
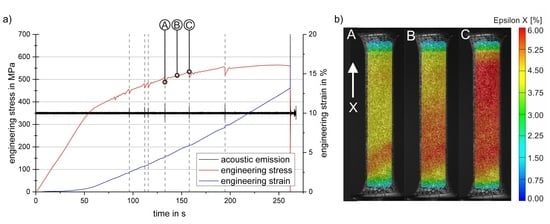
3. Results and Discussion
4. Summary and Conclusions
Supplementary Materials
Acknowledgments
Author Contributions
Conflicts of Interest
References
- Böhlke, T.; Bondár, G.; Estrin, Y.; Lebyodkin, M.A. Geometrically non-linear modeling of the Portevin-Le Chatelier effect. Comput. Mater. Sci. 2009, 44, 1076–1088. [Google Scholar] [CrossRef]
- Chmelἱk, F.; Pink, E.; Król, J.; Balἱk, J.; Pešička, J.; Lukáč, P. Mechanisms of serrated flow in aluminium alloys with precipitates investigated by acoustic emission. Acta Mater. 1998, 46, 4435–4442. [Google Scholar] [CrossRef]
- Delaunois, F.; Denil, E.; Marchal, Y.; Vitry, V. Accelerated aging and Portevin-Le Chatelier effect in AA 2024. Mater. Sci. Forum 2017, 879, 524–529. [Google Scholar] [CrossRef]
- Gupta, S.; Beaudoin, A.J.; Chevy, J. Strain rate jump induced negative strain rate sensitivity (NSRS) in aluminum alloy 2024: Experiments and constitutive modeling. Mater. Sci. Eng. A 2017, 683, 143–152. [Google Scholar] [CrossRef]
- Jiang, H.; Zhang, Q.; Chen, X.; Chen, Z.; Jiang, Z.; Wu, X.; Fan, J. Three types of Portevin-Le Chatelier effects: Experiment and modelling. Acta Mater. 2007, 55, 2219–2228. [Google Scholar] [CrossRef]
- Jiang, H.; Zhang, Q.; Wu, X.; Fan, J. Spatiotemporal aspects of the Portevin-Le Chatelier effect in annealed and solution-treated aluminum alloys. Scr. Mater. 2006, 54, 2041–2045. [Google Scholar] [CrossRef]
- Jiang, Z.; Zhang, Q.; Jiang, H.; Chen, Z.; Wu, X. Spatial characteristics of the Portevin-Le Chatelier deformation bands in Al-4 at%Cu polycrystals. Mater. Sci. Eng. A 2005, 403, 154–164. [Google Scholar] [CrossRef]
- Rashkeev, S.N.; Glazov, M.V.; Barlat, F. Strain-rate sensitivity limit diagrams and plastic instabilities in a 6xxx series aluminum alloy. Part I: Analysis of temporal stress-strain serrations. Comput. Mater. Sci. 2002, 24, 295–309. [Google Scholar] [CrossRef]
- Serajzadeh, S.; Ranjbar Motlagh, S.; Mirbagheri, S.M.H.; Akhgar, J.M. Deformation behavior of AA2017-SiCp in warm and hot deformation regions. Mater. Des. 2015, 67, 318–323. [Google Scholar] [CrossRef]
- Wagner, S.; Härtel, M.; Frint, P.; Wagner, M.F.X. Influende of ECAP on the formability of a particle reinforced 2017 aluminum alloy. J. Phys. Conf. Ser. 2017, 755, 11001. [Google Scholar] [CrossRef]
- Xiang, G.F.; Zhang, Q.C.; Liu, H.W.; Wu, X.P.; Ju, X.Y. Time-resolved deformation measurements of the Portevin-Le Chatelier bands. Scr. Mater. 2007, 56, 721–724. [Google Scholar] [CrossRef]
- Zhang, Q.; Jiang, Z.; Jiang, H.; Chen, Z.; Wu, X. On the propagation and pulsation of Portevin-Le Chatelier deformation bands: An experimental study with digital speckle pattern metrology. Int. J. Plast. 2005, 21, 2150–2173. [Google Scholar] [CrossRef]
- Zhang, S.; McCormick, P.G.; Estrin, Y. The morphology of Portevin-Le Châtelier bands: Finite element simulation for Al-Mg-Si. Acta Mater. 2001, 49, 1087–1094. [Google Scholar] [CrossRef]
- Dierke, H.; Krawehl, F.; Graff, S.; Forest, S.; Šachl, J.; Neuhäuser, H. Portevin-LeChatelier effect in Al-Mg alloys: Influence of obstacles-experiments and modelling. Comput. Mater. Sci. 2007, 39, 106–112. [Google Scholar] [CrossRef]
- Chmelík, F.; Klose, F.B.; Dierke, H.; Šachl, J.; Neuhäuser, H.; Lukáč, P. Investigating the Portevin-Le Châtelier effect in strain rate and stress rate controlled tests by the acoustic emission and laser extensometry techniques. Mater. Sci. Eng. A 2007, 462, 53–60. [Google Scholar] [CrossRef]
- Liang, S.; Qing-Chuan, Z.; Peng-Tao, C. Influence of solute cloud and precipitates on spatiotemporal characteristics of Portevin-Le Chatelier effect in A2024 aluminum alloys. Chin. Phys. B 2009, 18, 3500–3507. [Google Scholar] [CrossRef]
- Lebedkina, T.A.; Lebyodkin, M.A. Effect of deformation geometry on the intermittent plastic flow associated with the Portevin-Le Chatelier effect. Acta Mater. 2008, 56, 5567–5574. [Google Scholar] [CrossRef]
- Grzegorczyk, B.; Ozgowicz, W.; Kalinowska-Ozgowicz, E.; Kowalski, A. Investigation of the Portevin-Le Chatelier effect by the acoustic emission. J. Achiev. Mater. Manuf. Eng. 2013, 60, 7–14. [Google Scholar]
- Cottrell, A.H. LXXXVI. A note on the Portevin-Le Chatelier effect. Lond. Edinb. Dublin Philos. Mag. J. Sci. 1953, 44, 829–832. [Google Scholar] [CrossRef]
- Robinson, J.M.; Shaw, M.P. Microstructural and mechanical influences on dynamic strain aging phenomena. Int. Mater. Rev. 1994, 39, 113–122. [Google Scholar] [CrossRef]
- Yilmaz, A. The Portevin-Le Chatelier effect: A review of experimental findings. Sci. Technol. Adv. Mater. 2011, 12, 063001. [Google Scholar] [CrossRef] [PubMed]
- Rodriguez, P.; Venkadesan, S. Serrated Plastic Flow Revisited. Solid State Phenom. 1995, 42–43, 257–266. [Google Scholar] [CrossRef]
- Rizzi, E.; Hähner, P. On the Portevin-Le Chatelier effect: Theoretical modeling and numerical results. Int. J. Plast. 2004, 20, 121–165. [Google Scholar] [CrossRef]
- Le Chatelier, A. Influence du temps et de la température sur les essais au choc. Rev. Met. Paris 1909, 6, 914–917. [Google Scholar] [CrossRef]
- Portevin, A.; Le Chatelier, F. Sur un phénomène observé lors de l’essai de traction d’alliages en cours de transformation. Comptes Rendus Acad. Sci. Paris 1923, 176, 507–510. [Google Scholar]
- Considère, M. Memoire sur l’emploi du fer et de l’acier dans les constructions. Ann. Ponts Chaussees 1885, 9, 574–775. [Google Scholar]
- Hähner, P. On the physics of the Portevin-Le Châtelier effect part 1: The statistics of dynamic strain ageing. Mater. Sci. Eng. A 1996, 207, 208–215. [Google Scholar] [CrossRef]
- Hähner, P. On the physics of the Portevin-Le Chgttelier effect part 2: From microscopic to macroscopic behaviour. Mater. Sci. Eng. A 1996, 207, 216–223. [Google Scholar] [CrossRef]
- Hähner, P.; Ziegenbein, A.; Rizzi, E.; Neuhäuser, H. Spatiotemporal analysis of Portevin–Le Châtelier deformation bands: Theory, simulation, and experiment. Phys. Rev. B 2002, 65, 134109. [Google Scholar] [CrossRef]
- Bross, S.; Hähner, P.; Steck, E.A. Mesoscopic simulations of dislocation motion in dynamic strain ageing alloys. Comput. Mater. Sci. 2003, 26, 46–55. [Google Scholar] [CrossRef]
- Kubin, L.P.; Estrin, Y. Evolution of dislocation densities and the critical conditions for the Portevin-Le Châtelier effect. Acta Metall. Mater. 1990, 38, 697–708. [Google Scholar] [CrossRef]
- Fritsch, S.; Scholze, M.; Wagner, M.F.X. Influence of thermally activated processes on the deformation behavior during low temperature ECAP. IOP Conf. Ser. Mater. Sci. Eng. 2016, 118, 012030. [Google Scholar] [CrossRef]
- Härtel, M.; Wagner, S.; Frint, P.; Wagner, M.F.X. Effects of particle reinforcement and ECAP on the precipitation kinetics of an Al-Cu alloy. IOP Conf. Ser. Mater. Sci. Eng. 2014, 63, 012080. [Google Scholar] [CrossRef]
- Härtel, M.; Frint, P.; Abstoss, K.G.; Wagner, M.F.-X. Effect of Creep and Aging on the Precipitation Kinetics of an Al-Cu Alloy after One Pass of ECAP. Adv. Eng. Mater. 2017, 1700307, 1700307. [Google Scholar] [CrossRef]
- Wagner, S.; Siebeck, S.; Hockauf, M.; Nestler, D.; Podlesak, H.; Wielage, B.; Wagner, M.F.X. Effect of SiC-reinforcement and equal-channel angular pressing on microstructure and mechanical properties of AA2017. Adv. Eng. Mater. 2012, 14, 388–393. [Google Scholar] [CrossRef]
- McCormick, P.G. Theory of flow localisation due to dynamic strain ageing. Acta Metall. 1988, 36, 3061–3067. [Google Scholar] [CrossRef]
- Balík, J.; Lukáč, P.; Kubin, L.P. Inverse critical strains for jerky flow in Al-Mg alloys. Scr. Mater. 2000, 42, 465–471. [Google Scholar] [CrossRef]
- Picu, R.C.; Vincze, G.; Ozturk, F.; Gracio, J.J.; Barlat, F.; Maniatty, A.M. Strain rate sensitivity of the commercial aluminum alloy AA5182-O. Mater. Sci. Eng. A 2005, 390, 334–343. [Google Scholar] [CrossRef]
- Schmahl, W.W.; Khalil-Allafi, J.; Hasse, B.; Wagner, M.; Heckmann, A.; Somsen, C. Investigation of the phase evolution in a super-elastic NiTi shape memory alloy (50.7 at.%Ni) under extensional load with synchrotron radiation. Mater. Sci. Eng. A 2004, 378, 81–85. [Google Scholar] [CrossRef]
- Cai, Y.; Zhang, Q.; Yang, S.; Fu, S.; Wang, Y. Experimental Study on Three-Dimensional Deformation Field of Portevin-Le Chatelier Effect Using Digital Image Correlation. Exp. Mech. 2016, 56, 1243–1255. [Google Scholar] [CrossRef]
- Schäfer, A.; Wagner, M.F.-X.; Pelegrina, J.L.; Olbricht, J.; Eggeler, G. Localization events and microstructural evolution in ultra fine grained NiTi shape memory alloys during thermo-mechanical loading. Adv. Eng. Mater. 2010, 12, 453–459. [Google Scholar] [CrossRef]
- Delpueyo, D.; Balandraud, X.; Grédiac, M. Calorimetric signature of the Portevin-Le Châtelier effect in an aluminum alloy from infrared thermography measurements and heat source reconstruction. Mater. Sci. Eng. A 2016, 651, 135–145. [Google Scholar] [CrossRef]
- Elibol, C.; Wagner, M.F.-X. Investigation of the stress-induced martensitic transformation in pseudoelastic NiTi under uniaxial tension, compression and compression-shear. Mater. Sci. Eng. A 2004, 621, 76–81. [Google Scholar] [CrossRef]
- Le Cam, J.-B.; Robin, E.; Leotoing, L.; Guines, D. Calorimetric analysis of Portevin-Le Chatelier bands under equibiaxial loading conditions in Al–Mg alloys: Kinematics and mechanical dissipation. Mech. Mater. 2017, 105, 80–88. [Google Scholar] [CrossRef]
- Zdunek, J.; Płowiec, J.; Spychalski, W.L.; Mizera, J. Acoustic emission studies of the Portevin-Le Chatelier effect in Al-Mg-Mn (5182) alloy. Inż. Mater. 2011, 32, 889–894. [Google Scholar]
- Chemelik, F.; Ziegenbein, A.; Neuhauser, H.; Lukac, P. Investigating the Portevin–Le Châtelier effect by the acoustic emission and laser extensometry techniques. Mater. Sci. Eng. A 2002, 324, 200–207. [Google Scholar] [CrossRef]
- Wevers, M. Listening to the sound of materials: Acoustic emission for the analysis of material behaviour. NDT E Int. 1997, 30, 99–106. [Google Scholar] [CrossRef]
- Estrin, Y.; Lebyodkin, M.A. The influence of dispersion particles on the Portevin-Le Chatelier effect: From average particle characteristics to particle arrangement. Mater. Sci. Eng. A 2004, 387–389, 195–198. [Google Scholar] [CrossRef]
- Thevenet, D.; Mliha-Touati, M.; Zeghloul, A. The effect of precipitation on the Portevin-Le Chatelier effect in an Al-Zn-Mg-Cu alloy. Mater. Sci. Eng. A 1999, 266, 175–182. [Google Scholar] [CrossRef]
- McCormick, P.G. A model for the Portevin-Le Chatelier effect in substitutional alloys. Acta Metall. 1972, 20, 351–354. [Google Scholar] [CrossRef]
- Beukel, A. Theory of the effect of dynamic strain aging on mechanical properties. Phys. Status Solidi 1975, 30, 197–206. [Google Scholar] [CrossRef]
- Mulford, R.A.; Kocks, U.F. New observations on the mechanisms of dynamic strain aging and of jerky flow. Acta Metall. 1979, 27, 1125–1134. [Google Scholar] [CrossRef]
- Lebyodkin, M.; Dunin-Barkowskii, L.; Bréchet, Y.; Estrin, Y.; Kubin, L.P. Spatio-temporal dynamics of the Portevin-Le Chatelier effect: Experiment and modelling. Acta Mater. 2000, 48, 2529–2541. [Google Scholar] [CrossRef]
- Staab, T.E.M.; Haaks, M.; Modrow, H. Early precipitation stages of aluminum alloys-The role of quenched-in vacancies. Appl. Surf. Sci. 2008, 255, 132–135. [Google Scholar] [CrossRef]
- Sauvage, X.; Wilde, G.; Divinski, S.V.; Horita, Z.; Valiev, R.Z. Grain boundaries in ultrafine grained materials processed by severe plastic deformation and related phenomena. Mater. Sci. Eng. A 2012, 540, 1–12. [Google Scholar] [CrossRef]
- Kim, W.J.; Chung, C.S.; Ma, D.S.; Hong, S.I.; Kim, H.K. Optimization of strength and ductility of 2024 Al by equal channel angular pressing (ECAP) and post-ECAP aging. Scr. Mater. 2003, 49, 333–338. [Google Scholar] [CrossRef]
- Divinski, S.V.; Reglitz, G.; Rösner, H.; Estrin, Y.; Wilde, G. Ultra-fast diffusion channels in pure Ni severely deformed by equal-channel angular pressing. Acta Mater. 2011, 59, 1974–1985. [Google Scholar] [CrossRef]
- Schwink, C.; Nortmann, A. The present experimental knowledge of dynamic strain ageing in binary f.c.c. solid solutions. Mater. Sci. Eng. A 1997, 234–236, 1–7. [Google Scholar] [CrossRef]
- Chihab, K.; Estrin, Y.; Kubin, L.P.; Vergnol, J. The kinetics of the Portevin-Le Chatelier bands in an Al-5at%Mg alloy. Scr. Metall. 1987, 21, 203–208. [Google Scholar] [CrossRef]
- Ling, C.P.; McCormick, P.G. Strain rate sensitivity and transient behaviour in an Al-Mg-Si alloy. Acta Metall. Mater. 1990, 38, 2631–2635. [Google Scholar] [CrossRef]








| Element | Cu | Mg | Mn | Fe | Si | Cr | Ni | Ti | Zn | Al |
|---|---|---|---|---|---|---|---|---|---|---|
| AA2017 Content in wt. % | 3.83 | 0.71 | 0.64 | 0.17 | 0.065 | 0.017 | 0.0049 | 0.0025 | <0.00100 | bal. |
| AA2017 (10 vol. % SiC) Content in wt. % | 3.81 | 0.68 | 0.49 | 0.27 | 8.26 | 0.010 | 0.016 | 0.016 | 0.11 |
| Strain Rate Jump in s−1 | 10−5 to 10−4 | 10−4 to 10−3 | 10−3 to 10−2 |
|---|---|---|---|
| True Strain in% | 4.61 | 5.62 | 7.68 |
| m base material | −0.0044 ± 0.0006 | −0.0083 ± 0.0006 | −0.0105 ± 0.0011 |
| m reinforced | −0.0231 ± 0.0004 | −0.0062 ± 0.0034 | −0.0060 ± 0.0001 |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Härtel, M.; Illgen, C.; Frint, P.; Wagner, M.F.-X. On the PLC Effect in a Particle Reinforced AA2017 Alloy. Metals 2018, 8, 88. https://doi.org/10.3390/met8020088
Härtel M, Illgen C, Frint P, Wagner MF-X. On the PLC Effect in a Particle Reinforced AA2017 Alloy. Metals. 2018; 8(2):88. https://doi.org/10.3390/met8020088
Chicago/Turabian StyleHärtel, Markus, Christian Illgen, Philipp Frint, and Martin Franz-Xaver Wagner. 2018. "On the PLC Effect in a Particle Reinforced AA2017 Alloy" Metals 8, no. 2: 88. https://doi.org/10.3390/met8020088
APA StyleHärtel, M., Illgen, C., Frint, P., & Wagner, M. F. -X. (2018). On the PLC Effect in a Particle Reinforced AA2017 Alloy. Metals, 8(2), 88. https://doi.org/10.3390/met8020088