Investigating the Apparent Seismic Diffusivity of Near-Receiver Geology at Mount St. Helens Volcano, USA
Abstract
:1. Introduction
2. Mount St. Helens Volcano, USA: Geological and Seismic Structure
3. Data and Method
3.1. “Drumbeat” Seismicity
3.2. Diffusion Model Theory
3.3. Data Processing
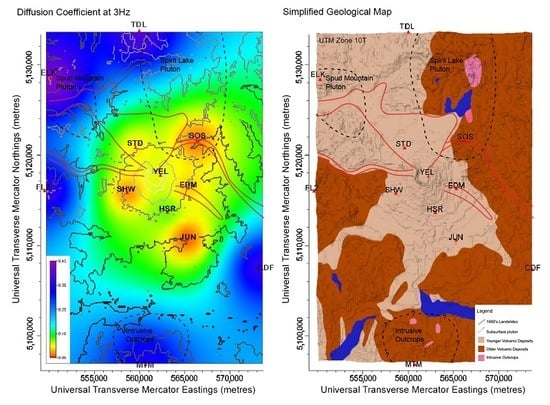
4. Results and Discussion
4.1. Diffusion
4.2. Attenuation
5. Conclusions
Supplementary Materials
Acknowledgments
Author Contributions
Conflicts of Interest
References
- Ferreira, A.M.G.; Woodhouse, J.H. Source, Path and receiver effects on seismic surface waves. Geophys. J. Int. 2007, 168, 109–132. [Google Scholar] [CrossRef]
- Shearer, P.M.; Orcutt, J.A. Surface and near-surface effects on seismic waves—Theory and borehole seismometer results. Bull. Seismol. Soc. Am. 1987, 77, 1168–1196. [Google Scholar]
- Neuberg, J.; O’Gorman, C. A Model of the Seismic Wavefield in Gas-Charged Magma: Application to Soufriere Hills Volcano, Montserrat; Memoirs 21.1; Geological Society of London: London, UK, 2002; pp. 603–609. [Google Scholar]
- Bean, C.; Lokmer, I.; O’Brien, G. Influence of near-surface volcanic structure on long-period seismic signals and on moment tensor inversions: Simulated examples from Mount Etna. J. Geophys. Res. Solid Earth 2008, 113. [Google Scholar] [CrossRef]
- Aki, K. Analysis of the seismic coda of local earthquakes as scattered waves. J. Geophys. Res. 1969, 74, 615–631. [Google Scholar] [CrossRef]
- Aki, K. Attenuation of shear-waves in the lithosphere for frequencies from 0.05 to 25 Hz. Phys. Earth Planet. Inter. 1980, 21, 50–60. [Google Scholar] [CrossRef]
- Sato, H.; Fehler, M.C. Seismic Wave Propagation and Scattering in the Heterogeneous Earth; Springer: Berlin, Germany, 1998. [Google Scholar]
- Wegler, U.; Lϋhr, B.-G. Scattering behaviour at Merapi volcano (Java) revealed from an active seismic experiment. Geophys. J. Int. 2001, 145, 579–592. [Google Scholar] [CrossRef]
- Wegler, U. Analysis of multiple scattering at Vesuvius volcano, Italy, using data of the TomoVes active seismic experiment. J. Volcanol. Geotherm. Res. 2003, 128, 45–63. [Google Scholar] [CrossRef]
- Sato, H.; Fehler, M.C.; Maeda, T. Seismic Wave Propagation and Scattering in the Heterogeneous Earth, 2nd ed.; Springer: New York, NY, USA, 2012; 494p. [Google Scholar]
- Mavko, G.; Mukerji, T.; Dvorkin, J. The Rock Physics Handbook: Tools for Seismic Analysis of Porous Media; Cambridge University Press: Cambridge, UK, 2009. [Google Scholar]
- De Siena, L.; Thomas, C.; Waite, G.P.; Moran, S.C.; Klemme, S. Attenuation and scattering tomography of the deep plumbing system of Mount St. Helens. J. Geophys. Res. 2014, 119, 8223–8238. [Google Scholar] [CrossRef]
- De Siena, L.; Calvet, M.; Watson, K.J.; Jonkers, A.R.T.; Thomas, C. Seismic scattering and absorption mapping of debris flows, feeding paths, and tectonic units at Mount St. Helens. Earth Planet. Sci. Lett. 2016, 442, 21–31. [Google Scholar] [CrossRef]
- Cheney, E.S. What is the age and extent of the Cascade Magmatic Arc? Wash. Geol. 1997, 25, 28–32. [Google Scholar]
- Waite, G.P.; Chouet, B.A.; Dawson, P.B. Eruption Dynamics at Mount St. Helens Imaged from Broadband Seismic Waveforms: Interaction of the Shallow Magmatic and Hydrothermal Systems. J. Geophys. Res. 2008. [Google Scholar] [CrossRef]
- Kiser, E.; Palomeras, I.; Levander, A.; Zelt, C.; Harder, S.; Schmandt, B.; Hansen, S.; Creager, K.; Ulberg, C. Magma reservoirs from the upper crust to the Moho inferred from high-resolution VP and Vs models beneath Mount St. Helens, Washington State, USA. Geology 2016, 44, 411–414. [Google Scholar] [CrossRef]
- Moran, S.C.; Lees, J.; Malone, S. P wave crustal velocity structure in the greater Mount Rainier area from local earthquake tomography. J. Geophys. Res. 1999, 104, 10775–10786. [Google Scholar] [CrossRef]
- Lees, J.M. The magma system of Mount St. Helens; non-linear high-resolution P wave tomography. J. Volcanol. Geotherm. Res. 1992, 53, 103–116. [Google Scholar] [CrossRef]
- Nolet, G. Seismic wave propagation and seismic tomography. In Seismic Tomography; Springer: Dordrecht, The Netherlands, 1987; pp. 1–23. [Google Scholar]
- Waite, G.P.; Moran, S.C. VP Structure of Mount St. Helens, Washington, USA, Imaged with Local Earthquake Tomography. J. Volcanol. Geotherm. Res. 2009, 182, 113–122. [Google Scholar] [CrossRef]
- Hill, G.J.; Caldwell, T.G.; Heise, W.; Chertkoff, D.G.; Bibby, H.M.; Burgess, M.K.; Cas, R.A. Distribution of melt beneath Mount St Helens and Mount Adams inferred from magnetotelluric data. Nat. Geosci. 2009, 2, 785–789. [Google Scholar] [CrossRef]
- Hansen, S.M.; Schmandt, B.; Levander, A.; Kiser, E.; Vidale, J.E.; Abers, G.A.; Creager, K.C. Seismic evidence for a cold serpentinized mantle wedge beneath Mount St Helens. Nat. Commun. 2016, 7, 13242. [Google Scholar] [CrossRef] [PubMed]
- Evarts, R.C.; Ashley, R.P.; Smith, J.G. Geology of the Mount St. Helens area: Record of discontinuous volcanic and plutonic activity in the Cascade Arc of southern Washington. J. Geophys. Res. 1987, 92, 10155–10169. [Google Scholar] [CrossRef]
- Poppeliers, C. The Effects of the Near-Surface Geology on P–S Strain Energy Partitioning of Diffusive Seismic Coda: Preliminary Observations and Results. Bull. Seismol. Soc. Am. 2015. [Google Scholar] [CrossRef]
- Huntting, M.T.; Bennett, W.A.G.; Livingston, V.E., Jr.; Moen, W.S. Geologic Map of Washington: Washington Division of Mines and Geology, Scale 1:500,000; Department of Natural Resources, Division of Geology and Earth Resources: Olympia, WA, USA, 1961. [Google Scholar]
- Vinciguerra, S.; Elsworth, D.; Malone, S. The 1980 pressure response and flank failure of Mount St. Helens (USA) inferred from seismic scaling exponents. J. Volcanol. Geotherm. Res. 2005, 144, 155–168. [Google Scholar] [CrossRef]
- Kendrick, J.E.; Lavallée, Y.; Hirose, T.; Di Toro, G.; Hornby, A.J.; De Angelis, S.; Dingwell, D.B. Volcanic drumbeat seismicity caused by stick-slip motion and magmatic frictional melting. Nat. Geosci. 2014, 7, 438–442. [Google Scholar] [CrossRef]
- Major, J.J.; Scott, W.E.; Driedger, C.; Dzurisin, D. Mount St. Helens Erupts Again; Activity from September 2004 through March 2005; U.S. Geological Survey: Reston, VA, USA, 2005; 4p.
- Dainty, A.M.; Toksōz, M.N. Seismic codas on the earth and the moon: A comparison. Phys. Earth Planet. Inter. 1981, 26, 250–260. [Google Scholar] [CrossRef]
- Galluzzo, D.; La Rocca, M.; Margerin, L.; Del Pezzo, E.; Scarpa, R. Attenuation and velocity structure from diffuse coda waves: Constraints from underground array data. Phys. Earth Planet. Inter. 2015, 240, 34–42. [Google Scholar] [CrossRef]
- Jing, Y.; Zeng, Y.; Lin, G. High-frequency seismogram envelope inversion using a multiple nonisotropic scattering model: Application to aftershocks of the 2008 wells earthquake. Bull. Seismol. Soc. Am. 2014, 104, 823–839. [Google Scholar] [CrossRef]
- Gusev, A.A.; Abubakirov, I.R. Simulated envelopes of non-isotropically scattered body waves as compared to observed ones: Another manifestation of fractal heterogeneity. Geophys. J. Int. 1996, 127, 49–60. [Google Scholar] [CrossRef]
- Calvet, M.; Margerin, L. Lapse-time dependence of coda Q: Anisotropic multiple-scattering models and application to the Pyrenees. Bull. Seismol. Soc. Am. 2013, 103, 1993–2010. [Google Scholar] [CrossRef]
- Gao, Y.; Zhang, N. Scattering cylindrical SH waves induced by a symmetrical V-shaped canyon: Near-source topographic effects. Geophys. J. Int. 2013, 193, 874–885. [Google Scholar] [CrossRef]
- Walder, J.S.; Schilling, S.P.; Sherrod, D.R.; Vallance, J.W. Evolution of Crater Glacier, Mount St. Helens, Washington, September 2006—November 2009; Open-File Report; U.S. Geological Survey: Reston, VA, USA, 2010; p. 34.
- Chaput, J.; Campillo, M.; Aster, R.C.; Roux, P.; Kyle, P.R.; Knox, H.; Czoski, P. Multiple scattering from icequakes at Erebus volcano, Antarctica: Implications for imaging at glaciated volcanoes. J. Geophys. Res. Solid Earth 2015, 120, 1129–1141. [Google Scholar] [CrossRef]
- De Siena, L.; Del Pezzo, E.; Thomas, C.; Curtis, A.; Margerin, L. Seismic energy envelopes in volcanic media: In need of boundary conditions. Geophys. J. Int. 2013, 195, 1102–1119. [Google Scholar] [CrossRef]
- Prudencio, J.; Ibáñez, J.; García-Yeguas, A.; Del Pezzo, E. Spatial distribution of intrinsic and scattering seismic attenuation in active volcanic islands, II: Deception island images. Geophys. J. Int. 2013, 195, 1957–1969. [Google Scholar] [CrossRef]
- Prudencio, J.; De Siena, L.; Ibáñez, J.; Del Pezzo, E.; García-Yeguas, A.; Díaz-Moreno, A. The 3D attenuation structure of Deception Island (Antarctica). Surv. Geophys. 2015, 36, 371–390. [Google Scholar] [CrossRef]
- Prudencio, J.; Del Pezzo, E.; Ibáñez, J.; Giampiccolo, E.; Patané, D. Two-dimensional seismic attenuation images of Stromboli Island using active data. Geophys. Res. Lett. 2015, 42. [Google Scholar] [CrossRef]
- Prudencio, J.; Aoki, Y.; Takeo, M.; Ibáñez, J.M.; Del Pezzo, E.; Song, W. Separation of scattering and intrinsic attenuation at Asama volcano (Japan): Evidence of high volcanic structural contrasts. J. Volcanol. Geotherm. Res. 2017, 333, 96–103. [Google Scholar] [CrossRef]
- Prudencio, J.; Taira, T.; Aoki, Y.; Aoyama, H.; Onizawa, S. Intrinsic and scattering attenuation images of Usu volcano, Japan. Bull. Volcanol. 2017, 79. [Google Scholar] [CrossRef]
- Parsons, T.; Blakely, R.J.; Brocher, T.M.; Christensen, N.I.; Fisher, M.A.; Flueh, E.; Kilbride, F.; Luetgert, J.H.; Miller, K.; ten Brink, U.S.; et al. Crustal structure of the Cascadia fore arc of Washington. In Earthquake Hazards of the Pacific Northwest Costal and Marine Regions; USGS Professional Paper; Kayen, R., Ed.; United States Geological Survey: Reston, VA, USA, 2005; Volume 1661D. [Google Scholar]













| Diffusion | 3 Hz | 6 Hz | 9 Hz | 12 Hz | 15 Hz | 18 Hz |
| On Volcano | ||||||
| EDM (7) | 0.081 ± 0.027 (5) | 0.066 ± 0.017 (3) | 0.164 ± 0.041 (5) | 0.274 ± 0.159 (5) | 0.501 ± 0.319 (4) | 0.105 ± 0.626 (5) |
| HSR (49) | 0.13 ± 0.074 (44) | 0.12 ± 0.039 (43) | 0.166 ± 0.02 (19) | 0.197 ± 0.045 (35) | 0.277 ± 0.034 (25) | 0.201 ± 0.528 (43) |
| JUN (79) | 0.056 ± 0.001 (13) | 0.083 ± 0.004 (14) | 0.132 ± 0.007 (17) | 0.146 ± 0.011 (23) | 0.146 ± 0.022 (42) | 0.24 ± 0.526 (78) |
| SHW (77) | 0.066 ± 0.001 (13) | 0.137 ± 0.025 (39) | 0.126 ± 0.004 (15) | 0.145 ± 0.007 (14) | 0.195 ± 0.035 (33) | 0.331 ± 0.115 (49) |
| SOS (56) | 0.062 ± 0.004 (14) | 0.053 ± 0.007 (10) | 0.136 ± 0.009 (19) | 0.101 ± 0.003 (12) | 0.102 ± 0.014 (23) | 0.176 ± 0.019 (25) |
| STD (75) | 0.091 ± 0.018 (56) | 0.126 ± 0.048 (59) | 0.174 ± 0.054 (66) | 0.209 ± 0.049 (39) | 0.144 ± 0.019 (32) | 0.184 ± 0.027 (31) |
| YEL (9) | 0.103 ± 0.028 (8) | 0.088 ± 0.332 (8) | 0.157 ± 0.045 (5) | 0.255 ± 0.08 (8) | 0.344 ± 0.017 (5) | 0.371 ± 0.044 (4) |
| Off Volcano | ||||||
| CDF (98) | 0.361 ± 0.025 (28) | 0.487 ± 0.043 (33) | 0.556 ± 0.045 (32) | 0.642 ± 0.084 (45) | 1.059 ± 0.171 (66) | 1.236 ± 0.26 (78) |
| ELK (74) | 0.433 ± 0.015 (15) | 0.345 ± 0.005 (10) | 0.767 ± 0.041 (20) | 0.576 ± 0.013 (13) | 0.679 ± 0.018 (14) | 1.126 ± 0.057 (18) |
| FL2 (87) | 0.367 ± 0.058 (37) | 0.568 ± 0.074 (35) | 0.599 ± 0.029 (22) | 0.793 ± 0.237 (86) | 0.77 ± 0.03 (16) | 1.434 ± 0.768 (85) |
| MTM (26) | 0.335 ± 0.018 (7) | 0.422 ± 0.028 (10) | 0.632 ± 0.014 (7) | 0.628 ± 0.01 (7) | 1.015 ± 0.039 (6) | 1.364 ± 0.127 (15) |
| TDL (81) | 0.389 ± 0.054 (50) | 0.369 ± 0.024 (28) | 0.605 ± 0.136 (80) | 0.584 ± 0.023 (22) | 0.945 ± 0.066 (30) | 1.568 ± 0.497 (71) |
| Attenuation | 3 Hz | 6 Hz | 9 Hz | 12 Hz | 15 Hz | 18 Hz |
| On Volcano | ||||||
| EDM (7) | 0.1 ± 0.03 (3) | 0.07 ± 0.059 (3) | 0.085 ± 0.026 (4) | −0.124 ± 0.066 (3) | −0.129 ± 0.009 (3) | −0.013 ± 0.042 (4) |
| HSR (49) | 0.155 ± 0.005 (6) | 0.105 ± 0.005 (6) | 0.11 ± 0.005 (7) | −0.002 ± 0.007 (11) | 0.04 ± 0.004 (6) | −0.01 ± 0.007 (6) |
| JUN (79) | 0.166 ± 0.003 (12) | 0.112 ± 0.013 (19) | 0.042 ± 0.003 (8) | 0.064 ± 0.003 (9) | 0.045 ± 0.003 (8) | 0.037 ± 0.004 (9) |
| SHW (77) | 0.108 ± 0.003 (8) | 0.021 ± 0.003 (9) | 0.024 ± 0.003 (6) | −0.018 ± 0.003 (6) | −0.078 ± 0.003 (7) | −0.109 ± 0.005 (8) |
| SOS (56) | 0.235 ± 0.008 (11) | 0.023 ± 0.005 (6) | 0.12 ± 0.009 (11) | 0.217 ± 0.007 (6) | 0.158 ± 0.007 (9) | 0.238 ± 0.007 (7) |
| STD (75) | 0.12 ± 0.004 (10) | 0.116 ± 0.003 (9) | 0.087 ± 0.003 (12) | 0.126 ± 0.003 (10) | 0.078 ± 0.004 (11) | 0.139 ± 0.006 (7) |
| YEL (9) | 0.179 ± 0.025 (3) | -0.004 ± 0.008 (3) | 0.132 ± 0.004 (5) | 0.006 ± 0.021 (3) | 0.023 ± 0.012 (5) | −0.17 ± 0.033 (4) |
| Off Volcano | ||||||
| CDF (98) | 0.174 ± 0.016 (26) | 0.092 ± 0.012 (26) | 0.133 ± 0.002 (9) | 0.095 ± 0.003 (8) | 0.041 ± 0.001 (8) | 0.054 ± 0.003 (8) |
| ELK (74) | 0.126 ± 0.004 (9) | 0.132 ± 0.001 (6) | 0.026 ± 0.002 (7) | 0.099 ± 0.019 (29) | 0.153 ± 0.007 (9) | 0.076 ± 0.004 (8) |
| FL2 (87) | 0.152 ± 0.002 (7) | 0.045 ± 0.002 (7) | 0.051 ± 0.004 (8) | 0.056 ± 0.003 (11) | 0.007 ± 0.003 (7) | 0.012 ± 0.004 (9) |
| MTM (26) | 0.123 ± 0.006 (5) | 0.093 ± 0.006 (6) | −0.069 ± 0.012 (6) | 0.044 ± 0.011 (6) | −0.104 ± 0.007 (5) | 0.027 ± 0.013 (7) |
| TDL (81) | 0.154 ± 0.003 (10) | 0.155 ± 0.002 (6) | 0.145 ± 0.002 (7) | 0.163 ± 0.011 (18) | 0.108 ± 0.003 (9) | −0.019 ± 0.004 (7) |
| Station | CDF | EDM | ELK | FL2 | HSR | JUN | MTM | SHW | SOS | STD | TDL | YEL |
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Diffusion | 0.56 | 0.16 | 0.77 | 0.6 | 0.16 | 0.13 | 0.63 | 0.13 | 0.14 | 0.17 | 0.6 | 0.16 |
| r (km) | 10 | 4 | 10 | 10 | 4 | 4 | 10 | 4 | 4 | 4 | 10 | 4 |
| Level of Fit | 98% | 95% | 90% | 98% | 73% | 98% | 98% | 95% | 95% | 99% | 90% | 95% |
| Station | CDF | EDM | ELK | FL2 | HSR | JUN | MTM | SHW | SOS | STD | TDL | YEL |
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Diffusion | 0.89 | 0.26 | 1.2 | 0.36 | 0.3 | 0.2 | 0.85 | 0.21 | 0.12 | 0.29 | 0.57 | 0.16 |
| r (km) | 12.6 | 4 | 11.8 | 10.8 | 4.5 | 5 | 11.6 | 5.1 | 4.5 | 5.2 | 11.8 | 4 |
| Level of Fit | 99% | 96% | 90% | 99% | 74% | 96% | 99% | 96% | 99% | 99% | 90% | 95% |
© 2017 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
King, T.; Benson, P.; De Siena, L.; Vinciguerra, S. Investigating the Apparent Seismic Diffusivity of Near-Receiver Geology at Mount St. Helens Volcano, USA. Geosciences 2017, 7, 130. https://doi.org/10.3390/geosciences7040130
King T, Benson P, De Siena L, Vinciguerra S. Investigating the Apparent Seismic Diffusivity of Near-Receiver Geology at Mount St. Helens Volcano, USA. Geosciences. 2017; 7(4):130. https://doi.org/10.3390/geosciences7040130
Chicago/Turabian StyleKing, Thomas, Philip Benson, Luca De Siena, and Sergio Vinciguerra. 2017. "Investigating the Apparent Seismic Diffusivity of Near-Receiver Geology at Mount St. Helens Volcano, USA" Geosciences 7, no. 4: 130. https://doi.org/10.3390/geosciences7040130
APA StyleKing, T., Benson, P., De Siena, L., & Vinciguerra, S. (2017). Investigating the Apparent Seismic Diffusivity of Near-Receiver Geology at Mount St. Helens Volcano, USA. Geosciences, 7(4), 130. https://doi.org/10.3390/geosciences7040130