Interleaved Boost Converter with ZVT-ZCT for the Main Switches and ZCS for the Auxiliary Switch
Abstract
:1. Introduction
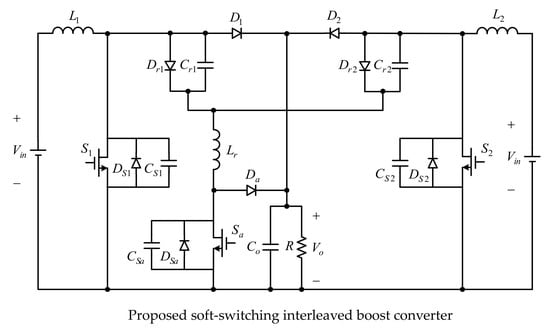
2. Two-Phase Converter with Proposed Soft Switching
3. Basic Operating Principles
4. Proposed Control Strategy
5. Design of the Key Components
5.1. Design of L1 and L2
5.2. Design of Co
5.3. Design of Lr, Cr1 and Cr2
6. Experimental Results
7. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Lee, F.C. High-frequency quasi-resonant and multi-resonant converter technologies. In Proceedings of the 14 Annual Conference of Industrial Electronics Society, Singapore, 24–28 October 1988; pp. 509–521. [Google Scholar]
- Chuang, Y.; Ke, Y. A novel high-efficiency battery charger with a buck zero-voltage-switching resonant converter. IEEE Trans. Energy Convers. 2007, 22, 848–854. [Google Scholar] [CrossRef]
- Mousavi, A.; Das, P.; Moschopoulos, G. A comparative study of a New ZCS DC-DC full-bridge boost converter with a ZVS active-clamp converter. IEEE Trans. Power Electron. 2012, 27, 1347–1358. [Google Scholar] [CrossRef]
- Ge, T.; Carpenter, B.; Ngo, K.D.T. Resonant cross-commutated Dc-Dc converter. IEEE Trans. Ind. Electron. 2017, 64, 8782–8785. [Google Scholar] [CrossRef]
- Hong, J.; Deng, X.; Zhang, G.; Huang, Z.; Li, X.; Zhang, Y. Sneak circuit identification of an improved boost converter with soft-switching realization. IEEE J. Emerg. Sel. Top. Power Electron. 2019, 7, 2394–2402. [Google Scholar] [CrossRef]
- Lin, B.; Hsieh, F. Soft-switching zeta-flyback converter with a buck-boost type of active clamp. IEEE Trans. Ind. Electron. 2007, 54, 2813–2822. [Google Scholar]
- Han, S.K.; Yoon, H.K.; Moon, G.W.; Youn, M.J.; Kim, Y.H.; Lee, K.H. A new active clamping zero-voltage switching PWM current-fed half-bridge converter. IEEE Trans. Power Electron. 2005, 20, 1271–1279. [Google Scholar] [CrossRef]
- Lee, Y.; Lin, B. Adding active clamping and soft switching to boost-flyback single-stage isolated power-factor-corrected power supplies. IEEE Trans. Power Electron. 1997, 12, 1017–1027. [Google Scholar]
- Watson, R.; Hua, G.C.; Lee, F.C. Characterization of an active clamp flyback topology for power factor correction applications. IEEE Trans. Power Electron. 1996, 11, 191–198. [Google Scholar] [CrossRef]
- Tuomainen, V.; Kyyra, J. Effect of resonant transition on efficiency of forward converter with active clamp and self-driven SRs. IEEE Trans. Power Electron. 2005, 20, 315–323. [Google Scholar] [CrossRef]
- Zhao, Q.; Lee, F.C. High-efficiency, high step-up DC-DC converters. IEEE Trans. Power Electron. 2003, 18, 65–73. [Google Scholar] [CrossRef] [Green Version]
- Lin, B.-R.; Huang, C.E.; Wang, D. Analysis and implementation of a zero-voltage switching forward converter with a synchronous rectifier. IEE Proc. Electr. Power Appl. 2005, 152, 1085–1092. [Google Scholar] [CrossRef]
- Lo, Y.; Lin, J. Active-clamping ZVS flyback converter employing two transformers. IEEE Trans. Power Electron. 2007, 22, 2416–2423. [Google Scholar] [CrossRef]
- Watson, R.; Lee, F.C.; Hua, G.C. Utilization of an active-clamp circuit to achieve soft switching in flyback converters. IEEE Trans. Power Electron. 1996, 11, 162–169. [Google Scholar] [CrossRef]
- Wu, T.; Lai, Y.; Hung, J.; Chen, Y. Boost converter with coupled inductors and buck-boost type of active clamp. IEEE Trans. Ind. Electron. 2008, 55, 154–162. [Google Scholar] [CrossRef]
- Xinke, W.; Junming, Z.; Xin, Y.; Zhaoming, Q. Analysis and design for a new ZVS DC-DC converter with active clamping. IEEE Trans. Power Electron. 2006, 21, 1572–1579. [Google Scholar]
- Suryawanshi, H.M.; Ramteke, M.R.; Thakre, K.L.; Borghate, V.B. Unity-power-factor operation of three-phase AC-DC soft switched converter based on boost active clamp topology in modular approach. IEEE Trans. Power Electron. 2008, 23, 229–236. [Google Scholar] [CrossRef]
- Duarte, C.M.C.; Fiori, V.M. A new ZVS-PWM active-clamping buck-boost converter. In Proceedings of the IEEE PESC’05, Recife, Brazil, 16–16 June 2005; pp. 1429–1433. [Google Scholar]
- Ma, Y.; Wu, X.; Xie, X.; Chen, G.; Qian, Z. A new ZVS-PWM buck converter with an active camping cell. In Proceedings of the IEEE IECON’07, Taipei, Taiwan, 5–8 November 2007; pp. 1592–1597. [Google Scholar]
- Lin, B.; Huang, C.; Chiang, H. Analysis of a soft switching PWM active clamp Cuk converter. In Proceedings of the IEEE ICIEA’07, Harbin, China, 23–25 May 2007; pp. 2311–2316. [Google Scholar]
- Tseng, C.; Chen, C. A novel ZVT PWM Cuk power-factor corrector. IEEE Trans. Ind. Electron. 1999, 46, 780–787. [Google Scholar] [CrossRef]
- Hua, G.; Leu, C.; Jiang, Y.; Lee, F.C.Y. Novel zero-voltage-transition PWM converters. IEEE Trans. Power Electron. 1994, 9, 213–219. [Google Scholar]
- Tseng, C.; Chen, C. Novel ZVT-PWM converters with active snubbers. IEEE Trans. Power Electron. 1998, 13, 861–869. [Google Scholar] [CrossRef] [Green Version]
- Cho, J.; Baek, J.; Yoo, D.; Lee, H. Reduced conduction loss zero-voltage-transition power factor correction converter with low cost. IEEE Trans. Ind. Electron. 1998, 45, 395–400. [Google Scholar]
- Martins, M.L.d.S.; Hey, H.L. Self-commutated auxiliary circuit ZVT PWM converters. IEEE Trans. Power Electron. 2004, 19, 1435–1445. [Google Scholar] [CrossRef]
- Li, W.; He, X. ZVT interleaved boost converters for high-efficiency, high step-up DC-DC conversion. IET Electr. Power Appl. 2007, 1, 284–290. [Google Scholar] [CrossRef]
- Wang, D.; He, X.; Zhao, R. ZVT interleaved boost converters with built-in voltage doubler and current auto-balance characteristic. IEEE Trans. Power Electron. 2008, 23, 2847–2854. [Google Scholar] [CrossRef]
- Das, P.; Moschopoulos, G. A comparative study of zero-current-transition PWM converters. IEEE Trans. Ind. Electron. 2007, 54, 1319–1328. [Google Scholar] [CrossRef]
- Bodur, H.; Bakan, A.F. An improved ZCT-PWM DC-DC converter for high-power and frequency applications. IEEE Trans. Ind. Electron. 2004, 51, 89–98. [Google Scholar] [CrossRef]
- Adib, E.; Farzaneh-fard, H. New zero current transition PWM converters. In Proceedings of the IEEE ICIT’06, Mumbai, India, 15–17 December 2006; pp. 2131–2136. [Google Scholar]
- Duarte, C.M.C.; Barbi, I. A family of ZVS-PWM active-clamping DC-to-DC converters: Synthesis, analysis, design, and experimentation. IEEE Trans. Circuits Syst. I Fundam. Theory Appl. 1997, 44, 698–704. [Google Scholar] [CrossRef]
- Kumar, A.P.; Subrahmanya Kumar Bhajana, V.V.; Drabek, P. A novel ZVT/ZCT bidirectional DC-DC converter for energy storage applications. In Proceedings of the IEEE SPEEDAM’16, Anacapri, Italy, 22–24 June 2016; pp. 979–983. [Google Scholar]
- De, O.; Stein, C.M.; Hey, H.L. A true ZCZVT commutation cell for PWM converters. IEEE Trans. Power Electron. 2000, 15, 185–193. [Google Scholar]
- Hu, Z.; Zhang, B.; Deng, W. Study on novel ZVZCT PWM DC-DC converter family. In Proceedings of the IEEE IPEMC’04, Xi’an, China, 14–16 August 2004; pp. 154–159. [Google Scholar]
- Cho, J.; Baek, J.; Rim, G.; Kang, I. Novel zero-voltage-transition PWM multiphase converters. IEEE Trans. Power Electron. 1998, 13, 152–159. [Google Scholar]
- Tseng, S.-Y.; Shiang, J.-Z.; Chang, H.H.; Jwo, W.-S.; Hsieh, C.-T. A novel turn-on/off snubber for interleaved boost converters. In Proceedings of the IEEE PESC’07, Qrlando, FL, USA, 17–21 June 2007; pp. 2341–2347. [Google Scholar]
- Hwu, K.I.; Tu, W.C.; Hon, M.J. A dimmable LED driver based on current balancing transformer with magnetizing energy recycling considered. IEEE J. Display Technol. 2014, 10, 388–395. [Google Scholar] [CrossRef]
- Hwu, K.I.; Jiang, W.Z.; Hsiao, C.W. Dimmable LED driver based on twin-bus converter and differential-mode transformer. IEEE J. Disp. Technol. 2016, 12, 1122–1129. [Google Scholar] [CrossRef]
- Qu, X.; Wong, S.C.; Chi, K.T. An improved LCLC current-source-output multistring LED driver with capacitive current balancing. IEEE Trans. Power Electron. 2015, 30, 5783–5791. [Google Scholar] [CrossRef]
- Ye, Y.; Eric Cheng, K.W.; Lin, J.; Wang, D. Single-switch multichannel current-balancing LED drive circuits based on optimized SC techniques. IEEE Trans. Ind. Electron. 2015, 62, 4761–4768. [Google Scholar] [CrossRef]
- Chiub, C.; Chen, K. A high accuracy current-balanced control technique for LED backlight. In Proceedings of the IEEE PESC’08, Rhodes, Greece, 15–19 June 2008; pp. 4202–4206. [Google Scholar]
- Yu, W.; Lai, J.; Ma, H.; Zheng, C. High-Efficiency dc-dc converter with twin bus for dimmable LED lighting. IEEE Trans. Power Electron. 2011, 26, 2095–2100. [Google Scholar] [CrossRef]
- Lohaus, L.; Liao, L.; Strache, S.; Wunderlich, R.; Heinen, S. Energy efficient current control technique for driving high power LEDs. In Proceedings of the VDE PRIME’12, Aachen, Germany, 12–15 June 2012; pp. 75–78. [Google Scholar]
- Li, S.N.; Zhong, W.X.; Chen, W.; Hui, R.S.Y. Novel self-configurable current-mirror techniques for reducing current imbalance in parallel light-emitting diode (LED) strings. IEEE Trans. Power Electron. 2012, 27, 2153–2162. [Google Scholar] [CrossRef] [Green Version]
- Li, Y.; Chen, C. A novel primary-side regulation scheme for single-stage high-power-factor ac-dc LED driving circuit. IEEE Trans. Ind. Electron. 2013, 60, 4978–4986. [Google Scholar] [CrossRef]





























| System Parameters | Specifications |
|---|---|
| Operating mode | CCM |
| Input voltage (Vin) | |
| Output voltage (Vo) | 42 V |
| Rated output current (Io,rated) | 6 A |
| Minimum output current (Io,min) | 0.3 A |
| Switching frequency (fs) | 25 kHz |
| Components | Specifications |
|---|---|
| Input Inductor for the first phase (L1) | 720 μH |
| Input Inductor for the second phase (L2) | 720 μH |
| Output capacitor (Co) | 680 μF |
| Resonant inductor (Lr) | 6 μH |
| Resonant capacitor for the first phase (Cr1) | 220 μF |
| Resonant capacitor for the second phase (Cr2) | 220 μF |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hwu, K.-I.; Shieh, J.-J.; Jiang, W.-Z. Interleaved Boost Converter with ZVT-ZCT for the Main Switches and ZCS for the Auxiliary Switch. Appl. Sci. 2020, 10, 2033. https://doi.org/10.3390/app10062033
Hwu K-I, Shieh J-J, Jiang W-Z. Interleaved Boost Converter with ZVT-ZCT for the Main Switches and ZCS for the Auxiliary Switch. Applied Sciences. 2020; 10(6):2033. https://doi.org/10.3390/app10062033
Chicago/Turabian StyleHwu, Kuo-Ing, Jenn-Jong Shieh, and Wen-Zhuang Jiang. 2020. "Interleaved Boost Converter with ZVT-ZCT for the Main Switches and ZCS for the Auxiliary Switch" Applied Sciences 10, no. 6: 2033. https://doi.org/10.3390/app10062033
APA StyleHwu, K. -I., Shieh, J. -J., & Jiang, W. -Z. (2020). Interleaved Boost Converter with ZVT-ZCT for the Main Switches and ZCS for the Auxiliary Switch. Applied Sciences, 10(6), 2033. https://doi.org/10.3390/app10062033