Figure 1.
Time series of hourly (a) wind speed, (b) wind direction, (c) air temperature, (d) relative humidity and (e) air pressure obtained by the manned weather station at Zhongshan Station, Antarctica.
Figure 1.
Time series of hourly (a) wind speed, (b) wind direction, (c) air temperature, (d) relative humidity and (e) air pressure obtained by the manned weather station at Zhongshan Station, Antarctica.
Figure 2.
The monthly meteorological data of Zhongshan Station. (a) wind speed, (b) solar radiation and day length, (c) air temperature.
Figure 2.
The monthly meteorological data of Zhongshan Station. (a) wind speed, (b) solar radiation and day length, (c) air temperature.
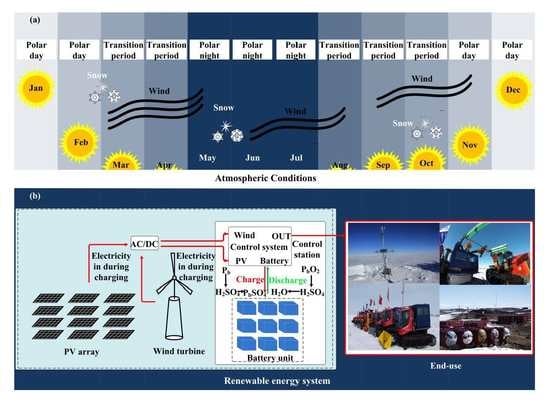
Figure 3.
(a) Atmospheric conditions, (b) Schematic of the renewable energy system.
Figure 3.
(a) Atmospheric conditions, (b) Schematic of the renewable energy system.
Figure 4.
Equivalent circuit of the solar cell.
Figure 4.
Equivalent circuit of the solar cell.
Figure 5.
(a–f) show the relationship between battery voltage and battery capacity at −50 °C, −40 °C, −30 °C, −20 °C, −10 °C, 0 °C, respectively.
Figure 5.
(a–f) show the relationship between battery voltage and battery capacity at −50 °C, −40 °C, −30 °C, −20 °C, −10 °C, 0 °C, respectively.
Figure 6.
(a–g) show the coefficients a(Ti), b(Ti), c(Ti), d(Ti), e(Ti), f(Ti) and g(Ti) from −50 °C to 0 °C, respectively.
Figure 6.
(a–g) show the coefficients a(Ti), b(Ti), c(Ti), d(Ti), e(Ti), f(Ti) and g(Ti) from −50 °C to 0 °C, respectively.
Figure 7.
The flowchart of the temperature control strategy.
Figure 7.
The flowchart of the temperature control strategy.
Figure 8.
The simulation results of (a) the wind speed, (b) wind turbine output power and (c) rotational speed of wind turbine.
Figure 8.
The simulation results of (a) the wind speed, (b) wind turbine output power and (c) rotational speed of wind turbine.
Figure 9.
The simulation results of (a) the wind speed, (b) wind turbine output power and (c) rotational speed of wind turbine at abrupt wind speeds.
Figure 9.
The simulation results of (a) the wind speed, (b) wind turbine output power and (c) rotational speed of wind turbine at abrupt wind speeds.
Figure 10.
The flowchart of the perturbation and observation method with adaptive variable step.
Figure 10.
The flowchart of the perturbation and observation method with adaptive variable step.
Figure 11.
The flowchart of the power supply strategy.
Figure 11.
The flowchart of the power supply strategy.
Figure 12.
Block diagram of the standalone renewable energy system by hybrid solar–wind system.
Figure 12.
Block diagram of the standalone renewable energy system by hybrid solar–wind system.
Figure 13.
The DC/DC conversion circuit and power supply circuit.
Figure 13.
The DC/DC conversion circuit and power supply circuit.
Figure 14.
The solar/wind charging circuit.
Figure 14.
The solar/wind charging circuit.
Figure 15.
Actual monthly load power, air temperature and fuel consumption in Zhongshan Station.
Figure 15.
Actual monthly load power, air temperature and fuel consumption in Zhongshan Station.
Figure 16.
The power generation of the standalone renewable energy system in Zhongshan Station from 2014 to 2015.
Figure 16.
The power generation of the standalone renewable energy system in Zhongshan Station from 2014 to 2015.
Table 1.
Sensor information of the manned weather station.
Table 1.
Sensor information of the manned weather station.
| Sensor Name | Performance | Sensor Model |
|---|
| Wind speed | Temperature range: −60–30 °C Accuracy: 0.5 m/s | Wind Monitor Model 05103-45, R.M.Young, Traverse City, MI, USA |
| Wind direction | Temperature range: −60–30 °C Accuracy: 0.3° | Wind Monitor Model 05103-45, R.M.Young, Traverse City, MI, USA |
| Air temperature | Temperature range: −60–10 °C Accuracy: 0.1 °C | HMP155A, Vaisala, Vantaa, Finland |
| Air humidity | Temperature range: −60–10 °C Accuracy: 2%RH | HMP155A, Vaisala, Vantaa, Finland |
| Atmospheric pressure | Temperature range: −60–30 °C Accuracy: 0.6 hPa | PTB110, Vaisala, Vantaa, Finland |
Table 2.
Advantages and disadvantages of different silicon solar cells.
Table 2.
Advantages and disadvantages of different silicon solar cells.
| Material Name | Advantages | Disadvantages |
|---|
| Monocrystalline silicon | High conversion efficiency, mature technology and small footprint | Expensive and high requirement for incident angle of sunlight |
| Polysilicon | The conversion efficiency is higher than that of amorphous silicon, the manufacturing cost is lower than that of monocrystalline silicon, and the low requirement for incident angle of sunlight | Conversion efficiency is lower than monocrystalline silicon, and the process is complicated |
| Amorphous silicon | Minimum requirement for incident angle of sunlight and high acceptance rate of astigmatism | Low conversion efficiency |
Table 3.
Key specifications of the photovoltaic (PV) panels.
Table 3.
Key specifications of the photovoltaic (PV) panels.
| Characteristics | Value | Unit |
|---|
| Open circuit voltage (Voc) | 42.64 | V |
| Optimum operating voltage (Vmp) | 34.96 | V |
| Short circuit current (Isc) | 9.48 | A |
| Optimum operating current (Imp) | 8.59 | A |
| Maximum power at STC 1 (Pmax) | 300 | W |
| Operating temperature | −50 to 85 | °C |
| Size | 1956 × 992 × 50 | mm |
Table 4.
Key specifications of the wind turbine.
Table 4.
Key specifications of the wind turbine.
| Characteristics | Value | Unit |
|---|
| Cut in speed | 2.5 | m/s |
| Rated wind speed | 10 | m/s |
| Cut off speed | 45 | m/s |
| Rated power | 10 | KW |
| Peak power | 12 | KW |
| Diameter of impeller | 7.8 | m |
| Operating temperature | −50 to 85 | °C |
| Number of blades | 3 | |
| Generator type | Three-phase AC permanent magnet generator | |
| Blade material | Reinforced glass steel | |
Table 5.
Key performance comparisons of each battery.
Table 5.
Key performance comparisons of each battery.
| Classification | Electrolyte | Principle | Operating Temperature (°C) |
|---|
| Lead–acid batteries | Dilute sulfuric acid | Oxidation-reduction reaction | −50 to 70 |
| Lithium–ion batteries | Organic lithium salt electrolyte | Ion migration | −50 to 70 |
| Nickel–cadmium batteries | Potassium hydroxide aqueous solution | Oxidation-reduction reaction | −20 to 45 |
| Nickel–hydrogen batteries | Potassium hydroxide aqueous solution | Oxidation-reduction reaction | −20 to 60 |
| Sodium–sulfur batteries | Na-β-Al2O3 | Chemical reaction | 300 to 350 |
Table 6.
Key specifications of the low-temperature test chamber.
Table 6.
Key specifications of the low-temperature test chamber.
| Characteristics | Value | Unit |
|---|
| Rated power | 668 | W |
| Rated voltage | 220 | V |
| Temperature range | −60 to 86 | °C |
| Temperature adjustment accuracy | 0.1 | °C |
| Effective volume | 340 | L |
| Noise level | 52 | dB |
Table 7.
Parameters of the transfer function.
Table 7.
Parameters of the transfer function.
| Parameter | Value |
|---|
| k | 0.8 |
| T | 48.75 |
| τ | 11.2 |
Table 8.
Terminal names and general descriptions of the electrical interface of the control system.
Table 8.
Terminal names and general descriptions of the electrical interface of the control system.
| Terminal Name | Description | Direction |
|---|
| P6.7 | Voltage monitoring | Input |
| P7.5 | Current monitoring | Input |
| P6.0 | Battery condition monitoring | Input |
| P6.1 | Wind turbine unloading | Output |
| P1.1, P1.2, P4.5, P4.6 | PWM control | Output |
| P4.0, P4.1, P4.2, P4.3 | PWM drive | Output |
Table 9.
Relevant parameters of the DC/DC conversion circuit.
Table 9.
Relevant parameters of the DC/DC conversion circuit.
| Parameter | Value | Unit |
|---|
| C1 | 1 | μF |
| C2 | 1 | μF |
| C3 | 3.3 | nF |
| C4 | 0.1 | μF |
| C5 | 10 | μF |
| R1 | 13 | KΩ |
| R2 | 18 | KΩ |
| R3 | 156 | KΩ |
| L1 | 3.3 | μH |
Table 10.
Relevant parameters of the power supply circuit.
Table 10.
Relevant parameters of the power supply circuit.
| Parameter | Value | Unit |
|---|
| C6 | 100 | μF |
| C7 | 0.1 | μF |
| C8 | 0.1 | μF |
| C9 | 100 | μF |
| C10 | 0.1 | μF |
| C11 | 0.1 | μF |
| C12 | 0.47 | μF |
Table 11.
Relevant parameters of the solar/wind charging circuit.
Table 11.
Relevant parameters of the solar/wind charging circuit.
| Parameter | Value | Unit |
|---|
| C13 | 470 | μF |
| C14 | 330 | μF |
| C15 | 100 | pF |
| C16 | 100 | pF |
| C17 | 0.1 | μF |
| C18 | 0.1 | μF |
| C19 | 0.1 | μF |
| R4 | 30 | KΩ |
| R5 | 15 | KΩ |
| R6 | 15 | KΩ |
| R7 | 30 | KΩ |
| R8 | 30 | KΩ |
| L2 | 100 | mH |
| L3 | 100 | mH |
Table 12.
Monthly fuel consumption of Zhongshan Station.
Table 12.
Monthly fuel consumption of Zhongshan Station.
| | Jan | Feb | Mar | Apr | May | Jun | Jul | Aug | Sep | Oct | Nov | Dec |
|---|
| Fuel consumption(t) | 18.7 | 18.4 | 19.31 | 19.81 | 21.7 | 21.5 | 22.5 | 21.83 | 20.46 | 20.12 | 17.9 | 19.1 |