Experimental and Theoretical Analysis of Lead Pb2+ and Cd2+ Retention from a Single Salt Using a Hollow Fiber PES Membrane
Abstract
:1. Introduction
2. Experimental Work
2.1. Materials and Methods
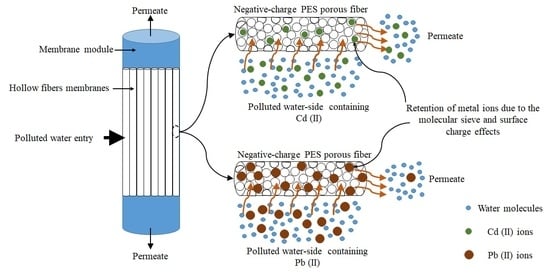
2.2. Membrane Filtration and Heavy Metal Rejection
2.3. Models of Membrane Transport
2.3.1. Film Theory
2.3.2. Combined Film Theory/Solution-Diffusion Model (CFSD)
2.3.3. Combined Film Theory-Spiegler-Kedem Model (CFSK)
2.3.4. Combined Film Theory-Finely-Porous Model (CFFP)
2.3.5. Concentration Polarization Model (CPM) and the Enrichment Factor (Eo)
2.3.6. Comparison of the Experimental Results (S2) and Model Predictions
2.3.7. Calculation of the Péclet Number (Pe)
3. Results and Discussion
3.1. Effect of the Feed pH on the Membrane Performance
3.2. Effect of Heavy Metal Concentration on the PES Hollow Fiber Performance
3.3. Estimating Parameters for the Membrane and Coefficient of Mass Transfer
3.4. Estimation of the Concentration Polarization Model (CPM), Enrichment Factors (Eo and E), and Péclet Number (Pe)
3.5. Comparative Study of PES Membranes
4. Conclusions
Author Contributions
Funding
Conflicts of Interest
Nomenclature
| A | Membrane surface area, m2 |
| bf | Factor measure of friction between the solute molecules and the membrane pore wall, calculated from bf = 1 + fsm/ fsw |
| C | Solute concentration in the boundary layer, g/m3 |
| Cb | Average bulk concentration, g/m3 |
| Cf | Concentration of solute in the feed, g/m3 |
| CFFP | Combined film theory-finely-porous model |
| CFSD | Combined film theory-solution-diffusion model |
| CFSK | Combined film theory-Spiegler-Kedem model |
| Cm | Solute concentration at the membrane surface/water (solvent) interface, g/m3 |
| CP | Concentration Polarization |
| Cp | Concentration of solute in permeate, g/m3 |
| CPM | Concentration Polarization Model |
| Cr | Concentration of solute in retentate, g/m3 |
| Dam K/ | Solute transport parameter, cm/s |
| D | Diffusion coefficient, cm2/s |
| Dab | Diffusivity of solute a in solvent b, cm2/s |
| Dam | Diffusivity of salt a on surface membrane, cm2/s |
| Eo | Enrichment Factor, known as Cp/Cm |
| F | Flow parameter defined in Equation (15) |
| fsm | Friction coefficient between solute and membrane |
| fsw | Friction coefficient between solute and solvent (water) |
| JS | Solute flux through membrane, m3/m2s |
| Jv | Convective + Diffusive mass transfer rate, m3/m2s |
| K | Solute partition coefficient |
| k | Mass transfer coefficient expressed as |
| Pe | Péclet number (a dimensionless number) |
| PM | Salt permeability, L/m2·h |
| Ps | Overall permeability coefficient |
| PWP | Pure water permeability |
| R | True solute rejections |
| Rexp | Experimental rejection |
| Ro | Observed rejection |
| Rth | Theoretical rejection |
| t | Collected permeate time, h |
| TMP | Transmembrane pressure, bar |
| V | Permeate volume, L |
| x | Distance from the membrane layer, m |
Greek Letters
| Membrane void fraction | |
| Tortuosity | |
| Layer thickness; thickness of the boundary layer, m | |
| Effective membrane thickness | |
| Transmembrane pressure, bar | |
| Osmotic pressure difference, bar |
References
- Al-Rashdi, B.; Somerfield, C.; Hilal, N. Heavy Metals Removal Using Adsorption and Nanofiltration Techniques. Sep. Purif. Rev. 2011, 40, 209–259. [Google Scholar] [CrossRef]
- Moore, J.W.; Ramamoorthy, S. Heavy Metals in Natural Waters; Springer: New York, NY, USA, 1985; pp. 112–119. [Google Scholar]
- Fane, J.A.G. Membranes for water production and wastewater reuse. Desalination 1996, 106, 1–9. [Google Scholar] [CrossRef]
- Liu, J.; Chen, H.; Mao, X.; Jin, X. Determination of trace copper, lead, cadmium, and iron in environmental and biological samples by flame atomic absorption spectroscopy coupled to flow injection on-line coprecipitation pre-concentration using DDTC-nickel as coprecipitate carrier. Int. J. Environ. Anal. Chem. 2000, 76, 267–282. [Google Scholar] [CrossRef]
- Zhou, P.; Huang, J.-C.; Li, A.W.; Wei, S. Heavy metal removal from wastewater in fluidized bed reactor. Water Res. 1999, 33, 1918–1924. [Google Scholar] [CrossRef]
- Ahluwalia, S.S.; Goyal, D. Microbial and plant derived biomass for removal of heavy metals from wastewater. Bioresour. Technol. 2007, 98, 2243–2257. [Google Scholar] [CrossRef] [PubMed]
- Zhao, D.; Yang, X.; Zhang, H.; Chenab, C.; Wang, X. Effect of environmental conditions on Pb(II) adsorption on β-MnO2. Chem. Eng. J. 2010, 164, 49–55. [Google Scholar] [CrossRef]
- Anbia, M.; Mohammadi, N. A fast and efficient method for the removal of hexavalent chromium from aqueous solutions. J. Porous Mater. 2010, 18, 13–21. [Google Scholar] [CrossRef]
- Sarvi, M.N.; Bee, T.B.; Gooi, C.K.; Woonton, B.W.; Gee, M.L.; O’Connor, A.J. Development of functionalized mesoporous silica for adsorption and separation of dairy proteins. Chem. Eng. J. 2014, 235, 244–251. [Google Scholar] [CrossRef]
- Kozlowski, C.; Walkowiak, W. Applicability of liquid membranes in chromium (VI) transport with amines as ion carriers. J. Membr. Sci. 2005, 266, 143–150. [Google Scholar] [CrossRef]
- Ortega, L.M.; Lebrun, R.; Blais, J.-F.; Hausler, R.; Drogui, P. Effectiveness of soil washing, nanofiltration and electrochemical treatment for the recovery of metal ions coming from a contaminated soil. Water Res. 2008, 42, 1943–1952. [Google Scholar] [CrossRef]
- Al-Jubouri, S.M.; Holmes, S.M. Immobilization of cobalt ions using hierarchically porous 4A zeolite-based carbon composites: Ion-exchange and solidification. J. Water Process. Eng. 2020, 33, 101059. [Google Scholar] [CrossRef]
- Soares, M.C.V.; Bertrans, M.A.; Lemos, F.A.; Masson, I.O.C. Removal of lead, cadmium and zinc from industrial effluents using nanofiltration and reverse osmosis membranes. In Proceedings of the XIII International Conference on Heavy Metals in the Environment, Rio de Janeiro, Brazil, 5–6 June 2005. [Google Scholar]
- Mehiguene, K.; Garba, Y.; Taha, S.; Gondrexon, N.; Dorange, G. Influence of operating conditions on the retention of copper and cadmium in aqueous solutions by nanofiltration: Experimental results and modelling. Sep. Purif. Technol. 1999, 15, 181–187. [Google Scholar] [CrossRef]
- Ritchie, S.M.C.; Bhattacharyya, D. Membrane-based hybrid processes for high water recovery and selective inorganic pollutant separation. J. Hazard. Mater. 2002, 92, 21–32. [Google Scholar] [CrossRef]
- Evina, K.; Simos, M.; Katherine, J.H.; Maria, L. Use of ultrafiltration membranes and aluminosilicate minerals for nickel removal from industrial wastewater. J. Membr. Sci. 2010, 360, 234–249. [Google Scholar]
- Linde, K.; Jönsson, A.-S.; Wimmerstedt, R. Treatment of three types of landfill leachate with reverse osmosis. Desalination 1995, 101, 21–30. [Google Scholar] [CrossRef]
- Peters, T.A. Purification of landfill leachate with reverse osmosis and nanofiltration. Desalination 1998, 119, 289–293. [Google Scholar] [CrossRef]
- Tanninen, J.; Mänttäri, M.; Nyström, M. Effect of salt mixture concentration on fractionation with NF membranes. J. Membr. Sci. 2006, 283, 57–64. [Google Scholar] [CrossRef]
- Wang, D.X.; Wang, X.L.; Tomi, Y.; Ando, M.; Shintani, T. Modeling the separation performance of nanofiltration membranes for the mixed salts solution. J. Membr. Sci. 2006, 280, 734–743. [Google Scholar] [CrossRef]
- Basile, A.; Cassano, A.; Rastogi, N.K. (Eds.) Advances in Membrane Technologies for Water Treatment: Materials, Processes and Applications; Elsevier: Amsterdam, The Netherlands, 2015. [Google Scholar]
- Chaabane, T.; Taha, S.; Ahmed, M.T.; Maachi, R.; Dorange, G. Removal of copper from industrial effluent using a spiral wound module—Film theory and hydrodynamic approach. Desalination 2006, 200, 403–405. [Google Scholar] [CrossRef]
- Artuğ, G.; Hapke, J. Characterization of nanofiltration membranes by their morphology, charge and filtration performance parameters. Desalination 2006, 200, 178–180. [Google Scholar] [CrossRef]
- Fatin-Rouge, N.; Szymczyk, A.; Özdemir, E.; Vidonne, A.; Fievet, P. Retention of single and mixed inorganic electrolytes by a polyamide nanofiltration membrane. Desalination 2006, 200, 133–134. [Google Scholar] [CrossRef]
- Wang, Z.; Liu, G.; Fan, Z.; Yang, X.; Wang, J.; Wang, S. Experimental study on treatment of electroplating wastewater by nanofiltration. J. Membr. Sci. 2007, 305, 185–195. [Google Scholar] [CrossRef]
- Ballet, G.T.; Gzara, L.; Hafiane, A.; Dhahbi, M. Transport coefficients and cadmium salt rejection in nanofiltration membrane. Desalination 2004, 167, 369–376. [Google Scholar] [CrossRef]
- Al-Rashdi, B.; Johnson, D.; Hilal, N. Removal of heavy metal ions by nanofiltration. Desalination 2013, 315, 2–17. [Google Scholar] [CrossRef]
- Zhu, W.-P.; Sun, S.-P.; Gao, J.; Fu, F.-J.; Chung, T.-S.; Chung, T.-S. Dual-layer polybenzimidazole/polyethersulfone (PBI/PES) nanofiltration (NF) hollow fiber membranes for heavy metals removal from wastewater. J. Membr. Sci. 2014, 456, 117–127. [Google Scholar] [CrossRef]
- Perkin-Elmer. Analytical Methods for Atomic Absorption Spectroscopy; The Perkin-Elmer Corporation: Waltham, MA, USA, 1996. [Google Scholar]
- Tanninen, J.; Platt, S.; Weis, A.; Nyström, M. Long-term acid resistance and selectivity of NF membranes in very acidic conditions. J. Membr. Sci. 2004, 240, 11–18. [Google Scholar] [CrossRef]
- Mänttäri, M.; Pekuri, T.; Nyström, M. NF270, a new membrane having promising characteristics and being suitable for treatment of dilute effluents from the paper industry. J. Membr. Sci. 2004, 242, 107–116. [Google Scholar] [CrossRef]
- Alsalhy, Q.F.; Mohammed, A.A.; Ahmed, S.H.; Rashid, K.T.; AlSaadi, M.A. Estimation of Nanofiltration Membrane Transport Parameters for Cobalt Ions Removal from Aqueous Solutions. Desalin. Water Treat. 2018, 108, 235–245. [Google Scholar] [CrossRef]
- Alsalhy, Q.F.; Algebory, S.; Alwan, A.P.D.M.; Simone, S.; Figoli, A.; Drioli, E. Hollow Fiber Ultrafiltration Membranes from Poly(Vinyl Chloride): Preparation, Morphologies, and Properties. Sep. Sci. Technol. 2011, 46, 2199–2210. [Google Scholar] [CrossRef]
- Alsalhy, Q.F.; Rashid, K.; Noori, W.A.; Simone, S.; Figoli, A.; Drioli, E. Poly(vinyl chloride) hollow-fiber membranes for ultrafiltration applications: Effects of the internal coagulant composition. J. Appl. Polym. Sci. 2011, 124, 2087–2099. [Google Scholar] [CrossRef]
- Alsalhy, Q.F.; Merza, A.S.; Rashid, K.T.; Adam, A.; Figoli, A.; Simone, S.; Drioli, E. Preparation and Characterization of poly(vinyl chloride)/poly (styrene)/poly (ethylene glycol) hollow-fiber membranes. J. Appl. Polym. Sci. 2013, 130, 989–1004. [Google Scholar] [CrossRef]
- Semiao, A.J.C.; Schafer, A.I. Estrogenic micro-pollutant adsorption dynamics onto nanofiltration membranes. J. Membr. Sci. 2011, 381, 132–141. [Google Scholar] [CrossRef]
- Hajarat, R.A. The Use of Nanofiltration Membrane in Desalinating Brackish Water. Ph.D. Thesis, University of Manchester, Manchester, UK, 2010. [Google Scholar]
- Gherasim, C.-V.; Cuhorka, J.; Mikulášek, P. Analysis of lead(II) retention from single salt and binary aqueous solutions by a polyamide nanofiltration membrane: Experimental results and modelling. J. Membr. Sci. 2013, 436, 132–144. [Google Scholar] [CrossRef]
- Bird, R.B.; Stewart, W.E.; Lightfoot, E.N. Transport Phenomena, 2nd ed.; John Wiley & Sons, Inc.: New York, NY, USA, 2002. [Google Scholar]
- Lee, S.; Amy, G.; Cho, J. Applicability of Sherwood correlations for natural organic matter (NOM) transport in nanofiltration (NF) membranes. J. Membr. Sci. 2004, 240, 49–65. [Google Scholar] [CrossRef]
- Desai, K.R.; Murthy, Z. Removal of Ag(I) and Cr(VI) by Complexation-Ultrafiltration and Characterization of the Membrane by CFSK Model. Sep. Sci. Technol. 2014, 49, 2620–2629. [Google Scholar] [CrossRef]
- Kedem, O.; Spiegler, K. Thermodynamics of Hyper-filtration (Reverse Osmosis): Criteria for Efficient Membrane. Desalination 1966, 1, 311–326. [Google Scholar]
- Murthy, Z.; Chaudhari, L.B. Application of nanofiltration for the rejection of nickel ions from aqueous solutions and estimation of membrane transport parameters. J. Hazard. Mater. 2008, 160, 70–77. [Google Scholar] [CrossRef] [PubMed]
- Vaidya, S.Y.; Simaria, A.V.; Murthy, Z.V.P. Reverse Osmosis Transport Models Evaluation: A new Approach. Ind. J. Chem. Tech. 2001, 8, 335–343. [Google Scholar]
- Baker, R.W. Membrane Technology and Applications; Wiley: New York, NY, USA, 2004. [Google Scholar]
- Foo, K.Y.; Hameed, B. Insights into the modeling of adsorption isotherm systems. Chem. Eng. J. 2010, 156, 2–10. [Google Scholar] [CrossRef]
- Belfort, G.; Davis, R.H.; Zydney, A.L. The Behavior of Suspensions and Macromolecular Solutions in Cross Flow Microfiltration. J. Membr. Sci. 1994, 1, 96. [Google Scholar]
- Childress, A.E.; Elimelech, M. Relating Nanofiltration Membrane Performance to Membrane Charge (Electrokinetic) Characteristics. Environ. Sci. Technol. 2000, 34, 3710–3716. [Google Scholar] [CrossRef]
- Petrucci, R.H.; Harwood, W.S. General Chemistry: Principle and Modern Application, 6th ed.; Prentice Hall: Upper Saddle River, NJ, USA, 1993. [Google Scholar]
- Bazaraa, M.S.; Sherali, H.D.; Shetty, C.M. Nonlinear Programming: Theory and Algorithms, 2nd ed.; Wiley: New York, NY, USA, 1993. [Google Scholar]
- Al-Zoubi, H. Development of Novel Approach to the Prediction of Nanofiltration Membrane Performance Using Advanced Atomic Force Microscopy. Ph.D. Thesis, University of Nottingham, Nottingham, UK, 2006. [Google Scholar]
- Schaep, J.; Van Der Bruggen, B.; Uytterhoeven, S.; Croux, R.; Vandecasteele, C.; Wilms, D.; Van Houtte, E.; Vanlerberghe, F. Removal of hardness from groundwater by nanofiltration. Desalination 1998, 119, 295–301. [Google Scholar] [CrossRef]
- Wang, X.-L.; Tsuru, T.; Nakao, S.-I.; Kimura, S. Electrolyte transport through nanofiltration membranes by the space-charge model and the comparison with Teorell-Meyer-Sievers model. J. Membr. Sci. 1995, 103, 117–133. [Google Scholar] [CrossRef]
- Murthy, Z.; Chaudhari, L.B. Separation of binary heavy metals from aqueous solutions by nanofiltration and characterization of the membrane using Spiegler–Kedem model. Chem. Eng. J. 2009, 150, 181–187. [Google Scholar] [CrossRef]
- Soltanieh, M.; Gill’, W.N. Review of reverse osmosis membranes and transport models. Chem. Eng. Commun. 1981, 12, 279–363. [Google Scholar] [CrossRef]
- Borisov, I.L.; Kujawska, A.; Knozowska, K.; Volkov, A.; Kujawski, W. Influence of feed flow rate, temperature and feed concentration on concentration polarization effects during separation of water-methyl acetate solutions with high permeable hydrophobic pervaporation PDMS membrane. J. Membr. Sci. 2018, 564, 1–9. [Google Scholar] [CrossRef]
- Chabane, M.; Dahmani, B. Study on Removal Efficiency of Natural Organic Matter and Lead Metal Solution Using Nanofiltration Membrane. J. Chromatogr. Sep. Tech. 2012, 3, 1–4. [Google Scholar] [CrossRef] [Green Version]
- Maher, A.; Sadeghi, M.; Moheb, A. Heavy metal elimination from drinking water using nanofiltration membrane technology and process optimization using response surface methodology. Desalination 2014, 352, 166–173. [Google Scholar] [CrossRef]
- Gao, J.; Sun, S.-P.; Zhu, W.-P.; Chung, T.-S.; Chung, T.-S. Chelating polymer modified P84 nanofiltration (NF) hollow fiber membranes for high efficient heavy metal removal. Water Res. 2014, 63, 252–261. [Google Scholar] [CrossRef]
- Zeng, G.; He, Y.; Zhan, Y.; Zhang, L.; Pan, Y.; Zhang, C.; Yu, Z. Novel polyvinylidene fluoride nanofiltration membrane blended with functionalized halloysite nanotubes for dye and heavy metal ions removal. J. Hazard. Mater. 2016, 317, 60–72. [Google Scholar] [CrossRef]
- Bera, A.; Trivedi, J.S.; Kumar, S.B.; Chandel, A.K.S.; Haldar, S.; Jewrajka, S.K. Anti-organic fouling and anti-biofouling poly(piperazineamide) thin film nanocomposite membranes for low pressure removal of heavy metal ions. J. Hazard. Mater. 2018, 343, 86–97. [Google Scholar] [CrossRef] [PubMed]
- Figoli, A.; Ursino, C.; Santoro, S.; Ounifi, I.; Chekir, J.; Hafiane, A.; Ferjani, E. Cellulose Acetate Nanofiltration Membranes for Cadmium Remediation. J. Membr. Sci. Res. 2020, 6, 226–234. [Google Scholar]












| Membrane Code | Membrane Material | Average Pore Size (nm) | Pore size Distribution (nm) | Porosity (%) | Outer Diameter (µm) | Inner Diameter (µm) | Thickness (µm) |
|---|---|---|---|---|---|---|---|
| PES1 | PES (29%) | 52.04 | 25–100 | 52.5 | 1012 | 620 | 196 |
| PES2 | PES (27%) | 58.11 | 35–130 | 67.6 | 958.4 | 576 | 191.2 |
| PES3 | PES (27%) | 47.75 | 20–115 | 58.1 | 1005 | 603.6 | 200.7 |
| Metal Salts | pH metal | Cmetal (ppm) | Pressure |
|---|---|---|---|
| Pb(NO3)2 | 5.5, 5.7, 6, 6.2, 6.5 | 10, 50, 100, 200, 250 | 1 bar |
| Cd(NO3)2·4H2O | 5.5, 5.7, 6, 6.2, 6.5 | 10, 50, 100, 200, 250 | 1 bar |
| Pb(NO3)2 + Cd(NO3)2·4H2O | 6 ± 0.2 | 10 + 50, 50 + 10, 50 + 50 | 1 bar |
| Type of Membrane | No. of Set | pH | Feed Conc. (ppm) | CFSD Model | CFSK Model | CFFP Model | |||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| Dam K b/ δ × 104(cm/s) | k a × 103 (cm/s) | σ | PM × 105 (cm/s) | k a × 103 (cm/s) | S2 | ε/k a | εDab/ | ||||
| PES1 | 1 | 6.0 | 10 | 2.45 | 4.5 | 0.9101 | 5.53 | 20.72 | 0.007 | 7.72 | 9.45 |
| 2 | 6.0 | 50 | 2.48 | 4.33 | 0.9080 | 5.97 | 20.53 | 0.009 | 7.79 | 9.27 | |
| 3 | 6.0 | 100 | 2.51 | 4.25 | 0.9004 | 6.21 | 20.21 | 0.016 | 7.92 | 9.55 | |
| 4 | 6.0 | 200 | 2.42 | 4.11 | 0.8864 | 7.84 | 19.73 | 0.032 | 8.11 | 9.8 | |
| 5 | 6.0 | 250 | 2.38 | 4.03 | 0.8795 | 8.44 | 19.43 | 0.043 | 8.23 | 10.21 | |
| PES2 | 6 | 6.5 | 10 | 2.64 | 4.68 | 0.9211 | 6.33 | 21.12 | 0.024 | 7.58 | 9.75 |
| 7 | 6.5 | 50 | 2.58 | 4.55 | 0.9156 | 6.67 | 21.01 | 0.018 | 7.62 | 9.62 | |
| 8 | 6.5 | 100 | 2.47 | 4.46 | 0.9111 | 6.91 | 20.61 | 0.019 | 7.76 | 9.85 | |
| 9 | 6.5 | 200 | 2.38 | 4.43 | 0.8981 | 7.44 | 20.41 | 0.035 | 7.84 | 9.83 | |
| 10 | 6.5 | 250 | 2.39 | 4.23 | 0.8895 | 7.84 | 19.73 | 0.042 | 8.11 | 10.11 | |
| PES3 | 11 | 6.2 | 10 | 2.44 | 4.88 | 0.9255 | 6.43 | 20.92 | 0.00025 | 7.65 | 9.65 |
| 12 | 6.2 | 50 | 2.68 | 4.73 | 0.9246 | 6.58 | 20.71 | 0.00049 | 7.73 | 9.41 | |
| 13 | 6.2 | 100 | 2.57 | 4.58 | 0.9201 | 6.84 | 20.11 | 0.0021 | 7.96 | 9.55 | |
| 14 | 6.2 | 200 | 2.78 | 4.54 | 0.9181 | 7.14 | 19.71 | 0.007 | 8.12 | 9.91 | |
| 15 | 6.2 | 250 | 2.88 | 4.43 | 0.9095 | 7.74 | 19.13 | 0.0059 | 8.36 | 10.31 | |
| Type of Membrane | No. of Set | pH | Feed Conc. (ppm) | CFSD Model | CFSK Model | CFFP Model | |||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| Dam K b/ δ × 104(cm/s) | k a × 103 (cm/s) | σ | PM × 105 (cm/s) | k a × 103 (cm/s) | S2 | ε/k a | εDab/ | ||||
| PES1 | 1 | 6.5 | 10 | 2.87 | 4.77 | 0.9227 | 5.58 | 20.98 | 0.048 | 7.63 | 10.23 |
| 2 | 6.5 | 50 | 2.76 | 4.65 | 0.9219 | 6.11 | 20.61 | 0.029 | 7.76 | 10.56 | |
| 3 | 6.5 | 100 | 2.81 | 4.63 | 0.9205 | 6.54 | 20.33 | 0.057 | 7.87 | 10.66 | |
| 4 | 6.5 | 200 | 2.66 | 4.58 | 0.9198 | 7.23 | 20.01 | 0.053 | 8.00 | 10.98 | |
| 5 | 6.5 | 250 | 2.57 | 4.55 | 0.9187 | 8.67 | 19.88 | 0.074 | 8.05 | 11.32 | |
| PES2 | 6 | 6.2 | 10 | 2.97 | 4.87 | 0.9223 | 6.78 | 21.88 | 0.042 | 7.31 | 10.28 |
| 7 | 6.2 | 50 | 2.88 | 4.81 | 0.9119 | 7.11 | 20.91 | 0.04 | 7.65 | 10.38 | |
| 8 | 6.2 | 100 | 2.84 | 4.67 | 0.9122 | 7.45 | 20.73 | 0.048 | 7.72 | 10.76 | |
| 9 | 6.2 | 200 | 2.76 | 4.61 | 0.9089 | 8.13 | 20.91 | 0.134 | 7.65 | 10.86 | |
| 10 | 6.2 | 250 | 2.51 | 4.53 | 0.9087 | 8.77 | 19.73 | 0.185 | 8.11 | 11.12 | |
| PES3 | 11 | 6.5 | 10 | 2.67 | 4.89 | 0.9263 | 6.68 | 20.08 | 0.051 | 7.59 | 10.27 |
| 12 | 6.5 | 50 | 2.79 | 4.78 | 0.9219 | 6.01 | 20.95 | 0.112 | 7.64 | 10.67 | |
| 13 | 6.5 | 100 | 2.82 | 4.69 | 0.9202 | 7.15 | 20.43 | 0.184 | 7.83 | 10.69 | |
| 14 | 6.5 | 200 | 2.86 | 4.71 | 0.9189 | 8.03 | 20.11 | 0.254 | 7.96 | 11.58 | |
| 15 | 6.5 | 250 | 2.91 | 4.83 | 0.9127 | 8.25 | 19.83 | 0.082 | 8.07 | 11.82 | |
| Type of Membrane | No. of Set | pH | Feed Conc. (ppm) | Enrichment Factors | CPM | k a × 103 (cm/s) | Permeate Flux (×103) (cm/s) | Péclet Number (J/k a) | |
|---|---|---|---|---|---|---|---|---|---|
| E | Eo | Cm/Cb | |||||||
| PES1 | 1 | 6.0 | 10 | 0.0098 | 0.0100 | 1.0163 | 20.72 | 0.361 | 0.0174 |
| 2 | 6.0 | 50 | 0.0433 | 0.0440 | 1.0154 | 20.53 | 0.358 | 0.0175 | |
| 3 | 6.0 | 100 | 0.1648 | 0.1670 | 1.0135 | 20.21 | 0.353 | 0.0175 | |
| 4 | 6.0 | 200 | 0.4864 | 0.4900 | 1.0075 | 19.73 | 0.292 | 0.0148 | |
| 5 | 6.0 | 250 | 0.5063 | 0.5100 | 1.0072 | 19.43 | 0.289 | 0.0149 | |
| PES2 | 6 | 6.5 | 10 | 0.0240 | 0.025 | 1.0423 | 21.12 | 0.8972 | 0.0425 |
| 7 | 6.5 | 50 | 0.0538 | 0.056 | 1.0402 | 21.01 | 0.8778 | 0.0418 | |
| 8 | 6.5 | 100 | 0.3585 | 0.368 | 1.0264 | 20.61 | 0.8528 | 0.0414 | |
| 9 | 6.5 | 200 | 0.5301 | 0.540 | 1.0186 | 20.41 | 0.8194 | 0.0401 | |
| 10 | 6.5 | 250 | 0.5601 | 0.57 | 1.0177 | 19.73 | 0.8056 | 0.0408 | |
| PES3 | 11 | 6.2 | 10 | 0.0196 | 0.02 | 1.0210 | 20.92 | 0.4444 | 0.0212 |
| 12 | 6.2 | 50 | 0.0824 | 0.084 | 1.0196 | 20.71 | 0.4389 | 0.0212 | |
| 13 | 6.2 | 100 | 0.3493 | 0.354 | 1.0135 | 20.11 | 0.4333 | 0.0215 | |
| 14 | 6.2 | 200 | 0.4749 | 0.48 | 1.0108 | 19.71 | 0.4222 | 0.0214 | |
| 15 | 6.2 | 250 | 0.5049 | 0.51 | 1.0102 | 19.13 | 0.4167 | 0.0218 | |
| Type of Membrane | No. of Set | pH | Feed Conc. (ppm) | Enrichment Factors | CPM | k a × 103 (cm/s) | Permeate Flux (×103) (cm/s) | Péclet Number (J/k a) | |
|---|---|---|---|---|---|---|---|---|---|
| E | Eo | Cm/Cb | |||||||
| PES1 | 1 | 6.5 | 10 | 0.2177 | 0.2200 | 1.0104 | 20.98 | 0.278 | 0.0132 |
| 2 | 6.5 | 50 | 0.2674 | 0.2700 | 1.0097 | 20.61 | 0.275 | 0.0133 | |
| 3 | 6.5 | 100 | 0.3968 | 0.4000 | 1.0080 | 20.33 | 0.272 | 0.0134 | |
| 4 | 6.5 | 200 | 0.3868 | 0.3900 | 1.0082 | 20.01 | 0.264 | 0.0132 | |
| 5 | 6.5 | 250 | 0.5769 | 0.5800 | 1.0055 | 19.88 | 0.261 | 0.0131 | |
| PES2 | 6 | 6.2 | 10 | 0.4913 | 0.500 | 1.0178 | 21.88 | 0.8000 | 0.0366 |
| 7 | 6.2 | 50 | 0.5171 | 0.526 | 1.0173 | 20.91 | 0.7806 | 0.0373 | |
| 8 | 6.2 | 100 | 0.5461 | 0.555 | 1.0163 | 20.73 | 0.7667 | 0.0370 | |
| 9 | 6.2 | 200 | 0.6735 | 0.680 | 1.0097 | 20.91 | 0.6833 | 0.0327 | |
| 10 | 6.2 | 250 | 0.7344 | 0.740 | 1.0076 | 19.73 | 0.6444 | 0.0327 | |
| PES3 | 11 | 6.5 | 10 | 0.5555 | 0.560 | 1.0081 | 21.08 | 0.3861 | 0.0183 |
| 12 | 6.5 | 50 | 0.6057 | 0.610 | 1.0070 | 20.95 | 0.3806 | 0.0182 | |
| 13 | 6.5 | 100 | 0.6761 | 0.680 | 1.0058 | 20.43 | 0.3722 | 0.0182 | |
| 14 | 6.5 | 200 | 0.6963 | 0.700 | 1.0054 | 20.11 | 0.3639 | 0.0181 | |
| 15 | 6.5 | 250 | 0.7185 | 0.722 | 1.0048 | 19.83 | 0.3500 | 0.0177 | |
| Type of Membrane | Module | Material Removed (Aqueous Solution) | pH | Con. ppm | Pressure | Rejection % | Ref. |
|---|---|---|---|---|---|---|---|
| NF270 | Flat sheet | Pb(NO3)2/Cd(NO3)2 | 1.5–5 | 100–2000 | 3–5 bar | Cd2+ = 99% Pb2+ = 74% | [27] |
| Dual-layer NF | hollow fiber | Na2Cr2O7 CdCl2 pb(NO3)2 | 4.74 5.45 5.03 | 1000 | 1 bar | Cr2O7− = 98% Cd2+ = 95% Pb2+ = 93% | [28] |
| TFC-NF300 | polyamide thin film | CdCl2; NiSO4 | 5 | 5–150 | 2–20 atm | Cd2+ = 80% Ni2+ = 97% | [43] |
| ESNA1-4040 | polyamide thin film | pbCl2 | 1–12 | 20 | 4–16 Mpa | pb2+ = 93.3% | [57] |
| NF (JCM) | Polyamide flat sheet, spiral wound | Pb(NO3)2 NiSO4 | 3–4 | 1 | 5.8 bar for pb2+ 6 for Ni2+ | Pb2+ = 86% Ni2+ = 93% | [58] |
| (PEI) cross linked P84 | hollow fiber | pb(NO3)2 | 12 | 1000 | 13 bar | pb2+ = 91.05% | [59] |
| PVDF/APTES functionalized halloysite | Flat sheet | Cu2+, Cd2+ and Cr6+ homogeneous solution | 5.5 | 5 | 5 bar | Cd2+ = 44.2% | [60] |
| Poly/PIP PA layer modified PEI substrate: PES/Ag | Flat sheet | Pb2+, and Cd2+ solution | 5.0–7.0 | 100 | 5 bar | Cd2+ = 97% Pb2+ = 99% | [61] |
| cellulose acetate (CA) NF-23 | Flat sheet | Cd(NO3)2 | 2–12 | 0.001 mol/l | 9 bar | Cd2+ = 84% | [62] |
| PES1; PES2; PES3 | hollow fiber | (Cd(NO3)2·4H2O); (Pb(NO3)2) | 5.5–6.5 | 10–250 | 1 bar | Pb2+ = 99%; 97.5%; 98% Cd2+ = 78%; 49.2%; 44% | This study |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hadi, S.; Mohammed, A.A.; Al-Jubouri, S.M.; Abd, M.F.; Majdi, H.S.; Alsalhy, Q.F.; Rashid, K.T.; Ibrahim, S.S.; Salih, I.K.; Figoli, A. Experimental and Theoretical Analysis of Lead Pb2+ and Cd2+ Retention from a Single Salt Using a Hollow Fiber PES Membrane. Membranes 2020, 10, 136. https://doi.org/10.3390/membranes10070136
Hadi S, Mohammed AA, Al-Jubouri SM, Abd MF, Majdi HS, Alsalhy QF, Rashid KT, Ibrahim SS, Salih IK, Figoli A. Experimental and Theoretical Analysis of Lead Pb2+ and Cd2+ Retention from a Single Salt Using a Hollow Fiber PES Membrane. Membranes. 2020; 10(7):136. https://doi.org/10.3390/membranes10070136
Chicago/Turabian StyleHadi, Salwa, Ahmed A. Mohammed, Sama M. Al-Jubouri, Mahmood F. Abd, Hasan Shaker Majdi, Qusay F. Alsalhy, Khalid T. Rashid, Salah S. Ibrahim, Issam K. Salih, and Alberto Figoli. 2020. "Experimental and Theoretical Analysis of Lead Pb2+ and Cd2+ Retention from a Single Salt Using a Hollow Fiber PES Membrane" Membranes 10, no. 7: 136. https://doi.org/10.3390/membranes10070136
APA StyleHadi, S., Mohammed, A. A., Al-Jubouri, S. M., Abd, M. F., Majdi, H. S., Alsalhy, Q. F., Rashid, K. T., Ibrahim, S. S., Salih, I. K., & Figoli, A. (2020). Experimental and Theoretical Analysis of Lead Pb2+ and Cd2+ Retention from a Single Salt Using a Hollow Fiber PES Membrane. Membranes, 10(7), 136. https://doi.org/10.3390/membranes10070136