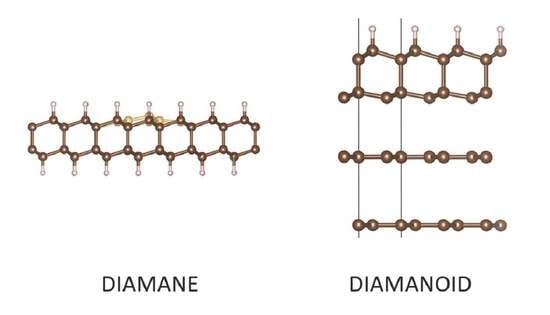
Progress on Diamane and Diamanoid Thin Film Pressureless Synthesis
Abstract
:1. Introduction
2. Materials and Methods
2.1. Pristine Graphene Materials
2.2. Hydrogenation Process
2.3. Material Characterization
2.4. Computational Details
2.5. Nomenclature
3. Results and Discussion
3.1. Hot-Filament Process to Efficiently Hydrogenate Graphene Materials
3.2. Genuine Diamane from 2LG
- (i)
- single 2LG domain with AA stacking (illustrated by Figure 2a);
- (ii)
- (iii)
- single 2LG domain made of two randomly stacked 1LG (illustrated by Figure 2c), otherwise designated as twisted 2LG;
- (iv)
- multiple 2LG domains, corresponding to the various combinations of cases (i) to (iii) above (illustrated by Figure 2d); this case happens when the selection aperture covers an area of the 2LG film where several neighboring domains separated by defect lines are present.
- (i)
- the G peak is no longer detected;
- (ii)
- the D peak which originates from defects in graphene sheets [37] is still not observed;
- (iii)
- a sharp peak (full width at half maximum (FWHM) of around 10–33 cm−1) at around 1344−1367 cm−1 has appeared.
3.3. Diamanoid/Graphene Hybrid from FLG
3.3.1. Model of Diamanoid/Graphene Hybrid
3.3.2. UV Raman Spectroscopy Analysis
3.3.3. Electron Diffraction
3.3.4. FTIR Microscopy
- The symmetrical sp3–C–H2 and symmetrical sp3–C–H3 modes are excluded as they would be simultaneously detected with their antisymmetrical counterparts.
- The olefinic sp2–C–H and aromatic sp2–C–H modes are excluded as they are typically detected at much higher wavenumber, above 2975 cm−1 in the case of free molecules [66].
- The symmetrical and anti-symmetrical olefinic C2H2 modes are excluded as they are typically detected at significantly higher wavenumber, above 2950 cm−1, in the case of free molecules [66]. Furthermore, they would be accompanied by their anti/symmetrical counterpart, which is not the case here.
- Our experimental value is consistent with the calculated C–H stretching mode frequency for graphane, 2H-(AB)D, (AB)D, and 2H-(ABC)D [2]. The C–H stretching mode frequencies of isolated H on graphene and on single vacancy in graphene are found to be much higher giving a further evidence of high hydrogenation rate.
3.3.5. Understanding Further the Characterization Results—Modeling
3.4. An Additional Route for the Formation of Diamond Grains in Space?
- (i)
- the (possible) extragalactic detection of planar C24 from the Spitzer Space Telescope [77]; recent DFT and coupled-cluster calculations on wavefunction stability showed that the graphene form of the C24 was the most stable of different types of C24 isomers, including the fullerene form, and that it best accounts for the astronomical data [78]; and
- (ii)
- the recent speculation from laboratory experiments that FLG grains could be formed in space from pyrene [79].
4. Conclusions
5. Patents
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Acknowledgments
Conflicts of Interest
References
- Chernozatonskii, L.A.; Sorokin, P.B.; Kvashnin, A.; Kvashnin, D.G. Diamane: Simulation of the structure and properties. J. Exp. Theor. Phys. Lett. 2009, 90, 134–138. [Google Scholar] [CrossRef] [Green Version]
- Piazza, F.; Gough, K.; Monthioux, M.; Puech, P.; Gerber, I.; Wiens, R.; Paredes, G.; Ozoria, C. Low temperature, pressureless sp2 to sp3 transformation of ultrathin, crystalline carbon films. Carbon 2019, 145, 10–22. [Google Scholar] [CrossRef]
- Chernozatonskii, L.A.; Sorokin, P.B.; Kuzubov, A.A.; Sorokin, B.P.; Kvashnin, A.G.; Kvashnin, D.G.; Avramov, P.V.; Yakobson, B.I. Influence of size effect on the electronic and elastic properties of diamond films with nanometer thickness. J. Phys. Chem. C 2011, 115, 132–136. [Google Scholar] [CrossRef] [Green Version]
- Chernozatonskii, L.A.; Mavrin, B.N.; Sorokin, P.B. Determination of ultrathin diamond films by Raman spectroscopy. Phys. Status Solidi B 2012, 249, 1550–1554. [Google Scholar] [CrossRef]
- Ruoff, R.S. Personal perspectives on graphene: New graphene-related materials on the horizon. MRS Bull. 2012, 37, 1314–1318. [Google Scholar] [CrossRef]
- Gupta, S.; Yang, J.H.; Yakobson, B.I. Two-level quantum systems in two-dimensional materials for single photon emission. Nano Lett. 2019, 19, 408–414. [Google Scholar] [CrossRef]
- Zheng, Z.; Zhan, H.; Nie, Y.; Xu, X.; Qi, D.; Gu, Y. Single layer diamond-A new ultrathin 2D carbon nanostructure for mechanical resonator. Carbon 2020, 161, 809–815. [Google Scholar] [CrossRef]
- Leenaerts, O.; Partoens, B.; Peeters, F.M. Hydrogenation of bilayer graphene and the formation of bilayer graphane from first principles. Phys. Rev. B 2009, 80, 245422. [Google Scholar] [CrossRef]
- Zhu, L.; Hu, H.; Chen, Q.; Wang, S.; Wang, J.; Ding, F. Formation and electronic properties of hydrogenated few layer graphene. Nanotechnology 2001, 22, 185202. [Google Scholar] [CrossRef]
- Odkhuu, D.; Sin, D.; Ruoff, R.S.; Park, N. Conversion of multilayer graphene into continuous ultrathin sp3-bonded carbon films on metal surfaces. Sci. Rep. 2013, 3, 3276. [Google Scholar] [CrossRef] [Green Version]
- Rajasekaran, S.; Abild-Pedersen, F.; Ogasawara, H.; Nilsson, A.; Kaya, S. Interlayer carbon bond formation induced by hydrogen adsorption in few-layer supported graphene. Phys. Rev. Lett 2013, 111, 085503. [Google Scholar] [CrossRef] [PubMed]
- Robertson, J. Diamond-like amorphous carbon. Mater. Sci. Eng. R 2002, 37, 129–281. [Google Scholar] [CrossRef] [Green Version]
- Piazza, F. Hard-hydrogenated tetrahedral amorphous carbon films by distributed electron cyclotron resonance plasma. Int. J. Refract. Met. Hard Mater. 2006, 24, 39–48. [Google Scholar] [CrossRef]
- Barboza, A.P.M.; Guimaraes, M.H.D.; Massote, D.V.P.; Campos, L.C.; Neto, N.M.B.; Cancado, L.G.; Lacerda, R.G.; Chacham, H.; Mazzoni, M.S.C.; Neves, B.R.A. Room temperature compression-induced diamondization of few-layer graphene. Adv. Mater. 2011, 23, 3014–3017. [Google Scholar] [CrossRef] [PubMed]
- Martins, L.G.P.; Matos, M.J.S.; Paschoal, A.R.; Freire, P.T.C.; Andrade, N.F.; Aguiar, A.L.; Kong, J.; Neves, B.R.A.; de Oliveira, A.B.; Mazzoni, M.S.C.; et al. Raman evidence for pressure-induced formation of diamondene. Nat. Comm 2017, 8, 96. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Gao, Y.; Cao, T.; Cellini, F.; Berger, C.; de Heer, W.A.; Tosatii, E.; Riedo, E.; Bongiorno, A. Ultrahard carbon film from epitaxial two-layer graphene. Nat. Nanotechnol. 2018, 13, 133–138. [Google Scholar] [CrossRef]
- Piazza, F.; Monthioux, M.; Puech, P.; Gerber, I. Towards a better understanding of the structure of diamanoïds and diamanoïd/graphene hybrids. Carbon 2020, 156, 234–241. [Google Scholar] [CrossRef]
- Piazza, F.; Puech, P.; Gerber, I.; Cruz, K.; Monthioux, M. Raman evidence for the successful synthesis of diamane. Carbon 2020, 169, 129–133. [Google Scholar] [CrossRef]
- Bakharev, P.V.; Huang, M.; Saxena, M.; Lee, S.W.; Joo, S.H.; O Park, S.; Dong, J.; Camacho-Mojica, D.C.; Jin, S.; Kwon, Y.; et al. Chemically induced transformation of chemical vapour deposition grown bilayer graphene into fluorinated single-layer diamond. Nat. Nanotechnol. 2020, 15, 59–66. [Google Scholar] [CrossRef] [Green Version]
- Regan, W.; Alem, N.; Alemán, B.; Geng, B.; Girit, Ç.; Maserati, L.; Wang, F.; Crommie, M.; Zettl, A. A direct transfer of layer-area graphene. Appl. Phys. Lett. 2010, 96, 113102-1–113102-3. [Google Scholar] [CrossRef] [Green Version]
- Piazza, F.; Morell, G.; Beltran-Huarac, J.; Paredes, G.; Ahmadi, M.; Guinel, M. Carbon nanotubes coated with diamond nanocrystals and silicon carbide by hot-filament chemical vapor deposition below 200 °C substrate temperature. Carbon 2014, 75, 113–123. [Google Scholar] [CrossRef]
- Wagner, J.; Wild, C.; Koidl, P. Resonance effects in Raman scattering from polycrystalline diamond films. Appl. Phys. Lett. 1991, 59, 779–781. [Google Scholar] [CrossRef]
- Kresse, G.; Hafner, J. Ab initio molecular dynamics for liquid metals. Phys. Rev. B 1993, 47, 558–561. [Google Scholar] [CrossRef]
- Kresse, G.; Furthmüller, J. Efficient iterative schemes for ab initio total-energy calculations using a plane-wave basis set. Phys. Rev. B 1996, 54, 11169–11186. [Google Scholar] [CrossRef]
- Blöchl, P.E. Projector augmented-wave method. Phys. Rev. B 1994, 50, 17953–17979. [Google Scholar] [CrossRef] [Green Version]
- Kresse, G.; Joubert, D. From ultrasoft pseudopotentials to the projector augmented-wave method. Phys. Rev. B 1999, 59, 1758–1775. [Google Scholar] [CrossRef]
- Perdew, J.P.; Burke, K.; Ernzerhof, M. Generalized gradient approximation made simple. Phys. Rev. Lett 1996, 77, 3865. [Google Scholar] [CrossRef] [Green Version]
- Hedin, L. New Method for Calculating the one-particle green’s function with application to the electron-gas problem. Phys. Rev. 1965, 139, A796–A823. [Google Scholar] [CrossRef]
- Shishkin, M.; Kresse, G. Implementation and performance of the frequency-dependent GW method within the PAW framework. Phys. Rev. B 2006, 74, 035101–035113. [Google Scholar] [CrossRef] [Green Version]
- Heyd, J.; Scuseria, G.E. Assessment and validation of a screened Coulomb hybrid density functional. J. Chem. Phys. 2004, 120, 7274–7280. [Google Scholar] [CrossRef]
- Paier, J.; Marsman, M.; Hummer, K.; Kresse, G.; Gerber, I.C.; Ángyán, J.A. Screened hybrid density functionals applied to solids. J. Chem. Phys. 2006, 124, 154709–154713. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Grimme, S.; Antony, J.; Ehrlich, S.; Krieg, H. A consistent and accurate ab initio parametrization of density functional dispersion correction (DFT-D) for the 94 elements H-Pu. J. Chem. Phys. 2010, 132, 154104. [Google Scholar] [CrossRef] [Green Version]
- Togo, A.; Tanaka, I. First principles phonon calculations in materials science. Scr. Mater. 2015, 108, 1–5. [Google Scholar] [CrossRef] [Green Version]
- Momma, K.; Izumi, F. VESTA 3 for three-dimensional visualization of crystal, volumetric and morphology data. J. Appl. Cryst. 2011, 44, 1272–1276. [Google Scholar] [CrossRef]
- Sofo, J.O.; Chaudhari, A.S.; Barber, G.D. Graphane: A two-dimensional hydrocarbon. Phys. Rev. B 2007, 75, 153401. [Google Scholar] [CrossRef] [Green Version]
- Pumera, M.; Wong, C.H.A. Graphane and hydrogenated graphene. Chem Soc. Rev. 2013, 42, 5987–5995. [Google Scholar] [CrossRef]
- Elias, D.C.; Nair, R.R.; Mohiuddin, T.M.G.; Morozov, S.V.; Blake, P.; Halsall, M.P.; Ferrari, A.C.; Boukhvalov, D.W.; Katsnelson, M.I.; Geim, A.K.; et al. Control of Graphene’s Properties by Reversible Hydrogenation: Evidence for Graphane. Science 2009, 323, 610. [Google Scholar] [CrossRef] [Green Version]
- Prelas, M.A.; Popovici, G.; Bigelow, L.K. Handbook of Industrial Diamonds and Diamond Films; Marcel Dekker: New York, NY, USA, 1998. [Google Scholar]
- Frenklach, M.; Wang, H. Detailed surface and gas-phase chemical kinetics of diamond deposition. Phys. Rev. B 1991, 43, 1520–1545. [Google Scholar] [CrossRef]
- Stiegler, J.; Lang, T.; Von Kaenel, Y.; Michler, J.; Blank, E. Activation energy for diamond growth from the carbon-hydrogen gas system at low temperatures. Appl. Phys. Lett. 1997, 70, 173–175. [Google Scholar] [CrossRef]
- Redman, S.A.; Chung, C.; Ashfold, M.N.R. H atom production on a hot filament chemical vapour deposition reactor. Diam. Relat. Mater. 1999, 8, 1383–1387. [Google Scholar] [CrossRef]
- Redman, S.A.; Chung, C.; Rosser, K.N.; Ashfold, M.N.R. Resonance enhanced multiphoton ionisation probing of H atoms in a hot filament chemical vapour deposition reactor. Phys. Chem. Chem. Phys. 1999, 1, 1415–1424. [Google Scholar] [CrossRef]
- Schäfer, L.; Klages, C.P.; Meier, U.; Kohse-Höinghaus, K. Atomic hydrogen concentration profiles at filaments used for chemical vapor deposition of diamond. Appl. Phys. Lett. 1991, 58, 571–573. [Google Scholar] [CrossRef] [Green Version]
- Chen, K.H.; Chuang, M.C.; Penney, C.M.; Banholzer, W.F. Temperature and concentration distribution of H2 and H atoms in hot-filament chemical-vapor deposition of diamond. J. Appl. Phys. 1992, 71, 1485–1493. [Google Scholar] [CrossRef]
- Connell, L.L.; Fleming, J.W.; Chu, H.N.; Vesteck, D.J., Jr.; Jensen, E.; Butler, J.E. Spatially resolved atomic hydrogen concentrations and molecular hydrogen temperature profiles in the chemical-vapor deposition of diamond. J. Appl. Phys. 1995, 78, 3622–3634. [Google Scholar] [CrossRef]
- Zumbach, V.; Schäfer, J.; Tobai, J.; Ridder, M.; Dreier, T.; Schaich, T.; Wolfrum, J.; Ruf, B.; Behrendt, F.; Deutschmann, O.; et al. Experimental investigation and computational modeling of hot filament diamond chemical vapor deposition. J. Chem. Phys. 1997, 107, 5918–5928. [Google Scholar] [CrossRef]
- Chenevier, M.; Cubertafon, J.C.; Campargue, A.; Booth, J.P. Measurement of atomic hydrogen in a hot filament reactor by two-photon laser-induced fluorescence. Diam. Relat. Mater. 1994, 3, 587–592. [Google Scholar] [CrossRef]
- Burgess, J.S.; Matis, B.R.; Robinson, J.T.; Bulat, F.A.; Perkins, F.K.; Houston, B.H.; Baldwin, J.W. Tuning the electronic properties of graphene by hydrogenation in a plasma enhanced chemical vapour deposition reactor. Carbon 2011, 49, 4420–4426. [Google Scholar] [CrossRef]
- Piazza, F.; González, J.A.; Velázquez, R.; De Jesús, J.; Rosario, S.A.; Morell, G. Diamond film synthesis at low temperature. Diam. Relat. Mater. 2006, 15, 109–116. [Google Scholar] [CrossRef]
- Piazza, F.; Morell, G. Synthesis of diamond at sub 300 °C substrate temperature. Diam. Relat. Mater. 2007, 16, 1950–1957. [Google Scholar] [CrossRef]
- Piazza, F.; Solá, F.; Resto, O.; Fonseca, L.F.; Morell, G. Synthesis of diamond nanocrystals on polyimide film. Diam. Relat. Mater. 2009, 18, 113–116. [Google Scholar] [CrossRef]
- Piazza, F. Carbon Nanotubes Conformally Coated with Diamond Nanocrystals or Silicon Carbide, Methods of Making the Same and Methods of Their Use. U.S. Patent 9,458,017, 14 November 2012. [Google Scholar]
- Puech, P.; Gerber, I.; Piazza, F.; Monthioux, M. Multiwavelength electron diffraction as a tool for identifying stacking sequences in 2D materials. arXiv 2020, arXiv:2012.08869. [Google Scholar]
- Paulmier, T.; Bell, J.M.; Fredericks, P.M. Deposition of nanocrystalline-graphite films by cathodic plasma electrolysis. Thin Solid Films 2007, 515, 2926–2934. [Google Scholar] [CrossRef] [Green Version]
- Knight, D.S.; White, W.B. Characterization of diamond films by Raman spectroscopy. J. Mater. Res. 1989, 4, 385–393. [Google Scholar] [CrossRef]
- Wu, B.R.; Xu, J.A. Zone-center modes of cubic and hexagonal diamond under high pressure: A first-principles study. Phys. Rev. B 1999, 60, 2964–2967. [Google Scholar] [CrossRef]
- Gilkes, K.W.R.; Prawer, S.; Nugent, K.W.; Robertson, J.; Sands, H.S.; Lifshitz, Y.; Shi, X. Direct quantitative detection of the sp3 bonding in diamond-like carbon films using ultraviolet and visible Raman spectroscopy. J. Appl. Phys. 2000, 87, 7283–7289. [Google Scholar] [CrossRef]
- Ferrari, A.C.; Robertson, J. Resonant Raman spectroscopy of disordered, amorphous, and diamond-like carbon. Phys. Rev. B 2001, 64, 075414-1–075414-13. [Google Scholar] [CrossRef] [Green Version]
- Prawer, S.; Nugent, K.W.; Jameieson, D.N.; Orwa, J.O.; Bursill, L.A.; Peng, J.L. The Raman spectrum of nanocrystalline diamond. Chem. Phys. Lett. 2000, 332, 93–97. [Google Scholar] [CrossRef]
- Ager, J.W., III; Veirs, D.K.; Rosenblatt, G.M. Spatially resolved Raman studies of diamaond films grown by chemical vapor deposition. Phys. Rev. B 1991, 43, 6491–6499. [Google Scholar] [CrossRef]
- Osswald, S.; Mochalin, V.N.; Havel, M.; Yushin, G.; Gogotsi, Y. Phonon confinement effects in the Raman spectrum of nanodiamond. Phys. Rev. B 2009, 80, 075419. [Google Scholar] [CrossRef]
- Zhang, K.; Ellad, B.T. Structural and electron diffraction scaling of twisted graphene bilayers. J. Mech. Phys. Solids 2018, 112, 225–238. [Google Scholar] [CrossRef]
- Yoo, H.; Engelke, R.; Carr, S.; Fang, S.; Zhang, K.; Cazeaux, P.; Sung, S.H.; Hovden, R.; Tsen, A.W.; Taniguchi, T.; et al. Atomic and electronic reconstruction at the van der Waals interface in twisted bilayer graphene. Nat. Mater. 2019, 18, 448–453. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Eliel, G.S.N.; Moutinho, M.V.O.; Gadelha, A.C.; Righi, A.; Campos, L.C.; Ribeiro, H.B.; Chiu, P.-W.; Watanabe, K.; Taniguchi, T.; Puech, P.; et al. Intralayer and interlayer electron-phonon interactions in twisted graphene heterostructures. Nat. Commun. 2018, 9, 1221. [Google Scholar] [CrossRef] [PubMed]
- Weiler, M.; Sattel, S.; Giessen, T.; Jung, K.; Ehrhardt, H.; Veerasamy, V.S.; Robertson, J. Preparation and properties of highly tetrahedral hydrogenated amorphous carbon. Phys. Rev. B 1996, 53, 1594–1608. [Google Scholar] [CrossRef] [PubMed]
- Fox, J.J.; Martin, A.E. Investigations of infra-red spectra. Determination of C–H frequencies (~3000 cm−1) in paraffins and olefins, with some observations on “polythenes”. Proc. R Soc. Lond. A 1940, 175, 208–233. [Google Scholar]
- Zheng, L.; Li, Z.; Bourdo, S.; Watanabe, F.; Ryerson, C.C.; Biris, A.S. Catalytic hydrogenation of graphene films. Chem. Commun. 2011, 47, 1213–1215. [Google Scholar] [CrossRef]
- Poh, H.L.; Sanek, F.; Sofer, Z.; Pumera, M. High-pressure hydrogenation of graphene: Towards graphane. Nanoscale 2012, 4, 7006–7011. [Google Scholar] [CrossRef]
- Subrahmanyam, K.S.; Kumar, P.; Maitra, U.; Govindaraj, A.; Hembram, K.P.S.S.; Waghmare, U.V.; Rao, C.N.R. Chemical storage of hydrogen in few-layer graphene. Proc. Natl. Acad. Sci. USA 2011, 108, 2674–2677. [Google Scholar] [CrossRef] [Green Version]
- Yang, Z.; Sun, Y.; Alemany, L.B.; Narayanan, T.N.; Billups, W.E. Birch reduction of graphite. Edge and interior functionalization by hydrogen. J. Am. Chem. Soc. 2012, 134, 18689–18694. [Google Scholar] [CrossRef]
- Schäfer, R.A.; Englert, J.M.; Wehrfritz, P.; Bauer, W.; Hauke, F.; Seyller, T.; Hirsch, A. On the Way to graphane-pronounced fluorescence of polyhydrogenated graphene. Angew. Chem. Int. Ed. 2013, 52, 754–757. [Google Scholar] [CrossRef]
- Krishna, R.; Titus, E.; Costa, L.C.; Menezes, J.C.J.M.D.S.; Correia, M.R.P.; Pinto, S.; Ventura, J.; Araújo, J.P.; Cavaleiro, J.A.S.; Gracio, J.J.A. Facile synthesis of hydrogenated reduced graphene oxide via hydrogen spillover mechanism. J. Mater. Chem. 2012, 22, 10457–10459. [Google Scholar] [CrossRef]
- Sofer, Z.; Jankovsky, O.; Simek, P.; Soferova, L.; Sedmidubsky, D.; Pumera, M. Highly hydrogenated graphene via active hydrogen reduction of graphene oxide in the aqueous phase at room temperature. Nanoscale 2014, 6, 2153–2160. [Google Scholar] [CrossRef]
- Hanfland, M.; Beister, H.; Syassen, K. Graphite under pressure: Equation of state and first-order Raman modes. Phys. Rev. B 1989, 39, 12598–12603. [Google Scholar] [CrossRef] [PubMed]
- Daulton, T.L. Extraterrestrial nanodiamonds in the cosmos. In Ultra Nanocrystalline Diamond, Synthesis, Properties, and Applications, 1st ed.; Shenderova, O.A., Gruen, D.M., Eds.; William Andrew Publishing: Norwich, NY, USA, 2006. [Google Scholar]
- Phelps, A.W. Interstellar Diamond. II. Growth and Identification. Lunar Planet. Sci. 1999. Abstract #1753. Available online: http://www.lpi.usra.edu/publications/abstracts.shtml (accessed on 20 January 2021).
- Garcia-Hernandez, D.A.; Iglesias-Groth, S.; Acosta-Pulido, J.A.; Manchado, A.; Garcia-Lario, P.; Stanghellini, L.; Villaver, E.; Shaw, R.A.; Cataldo, F. The formation of fullerenes: Clues from new C60, C70, and (possible) planar C24 detections in magellanic could planetary nebulae. Astrophys. J. Lett. 2011, 737, L30. [Google Scholar] [CrossRef] [Green Version]
- Sadjadi, S.; Kwok, S.; Cataldo, F.; García-Hernández, D.A.; Machado, A. A theoretical investigation of the possible detection of C24 in space. Fuller. Nanotub. Carbon Nanostruct. 2020, 28, 637–641. [Google Scholar] [CrossRef] [Green Version]
- Zhao, L.; Kaiser, R.I.; Xu, B.; Ablikim, U.; Ahmed, M.; Joshi, D.; Veber, G.; Fischer, F.R.; Mebel, A.M. Pyrene synthesis in circumstellar envelopes and its role in the formation of 2D nanostructures. Nat. Astron. 2018, 2, 413–419. [Google Scholar] [CrossRef]
- Piazza, F. Sp3-Bonded Carbon Materials, Methods of Manufacturing and Uses Thereof. WO Patent 2019/233901, 5 June 2018. [Google Scholar]

















| Material | sp3–C Stretching (cm−1) | T (cm−1) | TBL1 (cm−1) | TBL2 (cm−1) |
|---|---|---|---|---|
| Diamane | 1344–1367 | N.A. | N.A. | N.A. |
| Diamanoid/graphene hybrid | 1319–1337 | 1055–1071 | 1385 | 1669 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Piazza, F.; Monthioux, M.; Puech, P.; Gerber, I.C.; Gough, K. Progress on Diamane and Diamanoid Thin Film Pressureless Synthesis. C 2021, 7, 9. https://doi.org/10.3390/c7010009
Piazza F, Monthioux M, Puech P, Gerber IC, Gough K. Progress on Diamane and Diamanoid Thin Film Pressureless Synthesis. C. 2021; 7(1):9. https://doi.org/10.3390/c7010009
Chicago/Turabian StylePiazza, Fabrice, Marc Monthioux, Pascal Puech, Iann C. Gerber, and Kathleen Gough. 2021. "Progress on Diamane and Diamanoid Thin Film Pressureless Synthesis" C 7, no. 1: 9. https://doi.org/10.3390/c7010009
APA StylePiazza, F., Monthioux, M., Puech, P., Gerber, I. C., & Gough, K. (2021). Progress on Diamane and Diamanoid Thin Film Pressureless Synthesis. C, 7(1), 9. https://doi.org/10.3390/c7010009