Correlation between the Dimensions and Piezoelectric Properties of ZnO Nanowires Grown by PLI-MOCVD with Different Flow Rates
Abstract
:1. Introduction
2. Materials and Methods
2.1. ZnO NWs Grown by PLI-MOCVD
2.2. Characterizations
2.2.1. Morphological and Structural Property Measurements
2.2.2. Piezoelectric Property Measurements
3. Results
3.1. Morphological Properties
3.2. Growth Rate of ZnO NWs
3.3. Structural Properties of ZnO NW Arrays
3.4. Crystal Polarity and Piezoelectric Response
3.4.1. Crystal Polarity
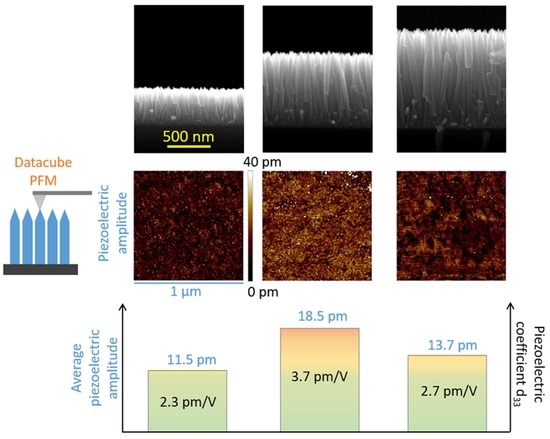
3.4.2. Piezoelectric Amplitude and Coefficient
4. Discussion
5. Conclusions
Supplementary Materials
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Wang, Z.L. Zinc Oxide Nanostructures: Growth, Properties and Applications Applications. J. Phys. Condens. Matter 2004, 16, R829. [Google Scholar] [CrossRef]
- Özgür, Ü.; Alivov, Y.I.; Liu, C.; Teke, A.; Reshchikov, M.A.; Doǧan, S.; Avrutin, V.; Cho, S.J.; Morkoç, H. A Comprehensive Review of ZnO Materials and Devices. J. Appl. Phys. 2005, 98, 041301. [Google Scholar] [CrossRef] [Green Version]
- Schmidt-Mende, L.; MacManus-Driscoll, J.L. ZnO—Nanostructures, Defects, and Devices. Mater. Today 2007, 10, 40–48. [Google Scholar] [CrossRef]
- Kołodziejczak-radzimska, A.; Jesionowski, T. Zinc Oxide—From Synthesis to Application: A Review. Materials 2014, 7, 2833–2881. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Wang, Z.L. Towards Self-Powered Nanosystems: From Nanogenerators to Nanopiezotronics. Adv. Funct. Mater. 2008, 18, 3553–3567. [Google Scholar] [CrossRef]
- Yang, Y.C.; Song, C.; Wang, X.H.; Zeng, F.; Pan, F. Giant Piezoelectric D33 Coefficient in Ferroelectric Vanadium Doped ZnO Films. Appl. Phys. Lett. 2008, 92, 012907. [Google Scholar] [CrossRef]
- Briscoe, J.; Dunn, S. Piezoelectric Nanogenerators—A Review of Nanostructured Piezoelectric Energy Harvesters. Nano Energy 2015, 14, 15–29. [Google Scholar] [CrossRef]
- Pan, L.; Sun, S.; Chen, Y.; Wang, P.; Wang, J.; Zhang, X.; Zou, J.J.; Wang, Z.L. Advances in Piezo-Phototronic Effect Enhanced Photocatalysis and Photoelectrocatalysis. Adv. Energy Mater. 2020, 10, 2000214. [Google Scholar] [CrossRef]
- Le, A.T.; Ahmadipour, M.; Pung, S. A Review on ZnO-Based Piezoelectric Nanogenerators: Synthesis, Characterization Techniques, Performance Enhancement and Applications. J. Alloys Compd. 2020, 844, 156172. [Google Scholar] [CrossRef]
- Goel, S.; Kumar, B. A Review on Piezo-/Ferro-Electric Properties of Morphologically Diverse ZnO Nanostructures. J. Alloys Compd. 2020, 816, 152491. [Google Scholar] [CrossRef]
- Wang, Z.L.; Song, J. Piezoelectric Nanogenerators Based on Zinc Oxide Nanowire Arrays. Science 2006, 312, 242–246. [Google Scholar] [CrossRef] [PubMed]
- Wang, X.; Song, J.; Liu, J.; Wang, Z.L. Direct-Current Nanogenerator Driven by Ultrasonic Waves. Science 2007, 316, 102–105. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Li, X.; Chen, M.; Yu, R.; Zhang, T.; Song, D.; Liang, R.; Zhang, Q.; Cheng, S.; Dong, L.; Pan, A.; et al. Enhancing Light Emission of ZnO-Nanofilm/Si-Micropillar Heterostructure Arrays by Piezo-Phototronic Effect. Adv. Mater. 2015, 27, 4447–4453. [Google Scholar] [CrossRef] [PubMed]
- Li, X.; Liang, R.; Tao, J.; Peng, Z.; Xu, Q.; Han, X.; Wang, X.; Wang, C.; Zhu, J.; Pan, C.; et al. Flexible Light Emission Diode Arrays Made of Transferred Si Microwires-ZnO Nanofilm with Piezo-Phototronic Effect Enhanced Lighting. ACS Nano 2017, 11, 3883–3889. [Google Scholar] [CrossRef] [PubMed]
- Zhang, B.P.; Wakatsuki, K.; Binh, N.T.; Usami, N.; Segawa, Y. Effects of Growth Temperature on the Characteristics of ZnO Epitaxial Films Deposited by Metalorganic Chemical Vapor Deposition. Thin Solid Film. 2004, 449, 12–19. [Google Scholar] [CrossRef]
- Park, J.Y.; Lee, D.J.; Yun, Y.S.; Moon, J.H.; Lee, B.; Kim, S.S. Temperature-Induced Morphological Changes of ZnO Grown by Metalorganic Chemical Vapor Deposition. J. Cryst. Growth 2005, 276, 158–164. [Google Scholar] [CrossRef]
- Malandrino, G.; Blandino, M.; Fragala, M.E.; Losurdo, M.; Bruno, G. Relationship between Nanostructure and Optical Properties of ZnO Thin Films. J. Phys. Chem. C 2008, 112, 9595–9599. [Google Scholar] [CrossRef]
- Pagni, O.; Leitch, A.W.R. Influence of VI:II Ratio on the Properties of MOCVD-Grown ZnO Thin Films. Phys. Status Solidi C Conf. 2004, 1, 2213–2218. [Google Scholar] [CrossRef]
- Kong, B.H.; Kim, D.C.; Mohanta, S.K.; Cho, H.K. Influence of VI/II Ratios on the Growth of ZnO Thin Films on Sapphire Substrates by Low Temperature MOCVD. Thin Solid Film. 2010, 518, 2975–2979. [Google Scholar] [CrossRef]
- Montenegro, D.N.; Souissi, A.; Martínez-Tomás, C.; Muñoz-Sanjosé, V.; Sallet, V. Morphology Transitions in ZnO Nanorods Grown by MOCVD. J. Cryst. Growth 2012, 359, 122–128. [Google Scholar] [CrossRef]
- Fanni, L.; Aebersold, A.B.; Morales-masis, M.; Alexander, D.T.L.; Hessler-wyser, A.; Nicolay, S. Increasing Polycrystalline Zinc Oxide Grain Size by Control of Film Preferential Orientation. Cryst. Growth Des. 2015, 15, 5886–5891. [Google Scholar] [CrossRef]
- Bui, Q.C.; Ardila, G.; Sarigiannidou, E.; Roussel, H.; Jiménez, C.; Chaix-Pluchery, O.; Guerfi, Y.; Bassani, F.; Donatini, F.; Mescot, X.; et al. Morphology Transition of ZnO from Thin Film to Nanowires on Silicon and Its Correlated Enhanced Zinc Polarity Uniformity and Piezoelectric Responses. ACS Appl. Mater. Interfaces 2020, 12, 29583–29593. [Google Scholar] [CrossRef] [PubMed]
- Tao, R.; Mouis, M.; Ardila, G. Unveiling the Influence of Surface Fermi Level Pinning on the Piezoelectric Response of Semiconducting Nanowires. Adv. Electron. Mater. 2017, 4, 1700299. [Google Scholar] [CrossRef]
- Garcia, A.J.L.; Mouis, M.; Consonni, V.; Ardila, G. Dimensional Roadmap for Maximizing the Piezoelectrical Response of ZnO Nanowire-Based Transducers: Impact of Growth Method. Nanomaterials 2021, 11, 941. [Google Scholar] [CrossRef]
- Jalabert, T.; Pusty, M.; Mouis, M.; Ardila, G. Investigation of the Diameter-Dependent Piezoelectric Response of Semiconducting ZnO Nanowires by Piezoresponse Force Microscopy and FEM Simulations. Nanotechnology 2023, 34, 115402. [Google Scholar] [CrossRef]
- Bui, Q.C.; Ardila, G.; Roussel, H.; Jiménez, C.; Gélard, I.; Chaix-Pluchery, O.; Mescot, X.; Boubenia, S.; Salem, B.; Consonni, V. Tuneable Polarity and Enhanced Piezoelectric Response of ZnO Thin Films Grown by Metal-Organic Chemical Vapour Deposition through the Flow Rate Adjustment. Mater. Adv. 2022, 3, 498–513. [Google Scholar] [CrossRef]
- Janotti, A.; Van De Walle, C.G. Native Point Defects in ZnO. Phys. Rev. B 2007, 76, 165202. [Google Scholar] [CrossRef]
- Chiu, H.-M.; Tsai, H.-J.; Hsu, W.-K.; Wu, J.-M. Experimental and Computational Insights in the Growth of Gallium-Doped Zinc Oxide Nanostructures with Superior Field Emission Properties. CrystEngComm 2013, 15, 5764–5775. [Google Scholar] [CrossRef]
- Lim, T.; Mirabedini, P.S.; Jung, K.; Greaney, P.A. High-Index Crystal Plane of ZnO Nanopyramidal Structures: Stabilization, Growth, and Improved Photocatalytic Performance. Appl. Surf. Sci. 2021, 536, 147326. [Google Scholar] [CrossRef]
- Cuscó, R.; Alarcón-lladó, E.; Ibáñez, J.; Artús, L.; Jiménez, J.; Wang, B.; Callahan, M.J. Temperature Dependence of Raman Scattering in ZnO. Phys. Rev. B 2007, 75, 165202. [Google Scholar] [CrossRef]
- Scepanovic, M.; Grujic-Brojcin, M.; Vojisavljevic, K.; Bernik, S.; Sreckovi, T. Raman Study of Structural Disorder in ZnO Nanopowders. J. Raman Spectrosc. 2010, 41, 914–921. [Google Scholar] [CrossRef]
- Ferrari, A.C.; Robertson, J. Interpretation of Raman Spectra of Disordered and Amorphous Carbon. Phys. Rev. B 2000, 61, 14095. [Google Scholar] [CrossRef] [Green Version]
- Znaidi, L.; Touam, T.; Vrel, D.; Souded, N.; Ben Yahia, S.; Brinza, O.; Fischer, A.; Boudrioua, A. AZO Thin Films by Sol-Gel Process for Integrated Optics. Coatings 2013, 3, 126–139. [Google Scholar] [CrossRef] [Green Version]
- Perillat-Merceroz, G.; Thierry, R.; Jouneau, P.-H.; Ferret, P.; Feuillet, G. Compared Growth Mechanisms of Zn-Polar ZnO Nanowires on O-Polar ZnO and on Sapphire. Nanotechnology 2012, 23, 125702. [Google Scholar] [CrossRef]
- Sallet, V.; Sartel, C.; Vilar, C.; Lusson, A.; Galtier, P. Opposite Crystal Polarities Observed in Spontaneous and Vapour-Liquid-Solid Grown ZnO Nanowires. Appl. Phys. Lett. 2013, 102, 182103. [Google Scholar] [CrossRef]
- Wang, Z.L.; Kong, X.Y.; Zuo, J.M. Induced Growth of Asymmetric Nanocantilever Arrays on Polar Surfaces. Phys. Rev. Lett. 2003, 91, 185502. [Google Scholar] [CrossRef]
- Gao, P.X.; Wang, Z.L. Substrate Atomic-Termination-Induced Anisotropic Growth of ZnO Nanowires/Nanorods by the VLS Process. J. Phys. Chem. B 2004, 108, 7534–7537. [Google Scholar] [CrossRef] [Green Version]
- Consonni, V.; Sarigiannidou, E.; Appert, E.; Bocheux, A.; Guillemin, S.; Donatini, F.; Robin, I.-C.; Kioseoglou, J.; Robaut, F. Selective Area Growth of Well-Ordered ZnO Nanowire Arrays with Controllable Polarity. ACS Nano 2014, 8, 4761–4770. [Google Scholar] [CrossRef] [PubMed]
- Frodason, Y.K.; Johansen, K.M.; Bjørheim, T.S.; Svensson, B.G. Zn Vacancy-Donor Impurity Complexes in ZnO. Phys. Rev. B 2018, 97, 104109. [Google Scholar] [CrossRef] [Green Version]








| O2 Flow Rate (sccm) | DEZn Flow Rate (g/min) | O2/DEZn Ratio | Temperature (°C) | Pressure (mbar) | |
|---|---|---|---|---|---|
| Sample 1 | 100 | 0.5 | 16 | 700 | 3 |
| Sample 2 | 300 | 0.5 | 48 | 700 | 3 |
| Sample 3 * | 500 | 0.5 | 79 | 700 | 3 |
| Sample 4 | 700 | 0.5 | 111 | 700 | 3 |
| Sample 5 | 500 | 0.4 | 99 | 700 | 3 |
| Sample 6 | 500 | 0.3 | 132 | 700 | 3 |
| Sample 7 | 500 | 0.2 | 198 | 700 | 3 |
| Flow Rates | Length (nm) | Diameter (nm) | Aspect Ratio | Density (109 NWs/cm2) |
|---|---|---|---|---|
| 100 sccm O2 0.5 g/min DEZn | 962 ± 78 | 82.3 ± 9.8 | 11.7 ± 2.3 | 9 ± 1 |
| 300 sccm O2 0.5 g/min DEZn | 1429 ± 55 | 74 ± 11.2 | 19.3 ± 3.7 | 13 ± 1 |
| 500 sccm O2 0.5 g/min DEZn | 1125 ± 31 | 72.9 ± 12.2 | 15.4 ± 3.0 | 13 ± 1 |
| 700 sccm O2 0.5 g/min DEZn | 1096 ± 32 | 61.7 ± 5.05 | 17.8 ± 2.0 | 14 ± 1 |
| 500 sccm O2 0.2 g/min DEZn | 362 ± 45 | 42.2 ± 3.8 | 8.6 ± 1.8 | 36 ± 1 |
| 500 sccm O2 0.3 g/min DEZn | 446 ± 75 | 50.5 ± 6.2 | 8.8 ± 2.6 | 30 ± 1 |
| 500 sccm O2 0.4 g/min DEZn | 865 ± 86 | 53.4 ± 7.3 | 16.2 ± 3.8 | 16 ± 1 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Bui, Q.C.; Consonni, V.; Jiménez, C.; Roussel, H.; Mescot, X.; Salem, B.; Ardila, G. Correlation between the Dimensions and Piezoelectric Properties of ZnO Nanowires Grown by PLI-MOCVD with Different Flow Rates. Nanoenergy Adv. 2023, 3, 220-235. https://doi.org/10.3390/nanoenergyadv3030011
Bui QC, Consonni V, Jiménez C, Roussel H, Mescot X, Salem B, Ardila G. Correlation between the Dimensions and Piezoelectric Properties of ZnO Nanowires Grown by PLI-MOCVD with Different Flow Rates. Nanoenergy Advances. 2023; 3(3):220-235. https://doi.org/10.3390/nanoenergyadv3030011
Chicago/Turabian StyleBui, Quang Chieu, Vincent Consonni, Carmen Jiménez, Hervé Roussel, Xavier Mescot, Bassem Salem, and Gustavo Ardila. 2023. "Correlation between the Dimensions and Piezoelectric Properties of ZnO Nanowires Grown by PLI-MOCVD with Different Flow Rates" Nanoenergy Advances 3, no. 3: 220-235. https://doi.org/10.3390/nanoenergyadv3030011
APA StyleBui, Q. C., Consonni, V., Jiménez, C., Roussel, H., Mescot, X., Salem, B., & Ardila, G. (2023). Correlation between the Dimensions and Piezoelectric Properties of ZnO Nanowires Grown by PLI-MOCVD with Different Flow Rates. Nanoenergy Advances, 3(3), 220-235. https://doi.org/10.3390/nanoenergyadv3030011