Next Article in Journal
Solubility and Thermodynamic Data of Febuxostat in Various Mono Solvents at Different Temperatures
Next Article in Special Issue
Selective Oxidation of Glycerol via Acceptorless Dehydrogenation Driven by Ir(I)-NHC Catalysts
Previous Article in Journal
Naphthalene Diimides Carrying Two β-Cyclodextrins Prefer Telomere RNA G-Quadruplex Recognition
 
 
Search for Articles:
Title / Keyword
Author / Affiliation / Email
Journal
Article Type
 
 
Advanced Search
 
Section
Special Issue
Volume
Issue
Number
Page
 
Journals
Molecules
Volume 27
Issue 13
10.3390/molecules27134052
molecules-logo
Submit to this Journal Review for this Journal Propose a Special Issue
► Article Menu

Article Menu

share Share announcement Help format_quote Cite question_answer Discuss in SciProfiles thumb_up
...
Endorse textsms
...
Comment
first_page
settings
Order Article Reprints
Font Type:
Arial Georgia Verdana
Font Size:
Aa Aa Aa
Line Spacing:
Column Width:
Background:
Article

Redox-Active Metal-Organic Frameworks with Three-Dimensional Lattice Containing the m-Tetrathiafulvalene-Tetrabenzoate

by
Hongrui Huang
1,
Zhi-Mei Yang
2,
Xiao-Cheng Zhou
2,
Gen Zhang
1,* and
Jian Su
1,2,*
1
School of Chemistry and Chemical Engineering, Nanjing University of Science and Technology, Nanjing 210094, China
2
State Key Laboratory of Coordination Chemistry, Nanjing University, Nanjing 210023, China
*
Authors to whom correspondence should be addressed.
Molecules 2022, 27(13), 4052; https://doi.org/10.3390/molecules27134052
Submission received: 19 May 2022 / Revised: 21 June 2022 / Accepted: 21 June 2022 / Published: 23 June 2022
(This article belongs to the Special Issue Synthesis, Characterization, and Catalytic Activity of Organometallic Complexes 2022)
Browse Figures
Versions Notes

Abstract

:
Metal-organic frameworks (MOFs) constructed by tetrathiafulvalene-tetrabenzoate (H4TTFTB) have been widely studied in porous materials, while the studies of other TTFTB derivatives are rare. Herein, the meta derivative of the frequently used p-H4TTFTB ligand, m-H4TTFTB, and lanthanide (Ln) metal ions (Tb3+, Er3+, and Gd3+) were assembled into three novel MOFs. Compared with the reported porous Ln-TTFTB, the resulted three-dimensional frameworks, Ln-m-TTFTB ([Ln2(m-TTFTB)(m-H2TTFTB)0.5(HCOO)(DMF)]·2DMF·3H2O), possess a more dense stacking which leads to scarce porosity. The solid-state cyclic voltammetry studies revealed that these MOFs show similar redox activity with two reversible one-electron processes at 0.21 and 0.48 V (vs. Fc/Fc+). The results of magnetic properties suggested Dy-m-TTFTB and Er-m-TTFTB exhibit slow relaxation of the magnetization. Porosity was not found in these materials, which is probably due to the meta-configuration of the m-TTFTB ligand that seems to hinder the formation of pores. However, the m-TTFTB ligand has shown to be promising to construct redox-active or electrically conductive MOFs in future work.

Graphical Abstract

1. Introduction

In the development of functional metal-organic frameworks (MOFs), MOFs with special reactivity are highly demanded. Redox-active MOFs with good stimuli response have shown potential applications in intelligent materials [1,2,3,4]. Focusing on the components of MOFs, the redox activity could arise from variable metal centers, redox-active organic linkers, and/or redox-active guest molecules confined in the cages or channels in the framework. The modulation of different redox states which show distinct physical properties can be achieved via post-synthetic redox reactions or electrochemical methods [5,6,7,8,9]. In various organic building blocks, the electron-rich tetrathiafulvalene (TTF) unit, owing to its two stable oxidized states, has become a famous redox-active building block in the construction of functional MOFs [1,10]. Among the TTF-carboxylate ligands, dimethylthio-tetrathiafulvalene-bicarboxylate (H2TTFBC), tetrathiafulvalene-tetracarboxylate (H4TTFTC), and tetrathiafulvalene-tetrabenzoate (H4TTFTB) are well-researched in the previous works [10,11,12,13,14,15,16,17]. For H4TTFTB, Dincă et al. first reported its synthesis and assembly of Zn2+ in a semiconductive MOF [18]. Since then, the self-assembly of H4TTFTB with diverse metal ions including Cd2+, Mn2+, Co2+, Ba2+, Fe2+/3+, Mg2+, In3+, and Zr4+ has been successfully realized [6,19,20,21,22,23,24,25,26]. _ENREF_15 In addition, the combination of H4TTFTB and lanthanide (Ln) ions has resulted in diverse two dimensional or three-dimensional MOFs [27,28,29,30]. For example, in 2019, Dincă et al. reported three polymorphic MOFs containing La3+ and TTFTB. These MOFs crystallize with unique topologies and exhibit different intermolecular π···π stacking interactions within the TTF moieties [29]. In summary, owing to the large angle between adjacent carboxylates in H4TTFTB, TTFTB-based MOFs always show good porosity.
Comparatively speaking, the meta-derivative of the frequently used p-TTFTB ligand is rarely reported. It can be predicted that H4TTFTB will show a different assembly behavior in the construction of MOFs. In 2020, Zuo et al. firstly reported a 2D MOF, In-m-TTFTB, which possesses a proton conductivity of 6.66 × 10−4 S cm−1 at 303 K and 98% relative humidity (RH) [31]. Recently, a series of persistent radical 2D MOFs were assembled by a hexanuclear rare-earth-cluster-based 1D chains and a (m-TTFTB)3 trimer building block [32]. These MOFs exhibit highly chemical and thermal stability. Due to efficient light absorption, intermolecular charge transfer, low thermal conductivity, and outstanding stability, Dy6-m-TTFTB-MOF shows excellent photothermal properties, an increase of 34.7 °C within 240 s under one-sun illumination [32]. Another kind of m-TTFTB-MOF was obtained by adjusting the synthetic conditions. This MOF possesses a low BET surface area of 129 m2·g−1 with a high near-infrared (NIR) photothermal conversion [33]. Further study revealed that the photothermal conversion of this MOF could be enhanced by redox doping and plasmon resonance. Even though the photothermal conversion of m-TTFTB-based MOFs is carefully studied [31,32], the semiconductive and magnetic properties of these MOFs have not been reached.
The present work reported three MOFs ([Ln2(m-TTFTB)(m-H2TTFTB)0.5(HCOO)(DMF)]·2DMF·3H2O) generated from m-TTFTB (Scheme 1) and lanthanide ions (Ln = Tb3+, Er3+, and Gd3+) using solvothermal methods. The framework consists of an improved inorganic component, a chain of Ln metals bound by carboxylates with a dense stacking. All the MOFs were studied by single-crystal X-ray diffraction structural characterizations and their redox activities, light absorption, electrical conductivities, and magnetic properties are discussed.

2. Results and Discussion

2.1. Crystal Structures

Analyses of the diffraction data for Tb-m-TTFTB, Er-m-TTFTB, and Gd-m-TTFTB (Figure S1) revealed that they are isostructural three-dimensional MOFs crystallizing in the triclinic space group P-1 (Table 1). For Tb-m-TTFTB, two kinds of crystallographic-independent Tb3+ ions and m-TTFTB ligands were observed, respectively (Figure S2). The coordination numbers of Tb1 and Tb2 atoms were both seven (Figure 1a,b). One of the m-TTFTB4− (L1) ligands was coordinated to eight Tb atoms (Figure S3). Another m-H2TTFTB2− (L2) was coordinated to six Tb atoms, and half of the carboxylates were in the protonated state (Figure S3). The Tb−O bond lengths were in the range of 2.266 to 2.478 Å (Table S1), which are comparable to those reported for Tb-MOFs [30,34]. The Tb1 pairs and Tb2 pairs linked by two anti–anti carboxylates (Tb1···Tb2 = 4.70 Å) form the one-dimensional structure (Figure 1c). The Tb pairs both have four bridging syn–syn carboxylates (four-blade paddle-wheel; Tb1···Tb1 = 4.39 Å, Tb2···Tb2 = 4.20 Å). In the reported structural parameters of Tb-TTFTB, the distances of Tb1···Tb1, Tb2···Tb2, and Tb1···Tb2 were 4.56, 4.21, and 5.47 Å, respectively [34]. In the chain structure of Tb-m-TTFTB, the increased number of binding carboxylates between Tb1···Tb1 and Tb1···Tb2 compared with Tb-TTFTB enhanced the interaction of the Tb centers and thus reduced the relative distance. Each one-dimensional chain is linked by the m-TTFTB4− (Figure 1e) to generate the two-dimensional network. The weak interactions of S···S (3.57 Å) and C···C (3.79 Å) in Tb-m-TTFTB, S···S (3.50 Å) and C···C (3.74 Å) in Er-m-TTFTB, and S···S (3.56 Å) and C···C (3.74 Å) in Gd-m-TTFTB were observed (Figure 1f). Notably, these S···S distances between the TTF linkers are even smaller than the distance in the conducting framework of Cd2(TTFTB) (3.65 Å) [19]. Finally, the three-dimensional framework of Tb-m-TTFTB (Figure 1d) can be assembled by the 2D plane in Figure 1e and interacted m-TTFTB4−. The three-dimensional structures along three unit cell axes showed non-porosity (Figure S4).
The absence of any other phases from Tb-m-TTFTB, Er-m-TTFTB, and Gd-m-TTFTB was confirmed by powder X-ray diffraction (PXRD) measurements in which the diffraction peak positions were similar to the calculated from single-crystal X-ray data of Tb-m-TTFTB (Figure 2). The advantages in stability originated from the interaction of TTF matrix and the protecting of the tight organic parts surrounding the rare earth centers (Figure 1c) [33]. In this case, reducing of coordination space is an efficient strategy to enhance the stability of the frameworks. The thermal stability was investigated. The TGA data for Ln-m-TTFTB showed that the frameworks have good thermal stability up to 450 °C (Figure S5) in the N2 atmosphere, which is comparable to the Ln-TTFTB series (nearly 500 °C) [28,34].

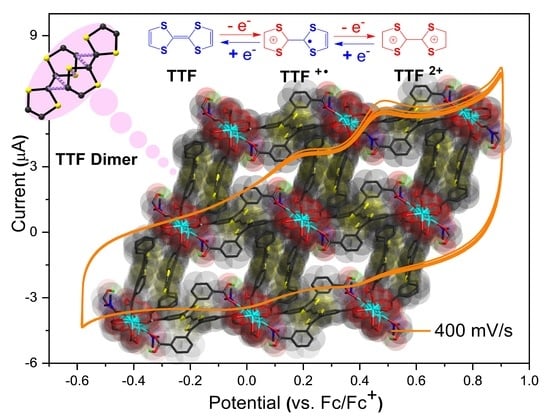
2.2. Cyclic Voltammetry

Solid-state direct current (DC) cyclic voltammetry (CV) studies on Ln-m-TTFTB were conducted in 0.1 M LiBF4 in CH3CN (Figure 3a and Figures S6 and S7). Upon scanning anodically, two reversible one-electron processes at around 0.21 and 0.48 V (vs. Fc/Fc+) were observed for all three MOFs. These processes are attributed to the TTF/TTF•+ and TTF•+/TTF2+ redox couples, respectively (inset of Figure 3a). In contrast to the CV of m-H4TTFTB (0.13 and 0.35 V (vs. Fc/Fc+)) [31], the two one-electron processes observed for Tb-m-TTFTB were shifted by ca. 0.1 V, which is attributed to both the coordination to terbium ions and the deprotonated nature of the ligand [35]. The current associated with the two redox processes were almost the same over multiple scans which is consistent with their reversible nature. In addition, faster sweep rates led to broader features because of slow diffusion kinetics through the framework (Figure 3b and Figures S6 and S7). The locations of these redox couples in Ln-m-TTFTB were similar to those of Ln-TTFTB, meaning that these two series of MOFs possess similar redox activity [34].

2.3. Absorption Spectra and Semiconducting Properties

Prior to studying the conductivities of these materials, the solid-state absorption spectra were obtained to gain insight into the influence of coordination on the optical and conducting properties. In Ln-m-TTFTB, there are three main absorption bands located in the region 250–550 nm (Figure 4a). These higher energy absorption bands are attributed to the n→π* or π→π* transition of the free ligand, which is similar to the absorption of the ligand m-H4TTFTB [33]. The absorption band around 760 nm can be assigned to the neutral (TTF)2 in the framework [36,37]. The peak located at 690 nm of the free ligand can be attributed to a small number of auto-oxidized TTF•+. Using these UV-vis-NIR adsorption data, we approximated the band gaps of the ligand and Ln-m-TTFTB through the Kubelka–Munk function. From the Tauc plots [38], the band gaps of m-H4TTFTB, Gd-m-TTFTB, Tb-m-TTFTB, and Er-m-TTFTB were approximately 1.87, 1.89, 1.90, and 1.92 eV, respectively (Figure 4b). In general, these values are similar to the Ln-TTFTB series. This result indicated that the different locations of the carboxyl groups have a slight influence in the band gap.
To better understand the conducting behaviors of these MOFs, conductivity studies were undertaken on the single crystal samples of Ln-m-TTFTB by the two-contact probe method. The room temperature electrical conductivities in the long horizontal direction of the single crystal (Figure S8) were 5.4 × 10−7, 9.6 × 10−7, and 1.0 × 10−7 S·cm−1 for Tb-m-TTFTB, Er-m-TTFTB, and Gd-m-TTFTB, respectively (Figures S9–S11 and Table S2). These values are nearly ten times of the reported Ln-TTFTB series (10−8 S·cm−1 in powder state), and this can be attributed to the lower contact resistance in single crystal (also ~10−8 S·cm−1 in powder state). The lower conducting performances of Ln-m-TTFTB originate from the lack of band formation or the poor electron transfer. Notably, the powder electrical conductivity is reported as 2.74 × 10−8 S·cm−1 for the free m-H4TTFTB [31]. The similar electrical conductivity between the free ligand and the assembled MOFs are predicted by the similar band gaps.

2.4. Magnetic Properties

Magnetic susceptibility measurements on fresh polycrystalline samples of Tb-m-TTFTB, Dy-m-TTFTB, and Er-m-TTFTB were performed in the temperature range 2–300 K under an applied magnetic field of 1000 Oe. Their χMT values at 300 K were 23.59, 28.01, and 23.22 cm3·K·mol−1 for Tb-m-TTFTB, Dy-m-TTFTB, and Er-m-TTFTB, respectively (Figure 5). These values are consistent with theoretical values for two independent Ln3+ ions, 23.64 cm3·K·mol−1 (Tb3+, 7F6, J = 6, g = 3/2), 28.34 cm3·K·mol−1 (Dy3+, 6H15/2, J = 15/2, g = 4/3), and 22.95 cm3·K·mol−1 (Er3+, 4I15/2, J = 15/2, g = 6/5) [34]. As the temperature was lowered, χMT decreased in each case as expected from antiferromagnetic interaction and/or the depopulation of excited states [39,40]. Focusing on the crystal structures, we can define three exchange interactions (Figure S12) within one chain due to the three different kinds of lanthanide dimer. Given that the Gd3+ ion is magnetically isotropic, the χMT of Gd-m-TTFTB (Figure S13) decreased in the high-temperature region due to the antiferromagnetic exchange interaction with J = −1 cm−1 ( H ^ E X = − 2 J 1 S G d 1 S G d 2 ) in dimers. In the low-temperature region, the slight upturn might be attributed to the ferromagnetic interaction and/or dipolar–dipolar interaction between dimers, or ferromagnetic intra-chain interaction. For Er-m-TTFTB, an upturn of the moment below ca. 6 K indicates one possible weak nearest neighbor ferromagnetic interaction and/or dipolar–dipolar interaction [41]. At 1.8 K, the isothermal magnetizations (M) versus field (H) reached 10.84, 11.71, and 11.08 Nβ in 70 kOe for Tb-m-TTFTB, Dy-m-TTFTB, and Er-m-TTFTB, respectively (Figures S14–S16). The low saturation of magnetization values maybe ascribed to the effects of low-lying excited states and/or magnetic anisotropy [42].
The temperature dependence of the alternating current (ac) susceptibilities (2 Oe) at different fixed frequencies (1.0–999 Hz) were measured for all MOFs. Under zero direct current (dc) field, Dy-m-TTFTB exhibited both in-phase (χ′) and out-of-phase (χ″) ac-susceptibilities (Figure 6a), but no peaks were observed. The ac-susceptibilities of Er-m-TTFTB and Tb-m-TTFTB exhibited no χ″ in zero dc field (Figures S17a and S18a). The frequency-dependent out-of-phase (χ″) ac-susceptibility below 6 K for Dy-m-TTFTB revealed the slow relaxation of the magnetization. However, no peak of χ″ was observed even at 999 Hz, likely due to a lower anisotropic energy barrier. The χ″ susceptibility increased with the increase of the frequency, suggesting that the peak maxima are to be found at lower temperatures or higher frequencies of the SQUID instrument. Even an increase in the dc field to 1000 Oe showed no peak above 2 K in the ac-susceptibilities of Dy-m-TTFTB and Er-m-TTFTB, while Tb-m-TTFTB exhibited no χ″ signals (Figure 6b, Figures S17b and S18b). With low energy barriers, no peaks above 1.8 K in the out-of-phase of ac susceptibility observed in the frequency region of 1.0–999 Hz were reasonable.

3. Materials and Methods

3.1. Materials and Methods

All the reagents and solvents were commercially available and used as received. FT-IR spectra were recorded on a Vector 27 Bruker Spectrophotometer by transmission through KBr pellets containing the ground crystals in the range 4000–400 cm−1. The powder X-ray diffraction patterns (PXRD) were collected at room temperature using a scan speed of 0.1 s/step on a Bruker Advance D8 diffractometer (40 kV, 40 mA) (Bruker, Karlsruhe, Germany) equipped with Cu radiation. Calculated PXRD patterns were generated using Mercury 3.0 [43]. Elemental analyses (EA) for C, H, and N were performed on a Perkin-Elmer 240C analyzer (PerkinElmer, Waltham, MA, USA). TGA data were obtained on a STA 449C thermal analysis system at a heating rate of 10 °C min−1 under N2 atmosphere. Magnetization measurements were performed using a Quantum Design SQUID VSM magnetometer (Quantum Design, Darmstadt, Germany) on polycrystalline samples for all compounds.

3.2. X-ray Structure

Single-crystal X-ray diffraction intensity data for Tb-m-TTFTB, Er-m-TTFTB, and Gd-m-TTFTB were collected on a Bruker D8 Venture diffractometer fitted with a PHOTON-100 CMOS detector, monochromatized microfocus Mo Kα radiation (λ = 0.71073 Å), and a nitrogen flow controlled by a KRYOFLEX II low-temperature attachment operating at 153 K. Raw data collection and reduction were controlled using APEX3 software (version 2016.9-0; Bruker, 2016) [44]. Absorption corrections were applied using the SADABS routine. The structures were solved by direct methods and refined by full-matrix least-squares on F2 using the SHELXTL software package (version-2018/3) [45]. Non-hydrogen atoms were refined with anisotropic displacement parameters during the final cycles. Hydrogen atoms of m-TTFTB, formate, and dimethylformamide (DMF) molecules were placed at calculated ideal positions and isotropic displacement parameters were used. Except for the coordinated DMF molecule, those free solvent molecules of DMF or water were highly disordered and were unable to be located and refined. The diffuse electron densities resulting from these residual molecules were removed from the data set using the SQUEEZE routine of PLATON and refined further using the data generated [46]. The final formulas of Tb-m-TTFTB, Er-m-TTFTB, and Gd-m-TTFTB ([Ln2(m-TTFTB)(m-H2TTFTB)0.5(HCOO)(DMF)]·2DMF·3H2O) were calculated from the SQUEEZE results and combined with charge balance, elemental analysis and TGA data. CCDC 1,914,385 (Tb-m-TTFTB), 1,914,387 (Er-m-TTFTB), and 1,914,384 (Gd-m-TTFTB) contain the supplementary crystallographic data for this paper. These data can be obtained free of charge from the Cambridge Crystallographic Data Centre via www.ccdc.cam.ac.uk/data_request/cif (accessed on 8 February 2020) using the accession identifiers CCDC-1,914,384, CCDC-1,914,385 and CCDC-1,914,387, respectively.

3.3. Synthesis of m-H4TTFTB

m-H4TTFTB (Figure S19) was prepared according to the reported method [31]. The method is briefly described in the supporting information.

3.4. Synthesis of Dy-m-TTFTB, [Dy2(m-TTFTB)(m-H2TTFTB)0.5 (HCOO)(DMF)]·2DMF·3H2O

Dy-m-TTFTB was prepared according to the reported method [33].

3.5. Synthesis of Tb-m-TTFTB, [Tb2(m-TTFTB)(m-H2TTFTB)0.5 (HCOO)(DMF)]·2DMF·3H2O

The dissolution of m-H4TTFTB (0.010 g, 0.015 mmol) in DMF (1 mL) was performed before addition of a solution of TbCl3·6H2O (0.010 g, ~0.027 mmol) in H2O (0.5 mL) followed by the addition of CF3COOH (0.17 mL) and chlorobenzene (2 mL). The mixture was heated to 140 °C for 48 h, and then allowed to cool to room temperature. Red rod-like crystals (0.009 g) of Tb-m-TTFTB were filtered and washed with DMF and CH3COCH3 three times. Yield 54% (based on m-H4TTFTB). Calcd for C61H53N3O20S6Tb2 (Mr = 1658.32 g/mol): C, 44.18; H, 3.22; N, 2.53%. Found: C, 43.58; H, 3.30; N, 2.32%. FT-IR (KBr, cm−1): 2929 w, 2360 w, 2341 w, 1676 m, 1653 m, 1635m, 1559 s, 1507 w, 1430 s, 1395 vs, 1254 w, 1163 w, 1083 w, 1000 w, 924 w, 799 m, 763 s, 684 m, 668 s, 657 s, 627 w, 557 w, 442 m.

3.6. Synthesis of Er-m-TTFTB, [Er2(m-TTFTB)(m-H2TTFTB)0.5(HCOO)(DMF)]·2DMF·3H2O

Er-m-TTFTB were synthesized under similar conditions of Tb-m-TTFTB except ErCl3·6H2O (0.010 g, ~0.027 mmol). Red rod-like crystals (0.010 g) of Er-m-TTFTB were filtered and washed with DMF and CH3COCH3 three times. Yield 60% (based on m-H4TTFTB). Calcd for C61H53N3O20S6Er2 (Mr = 1674.99 g/mol): C, 43.74; H, 3.19; N, 2.51%. Found: C, 42.65; H, 3.30; N, 2.43%. FT-IR (KBr, cm−1): 3057 w, 2929 w, 2360 w, 2343 w, 1680 m, 1653 m, 1636 m, 1590 m, 1569 m, 1430 s, 1400 vs, 1335 w, 1280 w, 1256 w, 1163 w, 1086 w, 1000 w, 924 w, 800 m, 763 s, 685 m, 668 m, 658 s, 640 w, 625 w, 560 w, 442 m.

3.7. Synthesis of Gd-m-TTFTB, [Gd2(m-TTFTB)(m-H2TTFTB)0.5(HCOO)(DMF)]·2DMF·3H2O

Gd-m-TTFTB. Red rod-like crystals (0.008 g) of Gd-m-TTFTB were filtered and washed with DMF and CH3COCH3 three times. Yield 48% (based on m-H4TTFTB). Calcd for C61H53N3O20S6Gd2 (Mr = 1654.97 g/mol): C, 44.27; H, 3.23; N, 2.54%. Found: C, 43.82; H, 3.11; N, 2.39%. FT-IR (KBr, cm−1): 2929 w, 2360 w, 2341 w, 1676 m, 1632 m, 1590 m, 1560 s,1428 s, 1399 vs, 1255 w, 1084 w, 1000 w, 924 w, 799 m, 763 s, 690 m, 668 m, 657 s, 640 w, 627 w, 556 w, 442 m.

3.8. Solid CV

Solid-state cyclic voltammetry measurements were performed in LiBF4/CH3CN as electrolyte using a Corrtest 4-channel electrochemical workstation and a three-electrode system. The CVs were recorded using a glassy carbon working electrode (3.0 mm diameter), a platinum wire auxiliary electrode, and an Ag wire quasi-reference electrode with the solutions of 0.1 M LiBF4 dissolved in distilled CH3CN. The sample was mounted on the glassy carbon working electrode by dipping the electrode into a paste made of the powder sample in ethanol. Ferrocene was added as an internal standard upon completion of each experiment. All potentials are reported in milli-Volts (mV) versus the Fc/Fc+ couple.

3.9. Solid-State Diffuse Reflectance Spectra

The UV-Vis-NIR data were obtained using a Harrick Praying Mantis attachment over the range 200–900 nm. Spectra are reported as the Kubelka–Munk transform.
Kubelka–Munck function:
K = (1 − R)2/2R
(Khυ)1/2 = B(hυ − Eg)
hυ: photon energy; K: reflection coefficient; B: characteristic constant of material; Eg: band gap.

3.10. Electrical Conductivity

The electrical conductivities of the needle-like single crystal samples using the two-probe method were obtained using a Keithley 2400 source meter (Keithley 2400, Tektronix, Beaverton, OR, USA) on CRX-4K Closed Cycle Refrigerator-based Probe Station at room temperature. The single crystal samples were lined in the vertical direction, which were connected by conductive carbon adhesive. All of the current-voltage (I–V) measurements were performed under ambient conditions by sweeping the voltage from −1.5 V to 1.5 V.

4. Conclusions

In summary, three redox-active MOFs were constructed by lanthanide metal ions (Tb3+, Er3+, and Gd3+) and m-TTFTB. These MOFs showed similar three-dimensional lattice with a dense stacking. It can be concluded that compared with H4TTFTB, the assembly of m-H4TTFTB tends to form a structure with almost no porosity. Magnetic study revealed that Dy-m-TTFTB and Er-m-TTFTB possess slow relaxation of the magnetization. In all, even though m-TTFTB-based MOFs seem not to be a good porous material, these dense stacking structures may enable them as good candidates for the study of charge transfer, electrical conductivity, and magnetic properties. Further studies focusing on the functional assembly of m-H4TTFTB and other metal building blocks are currently in progress in our group.

Supplementary Materials

The following supporting information can be downloaded at: https://www.mdpi.com/article/10.3390/molecules27134052/s1, Table S1: Selected bond lengths (Å) and angles (°) of Tb-m-TTFTB, Er-m-TTFTB, and Gd-m-TTFTB; Table S2: The shape parameters of Tb-m-TTFTB, Er-m-TTFTB, and Gd-m-TTFTB single crystals used for the calculating of electrical conductivity; Figure S1: The crystal photos of Tb-m-TTFTB (a), Er-m-TTFTB (b), and Gd-m-TTFTB (c); Figure S2: The asymmetric units of Tb-m-TTFTB. Displacement ellipsoids are drawn at the 50% probability level; Figure S3: The coordination environment of L1 (top) and L2 (down) in Tb-m-TTFTB; Figure S4. The three-dimensional structures of Tb-m-TTFTB along a, b, and c directions. Figure S5: The TGA plots of Tb-m-TTFTB, Er-m-TTFTB, and Gd-m-TTFTB under an N2 atmosphere; Figure S6: Solid state cyclic voltammograms of Er-m-TTFTB performed over three consecutive cycles (a) and at different scan rates (b). The experiments were conducted in 0.1 M LiBF4 in CH3CN electrolyte; Figure S7: Solid-state cyclic voltammograms of Gd-m-TTFTB were performed over three consecutive cycles (a) and at different scan rates (b). The experiments were conducted in 0.1 M LiBF4 in CH3CN electrolyte; Figure S8: The picture of the single crystal of Tb-m-TTFTB and the electric device used for the measurement of electrical conductivity; Figure S9: I–V curve of Tb-m-TTFTB; Figure S10: I–V curve of Er-m-TTFTB; Figure S11: I–V curve of Gd-m-TTFTB; Figure S12: Three kinds of exchange interactions in one-dimensional chain of Gd-m-TTFTB; Figure S13: Temperature dependence of the χMT for Gd-m-TTFTB measured in a 1000 Oe field. The red line is the simulation of two isolated Gd ions. The blue line is the simulation of Gd2 cluster only existing magnetic coupling; Figure S14: The field-dependent magnetizations from 0 to 70 kOe at 1.8 K for Tb-m-TTFTB; Figure S15: The field-dependent magnetizations from 0 to 70 kOe at 1.8 K for Dy-m-TTFTB; Figure S16: The field-dependent magnetizations from 0 to 70 kOe at 1.8 K for Er-m-TTFTB; Figure S17: (a) Temperature-dependent in-phase χ′ and out-of-phase χ″ ac susceptibility signals for Tb-m-TTFTB at the indicated frequencies under zero dc field. (b) Temperature-dependent in-phase χ′ and out-of-phase χ″ ac susceptibility signals for Tb-m-TTFTB at the indicated frequencies under 1000 dc field; Figure S18: (a) Temperature-dependent in-phase χ′ and out-of-phase χ″ ac susceptibility signals for Er-m-TTFTB at the indicated frequencies under zero dc field. (b) Temperature-dependent in-phase χ′ and out-of-phase χ″ ac susceptibility signals for Er-m-TTFTB at the indicated frequencies under 1000 dc field; Figure S19. The synthesis route of m-H4TTFTB.

Author Contributions

Conceptualization, J.S. and G.Z.; methodology, J.S.; formal analysis, H.H., Z.-M.Y., X.-C.Z. and J.S.; investigation, H.H., Z.-M.Y., X.-C.Z. and J.S.; writing—original draft preparation, H.H.; writing—review and editing, H.H., J.S. and G.Z.; supervision, J.S. and G.Z. All authors have read and agreed to the published version of the manuscript.

Funding

This research was funded by the Startup Funding from Nanjing University of Science and Technology (AE89991/376, AD41913) and the National Natural Science Foundation of China (22171136). G.Z. acknowledges the support of the Thousand Young Talent Plan.

Institutional Review Board Statement

Not applicable.

Informed Consent Statement

Not applicable.

Data Availability Statement

CCDC 1914384, 1914385, and 1914387 contains the supplementary crystallographic data for this paper. These data are provided free of charge by the joint Cambridge Crystallographic Data Centre www.ccdc.cam.ac.uk/structures (accessed on 8 February 2020) using the accession identifiers CCDC-1,914,384, CCDC-1,914,385 and CCDC-1,914,387, respectively. All other data can be obtained from the authors on request.

Acknowledgments

The authors are grateful to Jing-Lin Zuo in Nanjing University for his help on this work.

Conflicts of Interest

The authors declare no conflict of interest.

Sample Availability

Samples of the compounds are available from the authors.

References

  1. Ding, B.; Solomon, M.B.; Leong, C.F.; D’Alessandro, D.M. Redox-active ligands: Recent advances towards their incorporation into coordination polymers and metal-organic frameworks. Coord. Chem. Rev. 2021, 439, 213891. [Google Scholar] [CrossRef]
  2. D’Alessandro, D.M. Exploiting redox activity in metal-organic frameworks: Concepts, trends and perspectives. Chem. Commun. 2016, 52, 8957–8971. [Google Scholar] [CrossRef] [PubMed] [Green Version]
  3. Calbo, J.; Golomb, M.J.; Walsh, A. Redox-active metal–organic frameworks for energy conversion and storage. J. Mater. Chem. A 2019, 7, 16571–16597. [Google Scholar] [CrossRef]
  4. Souto, M.; Strutynski, K.; Melle-Franco, M.; Rocha, J. Electroactive Organic Building Blocks for the Chemical Design of Functional Porous Frameworks (MOFs and COFs) in Electronics. Chem. Eur. J. 2020, 26, 10912–10935. [Google Scholar] [CrossRef] [PubMed]
  5. Usov, P.M.; Fabian, C.; D’Alessandro, D.M. Rapid determination of the optical and redox properties of a metal-organic framework via in situ solid state spectroelectrochemistry. Chem. Commun. 2012, 48, 3945–3947. [Google Scholar] [CrossRef]
  6. Su, J.; Yuan, S.; Wang, H.Y.; Huang, L.; Ge, J.Y.; Joseph, E.; Qin, J.; Cagin, T.; Zuo, J.L.; Zhou, H.C. Redox-switchable breathing behavior in tetrathiafulvalene-based metal-organic frameworks. Nat. Commun. 2017, 8, 2008. [Google Scholar] [CrossRef] [PubMed]
  7. Litvinova, Y.M.; Gayfulin, Y.M.; Kovalenko, K.A.; Samsonenko, D.G.; van Leusen, J.; Korolkov, I.V.; Fedin, V.P.; Mironov, Y.V. Multifunctional Metal-Organic Frameworks Based on Redox-Active Rhenium Octahedral Clusters. Inorg. Chem. 2018, 57, 2072–2084. [Google Scholar] [CrossRef]
  8. Aubrey, M.L.; Wiers, B.M.; Andrews, S.C.; Sakurai, T.; Reyes-Lillo, S.E.; Hamed, S.M.; Yu, C.J.; Darago, L.E.; Mason, J.A.; Baeg, J.O.; et al. Electron delocalization and charge mobility as a function of reduction in a metal-organic framework. Nat. Mater. 2018, 17, 625–632. [Google Scholar] [CrossRef]
  9. Monni, N.; Oggianu, M.; Ashoka Sahadevan, S.; Mercuri, M.L. Redox Activity as a Powerful Strategy to Tune Magnetic and/or Conducting Properties in Benzoquinone-Based Metal-Organic Frameworks. Magnetochemistry 2021, 7, 109. [Google Scholar] [CrossRef]
  10. Wang, H.-Y.; Cui, L.; Xie, J.-Z.; Leong, C.F.; D’Alessandro, D.M.; Zuo, J.-L. Functional coordination polymers based on redox-active tetrathiafulvalene and its derivatives. Coord. Chem. Rev. 2017, 345, 342–361. [Google Scholar] [CrossRef]
  11. Huo, P.; Chen, T.; Hou, J.L.; Yu, L.; Zhu, Q.Y.; Dai, J. Ligand-to-Ligand Charge Transfer within Metal-Organic Frameworks Based on Manganese Coordination Polymers with Tetrathiafulvalene-Bicarboxylate and Bipyridine Ligands. Inorg. Chem. 2016, 55, 6496–6503. [Google Scholar] [CrossRef] [PubMed]
  12. Qin, Y.R.; Zhu, Q.Y.; Huo, L.B.; Shi, Z.; Bian, G.Q.; Dai, J. Tetrathiafulvalene-tetracarboxylate: An intriguing building block with versatility in coordination structures and redox properties. Inorg. Chem. 2010, 49, 7372–7381. [Google Scholar] [CrossRef] [PubMed]
  13. Huang, Y.D.; Huo, P.; Shao, M.Y.; Yin, J.X.; Shen, W.C.; Zhu, Q.Y.; Dai, J. A new type of charge-transfer salts based on tetrathiafulvalene-tetracarboxylate coordination polymers and methyl viologen. Inorg. Chem. 2014, 53, 3480–3487. [Google Scholar] [CrossRef]
  14. Jiang, M.; Weng, Y.G.; Zhou, Z.Y.; Ge, C.Y.; Zhu, Q.Y.; Dai, J. Cobalt Metal-Organic Frameworks Incorporating Redox-Active Tetrathiafulvalene Ligand: Structures and Effect of LLCT within the MOF on Photoelectrochemical Properties. Inorg. Chem. 2020, 59, 10727–10735. [Google Scholar] [CrossRef]
  15. Zhang, S.; Panda, D.K.; Yadav, A.; Zhou, W.; Saha, S. Effects of intervalence charge transfer interaction between pi-stacked mixed valent tetrathiafulvalene ligands on the electrical conductivity of 3D metal-organic frameworks. Chem. Sci. 2021, 12, 13379–13391. [Google Scholar] [CrossRef] [PubMed]
  16. Hu, J.J.; Li, Y.G.; Wen, H.R.; Liu, S.J.; Peng, Y.; Liu, C.M. A family of lanthanide metal-organic frameworks based on a redox-active tetrathiafulvalene-dicarboxylate ligand showing slow relaxation of magnetisation and electronic conductivity. Dalton Trans. 2021, 50, 14714–14723. [Google Scholar] [CrossRef] [PubMed]
  17. Leong, C.F.; Chan, B.; Faust, T.B.; D’Alessandro, D.M. Controlling charge separation in a novel donor–acceptor metal–organic framework via redox modulation. Chem. Sci. 2014, 5, 4724–4728. [Google Scholar] [CrossRef] [Green Version]
  18. Narayan, T.C.; Miyakai, T.; Seki, S.; Dinca, M. High charge mobility in a tetrathiafulvalene-based microporous metal-organic framework. J. Am. Chem. Soc. 2012, 134, 12932–12935. [Google Scholar] [CrossRef] [Green Version]
  19. Park, S.S.; Hontz, E.R.; Sun, L.; Hendon, C.H.; Walsh, A.; van Voorhis, T.; Dinca, M. Cation-dependent intrinsic electrical conductivity in isostructural tetrathiafulvalene-based microporous metal-organic frameworks. J. Am. Chem. Soc. 2015, 137, 1774–1777. [Google Scholar] [CrossRef] [Green Version]
  20. Chen, B.; Lv, Z.-P.; Leong, C.F.; Zhao, Y.; D’Alessandro, D.M.; Zuo, J.-L. Crystal Structures, Gas Adsorption, and Electrochemical Properties of Electroactive Coordination Polymers Based on the Tetrathiafulvalene-Tetrabenzoate Ligand. Cryst. Growth Des. 2015, 15, 1861–1870. [Google Scholar] [CrossRef]
  21. Souto, M.; Santiago-Portillo, A.; Palomino, M.; Vitorica-Yrezabal, I.J.; Vieira, B.J.C.; Waerenborgh, J.C.; Valencia, S.; Navalon, S.; Rey, F.; Garcia, H.; et al. A highly stable and hierarchical tetrathiafulvalene-based metal-organic framework with improved performance as a solid catalyst. Chem. Sci. 2018, 9, 2413–2418. [Google Scholar] [CrossRef] [PubMed] [Green Version]
  22. Souto, M.; Romero, J.; Calbo, J.; Vitorica-Yrezabal, I.J.; Zafra, J.L.; Casado, J.; Orti, E.; Walsh, A.; Minguez Espallargas, G. Breathing-Dependent Redox Activity in a Tetrathiafulvalene-Based Metal-Organic Framework. J. Am. Chem. Soc. 2018, 140, 10562–10569. [Google Scholar] [CrossRef] [PubMed] [Green Version]
  23. Park, S.S.; Hendon, C.H.; Fielding, A.J.; Walsh, A.; O’Keeffe, M.; Dinca, M. The Organic Secondary Building Unit: Strong Intermolecular pi Interactions Define Topology in MIT-25, a Mesoporous MOF with Proton-Replete Channels. J. Am. Chem. Soc. 2017, 139, 3619–3622. [Google Scholar] [CrossRef] [PubMed] [Green Version]
  24. Park, S.S.; Rieth, A.J.; Hendon, C.H.; Dinca, M. Selective Vapor Pressure Dependent Proton Transport in a Metal-Organic Framework with Two Distinct Hydrophilic Pores. J. Am. Chem. Soc. 2018, 140, 2016–2019. [Google Scholar] [CrossRef] [PubMed]
  25. Cadiau, A.; Xie, L.S.; Kolobov, N.; Shkurenko, A.; Qureshi, M.; Tchalala, M.R.; Park, S.S.; Bavykina, A.; Eddaoudi, M.; Dincă, M.; et al. Toward New 2D Zirconium-Based Metal–Organic Frameworks: Synthesis, Structures, and Electronic Properties. Chem. Mater. 2019, 32, 97–104. [Google Scholar] [CrossRef]
  26. Su, J.; Yuan, S.; Wang, T.; Lollar, C.T.; Zuo, J.L.; Zhang, J.; Zhou, H.C. Zirconium metal-organic frameworks incorporating tetrathiafulvalene linkers: Robust and redox-active matrices for in situ confinement of metal nanoparticles. Chem. Sci. 2020, 11, 1918–1925. [Google Scholar] [CrossRef] [Green Version]
  27. Xie, L.S.; Dinca, M. Novel Topology in Semiconducting Tetrathiafulvalene Lanthanide Metal-Organic Frameworks. Isr. J. Chem. 2018, 58, 1119–1122. [Google Scholar] [CrossRef]
  28. Su, J.; Yuan, S.; Li, J.; Wang, H.Y.; Ge, J.Y.; Drake, H.F.; Leong, C.F.; Yu, F.; D’Alessandro, D.M.; Kurmoo, M.; et al. Rare-Earth Metal Tetrathiafulvalene Carboxylate Frameworks as Redox-Switchable Single-Molecule Magnets. Chem. Eur. J. 2021, 27, 622–627. [Google Scholar] [CrossRef]
  29. Xie, L.S.; Alexandrov, E.V.; Skorupskii, G.; Proserpio, D.M.; Dinca, M. Diverse pi-pi stacking motifs modulate electrical conductivity in tetrathiafulvalene-based metal-organic frameworks. Chem. Sci. 2019, 10, 8558–8565. [Google Scholar] [CrossRef] [Green Version]
  30. Castells-Gil, J.; Manas-Valero, S.; Vitorica-Yrezabal, I.J.; Ananias, D.; Rocha, J.; Santiago, R.; Bromley, S.T.; Baldovi, J.J.; Coronado, E.; Souto, M.; et al. Electronic, Structural and Functional Versatility in Tetrathiafulvalene-Lanthanide Metal-Organic Frameworks. Chem. Eur. J. 2019, 25, 12636–12643. [Google Scholar] [CrossRef] [Green Version]
  31. Su, J.; He, W.; Li, X.-M.; Sun, L.; Wang, H.-Y.; Lan, Y.-Q.; Ding, M.; Zuo, J.-L. High Electrical Conductivity in a 2D MOF with Intrinsic Superprotonic Conduction and Interfacial Pseudo-capacitance. Matter 2020, 2, 711–722. [Google Scholar] [CrossRef] [Green Version]
  32. Su, J.; Xu, N.; Murase, R.; Yang, Z.M.; D’Alessandro, D.M.; Zuo, J.L.; Zhu, J. Persistent Radical Tetrathiafulvalene-Based 2D Metal-Organic Frameworks and Their Application in Efficient Photothermal Conversion. Angew. Chem. Int. Ed. 2021, 60, 4789–4795. [Google Scholar] [CrossRef] [PubMed]
  33. Su, J.; Cai, P.; Yan, T.; Yang, Z.M.; Yuan, S.; Zuo, J.L.; Zhou, H.C. Enhancing the photothermal conversion of tetrathiafulvalene-based MOFs by redox doping and plasmon resonance. Chem. Sci. 2022, 13, 1657–1664. [Google Scholar] [CrossRef] [PubMed]
  34. Su, J.; Hu, T.H.; Murase, R.; Wang, H.Y.; D’Alessandro, D.M.; Kurmoo, M.; Zuo, J.L. Redox Activities of Metal-Organic Frameworks Incorporating Rare-Earth Metal Chains and Tetrathiafulvalene Linkers. Inorg. Chem. 2019, 58, 3698–3706. [Google Scholar] [CrossRef] [PubMed]
  35. Leong, C.F.; Wang, C.-H.; Ling, C.D.; D’Alessandro, D.M. A spectroscopic and electrochemical investigation of a tetrathiafulvalene series of metal–organic frameworks. Polyhedron 2018, 154, 334–342. [Google Scholar] [CrossRef]
  36. Yoshizawa, M.; Kumazawa, K.; Fujita, M. Room-temperature and solution-state observation of the mixed-valence cation radical dimer of tetrathiafulvalene, [(TTF)2]+*, within a self-assembled cage. J. Am. Chem. Soc. 2005, 127, 13456–13457. [Google Scholar] [CrossRef]
  37. Lyskawa, J.; Salle, M.; Balandier, J.Y.; le Derf, F.; Levillain, E.; Allain, M.; Viel, P.; Palacin, S. Monitoring the formation of TTF dimers by Na+ complexation. Chem. Commun. 2006, 21, 2233–2235. [Google Scholar] [CrossRef] [PubMed]
  38. Tauc, J.; Grigorovici, R.; Vancu, A. Optical Properties and Electronic Structure of Amorphous Germanium. Phys. Status Solidi B 1966, 15, 627–637. [Google Scholar] [CrossRef]
  39. Layfield, R.A.; Murugesu, M. Lanthanides and Actinides in Molecular Magnetism; Wiley-VCH: Weinheim, Germany, 2014. [Google Scholar]
  40. Bosch, M. Lanthanide Metal-Organic Frameworks; Springer-Verlag: Berlin/Heidelberg, Germany, 2015. [Google Scholar]
  41. Jung, J.; le Natur, F.; Cador, O.; Pointillart, F.; Calvez, G.; Daiguebonne, C.; Guillou, O.; Guizouarn, T.; le Guennic, B.; Bernot, K. Experimental and theoretical evidence that electrostatics governs easy-axis orientation in Dy(III)-based molecular chains. Chem. Commun. 2014, 50, 13346–13348. [Google Scholar] [CrossRef]
  42. Goura, J.; Guillaume, R.; Rivière, E.; Chandrasekhar, V. Hexanuclear, Heterometallic, Ni3Ln3 Complexes Possessing O-Capped Homo- and Heterometallic Structural Subunits: SMM Behavior of the Dysprosium Analogue. Inorg. Chem. 2014, 53, 7815–7823. [Google Scholar] [CrossRef]
  43. Program, M. Mercury Program: Cambridge Crystallographic Data Centre, 12 Union Road, Cambridge U.K. Available online: http://www.ccdc.cam.ac.uk/ (accessed on 8 February 2020).
  44. Görbitz, C.H. What is the best crystal size for collection of X-ray data? Refinement of the structure of glycyl-L-serine based on data from a very large crystal. Acta Cryst. B 1999, 55, 1090–1098. [Google Scholar] [CrossRef] [PubMed]
  45. Sheldrick, G.M. A short history of SHELX. Acta Crystallogr. A 2008, 64 Pt 1, 112–122. [Google Scholar] [CrossRef] [PubMed] [Green Version]
  46. Spek, A.L. PLATON SQUEEZE: A tool for the calculation of the disordered solvent contribution to the calculated structure factors. Acta Crystallogr. C Struct. Chem. 2015, 71 Pt 1, 9–18. [Google Scholar] [CrossRef] [PubMed] [Green Version]
Molecules 27 04052 sch001 550
Scheme 1. Molecular structure of the m-H4TTFTB ligand.
Scheme 1. Molecular structure of the m-H4TTFTB ligand.
Molecules 27 04052 sch001
Molecules 27 04052 g001 550
Figure 1. Structure of Tb-m-TTFTB ([Tb2(m-TTFTB)(m-H2TTFTB)0.5(HCOO)(DMF)]·2DMF·3H2O): the coordination environments of Tb1 (a) and Tb2 (b); a one-dimensional chain in Tb-m-TTFTB (c); (d) view of the three-dimensional framework along the [111] direction; (e) the two-dimensional structure assembled by the 1D chains and m-TTFTB4− ligands; (f) the weak interaction between adjacent TTF linkers. Some of the m-TTFTB4− ligands are simplified to benzoate group for clarity.
Figure 1. Structure of Tb-m-TTFTB ([Tb2(m-TTFTB)(m-H2TTFTB)0.5(HCOO)(DMF)]·2DMF·3H2O): the coordination environments of Tb1 (a) and Tb2 (b); a one-dimensional chain in Tb-m-TTFTB (c); (d) view of the three-dimensional framework along the [111] direction; (e) the two-dimensional structure assembled by the 1D chains and m-TTFTB4− ligands; (f) the weak interaction between adjacent TTF linkers. Some of the m-TTFTB4− ligands are simplified to benzoate group for clarity.
Molecules 27 04052 g001
Molecules 27 04052 g002 550
Figure 2. Experimental and calculated powder X-ray diffraction patterns for Tb-m-TTFTB, Er-m-TTFTB, and Gd-m-TTFTB. PXRD was simulated based on the single-crystal structure of Tb-m-TTFTB by applying preferred orientation along the b-direction.
Figure 2. Experimental and calculated powder X-ray diffraction patterns for Tb-m-TTFTB, Er-m-TTFTB, and Gd-m-TTFTB. PXRD was simulated based on the single-crystal structure of Tb-m-TTFTB by applying preferred orientation along the b-direction.
Molecules 27 04052 g002
Molecules 27 04052 g003 550
Figure 3. Solid-state cyclic voltammograms of Tb-m-TTFTB performed over five consecutive cycles (a) and at different scan rates (b). The experiments were conducted in 0.1 M LiBF4 in CH3CN electrolyte. The inset is the redox reaction of the TTF.
Figure 3. Solid-state cyclic voltammograms of Tb-m-TTFTB performed over five consecutive cycles (a) and at different scan rates (b). The experiments were conducted in 0.1 M LiBF4 in CH3CN electrolyte. The inset is the redox reaction of the TTF.
Molecules 27 04052 g003
Molecules 27 04052 g004 550
Figure 4. The diffuse reflectance spectra (a) and the translated Tauc plots (b) for Tb-m-TTFTB, Er-m-TTFTB, and Gd-m-TTFTB.
Figure 4. The diffuse reflectance spectra (a) and the translated Tauc plots (b) for Tb-m-TTFTB, Er-m-TTFTB, and Gd-m-TTFTB.
Molecules 27 04052 g004
Molecules 27 04052 g005 550
Figure 5. Temperature dependence of the χMT measured in a 1000 Oe field.
Figure 5. Temperature dependence of the χMT measured in a 1000 Oe field.
Molecules 27 04052 g005
Molecules 27 04052 g006 550
Figure 6. (a) Temperature-dependent in-phase χ′ and out-of-phase χ″ ac susceptibility signals for Dy-m-TTFTB at the indicated frequencies under zero dc field. (b) Temperature-dependent in-phase χ′ and out-of-phase χ″ ac susceptibility signals for Dy-m-TTFTB at the indicated frequencies under 1000 Oe.
Figure 6. (a) Temperature-dependent in-phase χ′ and out-of-phase χ″ ac susceptibility signals for Dy-m-TTFTB at the indicated frequencies under zero dc field. (b) Temperature-dependent in-phase χ′ and out-of-phase χ″ ac susceptibility signals for Dy-m-TTFTB at the indicated frequencies under 1000 Oe.
Molecules 27 04052 g006
Table
Table 1. Crystal and structure refinement parameters for Tb-m-TTFTB, Er-m-TTFTB, and Gd-m-TTFTB.
Table 1. Crystal and structure refinement parameters for Tb-m-TTFTB, Er-m-TTFTB, and Gd-m-TTFTB.
Tb-m-TTFTBEr-m-TTFTBGd-m-TTFTB
CCDC number1,914,3851,914,3871,914,384
Empirical formulaC61H53N3O20S6Tb2C61H53N3O20S6Er2C61H53N3O20S6Gd2
Formula weight1658.321674.991654.97
Temperature (K)153153153
Crystal systemTriclinicTriclinicTriclinic
Wavelength (Å)0.710730.710730.71073
Space groupP-1 (No. 2)P-1 (No. 2)P-1 (No. 2)
a (Å)15.5977 (10)15.371 (16)15.5779 (7)
b (Å)15.696 (1)15.15 (3)15.7382 (7)
c (Å)16.6914 (11)16.441 (18)16.7290 (7)
α (°)106.077 (1)105.29 (4)106.761 (1)
β (°)114.426 (1)115.493 (15)114.410 (1)
γ (°)104.542 (1)104.25 (3)104.065 (2)
Volume (Å3)3248.0 (4)3036 (8)3253.0 (3)
Z222
d (g·cm−3)1.4911.6131.485
F(000)143214441428
Absorption coefficient, μ/mm−12.4113.0132.272
Reflections total29,06928,22329,492
Reflections independent14,33213,86715,158
θ-range (°)1.5–27.31.5–27.92.1–27.7
Rint0.0290.0270.025
R1, (a) wR2 (I ≥ 2σ(I)) (b)0.0327/0.10310.0445/0.13750.0295/0.0775
R1, wR2 (all data)0.0424/0.13140.0546/0.16140.0353/0.0805
GOF1.051.101.06
Largest diff. peak and hole (eÅ−3)1.66/−1.122.70/−1.712.49/−1.15
(a) R1 = Σ||Fo| − |Fc||/Σ|Fo|; (b) wR2 = [Σw(Fo2 – Fc2)2/Σw(Fo2)2]1/2.
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Share and Cite

MDPI and ACS Style

Huang, H.; Yang, Z.-M.; Zhou, X.-C.; Zhang, G.; Su, J. Redox-Active Metal-Organic Frameworks with Three-Dimensional Lattice Containing the m-Tetrathiafulvalene-Tetrabenzoate. Molecules 2022, 27, 4052. https://doi.org/10.3390/molecules27134052

AMA Style

Huang H, Yang Z-M, Zhou X-C, Zhang G, Su J. Redox-Active Metal-Organic Frameworks with Three-Dimensional Lattice Containing the m-Tetrathiafulvalene-Tetrabenzoate. Molecules. 2022; 27(13):4052. https://doi.org/10.3390/molecules27134052

Chicago/Turabian Style

Huang, Hongrui, Zhi-Mei Yang, Xiao-Cheng Zhou, Gen Zhang, and Jian Su. 2022. "Redox-Active Metal-Organic Frameworks with Three-Dimensional Lattice Containing the m-Tetrathiafulvalene-Tetrabenzoate" Molecules 27, no. 13: 4052. https://doi.org/10.3390/molecules27134052

APA Style

Huang, H., Yang, Z. -M., Zhou, X. -C., Zhang, G., & Su, J. (2022). Redox-Active Metal-Organic Frameworks with Three-Dimensional Lattice Containing the m-Tetrathiafulvalene-Tetrabenzoate. Molecules, 27(13), 4052. https://doi.org/10.3390/molecules27134052

Article Metrics

Back to TopTop