Maximum Power Point Tracking Sensorless Control of an Axial-Flux Permanent Magnet Vernier Wind Power Generator
Abstract
:1. Introduction
2. AFPM-VM Design
2.1. Machine Structure
2.2. Working Principle
2.3. Analysis Method
2.4. Advantages of the Proposed AFPM-VM
- (1)
- By introducing Vernier structure into the AFPM machine, the magnetic gear effect works in the designed generator. The electrical frequency is multiples of the shaft rotating frequency, which is very suitable for low-speed direct-drive wind power generation.
- (2)
- The proposed generator can produce high-frequency stator windings currents when shaft speed is low, and thus high speed sensorless control strategy can be easily implemented.
- (3)
- The Vernier machine design inherently provides a convenient design method to accommodate a large number of PM poles in rotor, and small number of slots and winding pole pairs in stator. This design enables a high filling factor of the inner stator space to accommodate the armature windings and very suitable for low-speed high-torque direct-drive operation;
- (4)
- The dual rotors allow for direct coupling with the wind turbine, thus alleviating the bearing requirements and improving the mechanical integrity;
- (5)
- Dual PMs can interaction with both sides of the stator windings, which can improve the torque density. Concentrated winding connection in the stator results in a compact structure to improve the torque density accordingly;
- (6)
- The concentrated winding connection reduces the end-windings, and makes the assembling convenient.
3. Sensorless MPPT Control of AFPM-VM
3.1. Hardware Setup and Working Principle for Low Rotor Speed
3.2. Position and Velocity Estimation
3.3. Sensorless MPPT Control of AFPM-VM
4. Experiment Results
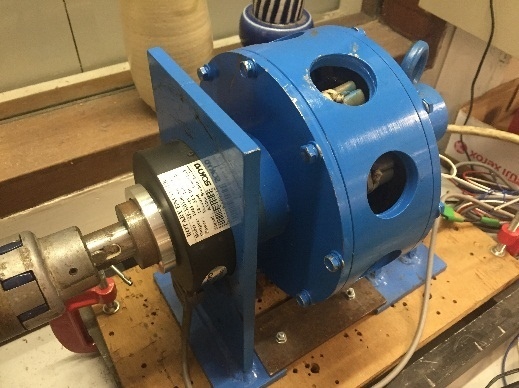
4.1. Proposed Machine and Experimental Setup
4.2. Machine Design and Position Estimation Verification
4.3. Sensorless MPPT Control
5. Conclusions
Acknowledgments
Author Contributions
Conflicts of Interest
References
- Niu, S.; Ho, S.L.; Fu, W.N. A Novel Direct-Drive Dual-Structure Permanent Magnet Machine. IEEE Trans. Magn. 2010, 46, 2036–2039. [Google Scholar] [CrossRef]
- Atallah, K.; Howe, D. A novel high-performance magnetic gear. IEEE Trans. Magn. 2001, 37, 2844–2846. [Google Scholar] [CrossRef]
- Niu, S.; Chen, N.; Ho, S.L.; Fu, W.N. Design Optimization of Magnetic Gears Using Mesh Adjustable Finite-Element Algorithm for Improved Torque. IEEE Trans. Magn. 2012, 48, 4156–4159. [Google Scholar] [CrossRef]
- Ho, S.L.; Niu, S.; Fu, W.N. Design and Comparison of Vernier Permanent Magnet Machines. IEEE Trans. Magn. 2011, 47, 3280–3283. [Google Scholar] [CrossRef]
- Li, J.; Chau, K.T.; Jiang, J.Z.; Liu, C.; Li, W. A New Efficient Permanent-Magnet Vernier Machine for Wind Power Generation. IEEE Trans. Magn. 2010, 46, 1475–1478. [Google Scholar] [CrossRef] [Green Version]
- Zhao, Y.; Zhang, Z.; Qiao, W.; Wu, L. An Extended Flux Model-Based Rotor Position Estimator for Sensorless Control of Salient-Pole Permanent-Magnet Synchronous Machines. IEEE Trans. Power Electr. 2015, 30, 4412–4422. [Google Scholar] [CrossRef]
- Kommuri, S.K.; Veluvolu, K.C.; Defoort, M.; Soh, Y.C. Higher-Order Sliding Mode Observer for Speed and Position Estimation in PMSM. Math. Probl. Eng. 2014, 2014, 1–12. [Google Scholar] [CrossRef]
- Khlaief, A.; Boussak, M.; Châari, A. A MRAS-based stator resistance and speed estimation for sensorless vector controlled IPMSM drive. Electr. Power Syst. Res. 2014, 108, 1–15. [Google Scholar] [CrossRef]
- Yuan, Q.; Yang, Z.; Lin, F.; Sun, H. Sensorless Control of Permanent Magnet Synchronous Motor with Stator Flux Estimation. J. Comput. 2013, 8, 108–112. [Google Scholar] [CrossRef]
- Jouili, M.; Jarray, K.; Koubaa, Y.; Boussak, M. Luenberger state observer for speed sensorless ISFOC induction motor drives. Electr. Power Syst. Res. 2012, 89, 139–147. [Google Scholar] [CrossRef]
- Bernardes, T.; Montagner, V.F.; Grundling, H.A.; Pinheiro, H. Discrete-Time Sliding Mode Observer for Sensorless Vector Control of Permanent Magnet Synchronous Machine. IEEE Trans. Ind. Electron. 2014, 61, 1679–1691. [Google Scholar] [CrossRef]
- Feng, Y.; Yu, X.; Han, F. High-Order Terminal Sliding-Mode Observer for Parameter Estimation of a Permanent-Magnet Synchronous Motor. IEEE Trans. Ind. Electron. 2013, 60, 4272–4280. [Google Scholar] [CrossRef]
- Qiao, Z.; Shi, T.; Wang, Y.; Yan, Y.; Xia, C.; He, X. New Sliding-Mode Observer for Position Sensorless Control of Permanent-Magnet Synchronous Motor. IEEE Trans. Ind. Electron. 2013, 60, 710–719. [Google Scholar] [CrossRef]




















| Parameter | Value |
|---|---|
| Input voltage (V) | 155 |
| Rated torque (Nm) | 4 |
| Rated speed (rpm) | 400 |
| Outer diameter of rotor (mm) | 60 |
| Inner diameter of rotor (mm) | 20 |
| Outer diameter of stator (mm) | 60 |
| Inner diameter of stator (mm) | 35 |
| Stator thickness (mm) | 50 |
| Axial length of rotor PMs (mm) | 25 |
| PM thickness (mm) | 3 |
| Air-gap (mm) | 1 |
| Turn number per phase | 45 |
| Rotor pole-pair number | 23 |
| Stator pole-pair number | 4 |
| Stator fake tooth number | 27 |
© 2016 by the authors; licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC-BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Luo, X.; Niu, S. Maximum Power Point Tracking Sensorless Control of an Axial-Flux Permanent Magnet Vernier Wind Power Generator. Energies 2016, 9, 581. https://doi.org/10.3390/en9080581
Luo X, Niu S. Maximum Power Point Tracking Sensorless Control of an Axial-Flux Permanent Magnet Vernier Wind Power Generator. Energies. 2016; 9(8):581. https://doi.org/10.3390/en9080581
Chicago/Turabian StyleLuo, Xiang, and Shuangxia Niu. 2016. "Maximum Power Point Tracking Sensorless Control of an Axial-Flux Permanent Magnet Vernier Wind Power Generator" Energies 9, no. 8: 581. https://doi.org/10.3390/en9080581
APA StyleLuo, X., & Niu, S. (2016). Maximum Power Point Tracking Sensorless Control of an Axial-Flux Permanent Magnet Vernier Wind Power Generator. Energies, 9(8), 581. https://doi.org/10.3390/en9080581