Sorption Properties of Specific Polymeric Microspheres towards Desethyl-Terbuthylazine and 2-Hydroxy-Terbuthylazine: Batch and Column Studies
Abstract
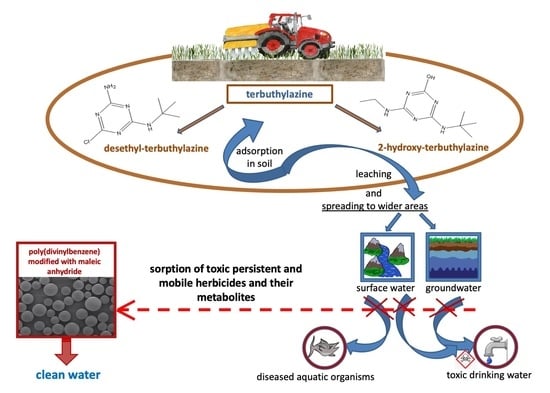
:1. Introduction
2. Materials and Methods
2.1. Materials
2.2. Sorption Studies
2.2.1. Batch Studies
2.2.2. Fixed Bed Sorption Studies
3. Results and Discussion
3.1. Adsorbent Characterization
3.2. Batch Sorption Experiments
3.2.1. Adsorption Kinetics
3.2.2. Adsorption Isotherms
3.2.3. Sorption Models
3.3. Fixed-Bed Column Studies
3.3.1. Sorption Studies
3.3.2. Sorption Models
3.3.3. Desorption Studies
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Hvězdová, M.; Kosubová, P.; Košíková, M.; Scherr, K.E.; Šimek, Z.; Brodský, L.; Šudoma, M.; Škulcová, L.; Sáňka, M.; Svobodová, M.; et al. Currently and recently used pesticides in Central European arable soils. Sci. Total Environ. 2018, 613–614, 361–370. [Google Scholar] [CrossRef]
- Khan, M.A.; Brown, C.D. Influence of commercial formulation on the sorption and leaching behaviour of propyzamide in soil. Sci. Total Environ. 2017, 578, 158–166. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Silva, T.S.; Souza, M.D.F.; Teófilo, T.M.D.S.; Dos Santos, M.S.; Porto, M.A.F.; Souza, C.M.M.; Dos Santos, J.B.; Silva, D.V. Use of neural networks to estimate the sorption and desorption coefficients of herbicides: A case study of diuron, hexazinone, and sulfometuron-methyl in Brazil. Chemosphere 2019, 236, 124333. [Google Scholar] [CrossRef] [PubMed]
- Arp, H.P.H.; Brown, T.N.; Berger, U.; Hale, S.E. Ranking REACH registered neutral, ionizable and ionic organic chemicals based on their aquatic persistency and mobility. Environ. Sci. Process. Impacts 2017, 19, 939–955. [Google Scholar] [CrossRef] [PubMed]
- Reemtsma, T.; Berger, U.; Arp, H.P.H.; Gallard, H.; Knepper, T.P.; Neumann, M.; Quintana, J.B.; De Voogt, P. Mind the Gap: Persistent and Mobile Organic Compounds—Water Contaminants That Slip Through. Environ. Sci. Technol. 2016, 50, 10308–10315. [Google Scholar] [CrossRef] [Green Version]
- Rüdel, H.; Körner, W.; Letzel, T.; Neumann, M.; Nödler, K.; Reemtsma, T. Persistent, mobile and toxic substances in the environment: A spotlight on current research and regulatory activities. Environ. Sci. Eur. 2020, 32, 1–11. [Google Scholar] [CrossRef]
- Loos, R.; Locoro, G.; Comero, S.; Contini, S.; Schwesig, D.; Werres, F.; Balsaa, P.; Gans, O.; Weiss, S.; Blaha, L.; et al. Pan-European survey on the occurrence of selected polar organic persistent pollutants in ground water. Water Res. 2010, 44, 4115–4126. [Google Scholar] [CrossRef] [PubMed]
- European Commission. Directive 2006/118/EC of the European Parliament and the Council of 12th of December 2006 on the protection of groundwater against pollution and deterioration. Off. J. Eur. Union 2006, L 372/19, 19–31. [Google Scholar]
- Mladinic, M.; Želježić, D.; Shaposhnikov, S.A.; Collins, A.R. The use of FISH-comet to detect c-Myc and TP 53 damage in extended-term lymphocyte cultures treated with terbuthylazine and carbofuran. Toxicol. Lett. 2012, 211, 62–69. [Google Scholar] [CrossRef]
- Lovaković, B.T.; Pizent, A.; Kašuba, V.; Kopjar, N.; Micek, V.; Mendaš, G.; Dvoršćak, M.; Mikolić, A.; Milić, M.; Žunec, S.; et al. Effects of sub-chronic exposure to terbuthylazine on DNA damage, oxidative stress and parent compound/metabolite levels in adult male rats. Food Chem. Toxicol. 2017, 108, 93–103. [Google Scholar] [CrossRef]
- Dos Santos, E.V.; Sáez, C.; Martínez-Huitle, C.A.; Cañizares, P.; Rodrigo, M.A. Combined soil washing and CDEO for the removal of atrazine from soils. J. Hazard. Mater. 2015, 300, 129–134. [Google Scholar] [CrossRef]
- Velisek, J.; Stara, A.; Zuskova, E.; Kouba, A. Effects of three triazine metabolites and their mixture at environmentally relevant concentrations on early life stages of marbled crayfish (Procambarus fallax f. virginalis). Chemosphere 2017, 175, 440–445. [Google Scholar] [CrossRef]
- Tasca, A.L.; Puccini, M.; Fletcher, A. Terbuthylazine and desethylterbuthylazine: Recent occurrence, mobility and removal techniques. Chemosphere 2018, 202, 94–104. [Google Scholar] [CrossRef] [Green Version]
- Gardo Gold Plant Protection Product Characterization. Available online: https://ctgb.blob.core.windows.net/documents/84656e937a8f90a5345e1eac8c26018c_20060801_13145_02.html (accessed on 4 March 2021).
- Čelić, M.; Jaén-Gil, A.; Briceño-Guevara, S.; Rodríguez-Mozaz, S.; Gros, M.; Petrović, M. Extended suspect screening to identify contaminants of emerging concern in riverine and coastal ecosystems and assessment of environmental risks. J. Hazard. Mater. 2021, 404, 124102. [Google Scholar] [CrossRef]
- Robles-Molina, J.; Gilbert-López, B.; García-Reyes, J.F.; Molina-Díaz, A. Monitoring of selected priority and emerging contaminants in the Guadalquivir River and other related surface waters in the province of Jaén, South East Spain. Sci. Total Environ. 2014, 479–480, 247–257. [Google Scholar] [CrossRef]
- Koutnik, D.; Stara, A.; Zusková, E.; Kouba, A.; Velisek, J. The chronic effects of terbuthylazine-2-hydroxy on early life stages of marbled crayfish (Procambarus fallax f. virginalis). Pestic. Biochem. Physiol. 2017, 136, 29–33. [Google Scholar] [CrossRef]
- Velisek, J.; Stara, A.; Koutnik, D.; Machova, J. Effect of Terbuthylazine-2-hydroxy at Environmental Concentrations on Early Life Stages of Common Carp (Cyprinus carpio L.). BioMed Res. Int. 2014, 2014, 621304. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Velisek, J.; Koutnik, D.; Zuskova, E.; Stara, A. Effects of the terbuthylazine metabolite terbuthylazine-desethyl on common carp embryos and larvae. Sci. Total Environ. 2016, 539, 214–220. [Google Scholar] [CrossRef]
- European Food Safety Authority. Conclusion on the peer review of the pesticide risk assessment of the active substance terbuthylazine. EFSA J. 2011, 9. [Google Scholar] [CrossRef] [Green Version]
- Kiefer, K.; Müller, A.; Singer, H.; Hollender, J. New relevant pesticide transformation products in groundwater detected using target and suspect screening for agricultural and urban micropollutants with LC-HRMS. Water Res. 2019, 165, 114972. [Google Scholar] [CrossRef] [Green Version]
- Abdourahime, H.; Anastassiadou, M.; Arena, M.; Auteri, D.; Barmaz, S.; Brancato, A.; Bura, L.; Cabrera, L.C.; Chaideftou, E.; Chiusolo, A.; et al. Updated peer review of the pesticide risk assessment for the active substance terbuthylazine in light of confirmatory data submitted. EFSA J. 2019, 17, e05817. [Google Scholar] [CrossRef]
- European Commission. Directive (EU) 2020/2184 of the European Parliament and of the Council of 16 December 2020 on the quality of water intended for human consumption (recast). Off. J. Eur. Union 2020, L 435/1, 1–62. [Google Scholar]
- Guzzella, L.; Pozzoni, F.; Giuliano, G. Herbicide contamination of surficial groundwater in Northern Italy. Environ. Pollut. 2006, 142, 344–353. [Google Scholar] [CrossRef] [PubMed]
- Hermosin, M.; Calderon, M.J.; Real, M.; Cornejo, J. Impact of herbicides used in olive groves on waters of the Guadalquivir river basin (southern Spain). Agric. Ecosyst. Environ. 2013, 164, 229–243. [Google Scholar] [CrossRef]
- Karlsson, A.S.; Lesch, M.; Weihermüller, L.; Thiele, B.; Disko, U.; Hofmann, D.; Vereecken, H.; Spielvogel, S. Pesticide contamination of the upper Elbe River and an adjacent floodplain area. J. Soils Sediments 2020, 20, 2067–2081. [Google Scholar] [CrossRef] [Green Version]
- Masiá, A.; Campo, J.; Navarro-Ortega, A.; Barceló, D.; Picó, Y. Pesticide monitoring in the basin of Llobregat River (Catalonia, Spain) and comparison with historical data. Sci. Total Environ. 2015, 503–504, 58–68. [Google Scholar] [CrossRef]
- Cotton, J.; Leroux, F.; Broudin, S.; Poirel, M.; Corman, B.; Junot, C.; Ducruix, C. Development and validation of a multiresidue method for the analysis of more than 500 pesticides and drugs in water based on on-line and liquid chromatography coupled to high resolution mass spectrometry. Water Res. 2016, 104, 20–27. [Google Scholar] [CrossRef]
- Fingler, S.; Mendaš, G.; Dvoršćak, M.; Stipičević, S.; Vasilić, Ž.; Drevenkar, V. Herbicide micropollutants in surface, ground and drinking waters within and near the area of Zagreb, Croatia. Environ. Sci. Pollut. Res. 2016, 24, 11017–11030. [Google Scholar] [CrossRef]
- Tasca, A.L.; Fletcher, A. State of the art of the environmental behaviour and removal techniques of the endocrine disruptor 3,4-dichloroaniline. J. Environ. Sci. Health Part A 2018, 53, 260–270. [Google Scholar] [CrossRef]
- Mesquita, D.P.; Quintelas, C.; Amaral, A.L.P.D.; Ferreira, E.C. Monitoring biological wastewater treatment processes: Recent advances in spectroscopy applications. Rev. Environ. Sci. Biotechnol. 2017, 16, 395–424. [Google Scholar] [CrossRef] [Green Version]
- Parlapiano, M.; Akyol, Ç.; Foglia, A.; Pisani, M.; Astolfi, P.; Eusebi, A.L.; Fatone, F. Selective removal of contaminants of emerging concern (CECs) from urban water cycle via Molecularly Imprinted Polymers (MIPs): Potential of upscaling and enabling reclaimed water reuse. J. Environ. Chem. Eng. 2021, 9, 105051. [Google Scholar] [CrossRef]
- Bagheri, A.R.; Aramesh, N.; Khan, A.A.; Gul, I.; Ghotekar, S.; Bilal, M. Molecularly imprinted polymers-based adsorption and photocatalytic approaches for mitigation of environmentally-hazardous pollutants—A review. J. Environ. Chem. Eng. 2021, 9, 104879. [Google Scholar] [CrossRef]
- El-Said, W.A.; El-Khouly, M.E.; Ali, M.H.; Rashad, R.T.; Elshehy, E.A.; Al-Bogami, A.S. Synthesis of mesoporous silica-polymer composite for the chloridazon pesticide removal from aqueous media. J. Environ. Chem. Eng. 2018, 6, 2214–2221. [Google Scholar] [CrossRef]
- Ronka, S. Removal of triazine-based herbicides on specific polymeric sorbent: Batch studies. Pure Appl. Chem. 2016, 88, 1167–1177. [Google Scholar] [CrossRef]
- Ronka, S. Removal of triazine-based herbicides on specific polymeric sorbent: Fixed bed column studies. Pure Appl. Chem. 2016, 88, 1179–1189. [Google Scholar] [CrossRef]
- Ronka, S.; Kujawska, M.; Juśkiewicz, H. Triazines removal by selective polymeric adsorbent. Pure Appl. Chem. 2014, 86, 1755–1769. [Google Scholar] [CrossRef]
- Ronka, S.; Kucharski, M. Application of novel polymeric, highly specific adsorbent for the removal of terbuthylazine from complex environmental samples. Int. J. Environ. Anal. Chem. 2020, 1–14. [Google Scholar] [CrossRef]
- Stranix, B.R.; Darling, G.D. Cycloaddition Functional Polymers from (Vinyl)polystyrene. U.S. Patent 6,534,611 B1, 18 March 2003. [Google Scholar]
- Kica, M.; Ronka, S. The Removal of Atrazine from Water using Specific Polymeric Adsorbent. Sep. Sci. Technol. 2014, 49, 1634–1642. [Google Scholar] [CrossRef]
- Schmitt, P.; Garrison, A.; Freitag, D.; Kettrup, A. Separation of s-triazine herbicides and their metabolites by capillary zone electrophoresis as a function of pH. J. Chromatogr. A 1996, 723, 169–177. [Google Scholar] [CrossRef]
- PPDB. Available online: https://sitem.herts.ac.uk/aeru/ppdb/en/Reports/1494.htm (accessed on 4 March 2021).
- EPA (United States Environmental Protection Agency). Available online: https://comptox.epa.gov/dashboard/dsstoxdb/results?search=DTXSID80184211#details (accessed on 4 March 2021).
- PPDB. Available online: https://sitem.herts.ac.uk/aeru/ppdb/en/Reports/1495.htm (accessed on 4 March 2021).
- LGC. Safety Data Sheet According to 1907/2006/EC. Article 31. Available online: https://assets.lgcstandards.com/sys-master%2Fpdfs%2Fh84%2Fh35%2F10141506240542%2FSDS_DRE-C17305000_ST-WB-MSDS-2922617-1-1-1.PDF (accessed on 4 March 2021).
- Kaleta, J. Removal of methylene blue from aqueous solution by adsorption. Arch. Environ. Prot. 2007, 33, 45–54. [Google Scholar]
- Stranix, B.R.; Darling, G.D. Functional Polymers from Vinylpolystyrene. Diels-Alder Reactions with Olefins. J. Org. Chem. 1997, 62, 9001–9004. [Google Scholar] [CrossRef]
- Simonin, J.-P. On the comparison of pseudo-first order and pseudo-second order rate laws in the modeling of adsorption kinetics. Chem. Eng. J. 2016, 300, 254–263. [Google Scholar] [CrossRef] [Green Version]
- Liu, S. Cooperative adsorption on solid surfaces. J. Colloid Interface Sci. 2015, 450, 224–238. [Google Scholar] [CrossRef] [PubMed]
- Kamga, F.T. Modeling adsorption mechanism of paraquat onto Ayous (Triplochiton scleroxylon) wood sawdust. Appl. Water Sci. 2018, 9, 1. [Google Scholar] [CrossRef] [Green Version]
- Itodo, A.U.; Itodo, H.U. Sorption energies estimation using Dubinin-Radushkevich and temkin adsorption isotherms. Life Sci. J. 2010, 7, 68–76. [Google Scholar]
- Chabani, M.; Amrane, A.; Bensmaili, A. Kinetic modelling of the adsorption of nitrates by ion exchange resin. Chem. Eng. J. 2006, 125, 111–117. [Google Scholar] [CrossRef]
- Software Origin Pro 2021, Version 9.8.0.200; OriginLab Corporation: Northampton, MA, USA, 2021.
- Singh, S.K.; Mehta, D.; Sehgal, D. Fixed Bed Column Study and Adsorption Modelling on the Adsorption of Malachite Green dye from wastewater using Acid Activated Sawdust. Int. J. Adv. Res. 2015, 3, 521–529. [Google Scholar]
- Majumdar, S.; Baishya, A.; Mahanta, D. Kinetic and Equilibrium Modeling of Anionic Dye Adsorption on Polyaniline Emeraldine Salt: Batch and Fixed Bed Column Studies. Fibers Polym. 2019, 20, 1226–1235. [Google Scholar] [CrossRef]
- Bharathi, K.S.; Ramesh, S.P.T. Fixed-bed column studies on biosorption of crystal violet from aqueous solution by Citrullus lanatus rind and Cyperus rotundus. Appl. Water Sci. 2013, 3, 673–687. [Google Scholar] [CrossRef] [Green Version]
- Homem, N.; Vieira, A.M.S.; Bergamasco, R.; Vieira, M.F. Low-cost biosorbent based on Moringa oleifera residues for herbicide atrazine removal in a fixed-bed column. Can. J. Chem. Eng. 2018, 96, 1468–1478. [Google Scholar] [CrossRef]
- Sharma, R.; Singh, B. Removal of Ni (II) ions from aqueous solutions using modified rice straw in a fixed bed column. Bioresour. Technol. 2013, 146, 519–524. [Google Scholar] [CrossRef] [PubMed]
- Djelloul, C.; Hamdaoui, O. Dynamic adsorption of methylene blue by melon peel in fixed-bed columns. Desalin. Water Treat. 2014, 56, 1–10. [Google Scholar] [CrossRef]
- Huang, M.-R.; Lu, H.-J.; Song, W.-D.; Li, X.-G. Dynamic Reversible Adsorption and Desorption of Lead Ions Through a Packed Column of Poly(m-phenylenediamine) Spheroids. Soft Mater. 2010, 8, 149–163. [Google Scholar] [CrossRef]
- Chittoo, B.S.; Sutherland, C. Column breakthrough studies for the removal and recovery of phosphate by lime-iron sludge: Modeling and optimization using artificial neural network and adaptive neuro-fuzzy inference system. Chin. J. Chem. Eng. 2020, 28, 1847–1859. [Google Scholar] [CrossRef]











| Herbicide | Structure | Molecular Weight | Solubility in Water 20 °C (mg L−1) | pKa * [41] | logKow * |
|---|---|---|---|---|---|
| desethyl-terbuthylazine |  | 201.66 | 327.1 [42] | ~2 | 1.94 [43] |
| 2-hydroxy-terbuthylazine |  | 211.26 | 7.19 [44] | ~5 | 2.54 [45] |
| Terbuthylazine Metabolite | Pseudo-First Kinetic Model | Pseudo-Second Kinetic Model | ||||
|---|---|---|---|---|---|---|
| R2 | qeq (mg g−1) | k1 (min−1) | R2 | qeq (mg g−1) | k2 (g mg−1 min−1) | |
| desethyl-terbuthylazine | 0.965 | 13.9 | 0.975 | 16.4 | ||
| 2-hydroxy-terbuthylazine | 0.910 | 42.4 | 0.991 | 115 | ||
| Terbuthylazine Metabolite | qeq (mg g−1) | R (%) | K (-) |
|---|---|---|---|
| desethyl-terbuthylazine | 21 | 80 | 2800 |
| 2-hydroxy-terbuthylazine | 113 | 88 | 24,400 |
| Isotherm Model | Parameter | Metabolite | |
|---|---|---|---|
| desethyl-terbuthylazine | 2-hydroxy-terbuthylazine | ||
| Langmuir | qm (mg g−1) | 263 | 476 |
| Kα (dm3 mg−1) | 0.01 | 0.05 | |
| R2 | 0.969 | 0.916 | |
| SSE * | |||
| Freundlich | 1/n | 1.37 | 1.01 |
| KF ((mg g−1)(L mg−1)1/n) | 1.98 | 20.81 | |
| R2 | 0.949 | 0.913 | |
| SSE * | 0.058 | 0.026 | |
| Temkin | AT (L g−1) | 0.61 | 1.05 |
| bT (J mol−1) | 201 | 40 | |
| R2 | 0.989 | 0.834 | |
| SSE * | 4.03 | 1051.26 | |
| Dubinin-Radushkevich | qs (mg g−1) | 21.6 | 94.9 |
| Kad (mol2 J−2) | |||
| E (kJ mol−1) | 0.5 | 0.9 | |
| R2 | 0.970 | 0.731 | |
| SSE * | 0.12 | 0.23 | |
| Terbuthylazine Metabolite | Mathematical Equation of the Breakthrough Curve | R2 |
|---|---|---|
| desethyl-terbuthylazine | y = 203.32x5 − 790.86x4 + 774.9x3 − 345.84x2 + 82.888x + 0.1131 | 0.996 |
| 2-hydroxy-terbuthylazine | y = 0.0056x5 − 0.1269x4 + 1.0373x3 − 4.074x2 + 8.6167x − 1.18137 | 0.987 |
| Terbuthylazine Metabolite | Dynamic Adsorption Capacity (mg g−1) | Bed OperationTime until Breakthrough tb (h) | Bed Operation Time until Exhausted te (h) | Height of Adsorption Front H0 (cm) | Mass Exchange Moving Rate u (cm min−1) | |
|---|---|---|---|---|---|---|
| Pt (Total) | Pu (Useful) | |||||
| desethyl-terbuthylazine | 10 | 1 | 0.25 | 5.75 | 3.13 | |
| 2-hydroxy-terbuthylazine | 113 | 39 | 8.50 | 75.75 | 2.80 | |
| Model | Parameter | Metabolite | |
|---|---|---|---|
| desethyl-terbuthylazine | 2-hydroxy-terbuthylazine | ||
| Adams–Bohart | (L mg−1 min−1) | ||
| (mg L−1) | 4254.5 | 45,294.4 | |
| R2 | 0.635 | 0.385 | |
| SSE * | 2.63 | 146.21 | |
| Thomas | (mL mg−1 min−1) | ||
| (mg g−1) | |||
| R2 | 0.915 | 0.606 | |
| SSE * | 4.39 | 185.40 | |
| Yoon–Nelson | (min−1) | ||
| (min) | 103.6 | 2489.8 | |
| R2 | 0.915 | 0.606 | |
| SSE * | 4.93 | 185.40 | |
| Wolborska | Β (1 h−1) | 0.0184 | 0.0332 |
| (mg L−1) | 1.18 | 15.24 | |
| R2 | 0.635 | 0.385 | |
| SSE * | 2.63 | 146.45 | |
| Terbuthylazine Metabolite | Desorption Efficiency (%) | Amount of Eluent (mL) | Desorption Time (min) |
|---|---|---|---|
| desethyl-terbuthylazine | 95 | 166 | 185 |
| 2-hydroxy-terbuthylazine | 84 | 284 | 315 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ronka, S.; Bodylska, W. Sorption Properties of Specific Polymeric Microspheres towards Desethyl-Terbuthylazine and 2-Hydroxy-Terbuthylazine: Batch and Column Studies. Materials 2021, 14, 2734. https://doi.org/10.3390/ma14112734
Ronka S, Bodylska W. Sorption Properties of Specific Polymeric Microspheres towards Desethyl-Terbuthylazine and 2-Hydroxy-Terbuthylazine: Batch and Column Studies. Materials. 2021; 14(11):2734. https://doi.org/10.3390/ma14112734
Chicago/Turabian StyleRonka, Sylwia, and Weronika Bodylska. 2021. "Sorption Properties of Specific Polymeric Microspheres towards Desethyl-Terbuthylazine and 2-Hydroxy-Terbuthylazine: Batch and Column Studies" Materials 14, no. 11: 2734. https://doi.org/10.3390/ma14112734
APA StyleRonka, S., & Bodylska, W. (2021). Sorption Properties of Specific Polymeric Microspheres towards Desethyl-Terbuthylazine and 2-Hydroxy-Terbuthylazine: Batch and Column Studies. Materials, 14(11), 2734. https://doi.org/10.3390/ma14112734