On the Use of Cross-Correlation between Volume Scattering and Helix Scattering from Polarimetric SAR Data for the Improvement of Ship Detection
Abstract
:1. Introduction
2. Azimuth Ambiguities Description
3. Experimental Data Description


| Key Parameters | Values |
|---|---|
| Acquisition time | 4-OCT-2000 |
| Wavelength (m) | 0.0567 (C-band)/0.24226 (L-band) |
| Polarization | HH/HV/VH/HH |
| Azimuth spacing Δρa (m) | 4.63 |
| Slant range spacing Δρr (m) | 3.331 |
| Looks number in azimuth | 9 |
| Looks number in range | 1 |
4. Polarimetric Scattering Characteristics of Ships and Azimuth Ambiguities
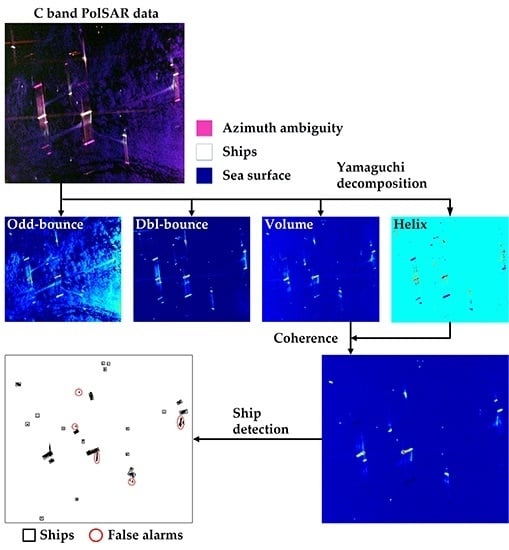
4.1. Yamaguchi Decomposition
4.2. Different Polarimetric Scattering Characteristics between Ships and Azimuth Ambiguities




5. Ship Detection Method

6. Exprimental Results and Discussion
6.1. Algorithm Verification


6.2. Discussion
6.2.1. The Comparison of Detection Performance


6.2.2. The Enhancement of TCR by Cross-Correlation between Volume and Helix Scattering

| Ship ID | Ship | Surrounding Sea | Target-to-Clutter Ratio | ||||||
|---|---|---|---|---|---|---|---|---|---|
| Vol | Hlx | Coh | Vol | Hlx | Coh | Vol | Hlx | Coh | |
| S6 | −14.927 | −17.351 | −28.868 | −24.866 | −28.198 | −45.731 | 9.939 | 10.847 | 16.863 |
| S7 | −6.963 | −17.587 | −21.542 | −23.430 | −28.261 | −44.755 | 16.467 | 10.674 | 23.213 |
| S11 | −12.987 | −17.834 | −27.876 | −25.516 | −28.155 | −46.011 | 12.529 | 10.321 | 18.135 |
| S12 | −17.526 | −16.567 | −30.626 | −25.847 | −28.353 | −46.367 | 8.321 | 11.786 | 15.741 |
| S17 | −10.810 | −15.460 | −23.688 | −25.691 | −28.239 | −46.232 | 14.881 | 12.779 | 22.544 |
| S19 | −7.813 | −16.846 | −21.735 | −25.624 | −28.188 | −46.025 | 17.811 | 11.342 | 24.290 |
| S21 | −7.465 | −10.916 | −15.956 | −25.231 | −28.187 | −45.895 | 17.766 | 17.271 | 29.939 |
| S22 | −20.065 | −14.634 | −29.222 | −24.219 | −28.229 | −45.367 | 4.154 | 13.595 | 16.145 |
7. Conclusions
Acknowledgments
Author Contributions
Conflicts of Interest
References
- Curlander, J.C.; Mcdonough, R.N. Synthetic Aperture Radar: Systems and Signal Processing; Wiley-Interscience: New York, NY, USA, 1991. [Google Scholar]
- Wang, C.; Wang, Y.; Liao, M. Removal of azimuth ambiguities and detection of a ship: Using polarimetric airborne C-band SAR images. Int. J. Remote Sens. 2012, 33, 3197–3210. [Google Scholar] [CrossRef]
- Wei, J.J.; Li, P.X.; Yang, J.; Zhang, J.; Lang, F. A new automatic ship detection method using L-band polarimetric SAR imagery. IEEE J. Sel. Top. Appl. Earth Observ. 2014, 7, 1383–1393. [Google Scholar]
- Crisp, D.J. The State-of the Art in Ship Detection in Synthetic Aperture Radar Imagery; DSTO Information Sciences Laboratory: Edinburgh, Australia, 2004. [Google Scholar]
- Moreira, A. Suppressing the azimuth ambiguities in synthetic aperture radar images. IEEE Trans. Geosci. Remote Sens. 1993, 31, 885–895. [Google Scholar] [CrossRef]
- Monti Guarnieri, A. Adaptive removal of azimuth ambiguities in SAR images. IEEE Trans. Geosci. Remote Sens. 2005, 43, 625–633. [Google Scholar] [CrossRef]
- Di Martino, G.; Iodice, A.; Riccio, D.; Ruello, G. Filtering of azimuth ambiguity in stripmap synthetic aperture radar images. IEEE J. Sel. Top. Appl. Earth Observ. 2014, 7, 3967–3978. [Google Scholar] [CrossRef]
- Liu, C. Effects of target motion on polarimetric SAR images. Can. J. Remote Sens. 2006, 32, 51–64. [Google Scholar] [CrossRef]
- Liu, C. Time-frequency analysis of PolSAR moving target data. Can. J. Remote Sens. 2007, 33, 237–249. [Google Scholar] [CrossRef]
- Liu, C.; Gierull, C.H. A new application for PolSAR imagery in the field of moving target indication/ship detection. IEEE Trans. Geosci. Remote Sens. 2007, 45, 3426–3436. [Google Scholar] [CrossRef]
- Velotto, D.; Soccorsi, M.; Lehner, S. Azimuth ambiguities removal for ship detection using full polarimetric X-band SAR data. In Proceedings of the 2012 International Geoscience Remote Sensing Symposium (IGARSS), Munich, Germany, 22–27 July 2012; pp. 7621–7624.
- Velotto, D.; Soccorsi, M.; Lehner, S. Azimuth ambiguities removal for ship detection using full polarimetric X-band SAR data. IEEE Trans. Geosci. Remote Sens. 2014, 52, 76–88. [Google Scholar] [CrossRef]
- Lee, J.S.; Pottier, E. Polarimetric Radar Imaging: From Basics to Applications; CRC Pressl Llc: Boca Raton, FL, USA, 2009. [Google Scholar]
- Souyris, J.C.; Henry, C.; Adragna, F. On the use of complex SAR image spectral analysis for target detection: Assessment of polarimetry. IEEE Trans. Geosci. Remote Sens. 2003, 41, 2725–2734. [Google Scholar] [CrossRef]
- Hwang, S.I.; Ouchi, K. On a novel approach using MLCC and CFAR for the improvement of ship detection by Synthetic Aperture Radar. IEEE Geosci. Remote Sens. Lett. 2010, 7, 391–395. [Google Scholar] [CrossRef]
- Freeman, A. The effects of noise on polarimetric SAR data. In Proceedings of the 1993 International Geoscience Remote Sensing Symposium (IGARSS), Tokyo, Japan, 18–21 August 1993; Volume 2, pp. 799–802.
- Yamaguchi, Y.; Moriyama, T.; Ishido, M.; Yamada, H. Four-component scattering model for polarimetric SAR image decomposition. IEEE Trans. Geosci. Remote Sens. 2005, 43, 1699–1706. [Google Scholar] [CrossRef]
- Yajima, Y.; Yamaguchi, Y.; Sato, R.; Yamada, H. POLSAR image analysis of wetlands using a modified four-component scattering power decomposition. IEEE Trans. Geosci. Remote Sens. 2008, 46, 1667–1673. [Google Scholar] [CrossRef]
- Yamaguchi, Y.; Sato, A.; Boerner, W.M.; Sato, R. Four-component scattering power decomposition with rotation of coherency matrix. IEEE Trans. Geosci. Remote Sens. 2011, 49, 2251–2258. [Google Scholar] [CrossRef]
- Sato, A.; Yamaguchi, Y.; Singh, G.; Sang-Eun, P. Four-component scattering power decomposition with extended volume scattering model. IEEE Geosci. Remote Sens. Lett. 2012, 9, 166–170. [Google Scholar] [CrossRef]
- Singh, G.; Yamaguchi, Y.; Park, S.E. General four-component scattering power decomposition with unitary transformation of coherency matrix. IEEE Trans. Geosci. Remote Sens. 2013, 51, 3014–3022. [Google Scholar] [CrossRef]
- Arnaud, A. Ship detection by SAR interferometry. In Proceedings of the 1999 International Geoscience Remote Sensing Symposium (IGARSS), Hamburg, Germany, 28 June–2 July 1999; pp. 2616–2618.
- Ouchi, K.; Tamaki, S.; Yaguchi, H.; Iehara, M. Ship detection based on coherence images derived from cross correlation of multilook SAR images. IEEE Geosci. Remote Sens. Lett. 2004, 1, 184–187. [Google Scholar] [CrossRef]
- Li, H.Y.; He, Y.J.; Wang, W.G. Improving ship detection with polarimetric SAR based on convolution between co-polarization channels. Sensors 2009, 9, 1221–1236. [Google Scholar] [CrossRef] [PubMed]
- Wang, Y.; Liu, H. A hierarchical ship detection scheme for high-resolution SAR images. IEEE Trans. Geosci. Remote Sens. 2012, 50, 4173–4184. [Google Scholar]
- Paes, R.L.; Lorenzzetti, J.A.; Gherardi, D.F.M. Ship detection using TerraSAR-X images in the campos basin (Brazil). IEEE Geosci. Remote Sens. Lett. 2010, 7, 545–548. [Google Scholar] [CrossRef]
© 2016 by the authors; licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons by Attribution (CC-BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wei, J.; Zhang, J.; Huang, G.; Zhao, Z. On the Use of Cross-Correlation between Volume Scattering and Helix Scattering from Polarimetric SAR Data for the Improvement of Ship Detection. Remote Sens. 2016, 8, 74. https://doi.org/10.3390/rs8010074
Wei J, Zhang J, Huang G, Zhao Z. On the Use of Cross-Correlation between Volume Scattering and Helix Scattering from Polarimetric SAR Data for the Improvement of Ship Detection. Remote Sensing. 2016; 8(1):74. https://doi.org/10.3390/rs8010074
Chicago/Turabian StyleWei, Jujie, Jixian Zhang, Guoman Huang, and Zheng Zhao. 2016. "On the Use of Cross-Correlation between Volume Scattering and Helix Scattering from Polarimetric SAR Data for the Improvement of Ship Detection" Remote Sensing 8, no. 1: 74. https://doi.org/10.3390/rs8010074
APA StyleWei, J., Zhang, J., Huang, G., & Zhao, Z. (2016). On the Use of Cross-Correlation between Volume Scattering and Helix Scattering from Polarimetric SAR Data for the Improvement of Ship Detection. Remote Sensing, 8(1), 74. https://doi.org/10.3390/rs8010074