The Influence of Printing Orientation on Surface Texture Parameters in Powder Bed Fusion Technology with 316L Steel
Abstract
:1. Introduction
1.1. 3D Printing
1.2. Literature Review
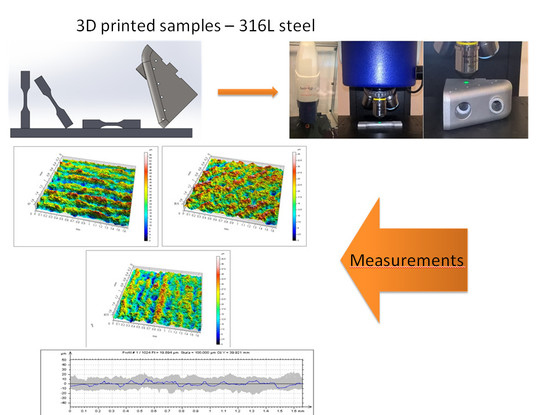
2. Materials and Methods
- Sq—mean square deviation of the surface from the reference surface (root mean square value of the ordinate values within a definition area (A)) calculated from the following equation:
- Ssk—surface asymmetry factor (slant) (quotient of the mean cube value of the ordinate values and the cube of Sq within a definition area (A)) calculated from the following equation:
- Sku—surface slope factor (kurtosis) (quotient of the mean quartic value of the ordinate values and the fourth power of Sq within a definition area (A)) calculated from the following equation:
- Sp—largest peak height value within a definition area, Sv—minus the smallest pit height value within a definition area, and Sz—sum of the maximum peak height value and the maximum pit height value within a definition area calculated from the following equations:
- Sa—arithmetic mean of the absolute of the ordinate values within a definition area (A) calculated from the following equation:
3. Results
4. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Hull, C. Apparatus for Production of Three-Dimensional Objects by Stereolithography. U.S. Patent 4575330A, 19 December 1986. [Google Scholar]
- Campbell, I.; Bourell, D.; Gibson, I. Additive manufacturing: Rapid prototyping comes of age. Rapid Prototyp. J. 2012, 18, 255–258. [Google Scholar] [CrossRef] [Green Version]
- Crump, S. Apparatus and Method for Creating Three-Dimensional Objects. U.S. Patent 5121329A, 9 June 1989. [Google Scholar]
- Deckard, C.R.; Beaman, J.J.; Darrah, J.F. Method for Selective Laser Sintering with Layerwise Cross-Scanning. U.S. Patent 5155324A, 17 October 1986. [Google Scholar]
- Feygin, M.; Shkolnik, A.; Diamond, M.N.; Dvorskiy, E. Laminated Object Manufacturing System. U.S. Patent 5730817A, 22 April 1996. [Google Scholar]
- Meiners, W.; Wissenbach, K.; Gasser, A. Shaped Body Especially Prototype or Replacement Part Production. DE Patent Application No. 19649865, 2 December 1996. [Google Scholar]
- Sachs, E.M.; Haggerty, J.S.; Cima, M.J.; Williams, P.A. Three-Dimensional Printing Techniques. U.S. Patent 5204055A, 20 April 1993. [Google Scholar]
- Leu, M.C.; Guo, N. Additive manufacturing: Technology, applications and research needs. Front. Mech. Eng. 2013, 8, 215–243. [Google Scholar] [CrossRef]
- Gibson, I.; Rosen, D.W.; Stucker, B. Additive Manufacturing Technologies: 3D Printing, Rapid Prototyping, and Direct Digital Manufacturing; Springer: New York, NY, USA, 2013. [Google Scholar]
- Adamczak, S.; Zmarzły, P.; Kozior, T.; Gogolewski, D. Analysis of the dimensional accuracy of casting models manufactured by fused deposition modeling technology. Eng. Mechan. 2017, 66–69. [Google Scholar]
- Zmarzły, P.; Kozior, T.; Gogolewski, D. Dimensional and Shape Accuracy of Foundry Patterns Fabricated Through Photo-Curing. Teh. Vjesn.-Tech. Gaz. 2019, 26, 1576–1584. [Google Scholar] [CrossRef]
- Zmarzły, P.; Kozior, T.; Gogolewski, D. Design guidelines for 3D printed molds for plastic casting. J. Eng. Fibers Fabrics 2020, 15, 1–10. [Google Scholar] [CrossRef]
- Bochnia, J.; Blasiak, S. Anisotrophy of mechanical properties of a material which is shaped incrementally using polyjet technology. Eng. Mechan. 2016, 74–77. [Google Scholar]
- Kozior, T.; Mamun, A.; Trabelsi, M.; Sabantina, L.; Ehrmann, A. Quality of the surface texture and mechanical properties of FDM printed samples after thermal and chemical treatment. Stroj. Vestn. 2020, 1, 1–10. [Google Scholar] [CrossRef] [Green Version]
- Dizon, J.R.C.; Espera, A.H.; Chen, Q.Y.; Advincula, R.C. Mechanical characterrization of 3D-printed polymers. Addit. Manuf. 2018, 20, 44–67. [Google Scholar] [CrossRef]
- Damon, J.; Dietrich, S.; Vollert, F.; Gibmeier, J.; Schulze, V. Process dependent porosity and the influence of shot peening on porosity morphology regarding selective laser melted AlSi10Mg parts. Addit. Manuf. 2018, 20, 77–89. [Google Scholar] [CrossRef]
- Kozior, T. Analysis of the Influence of Process Parameters of Selected Additive Technologies on the Geometry and Mechanical Properties of Products. Ph.D. Thesis, Kielce University of Technology, Kielce, Poland, 2018. [Google Scholar]
- Puigoriol, J.M.; Alsina, A.; Salazar-Martin, A.G.; Gomez-Gras, G.; Perez, M.A. Flexural fatigue properties of polycarbonate fused-deposition modelling specimens. Mater. Des. 2018, 155, 414–421. [Google Scholar] [CrossRef]
- Singh, S.; Prakash, C.; Antil, P.; Singh, R.; Krolczyk, G.; Pruncu, C.I. Analysis for Investigating the Quality Characteristics of Aluminium Matrix Composites Prepared through Fused Deposition Modelling Assisted Investment Casting. Materials 2019, 12, 1907. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Gapinski, B.; Wieczorowski, M.; Bak, A.; Pereira Dominguez, A.; Mathia, T. The assessment of accuracy of inner shapes manufactured by FDM. In Proceedings of the 21st International ESAFORM Conference on Material Forming (ESAFORM 2018)—AIP Conference Proceedings, Palermo, Italy, 23–25 April 2018; Volume 1960. [Google Scholar] [CrossRef]
- Niu, X.; Singh, S.; Garg, A.; Singh, H.; Panda, B.; Peng, X.; Zhang, Q. Review of materials used in laser-aided additive manufacturing processes to produce metallic products. Front. Mechan. Eng. 2019, 14, 282–298. [Google Scholar] [CrossRef] [Green Version]
- Khaled, T. Available online: https://www.faa.gov/aircraft/air_cert/design_approvals/csta/publications/media/additive_manufacturing.pdf (accessed on 31 August 2015).
- Unger, L.; Scheideler, M.; Meyer, P.; Harland, J.; Gorzen, A.; Wortmann, M.; Dreyer, A.; Ehrmann, A. Increasing Adhesion of 3D Printing on Textile Fabrics by Polymer Coating. Tekstilec 2018, 61, 265–271. [Google Scholar] [CrossRef]
- Kozior, T.; Mamun, A.; Trabelsi, M.; Wortmann, M.; Sabantina, L.; Ehrmann, A. Electrospinning on 3D Printed Polymers for Mechanically Stabilized Filter Composites. Polymers 2019, 11, 2034. [Google Scholar] [CrossRef] [Green Version]
- Kozior, T.; Mamun, A.; Trabelsi, M.; Sabantina, L.; Ehrmann, A. Stabilization of Electrospun Nanofiber Mats Used for Filters by 3D Printing. Polymers 2019, 11, 1618. [Google Scholar] [CrossRef] [Green Version]
- Kozior, T.; Blachowicz, T.; Ehrmann, A. Adhesion of three-dimensional printing on textile fabrics: Inspiration from and for other research areas. J. Eng. Fibers Fabrics 2020, 15, 1–6. [Google Scholar] [CrossRef] [Green Version]
- Coniglio, N.; Sivarupan, T.; Mansori, M.E. Investigation of process parameter effect on anisotropic properties of 3D printed sand molds. J. Adv. Manuf. Technol. 2018, 94, 2175–2185. [Google Scholar] [CrossRef]
- Casting 3D Printer Manufacturer. Available online: https://www.exone.com (accessed on 12 July 2018).
- Rokicki, P.; Budzik, G.; Kubiak, K.; Bernaczek, J.; Dziubek, T.; Magniszewski, M.; Nowotnik, A.; Sieniawski, J.; Matysiak, H.; Cygan, R.; et al. Rapid prototyping in manufacturing of core models of aircraft engine blades. Aircr. Eng. Aerosp. Technol. 2014, 86, 323–327. [Google Scholar] [CrossRef]
- Adamczak, S.; Zmarzły, P. Research of the influence of the 2D and 3D surface roughness parameters of bearing raceways on the vibration level. J. Phys. Conf. Ser. 2019, 1183, 1–10. [Google Scholar] [CrossRef]
- Segonds, F. Design by Additive Manufacturing: An application in aeronautics and defence. Virtual Phys. Prototyp. 2018, 13, 237–245. [Google Scholar] [CrossRef] [Green Version]
- Blasiak, S.; Takosoglu, I.E.; Laski, P.A. Optimizing the Flow Rate in a Pneumatic Directional Control Valve. Eng. Mechan. 2014, 96–99. [Google Scholar]
- Borsuk-Nastaj, B.; Młynarski, M. Selective laser melting (SLM) technique in fixed prosthetic restorations. Protet. Stomatol. 2012, LXII, 203–209. [Google Scholar]
- Dikova, T.; Vasilev, T.; Dzhendov, D.; Ivanova, E. Investigation the fitting accuracy of cast and SLM Co-Cr dental bridges using cad software. J. IMAB 2017, 23, 1688–1696. [Google Scholar] [CrossRef] [Green Version]
- Lipowicz, A. Development of Designing and Fabrication Methodology of biomedical Models with Generative Technologies. Ph.D. Thesis, Wrocław University of Science and Technology, Wrocław, Poland, 2008. [Google Scholar]
- Voon, S.L.; An, J.; Wong, G.; Zhang, Y.; Chua, C.K. 3D food printing: A categorised review of inks and their development. Virtual Phys. Prototyp. 2019, 1–16. [Google Scholar] [CrossRef]
- Blachowicz, T.; Ehrmann, A. 3D Printed MEMS Technology-Recent Developments and Applications. Micromachines 2020, 11, 434. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Joshi, N.; Kohler, E.; Enokssen, P. MEMS based micro aerial vehicles. J. Phys. Conf. Ser. 2016, 757, 012035. [Google Scholar] [CrossRef]
- Wang, Y.J.; Gao, L.B.; Fan, S.F.; Zhou, W.Z.; Li, X.; Lu, Y. 3D printed micro-mechanical device (MMD) for in situ tensile testing of micro/nanowires. Extreme Mechan. Lett. 2019, 33, 100575. [Google Scholar] [CrossRef]
- Thompson, A.; Senin, N.; Giusca, C.; Leach, R. Topography of selectively laser melted surfaces: A comparison of different measurement methods. CIRP Ann.-Manuf. Technol. 2017, 66, 543–546. [Google Scholar] [CrossRef]
- Thompson, A.; Körner, L.; Senin, N.; Lawes, S.; Maskery, I.; Leach, R. Measurement of internal surfaces of additively manufactured parts by X-ray computed tomography. In Proceedings of the 7th Conference on Industrial Computed Tomography 2017, Leuven, Belgium, 7–9 February 2017. [Google Scholar]
- Bartkowiak, T.; Brown, C.A. Multiscale 3D Curvature Analysis of Processed Surface Textures of Aluminum Alloy 6061 T6. Materials 2019, 12, 257. [Google Scholar] [CrossRef] [Green Version]
- Kamarudin, K.; Wahab, M.S.; Shayfull, Z.; Ahmed, A.; Raus, A.A. Dimensional Accuracy and Surface Roughness Analysis for AlSi10Mg Produced by Selective Laser Melting (SLM). Matec Web Conf. 2016. [Google Scholar] [CrossRef] [Green Version]
- Townsend, A.; Senin, N.; Blunt, L.; Leach, R.K.; Taylor, J.S. Surface texture metrology for metal additive manufacturing: A review. Precis. Eng. 2016, 46, 34–47. [Google Scholar] [CrossRef] [Green Version]
- Chlebus, E.; Kurzac, J.; Szymczyk, P.; Ziółkowski, G. Application of X-ray CT method for discontinuity and porosity detection in 316L stainless steel parts produced with SLM technology. Arch. Civ. Mechan. Eng. 2014, 14, 608–614. [Google Scholar] [CrossRef]
- Kurzynowski, T.; Gruber, K.; Stopyra, W.; Kuźnicka, B.; Chlebus, E. Correlation between process parameters, microstructure and properties of 316 L stainless steel processed by selective laser melting. Mater. Sci. Eng. 2018, 718, 64–73. [Google Scholar] [CrossRef]
- Kaynaka, Y.; Kitayb, O. The effect of post-processing operations on surface characteristics of 316L stainless steel produced by selective laser melting. Addit. Manuf. 2019, 26, 84–93. [Google Scholar] [CrossRef]
- Heiden, M.J.; Deibler, L.A.; Rodelas, J.M.; Koepke, J.R.; Tung, D.J.; Saiz, D.J.; Jared, B.H. Evolution of 316L stainless steel feedstock due to laser powder bed fusion process. Addit. Manuf. 2019, 25, 84–93. [Google Scholar] [CrossRef]
- Lodhia, M.J.K.; Deenb, K.M.; Greenlee-Wackerc, M.C.; Haidera, W. Additively manufactured 316L stainless steel with improved corrosion resistance and biological response for biomedical applications. Addit. Manuf. 2019, 27, 1–19. [Google Scholar] [CrossRef]
- Available online: https://www.astm.org/DATABASE.CART/HISTORICAL/E8E8M-13.htm (accessed on 28 June 2020).
- Concept Laser M2 - 3D Printer Manufacturer. Available online: https://www.concept-laser.de (accessed on 12 July 2019).
- ISO 4287: Geometrical Product Specification (GPS)-Surface Texture: Profile Method-Terms, Definition and Surface Texture Parameters; International Organization for Standardization (ISO): Geneva, Switzerland, 1997.
- ISO 25178-2: Geometrical Product Specifications (GPS)—Surface Texture: Areal—Part 2: Terms, Definitions and Surface Texture Parameters; International Organization for Standardization (ISO): Geneva, Switzerland, 2012.
- Whitehouse, D.J. Handbook of Surface and Nanometrology, 2nd ed.; CRC Press: Boca Raton, FL, USA, 2011. [Google Scholar]















| Component | Cr | Ni | Mo | Mn | Si | P | C | S | Fe |
|---|---|---|---|---|---|---|---|---|---|
| Indicative value, % | 16.5–18.5 | 10.0–13.0 | 2–2.5 | 0–2.0 | 0–1.0 | 0–0.045 | 0–0.030 | 0–0.030 | Balance |
| Properties | 90°, Upright | 45°, Polar Angle | 0°, Horizontal |
|---|---|---|---|
| Yield strength, Rp0,2 | 374 ± 5 N/mm2 | 385 ± 6 N/mm2 | 330 ± 8 N/mm2 |
| Tensile strength, Rm | 650 ± 5 N/mm2 | 640 ± 7 N/mm2 | 529 ± 8 N/mm2 |
| Elongation, A | (65 ± 4)% | (63 ± 5)% | (63 ± 5)% |
| Young’s modulus | ca. 200 × 103 N/mm2 | ||
| Hardness | 20 HRC | ||
| Sample Number | Rp, (µm) | Rv, (µm) | Rz, (µm) | Rc, (µm) | Rt, (µm) | Ra, (µm) | Rq, (µm) | Rsk | Rku | Rmr, (%) | Rdc, (µm) | RSm, (µm) | Rdq, (0) |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| 1 | 24.6 | 19.8 | 44.4 | 27.3 | 52.3 | 8.4 | 10.4 | 0.491 | 3.479 | 0.9 | 17.0 | 0.228 | 25.0 |
| 2 | 11.9 | 9.5 | 21.5 | 11.7 | 25.6 | 3.8 | 4.8 | 0.41 | 3.833 | 1.0 | 7.6 | 0.178 | 15.0 |
| 3 | 12.2 | 9.0 | 21.2 | 11.0 | 26.9 | 3.6 | 4.6 | 0.648 | 4.54 | 0.9 | 7.2 | 0.171 | 14.9 |
| 4 | 8.5 | 8.3 | 16.8 | 9.0 | 19.2 | 3.0 | 3.7 | −0.007 | 2.943 | 1.1 | 6.4 | 0.16 | 13.1 |
| 5 | 9.4 | 8.5 | 17.9 | 9.4 | 20.5 | 3.1 | 3.9 | 0.167 | 3.129 | 1.1 | 6.6 | 0.15 | 14.4 |
| 6 | 7.9 | 8.1 | 16.0 | 9.0 | 18.2 | 2.9 | 3.6 | −0.027 | 2.821 | 1.4 | 6.3 | 0.164 | 12.7 |
| 7 | 9.3 | 9.1 | 18.4 | 10.1 | 21.0 | 3.4 | 4.1 | 0.041 | 2.964 | 1.3 | 7.1 | 0.167 | 13.8 |
| 8 | 9.5 | 8.1 | 17.6 | 9.6 | 20.5 | 3.1 | 3.9 | 0.34 | 3.337 | 1.3 | 6.5 | 0.171 | 12.9 |
| 9 | 10.4 | 8.8 | 19.2 | 10.5 | 22.6 | 3.5 | 4.3 | 0.229 | 3.18 | 1.2 | 7.3 | 0.174 | 14.0 |
| 10 | 9.4 | 9.0 | 18.5 | 10.2 | 21.5 | 3.3 | 4.1 | 0.087 | 3.197 | 1.2 | 7.1 | 0.171 | 13.9 |
| 11.3 | 9.8 | 21.2 | 11.8 | 24.8 | 3.8 | 4.8 | 0.238 | 3.342 | 1.1 | 7.9 | 0.173 | 15.0 | |
| 4.9 | 3.5 | 8.3 | 5.5 | 10.0 | 1.6 | 2.0 | 0.228 | 0.512 | 0.2 | 3.2 | 0.021 | 3.6 | |
| UA0 | 1.5 | 1.1 | 2.6 | 1.7 | 3.2 | 0.5 | 0.6 | 0.072 | 0.162 | 0.1 | 1.0 | 0.007 | 1.1 |
| 11 | 9.2 | 9.6 | 18.8 | 11.4 | 21.7 | 3.6 | 4.4 | −0.071 | 2.923 | 1.5 | 7.6 | 0.199 | 12.4 |
| 12 | 13.7 | 12.0 | 25.7 | 16.2 | 29.4 | 4.9 | 6.0 | 0.22 | 2.962 | 1.1 | 10.3 | 0.222 | 14.9 |
| 13 | 13.4 | 11.1 | 24.5 | 15.3 | 29.9 | 4.9 | 6.0 | 0.445 | 3.481 | 1.3 | 10.2 | 0.234 | 14.1 |
| 14 | 12.1 | 11.2 | 23.4 | 14.7 | 26.7 | 4.7 | 5.7 | 0.149 | 2.771 | 1.2 | 10.1 | 0.23 | 13.9 |
| 15 | 11.4 | 10.8 | 22.3 | 13.3 | 24.7 | 4.2 | 5.1 | 0.059 | 2.724 | 1.2 | 8.8 | 0.208 | 14.0 |
| 16 | 12.1 | 12.6 | 24.7 | 15.7 | 28.7 | 4.8 | 5.9 | −0.028 | 2.735 | 1.1 | 10.4 | 0.232 | 14.3 |
| 17 | 11.8 | 11.4 | 23.1 | 15.0 | 26.2 | 4.5 | 5.5 | 0.081 | 2.727 | 1.2 | 9.6 | 0.223 | 14.0 |
| 18 | 10.4 | 10.1 | 20.5 | 11.8 | 23.4 | 3.8 | 4.7 | 0.048 | 3.08 | 1.2 | 7.8 | 0.183 | 14.3 |
| 19 | 11.3 | 10.5 | 21.8 | 12.4 | 25.8 | 4.1 | 5.0 | 0.099 | 3.238 | 1.2 | 8.5 | 0.205 | 13.4 |
| 20 | 12.0 | 11.6 | 23.5 | 14.4 | 27.1 | 4.6 | 5.6 | 0.127 | 2.871 | 1.2 | 9.7 | 0.212 | 14.6 |
| 11.7 | 11.1 | 22.8 | 14.0 | 26.4 | 4.4 | 5.4 | 0.113 | 2.951 | 1.2 | 9.3 | 0.215 | 14.0 | |
| 1.3 | 0.9 | 2.1 | 1.7 | 2.6 | 0.5 | 0.6 | 0.143 | 0.251 | 0.1 | 1.0 | 0.016 | 0.7 | |
| UA45 | 0.4 | 0.3 | 0.7 | 0.5 | 0.8 | 0.1 | 0.2 | 0.045 | 0.079 | 0.0 | 0.3 | 0.005 | 0.2 |
| 21 | 11.5 | 10.1 | 21.6 | 12.9 | 25.3 | 4.1 | 5.0 | 0.282 | 3.172 | 1.2 | 8.5 | 0.176 | 14.5 |
| 22 | 13.7 | 13.7 | 27.4 | 16.7 | 31.5 | 5.1 | 6.3 | −0.01 | 2.97 | 1.0 | 10.6 | 0.203 | 15.8 |
| 23 | 11.9 | 12.5 | 24.5 | 15.4 | 27.3 | 4.7 | 5.7 | −0.066 | 2.733 | 1.3 | 10.2 | 0.194 | 15.3 |
| 24 | 11.4 | 10.0 | 21.4 | 12.8 | 24.2 | 4.1 | 5.0 | 0.153 | 2.756 | 1.1 | 8.8 | 0.184 | 14.9 |
| 25 | 11.7 | 10.8 | 22.5 | 14.0 | 26.3 | 4.4 | 5.3 | 0.099 | 3.199 | 1.2 | 9.1 | 0.204 | 14.4 |
| 26 | 11.7 | 12.4 | 24.1 | 15.7 | 27.3 | 4.7 | 5.7 | −0.105 | 2.747 | 1.2 | 10.2 | 0.195 | 14.6 |
| 27 | 12.1 | 10.5 | 22.6 | 12.4 | 25.5 | 4.0 | 5.0 | 0.247 | 3.213 | 1.0 | 8.2 | 0.178 | 14.5 |
| 28 | 11.0 | 10.1 | 21.2 | 12.6 | 23.8 | 3.9 | 4.8 | 0.162 | 2.966 | 1.3 | 8.1 | 0.182 | 13.1 |
| 29 | 11.0 | 10.5 | 21.5 | 12.6 | 24.8 | 4.0 | 5.0 | 0.046 | 2.903 | 1.2 | 8.5 | 0.183 | 13.4 |
| 30 | 12.1 | 13.1 | 25.2 | 16.8 | 29.8 | 5.2 | 6.2 | −0.1 | 3.016 | 1.4 | 11.2 | 0.209 | 15.1 |
| 11.8 | 11.4 | 23.2 | 14.2 | 26.6 | 4.4 | 5.4 | 0.071 | 2.968 | 1.2 | 9.3 | 0.191 | 14.6 | |
| 0.8 | 1.4 | 2.0 | 1.8 | 2.5 | 0.5 | 0.5 | 0.140 | 0.186 | 0.1 | 1.1 | 0.012 | 0.8 | |
| UA90 | 0.2 | 0.4 | 0.6 | 0.6 | 0.8 | 0.1 | 0.2 | 0.044 | 0.059 | 0.0 | 0.4 | 0.004 | 0.3 |
| 11.6 | 10.8 | 22.4 | 13.3 | 25.9 | 4.2 | 5.2 | 0.141 | 3.087 | 1.2 | 8.9 | 0.193 | 14.5 | |
| 2.8 | 2.3 | 5.0 | 3.5 | 6.0 | 1.0 | 1.3 | 0.1841 | 0.3814 | 0.1 | 2.1 | 0.0236 | 2.1 | |
| UA | 0.5 | 0.4 | 0.9 | 0.6 | 1.1 | 0.2 | 0.2 | 0.0336 | 0.0696 | 0.0 | 0.4 | 0.0043 | 0.4 |
| Sample Number | Sq, (µm) | Ssk | Sku | Sp, (µm) | Sv, (µm) | Sz, (µm) | Sa, (µm) | Smr, (%) | Smc, (µm) | Sxp, (µm) | Sal | Str | Std,° | Sdq | Sdr, (%) |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| 1 | 17.1 | 1.085 | 4.381 | 70.8 | 42.1 | 113.0 | 13.2 | 0.01 | 22.5 | 20.5 | 0.109 | 0.389 | 26.499 | 0.68 | 19.9 |
| 2 | 8.7 | 1.182 | 8.776 | 66.1 | 24.2 | 90.2 | 6.5 | 0.012 | 10.3 | 14.5 | 0.083 | 0.1 | 3.974 | 0.433 | 8.6 |
| 3 | 7.4 | 1.272 | 6.821 | 42.1 | 20.0 | 62.0 | 5.4 | 0.013 | 8.6 | 11.3 | 0.065 | 0.444 | 153.521 | 0.43 | 8.5 |
| 4 | 5.7 | 0.005 | 3.008 | 24.6 | 21.1 | 45.6 | 4.6 | 0.004 | 7.4 | 11.3 | 0.054 | 0.107 | 0.146 | 0.374 | 6.6 |
| 5 | 5.9 | 0.039 | 2.95 | 34.9 | 23.1 | 58.0 | 4.8 | 0.001 | 7.7 | 11.5 | 0.049 | 0.059 | 0.099 | 0.417 | 8.1 |
| 6 | 5.7 | 0.146 | 2.989 | 23.1 | 21.8 | 44.9 | 4.5 | 0.003 | 7.4 | 10.8 | 0.052 | 0.13 | 0.12 | 0.37 | 6.5 |
| 7 | 8.4 | 0.229 | 2.703 | 32.3 | 25.4 | 57.7 | 6.9 | 0.002 | 11.1 | 14.8 | 0.05 | 0.061 | 0.076 | 0.436 | 8.9 |
| 8 | 8.4 | 0.703 | 3.74 | 36.0 | 22.9 | 58.8 | 6.6 | 0.013 | 11.1 | 12.7 | 0.08 | 0.096 | 4.43 | 0.388 | 7.0 |
| 9 | 12.2 | 1.114 | 4.258 | 47.4 | 28.7 | 76.1 | 9.2 | 0.016 | 17.5 | 15.1 | 0.118 | 0.142 | 8.701 | 0.413 | 8.0 |
| 10 | 6.2 | 0.214 | 3.108 | 27.2 | 22.5 | 49.7 | 4.9 | 0.007 | 8.0 | 11.5 | 0.054 | 0.355 | 0.143 | 0.403 | 7.6 |
| 11.6 | 0.650 | 3.745 | 49.0 | 32.3 | 81.3 | 9.1 | 0.009 | 15.3 | 16.0 | 0.082 | 0.372 | 13.321 | 0.542 | 13.8 | |
| 3.6 | 0.524 | 1.997 | 16.6 | 6.4 | 21.7 | 2.7 | 0.005 | 5.0 | 3.0 | 0.025 | 0.147 | 47.695 | 0.089 | 3.9 | |
| UA0 | 2.3 | 0.332 | 1.263 | 10.5 | 4.1 | 13.7 | 1.7 | 0.003 | 3.2 | 1.9 | 0.016 | 0.093 | 30.165 | 0.057 | 2.5 |
| 11 | 5.4 | −0.018 | 2.939 | 17.0 | 18.9 | 35.9 | 4.3 | 0.035 | 7.1 | 10.7 | 0.062 | 0.209 | 51.199 | 0.335 | 5.3 |
| 12 | 7.3 | 0.353 | 3.169 | 39.8 | 24.4 | 64.2 | 5.8 | 0.008 | 9.8 | 12.5 | 0.06 | 0.051 | 135.034 | 0.393 | 7.3 |
| 13 | 8.2 | 1.034 | 5.137 | 43.7 | 20.3 | 64.0 | 6.3 | 0.002 | 10.1 | 11.6 | 0.07 | 0.127 | 26.462 | 0.386 | 7.1 |
| 14 | 7.0 | 0.26 | 2.734 | 25.4 | 40.6 | 66.0 | 5.7 | 0.005 | 9.5 | 12.1 | 0.058 | 0.093 | 134.985 | 0.377 | 6.7 |
| 15 | 6.4 | 0.14 | 2.924 | 26.1 | 21.5 | 47.6 | 5.3 | 0.002 | 8.4 | 12.1 | 0.063 | 0.177 | 134.998 | 0.374 | 6.6 |
| 16 | 7.4 | 0.192 | 3.134 | 32.0 | 23.9 | 55.9 | 5.9 | 0.004 | 9.5 | 14.0 | 0.058 | 0.053 | 116.483 | 0.375 | 6.6 |
| 17 | 6.8 | 0.229 | 2.759 | 26.4 | 39.7 | 66.1 | 5.5 | 0.002 | 9.3 | 11.9 | 0.062 | 0.085 | 135.007 | 0.377 | 6.7 |
| 18 | 5.9 | −0.039 | 3.209 | 22.3 | 26.9 | 49.2 | 4.7 | 0.005 | 7.5 | 11.7 | 0.052 | 0.256 | 47.899 | 0.385 | 7.0 |
| 19 | 6.5 | 0.378 | 3.407 | 29.2 | 20.1 | 49.2 | 5.1 | 0.002 | 8.3 | 11.7 | 0.065 | 0.255 | 141.207 | 0.363 | 6.2 |
| 20 | 7.4 | 0.278 | 2.799 | 26.0 | 24.9 | 50.9 | 5.9 | 0.001 | 9.9 | 12.6 | 0.07 | 0.06 | 134.966 | 0.39 | 7.1 |
| 6.4 | 0.130 | 2.869 | 21.5 | 21.9 | 43.4 | 5.1 | 0.018 | 8.5 | 11.6 | 0.066 | 0.135 | 93.083 | 0.363 | 6.2 | |
| 1.4 | 0.209 | 0.099 | 6.4 | 4.3 | 10.6 | 1.1 | 0.024 | 2.0 | 1.3 | 0.006 | 0.105 | 59.232 | 0.039 | 1.3 | |
| UA45 | 0.9 | 0.132 | 0.063 | 4.0 | 2.7 | 6.7 | 0.7 | 0.015 | 1.3 | 0.8 | 0.004 | 0.067 | 37.462 | 0.025 | 0.8 |
| 21 | 5.9 | 0.422 | 3.385 | 24.1 | 22.5 | 46.6 | 4.7 | 0.022 | 7.9 | 10.1 | 0.058 | 0.135 | 85.581 | 0.363 | 6.2 |
| 22 | 7.3 | 0.303 | 3.106 | 28.4 | 23.2 | 51.6 | 5.8 | 0.001 | 9.4 | 12.8 | 0.058 | 0.07 | 93.487 | 0.381 | 6.8 |
| 23 | 6.8 | 0.078 | 2.99 | 24.5 | 25.4 | 49.9 | 5.4 | 0.009 | 8.9 | 12.6 | 0.057 | 0.398 | 93.759 | 0.377 | 6.7 |
| 24 | 5.9 | 0.226 | 2.828 | 24.0 | 18.8 | 42.8 | 4.8 | 0.001 | 7.8 | 10.5 | 0.065 | 0.148 | 89.969 | 0.377 | 6.7 |
| 25 | 6.6 | 0.487 | 4.254 | 54.8 | 20.6 | 75.4 | 5.2 | 0 | 8.3 | 11.8 | 0.075 | 0.411 | 89.996 | 0.368 | 6.3 |
| 26 | 6.8 | 0.074 | 3.081 | 25.1 | 28.5 | 53.6 | 5.4 | 0.008 | 8.9 | 12.8 | 0.058 | 0.33 | 63.504 | 0.358 | 6.1 |
| 27 | 6.2 | 0.336 | 3.296 | 24.1 | 19.6 | 43.6 | 4.9 | 0.007 | 8.1 | 11.2 | 0.078 | 0.181 | 90.037 | 0.362 | 6.2 |
| 28 | 5.8 | 0.315 | 3.205 | 26.7 | 18.8 | 45.5 | 4.6 | 0.001 | 7.6 | 10.2 | 0.058 | 0.238 | 85.563 | 0.324 | 5.0 |
| 29 | 5.8 | 0.113 | 2.988 | 25.2 | 18.6 | 43.9 | 4.6 | 0.006 | 7.6 | 11.1 | 0.06 | 0.072 | 89.973 | 0.327 | 5.1 |
| 30 | 7.5 | 0.132 | 2.856 | 25.6 | 24.9 | 50.6 | 6.0 | 0.025 | 9.8 | 13.9 | 0.06 | 0.274 | 89.999 | 0.366 | 6.4 |
| 6.7 | 0.277 | 3.121 | 24.9 | 23.7 | 48.6 | 5.3 | 0.024 | 8.8 | 12.0 | 0.059 | 0.205 | 87.790 | 0.365 | 6.3 | |
| 1.1 | 0.205 | 0.374 | 1.1 | 1.7 | 2.8 | 1.0 | 0.002 | 1.3 | 2.7 | 0.001 | 0.098 | 3.124 | 0.002 | 0.1 | |
| UA90 | 0.7 | 0.130 | 0.237 | 0.7 | 1.1 | 1.8 | 0.6 | 0.001 | 0.8 | 1.7 | 0.001 | 0.062 | 1.976 | 0.001 | 0.1 |
| 7.3 | 0.376 | 3.564 | 32.5 | 24.5 | 57.0 | 5.7 | 0.008 | 9.5 | 12.4 | 0.065 | 0.184 | 70.927 | 0.390 | 7.3 | |
| 2.3 | 0.381 | 1.306 | 12.9 | 6.2 | 15.7 | 1.7 | 0.008 | 3.1 | 2.0 | 0.016 | 0.123 | 52.658 | 0.061 | 2.6 | |
| UA | 0.4 | 0.070 | 0.238 | 2.3 | 1.1 | 2.9 | 0.3 | 0.001 | 0.6 | 0.4 | 0.003 | 0.022 | 9.614 | 0.011 | 0.5 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kozior, T.; Bochnia, J. The Influence of Printing Orientation on Surface Texture Parameters in Powder Bed Fusion Technology with 316L Steel. Micromachines 2020, 11, 639. https://doi.org/10.3390/mi11070639
Kozior T, Bochnia J. The Influence of Printing Orientation on Surface Texture Parameters in Powder Bed Fusion Technology with 316L Steel. Micromachines. 2020; 11(7):639. https://doi.org/10.3390/mi11070639
Chicago/Turabian StyleKozior, Tomasz, and Jerzy Bochnia. 2020. "The Influence of Printing Orientation on Surface Texture Parameters in Powder Bed Fusion Technology with 316L Steel" Micromachines 11, no. 7: 639. https://doi.org/10.3390/mi11070639
APA StyleKozior, T., & Bochnia, J. (2020). The Influence of Printing Orientation on Surface Texture Parameters in Powder Bed Fusion Technology with 316L Steel. Micromachines, 11(7), 639. https://doi.org/10.3390/mi11070639