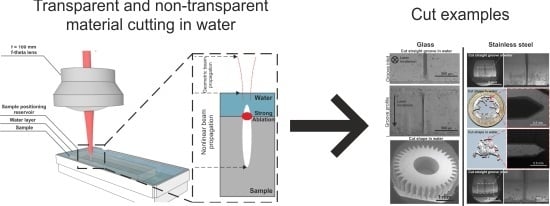
Analysis of the Micromachining Process of Dielectric and Metallic Substrates Immersed in Water with Femtosecond Pulses
Abstract
:1. Introduction
2. Experimental Section

2.1. Ablation Patterns Acquired while Focusing Intense Laser Pulses in Water
2.2. Optimization of Micromachining Process Using DOE Techniques
| Parameters | Numeric Values | ||
|---|---|---|---|
| (S) Scanning speed (mm/s) | 20 | 760 | 1500 |
| (T) Water layer thickness (mm) | 0.6 | 1 | 1.5 |
| (R) Repetition rate (kHz) | 33.3 | 116.6 | 200 |
| (F) Focal position (mm) | –1 | 0 | 1 |
2.3. Shadowgraphic Investigation

3. Results and Discussion
3.1. Ablation Patterns Acquired while Focusing Intense Laser Pulses in Water

3.2. Optimization of the Micromachining Process
| Parameters | Numeric Value Range | ||
|---|---|---|---|
| (S) Scanning speed (mm/s) | 20 | - | 760 |
| (T) Water layer thickness (mm) | 0.3 | - | 0.9 |
| (R) Repetition rate (kHz) | 33.3 | - | 108.3 |
| (F) Focal position (mm) | -1 | - | 0.1 |




3.3. Shadowgraphic Investigation

4. Conclusions
Acknowledgments
Author Contributions
Conflicts of Interest
References
- Osellame, R.; Cerullo, G.; Ramponi, R. Femtosecond Laser Micromachining; Springer-Verlag: Berlin, Germany, 2012. [Google Scholar]
- Misawa, H.; Juodkazis, S. 3D Laser Microfabrication Principles and Applications; Wiley-VCH Verlag: Weinheim, Germany, 2006. [Google Scholar]
- Schaffer, C.B.; Brodeur, A.; Mazur, E. Laser-induced breakdown and damage in bulk transparent materials induced by tightly focused femtosecond laser pulses. Meas. Sci. Technol. 2001, 12, 1784. [Google Scholar] [CrossRef]
- Couairon, A.; Mysyrowicz, A. Femtosecond Filamentation in Transparent Media. Phys. Rep. 2007, 44, 47–189. [Google Scholar] [CrossRef]
- Shah, L.; Tawneym, J.; Richardson, M.; Richardson, K. Self-Focusing During Femtosecond Micromachining of Silicate Glasses. IEEE J. Quantum Electron. 2004, 40, 57–68. [Google Scholar] [CrossRef]
- Ran, A.; Yan, L.; Yan-Ping, D.; Ying, F.; Hong, Y.; Qi-Huang, G. Laser Micro-Hole Drilling of Soda-Lime Glass with Femtosecond Pulses. Chin. Phys. Lett. 2004, 21, 2465. [Google Scholar] [CrossRef]
- Wu, Z.; Jiang, H.; Sun, Q.; Guo, H.; Yang, H.; Gong, Q. Micro-ablation at the front and rear surfaces of a fused silica window by using a femtosecond laser pulse in air. J. Appl. Opt. 2004, 6, 671. [Google Scholar] [CrossRef]
- Byer, R.L.; Harkin, A.; Ashmore, J.; Stone, H.W.; Shen, M.; Mazur, E. Morphology of femtosecond-laser-ablated borosilicate glass surfaces. Appl. Phys. Lett. 2003, 83, 3030–3032. [Google Scholar]
- Schaffer, Ch.B.; Brodeur, A.; Garcia, J.F.; Mazur, E. Micromachining bulk glass by use of femtosecond laser pulses with nanojoule energy. Opt. Lett. 2001, 26, 93–95. [Google Scholar] [CrossRef] [PubMed]
- Ye, D.; Guang-Jun, Y.; Guo-Rui, W.; Hong-Liang, M.; Xiao-Na, Y.; Hong, M. The effect of spherical aberration on temperature distribution inside glass by irradiation of a high repetition rate femtosecond pulse laser. Chin. Phys. B 2012, 21, 025201. [Google Scholar]
- Vitek, D.N.; Adams, D.E.; Johnson, A.; Tsai, P.S.; Backus, S.; Durfee, C.G.; Kleinfeld, D.; Squier, J.A. Temporally focused femtosecond laser pulses for low numerical aperture micromachining through optically transparent materials. Opt. Exp. 2010, 18, 18086–18094. [Google Scholar] [CrossRef] [PubMed]
- Vitek, D.N.; Block, E.; Bellouard, Y.; Adams, D.E.; Backus, S.; Kleinfeld, D.; Durfee, C.G.; Squier, J.A. Spatio-temporally focused femtosecond laser pulses for nonreciprocal writing in optically transparent materials. Opt. Exp. 2010, 18, 24673–24678. [Google Scholar] [CrossRef] [PubMed]
- Block, E.; Greco, M.; Vitek, D.; Masihzadeh, O.; Ammar, D.A.; Kahook, M.Y.; Mandava, N.; Durfee, C.; Squier, J. Simultaneous spatial and temporal focusing for tissue ablation. Opt. Exp. 2013, 4, 831–841. [Google Scholar] [CrossRef] [PubMed]
- Bellouard, Y.; Said, A.; Dugan, M.; Bado, P. Fabrication of high-aspect ratio microfluidic channels and tunnels using femtosecond laser pulses and chemical etching. Opt. Exp. 2004, 12, 2120–2129. [Google Scholar] [CrossRef]
- Gottmann, J.; Hermans, M.; Ortmann, J. Digital Photonic Production of Micro Structures in Glass by In-Volume Selective Laser-Induced Etching using a High Speed Micro Scanner. J. Laser Micro/Nanoeng. 2013, 8, 531–541. [Google Scholar] [CrossRef]
- Wortmann, D.; Gottmann, J.; Brandt, N.; Horn-Solle, H. Micro- and nanostructures inside sapphire by fs-laser irradiation and selective etching. Opt. Exp. 2008, 16, 1517–1522. [Google Scholar] [CrossRef]
- Bellouard, Y.; Champion, A.; Lenssen, B.; Matteucci, M.; Schaap, A.; Beresna, M.; Corbari, C.; Gecevičius, M.; Kazansky, P.; Chappuis, O.; et al. The femtoprint project. J. Laser Micro/Nanoeng. 2012, 7, 1–10. [Google Scholar] [CrossRef]
- Kiyama, S.; Matsuo, S.; Hashimoto, S.; Morihira, Y. Examination of etching agent and etching mechanism on femtosecond laser microfabrication of channels inside vitreous silica substrates. J. Phys. Chem. C 2009, 113, 11560. [Google Scholar] [CrossRef]
- Marcinkevičius, A.; Juodkazis, S.; Watanabe, M.; Miwa, M.; Matsuo, S.; Misawa, H.; Nishii, J. Femtosecond laser-assisted three-dimensional microfabrication in silica. Opt. Lett. 2001, 26, 277–279. [Google Scholar] [CrossRef]
- Izawa, Y.; Tanaka, S.; Kikuchi, H.; Tsurumi, Y. Debris-free in-air laser dicing for multi-layer MEMS by perforated internal transformation and thermally-induced crack propagation. In Proceedings of the IEEE International Micro Electro Mechanical Systems Symposium, Tucson, AZ, USA, 13–17 January 2006; pp. 822–827.
- Ohmura, E.; Fukuyo, F.; Fukumitsu, K.; Morita, H. Internal modified-layer formation mechanism into silicon with nanosecond laser. J. Achiev. Mater. Manuf. Eng. 2006, 17, 381–384. [Google Scholar]
- Banks, D.P.; Kaur, K.S.; Grivas, Ch.; Sones, C.; Gan-gopadhyay, P.; Ying, C.; Mills, J.; Mailis, S.; Zergioti, I.; Fardel, R.; et al. Femtosecond laser-induced forward transfer for the deposition of nanoscale transparent and solid-phase materials. In Proceedings of the LAMP International Laser Advanced Materials Processing Symposium, Kobe, Japan, 29 June–2 July 2009; pp. 206–214.
- Zakharova, O.V.; Godymchuk, A.Y.; Gusev, A.A.; Gulchenko, S.I.; Vasyukova, I.A.; Kuznetsov, D.V. Considerable Variation of Antibacterial Activity of Cu Nanoparticles Suspensions Depending on the Storage Time, Dispersive Medium, and Particle Sizes. Biomed. Res. Int. 2015, 2015, 1–11. [Google Scholar] [CrossRef] [PubMed]
- Karlsson, H.L.; Gustafsson, J.; Cronholm, P.; Moller, L. Copper oxide nanoparticles are highly toxic: A comparison between metal oxide nanoparticles and carbon nanotubes. Chem. Res. Toxicol. 2008, 21, 1726–1732. [Google Scholar] [CrossRef] [PubMed]
- Martinez-Castanon, G.A.; Nino-Martinez, N.; Martinez-Gutierrez, F.; Martinez-Mendoza, J.R.; Ruiz, F. Synthesis and antibacterial activity of silver nanoparticles with different sizes. J. Nanopart. Res. 2008, 10, 1343–1348. [Google Scholar] [CrossRef]
- Hwang, D.J.; Choi, T.Y.; Grigoropoulos, C.P. Liquid-assisted femtosecond laser drilling of straight and three-dimensional microchannels in glass. Appl. Phys. A 2004, 79, 605–612. [Google Scholar] [CrossRef]
- Butkus, S.; Paipulas, D.; Kaškelytė, D.; Gaižauskas, E.; Sirutkaitis, V. Improvement of Cut Quality in Rapid-Cutting of Glass Method via Femtosecond Laser Filamentation. J. Laser Micro/Nanoeng. 2015, 10, 59–63. [Google Scholar] [CrossRef]
- Wang, S.Y.; Ren, Y.; Cheng, C.W.; Chen, J.K.; Tzou, D.Y. Micromachining of Copper by femtosecond laser pulses. Appl. Surf. Sci. 2013, 265, 302–308. [Google Scholar] [CrossRef]
- Nikumb, S.; Chen, Q.; Li, C.; Reshef, H.; Zheng, H.Y.; Qiu, H.; Low, D. Precision glass machining, drilling and profile cutting by short pulse lasers. Thin Solid Films 2005, 477, 216–221. [Google Scholar] [CrossRef]
- Laakso, P.; Penttila, R.; Heimala, P. Effect of Shot Number on Femtosecond Laser Drilling of Silicon. J. Laser Micro/Nanoeng. 2010, 5, 273–276. [Google Scholar] [CrossRef]
- Ahmmed, K.M.; Grambow, C.; Kietzig, A. Fabrication of Micro/Nano Structures on Metals by Femtosecond Laser Micromachining. Micromachines 2014, 5, 1219–1253. [Google Scholar] [CrossRef]
- Butkus, S.; Gaižauskas, E.; Paipulas, D.; Viburys, Ž.; Kaškelytė, D.; Barkauskas, M.; Alesenkov, A.; Sirutkaitis, V. Rapid microfabrication of transparent materials using filamented femtosecond laser pulses. Appl. Phys. A 2014, 114, 81–90. [Google Scholar] [CrossRef]
- Butkus, S.; Paipulas, D.; Sirutkaitis, R.; Gaižauskas, E.; Sirutkaitis, V. Rapid Cutting and Drilling of Transparent Materials via Femtosecond Laser Filamentation. J. Laser Micro/Nanoeng. 2014, 9, 212–220. [Google Scholar] [CrossRef]
- Zhu, S.; Lu, Y.F.; Hong, M.H. Laser ablation of solid substrate in a water-confined environment. Appl. Phys. Lett. 2001, 79, 1396–1398. [Google Scholar] [CrossRef]
- Ohl, C.; Arora, M.; Dijkink, R.; Janve, V.; Lohse, D. Surface cleaning from laser-induced cavitation bubbles. Appl. Phys. Lett. 2006, 89, 074102. [Google Scholar] [CrossRef]
- Montgomery, D.C. Design and Analysis of Experiments, 1st ed.; Wiley, John & Sons: Hoboken, NJ, USA, 2002. [Google Scholar]
- Gaižauskas, E.; Dubietis, A.; Kudriašov, V.; Sirutkaitis, V.; Couairon, A.; Faccio, D.; di Trapani, P. On the role of conical waves in self-focusing and filamentation of femtosecond pulses. Top. Appl. Phys. 2009, 114, 457–479. [Google Scholar]
- Keldysh, L.V. Ionization in the field of a strong electromagnetic wave. Sov. Phys. JETP 1965, 20, 1307–1314. [Google Scholar]
- Couairon, A.; Gaižauskas, E.; Faccio, D.; Dubietis, A.; Di Trapani, P. Nonlinear X-wave formation by femtosecond filamentation in Kerr media. Phys. Rev. E 2006, 73, 016608. [Google Scholar] [CrossRef] [PubMed]
- Majus, D.; Jukna, V.; Valiulis, G.; Dubietis, A. Generation of periodic filament arrays by self-focusing of highly elliptical ultrashort pulsed laser beams. Phys. Rev. A 2009, 79, 033843. [Google Scholar] [CrossRef]
- Kudriašov, V.; Gaižauskas, E.; Sirutkaitis, V. Beam transformation and permanent modification in fused silica induced by femtosecond filaments. J. Opt. Soc. Am. B 2005, 22, 2619–2627. [Google Scholar] [CrossRef]
- Jeffwu, C.F.; Hamada, M.S. Planning, Analysis, and Optimization, 1st ed.; Wiley, John & Sons: Hoboken, NJ, USA, 2009. [Google Scholar]
© 2015 by the authors; licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons by Attribution (CC-BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Butkus, S.; Alesenkov, A.; Paipulas, D.; Gaižauskas, E.; Melninkaitis, A.; Kaškelytė, D.; Barkauskas, M.; Sirutkaitis, V. Analysis of the Micromachining Process of Dielectric and Metallic Substrates Immersed in Water with Femtosecond Pulses. Micromachines 2015, 6, 2010-2022. https://doi.org/10.3390/mi6121471
Butkus S, Alesenkov A, Paipulas D, Gaižauskas E, Melninkaitis A, Kaškelytė D, Barkauskas M, Sirutkaitis V. Analysis of the Micromachining Process of Dielectric and Metallic Substrates Immersed in Water with Femtosecond Pulses. Micromachines. 2015; 6(12):2010-2022. https://doi.org/10.3390/mi6121471
Chicago/Turabian StyleButkus, Simas, Aleksandr Alesenkov, Domas Paipulas, Eugenijus Gaižauskas, Andrius Melninkaitis, Dalia Kaškelytė, Martynas Barkauskas, and Valdas Sirutkaitis. 2015. "Analysis of the Micromachining Process of Dielectric and Metallic Substrates Immersed in Water with Femtosecond Pulses" Micromachines 6, no. 12: 2010-2022. https://doi.org/10.3390/mi6121471
APA StyleButkus, S., Alesenkov, A., Paipulas, D., Gaižauskas, E., Melninkaitis, A., Kaškelytė, D., Barkauskas, M., & Sirutkaitis, V. (2015). Analysis of the Micromachining Process of Dielectric and Metallic Substrates Immersed in Water with Femtosecond Pulses. Micromachines, 6(12), 2010-2022. https://doi.org/10.3390/mi6121471