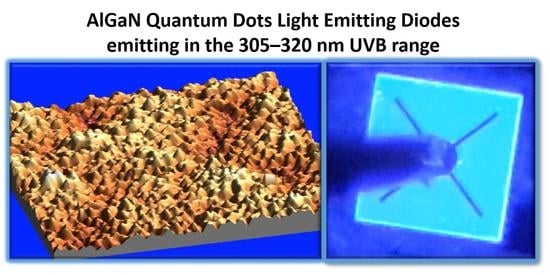
UVB LEDs Grown by Molecular Beam Epitaxy Using AlGaN Quantum Dots
Abstract
:1. Introduction
2. Materials and Methods
3. Results
3.1. Al0.2Ga0.8N QDs Structural and Optical Properties
3.2. QD-Based LEDs Characteristics
3.2.1. Photoluminescence
3.2.2. Electrical Properties
3.2.3. Electroluminescence
3.2.4. Optical Power and External Quantum Efficiency
4. Discussion
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Mukai, T.; Morita, D.; Nakamura, S. High-power UV InGaN/AlGaN double-heterostructure LEDs. J. Cryst. Growth 1998, 189/190, 778–781. [Google Scholar] [CrossRef]
- Taniyasu, Y.; Kasu, M.; Makimoto, T. An aluminium nitride light-emitting diode with a wavelength of 210 nanometres. Nature 2006, 441, 325–328. [Google Scholar] [CrossRef] [PubMed]
- Minamata Convention on Mercury. Available online: http://www.mercuryconvention.org/ (accessed on 30 August 2019).
- Kneissl, M. A Brief Review of III-Nitride UV Emitter Technologies and Their Applications. In III-Nitride Ultraviolet Emitters; Kneissl, M., Rass, J., Eds.; Series in Materials Science; Springer International Publishing: Cham, Switzerland, 2016; Volume 227, pp. 1–25. [Google Scholar]
- Santos, A.L.; Oliveira, V.; Baptista, I.; Henriques, I.; Gomes, N.C.M.; Almeida, A.; Correia, A.; Cunha, A. Wavelength dependence of biological damage induced by UV radiation on bacteria. Arch. Microbiol. 2013, 195, 63–74. [Google Scholar] [CrossRef] [PubMed]
- Ajmal Khan, M.; Maeda, N.; Jo, M.; Akamatsu, Y.; Tanabe, R.; Yamada, Y.; Hirayama, H. 13 mW operation of a 295–310 nm AlGaN UV-B LED with a p-AlGaN transparent contact layer for real world applications. J. Mater. Chem. C 2019, 7, 143–152. [Google Scholar] [CrossRef] [Green Version]
- Ajmal Khan, M.; Matsuura, E.; Kashima, Y.; Hirayama, H. Overcoming the current injection issue in the 310 nm band AlGaN UVB light-emitting diode. Jpn. J. Appl. Phys. 2020, 59, SAAD01. [Google Scholar] [CrossRef] [Green Version]
- Ding, K.; Avrutin, V.; Özgür, Ü.; Morkoç, H. Status of Growth of Group III-Nitride Heterostructures for Deep Ultraviolet Light-Emitting Diodes. Crystals 2017, 7, 300. [Google Scholar] [CrossRef] [Green Version]
- Nitta, S.; Yukawa, Y.; Watanabe, Y.; Kamiyama, S.; Amano, H.; Akasaki, I. Mass Transport of AlxGa1—xN. Phys. Status Solidi (a) 2002, 194, 485–488. [Google Scholar] [CrossRef]
- Kneissl, M.; Seong, T.-Y.; Han, J.; Amano, H. The emergence and prospects of deep-ultraviolet light-emitting diode technologies. Nat. Photonics 2019, 13, 233–244. [Google Scholar] [CrossRef]
- Kohno, T.; Sudo, Y.; Yamauchi, M.; Mitsui, K.; Kudo, H.; Okagawa, H.; Yamada, Y. Internal Quantum Efficiency and Nonradiative Recombination Rate in InGaN-Based Near-Ultraviolet Light-Emitting Diodes. Jpn. J. Appl. Phys. 2012, 51, 072102. [Google Scholar] [CrossRef]
- Imura, M.; Nakano, K.; Fujimoto, N.; Okada, N.; Balakrishnan, K.; Iwaya, M.; Kamiyama, S.; Amano, H.; Akasaki, I.; Noro, T.; et al. High-Temperature Metal-Organic Vapor Phase Epitaxial Growth of AlN on Sapphire by Multi Transition Growth Mode Method Varying V/III Ratio. Jpn. J. Appl. Phys. 2006, 45, 8639–8643. [Google Scholar] [CrossRef]
- Miyake, H.; Lin, C.H.; Tokoro, K.; Hiramatsu, K. Preparation of high-quality AlN on sapphire by high-temperature face-to-face annealing. J. Cryst. Growth 2016, 456, 155–159. [Google Scholar] [CrossRef] [Green Version]
- Hirayama, H. 231–261 nm AlGaN deep-ultraviolet light-emitting diodes fabricated on AlN multilayer buffers grown by ammonia pulse-flow method on sapphire. Appl. Phys. Lett. 2007, 91, 071901. [Google Scholar] [CrossRef]
- Kneissl, M.; Kolbe, T.; Chua, C.; Kueller, V.; Lobo, N.; Stellmach, J.; Knauer, A.; Rodriguez, H.; Einfeldt, S.; Yang, Z.; et al. Advances in group III-nitride-based deep UV light-emitting diode technology. Semicond. Sci. Technol. 2011, 26, 014036. [Google Scholar] [CrossRef]
- Liao, Y.; Thomidis, C.; Kao, C.-k.; Moustakas, T.D. AlGaN based deep ultraviolet light emitting diodes with high internal quantum efficiency grown by molecular beam epitaxy. Appl. Phys. Lett. 2011, 98, 081110. [Google Scholar] [CrossRef] [Green Version]
- Tanaka, S.; Lee, J.-S.; Ramvall, P.; Okagawa, H. A UV Light-Emitting Diode Incorporating GaN Quantum Dots. Jpn. J. Appl. Phys. 2003, 42, L885. [Google Scholar] [CrossRef]
- Hirayama, H.; Fujikawa, S. Quaternary InAlGaN quantum-dot ultraviolet light-emitting diode emitting at 335 nm fabricated by anti-surfactant method. Phys. Status Solidi (C) 2008, 5, 2312–2315. [Google Scholar] [CrossRef]
- Brault, J.; Damilano, B.; Kahouli, A.; Chenot, S.; Leroux, M.; Vinter, B.; Massies, J. Ultra-violet GaN/Al0.5Ga0.5N quantum dot based light emitting diodes. J. Cryst. Growth 2013, 363, 282–286. [Google Scholar] [CrossRef]
- Verma, J.; Kandaswamy, P.K.; Protasenko, V.; Verma, A.; Grace, X.H.; Jena, D. Tunnel-injection GaN quantum dot ultraviolet light-emitting diodes. Appl. Phys. Lett. 2013, 102, 041103. [Google Scholar] [CrossRef] [Green Version]
- Huault, T.; Brault, J.; Natali, F.; Damilano, B.; Lefebvre, D.; Nguyen, L.; Leroux, M.; Massies, J. Blue-light emission from GaN/Al0.5Ga0.5N quantum dots. Appl. Phys. Lett. 2008, 92, 051911. [Google Scholar] [CrossRef]
- Brault, J.; Rosales, D.; Damilano, B.; Leroux, M.; Courville, A.; Korytov, M.; Chenot, S.; Vennéguès, P.; Vinter, B.; DeMierry, P.; et al. Polar and semipolar GaN/Al0.5Ga0.5Nnanostructures for UV light emitters. Semicond. Sci. Technol. 2014, 29, 084001. [Google Scholar] [CrossRef]
- Himwas, C.; den Hertog, M.; Bellet-Amalric, E.; Songmuang, R.; Donatini, F.; Dang, L.S.; Monroy, E. Enhanced room-temperature mid-ultraviolet emission from AlGaN/AlN Stranski-Krastanov quantum dots. J. Appl. Phys. 2014, 116, 023502. [Google Scholar] [CrossRef]
- Matta, S.; Brault, J.; Ngo, T.-H.; Damilano, B.; Leroux, M.; Massies, J.; Gil, B. Photoluminescence properties of (Al,Ga)N nanostructures grown on Al0.5Ga0.5N (0001). Superlattices Microstruct. 2018, 114, 161–168. [Google Scholar] [CrossRef]
- Brault, J.; Huault, T.; Natali, F.; Damilano, B.; Lefebvre, D.; Leroux, M.; Korytov, M.; Massies, J. Tailoring the shape of GaN/AlxGa1−xN nanostructures to extend their luminescence in the visible range. J. Appl. Phys. 2009, 105, 033519. [Google Scholar] [CrossRef]
- Matta, S.; Brault, J.; Ngo, T.H.; Damilano, B.; Korytov, M.; Vennéguès, P.; Nemoz, M.; Massies, J.; Leroux, M.; Gil, B. Influence of the heterostructure design on the optical properties of GaN and Al0.1Ga0.9N quantum dots for ultraviolet emission. J. Appl. Phys. 2017, 122, 085706. [Google Scholar] [CrossRef]
- Himwas, C.; Songmuang, R.; Dang, L.S.; Bleuse, J.; Rapenne, L.; Sarigiannidou, E.; Monroy, E. Thermal stability of the deep ultraviolet emission from AlGaN/AlN Stranski-Krastanov quantum dots. Appl. Phys. Lett. 2012, 101, 241914. [Google Scholar] [CrossRef]
- Brault, J.; Matta, S.; Ngo, T.-H.; Al Khalfioui, M.; Valvin, P.; Leroux, M.; Damilano, B.; Korytov, M.; Brändli, V.; Vennéguès, P.; et al. Internal quantum efficiencies of AlGaN quantum dots grown by molecular beam epitaxy and emitting in the UVA to UVC ranges. J. Appl. Phys. 2019, 126, 205701. [Google Scholar] [CrossRef]
- Damilano, B.; Brault, J.; Massies, J. Formation of GaN quantum dots by molecular beam epitaxy using NH3 as nitrogen source. J. Appl. Phys. 2015, 118, 024304. [Google Scholar] [CrossRef]
- Daudin, B.; Widmann, F.; Feuillet, G.; Samson, Y.; Arlery, M.; Rouvière, J.L. Stranski-Krastanov growth mode during the molecular beam epitaxy of highly strained GaN. Phys. Rev. B 1997, 56, R7069–R7072. [Google Scholar] [CrossRef]
- Matta, S.; Brault, J.; Korytov, M.; Phuong Vuong, T.Q.; Chaix, C.; Al Khalfioui, M.; Vennéguès, P.; Massies, J.; Gil, B. Properties of AlN layers grown on c-sapphire substrate using ammonia assisted MBE. J. Cryst. Growth 2018, 499, 40–46. [Google Scholar] [CrossRef]
- Nemoz, M.; Dagher, R.; Matta, S.; Michon, A.; Vennéguès, P.; Brault, J. Dislocation densities reduction in MBE-grown AlN thin films by high temperature annealing. J. Cryst. Growth 2017, 461, 10–15. [Google Scholar] [CrossRef]
- Brault, J.; Al Khalfioui, M.; Matta, S.; Damilano, B.; Leroux, M.; Chenot, S.; Korytov, M.; Nkeck, J.E.; Vennéguès, P.; Duboz, J.Y.; et al. UVA and UVB light emitting diodes with AlyGa1-yN quantum dot active regions covering the 305 nm–335 nm range. Semicond. Sci. Technol. 2018, 33, 075007. [Google Scholar]
- Brault, J.; Matta, S.; Ngo, T.H.; Korytov, M.; Rosales, D.; Damilano, B.; Leroux, M.; Vennéguès, P.; Al Khalfioui, M.; Courville, A.; et al. Investigation of AlyGa1-yN/Al0.5Ga0.5N Quantum Dot Properties for the Design of Ultraviolet Emitters. Jpn. J. Appl. Phys. 2016, 55, 05FG06. [Google Scholar] [CrossRef]
- Iwata, Y.; Banal, R.G.; Ichikawa, S.; Funato, M.; Kawakami, Y. Emission mechanisms in Al-rich AlGaN/AlN quantum wells assessed by excitation power dependent photoluminescence spectroscopy. J. Appl. Phys. 2015, 117, 075701. [Google Scholar] [CrossRef]
- Nepal, N.; Nakarmi, M.L.; Lin, J.Y.; Jiang, H.X. Photoluminescence studies of impurity transitions in AlGaN alloys. Appl. Phys. Lett. 2006, 89, 092107. [Google Scholar] [CrossRef] [Green Version]
- Liang, Y.-H.; Towe, E. Progress in efficient doping of high aluminum-containing group III-nitrides. Appl. Phys. Rev. 2018, 5, 011107. [Google Scholar] [CrossRef]
- Piprek, J. How to decide between competing efficiency droop models for GaN-based light-emitting diodes. Appl. Phys. Lett. 2015, 107, 031101. [Google Scholar] [CrossRef]
- Leroux, M.; Brault, J.; Kahouli, A.; Elmaghraoui, D.; Damilano, B.; de Mierry, P.; Korytov, M.; Kim, J.-H.; Cho, Y.-H. Stark effect in ensembles of polar (0001) Al0.5Ga0.5N/GaN quantum dots and comparison with semipolar (11-22) ones. J. Appl. Phys. 2014, 116, 034308. [Google Scholar] [CrossRef]
- Bretagnon, T.; Kalliakos, S.; Lefebvre, P.; Valvin, P.; Gil, B.; Grandjean, N.; Dussaigne, A.; Damilano, B.; Massies, J. Time dependence of the photoluminescence of GaN/AlN quantum dots under high photoexcitation. Phys. Rev. B 2003, 68, 205301. [Google Scholar] [CrossRef]
- Ji, L.-W.; Su, Y.K.; Chang, S.J.; Chang, C.S.; Wu, L.W.; Lai, W.C.; Du, X.L.; Chen, H. InGaN/GaN multi-quantum dot light-emitting diodes. J. Cryst. Growth 2004, 263, 114–118. [Google Scholar] [CrossRef]
- Brault, J.; Damilano, B.; Vinter, B.; Vennéguès, P.; Leroux, M.; Kahouli, A.; Massies, J. AlGaN-Based Light Emitting Diodes Using Self-Assembled GaN Quantum Dots for Ultraviolet Emission. Jpn. J. Appl. Phys. 2013, 52, 08JG01. [Google Scholar] [CrossRef]
- Park, I.-K.; Kwon, M.-K.; Seo, S.-B.; Kim, J.-Y.; Lim, J.-H.; Park, S.-J. Ultraviolet light-emitting diodes with self-assembled InGaN quantum dots. Appl. Phys. Lett. 2007, 90, 111116. [Google Scholar] [CrossRef] [Green Version]
- Kahouli, A.; Kriouche, N.; Brault, J.; Damilano, B.; Vennéguès, P.; de Mierry, P.; Leroux, M.; Courville, A.; Tottereau, O.; Massies, J. GaN/Al0.5Ga0.5N (11-22) semipolar nanostructures: A way to get high luminescence efficiency in the near ultraviolet range. J. Appl. Phys. 2011, 110, 084318. [Google Scholar] [CrossRef]
- Cao, X.A.; Topol, K.; Shahedipour-Sandvik, F.; Teetsov, J.; Sandvik, P.M.; LeBoeuf, S.F.; Ebong, A.; Kretchmer, J.; Stokes, E.B.; Arthur, S.; et al. Influence of defects on electrical and optical characteristics of GaN/InGaN-based light-emitting diodes. Proc. SPIE 2002, 4776, 105–113. [Google Scholar]
- Zhang, Y.; Krishnamoorthy, S.; Akyol, F.; Bajaj, S.; Allerman, A.A.; Moseley, M.W.; Armstrong, A.M.; Rajan, S. Tunnel-injected sub-260 nm ultraviolet light emitting diodes. Appl. Phys. Lett. 2017, 110, 201102. [Google Scholar] [CrossRef] [Green Version]
- Fan Arcara, V.; Damilano, B.; Feuillet, G.; Vézian, S.; Ayadi, K.; Chenot, S.; Duboz, J.-Y. Ge doped GaN and Al05Ga0.5N-based tunnel junctions on top of visible and UV light emitting diodes. J. Appl. Phys. 2019, 126, 224503. [Google Scholar] [CrossRef]









Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Brault, J.; Khalfioui, M.A.; Matta, S.; Ngo, T.H.; Chenot, S.; Leroux, M.; Valvin, P.; Gil, B. UVB LEDs Grown by Molecular Beam Epitaxy Using AlGaN Quantum Dots. Crystals 2020, 10, 1097. https://doi.org/10.3390/cryst10121097
Brault J, Khalfioui MA, Matta S, Ngo TH, Chenot S, Leroux M, Valvin P, Gil B. UVB LEDs Grown by Molecular Beam Epitaxy Using AlGaN Quantum Dots. Crystals. 2020; 10(12):1097. https://doi.org/10.3390/cryst10121097
Chicago/Turabian StyleBrault, Julien, Mohamed Al Khalfioui, Samuel Matta, Thi Huong Ngo, Sébastien Chenot, Mathieu Leroux, Pierre Valvin, and Bernard Gil. 2020. "UVB LEDs Grown by Molecular Beam Epitaxy Using AlGaN Quantum Dots" Crystals 10, no. 12: 1097. https://doi.org/10.3390/cryst10121097
APA StyleBrault, J., Khalfioui, M. A., Matta, S., Ngo, T. H., Chenot, S., Leroux, M., Valvin, P., & Gil, B. (2020). UVB LEDs Grown by Molecular Beam Epitaxy Using AlGaN Quantum Dots. Crystals, 10(12), 1097. https://doi.org/10.3390/cryst10121097