Ageing and Langmuir Behavior of the Cage Occupancy in the Nitrogen Gas Hydrate
Abstract
:1. Introduction
2. Materials and Methods
2.1. Sample Preparation
2.2. Neutron Diffraction
2.3. Raman Spectra
3. Results and Discussion
3.1. Coupling Neutron Diffraction and Raman Scattering to Access Nitrogen Gas Hydrate Signatures
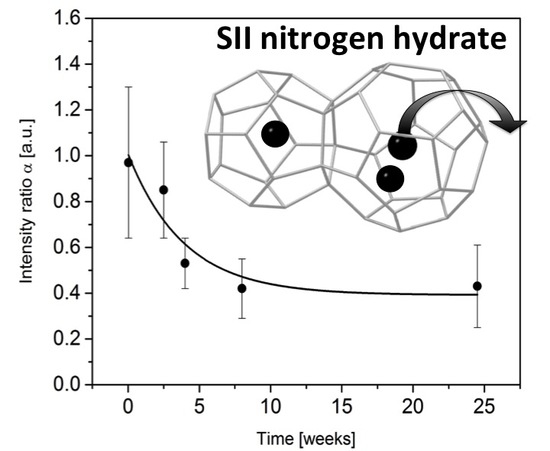
3.2. Monitoring the Ageing of the Nitrogen SII Hydrate
3.3. Langmuir Behavior of the Cage Occupancy in the SII Structure from Raman Scattering
- (i)
- the double occupancy of the LCs () impacts the high fugacity region of the ratio (above ca. 400 bar),
- (ii)
- the ratio decreases with the fugacity when (below ca. 400 bar),
- (iii)
- the ratio increases with the fugacity when (below ca. 400 bar).
4. Conclusions
Acknowledgments
Author Contributions
Conflicts of Interest
References
- Jeffrey, G.A. Hydrate inclusion compounds. In Comprehensive Supramolecular Chemistry; Atwood, J.L., Davies, J.E.D., Mac-Nicol, D.D., Vögtle, F., Eds.; Pergamon: Oxford, UK, 1996; Volume 6, pp. 757–788. [Google Scholar]
- Sloan, E.D.; Koh, C.A. Clathrate Hydrates of Natural Gases, 3rd ed.; Taylor & Francis-CRC Press: Boca Raton, FL, USA, 2008; ISBN 978-0-8493-9078-4. [Google Scholar]
- Von Stackelberg, M.; Muller, H.R. Feste Gashydrate II. Struktur und Raumchemie. Z. Electrochem. 1954, 58, 25–39. [Google Scholar]
- Broseta, D.; Ruffine, L.; Desmedt, A. (Eds.) Gas Hydrates 1: Fundamentals, Characterization and Modeling; Wiley-ISTE: London, UK, 2017. [Google Scholar]
- Ruffine, L.; Broseta, D.; Desmedt, A. (Eds.) Gas Hydrates 2: Geosciences and Applications; Wiley-ISTE: London, UK, 2018; under press. [Google Scholar]
- Koh, C.A.; Sloan, E.D.; Sum, A.K. Natural Gas Hydrates: Recent Advances and Challenges in Energy and Environmental Applications. AIChE J. 2007, 53, 1636–1643. [Google Scholar] [CrossRef]
- Yang, X.; Liu, H.; Li, Y. Research progress of separation technology based on hydrate formation. Huagong Xuebao/CIESC J. 2017, 68, 831–840. [Google Scholar]
- Handa, Y.P.; Ratcliffe, C.I.; Ripmeester, J.A.; Tse, J.S. Structural transition in mixed hydrates of xenon and krypton as a function of gas composition. J. Phys. Chem. 1990, 94, 4363–4365. [Google Scholar] [CrossRef]
- Yoon, J.-H.; Kawamura, T.; Ohtake, M.; Takeya, S.; Komai, T.; Yamamoto, Y.; Emi, H.; Kohara, M.; Tanaka, S.; Takano, O.; et al. Highly Selective Encaging of Carbon Dioxide Meolecules in the Mixed Carbon Dioxide and Nitrogen Hydrate at Low Temperatures. J. Phys. Chem. B 2006, 110, 17595–17599. [Google Scholar] [CrossRef] [PubMed]
- English, N.J.; Tse, J.S.; Carey, D. Mechanism for thermal conduction in various polymorphs of methane hydrate. Phys. Rev. B 2009, 80, 134306. [Google Scholar] [CrossRef]
- Veluswamy, G.P.; Kumar, R.; Linga, P. Hydrogen storage in clathrate hydrates: Current state of the art and future directions. Appl. Energy 2014, 122, 112–132. [Google Scholar] [CrossRef]
- Dyadin, Y.A.; Larionov, E.G.; Aladko, E.Y.; Manakov, A.Y.; Zhurko, F.V.; Mikina, T.V.; Komarov, V.Y.; Grachev, E.V. Clathrate formation in water-noble gas (hydrogen) systems at high pressures. J. Struct. Chem. 1999, 40, 790–795. [Google Scholar] [CrossRef]
- Mao, W.L.; Mao, H.K.; Goncharov, A.F.; Struzhkin, V.V.; Guo, Q.Z.; Hu, J.Z.; Shu, J.F.; Hemley, R.J.; Somayazulu, M.; Zhao, Y.S. Hydrogen clusters in clathrate hydrate. Science 2002, 297, 2247–2249. [Google Scholar] [CrossRef] [PubMed]
- Patchkovskii, S.; Tse, J.S. Thermodynamic stability of hydrogen clathrates. Proc. Natl. Acad. Sci. USA 2003, 100, 14645–14650. [Google Scholar] [CrossRef] [PubMed]
- Florusse, L.J.; Peters, C.J.; Schoonman, J.; Hester, H.C.; Koh, C.A.; Dec, S.F.; Marsh, K.N.; Sloan, E.D. Stable Low-PressureHydrogen Cluster Stored in binary Clathrate Hydrate. Science 2004, 306, 469–471. [Google Scholar] [CrossRef] [PubMed]
- Lee, H.; Lee, J.-W.; Kim, Y.D.; Park, J.; Seo, Y.T.; Zeng, H.; Moudrakovski, I.L.; Ratcliffe, C.I.; Ripmeester, J.A. Tuning clathrate hydrates for hydrogen storage. Nature 2005, 434, 743–746. [Google Scholar] [CrossRef] [PubMed]
- Pefoute, E.; Kemner, E.; Soetens, J.C.; Russina, M.; Desmedt, A. Diffusive motion of molecular hydrogen confined in THF clathrate hydrate. J. Phys. Chem. C 2012, 116, 16823–16829. [Google Scholar] [CrossRef]
- Ross, R.G.; Andersson, P.; Backstrom, G. Unusual PT dependence of thermal conductivity for a clathrate hydrate. Nature 1981, 290, 322–323. [Google Scholar] [CrossRef]
- Tse, J.S.; Klug, D.D.; Zhao, J.Y.; Sturhahn, W.; Alp, E.E.; Baumert, J.; Gutt, C.; Johnson, M.R.; Press, W. Anharmonic motions of Kr in the clathrate hydrate. Nat. Mater. 2005, 4, 917–921. [Google Scholar] [CrossRef] [PubMed]
- Englezos, P.; Lee, J.D. Gas Hydrates: A Cleaner Source of Energy and Opportunity for Innovative Technologies. Korean J. Chem. Eng. 2005, 22, 671–681. [Google Scholar] [CrossRef]
- Krivchikov, A.I.; Romantsova, O.O.; Korolyuk, O.A. The effect of proton ordering on the thermal conductivity of clathrate tetrahydrofuran hydrate. Low Temp. Phys. 2008, 34, 648–654. [Google Scholar] [CrossRef]
- Mootz, D.; Stabel, D. First examples of type I clathrate hydrates of strong acids: Polyhydrates of hexafluorophosphoric, tetrafluoroboric, and perchloric acid. J. Am. Chem. Soc. 1994, 116, 4141–4142. [Google Scholar] [CrossRef]
- Chapoy, A.; Anderson, R.; Tohidi, B. Low-pressure molecular hydrogen storage in semi-clathrate hydrates of quaternary ammonium compounds. J. Am. Chem. Soc. 2007, 129, 746–747. [Google Scholar] [CrossRef] [PubMed]
- Cha, J.H.; Shin, K.; Choi, S.; Lee, S.; Lee, H. Maximized proton conductivity of the HPF6 clathrate hydrate by structural transformation. J. Phys. Chem. C 2008, 112, 13332–13335. [Google Scholar] [CrossRef]
- Cha, J.H.; Shin, K.; Choi, S.; Lee, H. Ionic conductivity enhancement due to coguest inclusion in the pure ionic clathrate hydrates. J. Phys. Chem. C 2008, 112, 10573–10578. [Google Scholar] [CrossRef]
- Shin, K.; Cha, M.; Choi, S.; Dho, J.; Lee, H. Discrete Magnetic Patterns of Nonionic and Ionic Clathrate Hydrates. J. Am. Chem. Soc. 2008, 130, 17234–17235. [Google Scholar] [CrossRef] [PubMed]
- Shin, K.; Cha, J.O.; Seo, Y.; Lee, H. Physico-chemical properties of ionic clathrate hydrates. Chem. Asian J. 2010, 5, 22–34. [Google Scholar] [PubMed]
- Cha, J.H.; Lee, W.; Lee, H. Hydrogen gas sensor based on proton-conducting clathrate hydrate. Angew. Chem. Int. Ed. 2009, 48, 8687–8690. [Google Scholar] [CrossRef] [PubMed]
- Cha, M.; Shin, K.; Kwon, M.; Koh, D.Y.; Sung, B.; Lee, H. Superoxide ions entrapped in water cages of ionic clathrate hydrates. J. Am. Chem. Soc. 2010, 132, 3694–3696. [Google Scholar] [CrossRef] [PubMed]
- Desmedt, A.; Bedouret, L.; Pefoute, E.; Pouvreau, M.; Say-Liang-Fat, S.; Alvarez, M. Energy landscape of clathrate hydrates. Eur. Phys. J. Spec. Top. 2012, 213, 103–127. [Google Scholar] [CrossRef]
- Desmedt, A.; Lechner, R.E.; Lassegues, J.C.; Guillaume, F.; Cavagnat, D.; Grondin, J. Hydronium dynamics in the perchloric acid clathrate hydrate. Solid State Ion. 2013, 252, 19–25. [Google Scholar] [CrossRef]
- Bedouret, L.; Judeinstein, P.; Ollivier, J.; Combet, J.; Desmedt, A. Proton diffusion in the hexafluorophosphoric acid clathrate hydrate. J. Phys. Chem. B 2014, 118, 13357–13364. [Google Scholar] [CrossRef] [PubMed]
- Desmedt, A.; Martin-Gondre, L.; Nguyen, T.T.; Petuya, C.; Barandiaran, L.; Babot, O.; Toupance, T.; Grim, R.G.; Sum, A.K. Modifying the flexibility of water cages by co-including acidic species within clathrate hydrate. J. Phys. Chem. C 2015, 119, 8904–8911. [Google Scholar] [CrossRef]
- Broseta, D.; Torré, J.P.; Dicharry, C. Hydrate-based removal of CO2 from CH4 + CO2 gas streams. In Gas Hydrates 2: Geosiences and Applications; Ruffine, L., Broseta, D., Desmedt, A., Eds.; Wiley-ISTE: London, UK, 2018; Volume 2, under press. [Google Scholar]
- Qin, J.; Kuhs, W.F. Calibration of Raman Quantification Factors of Guest Molecules in Gas Hydrates and their Application to Gas Exchange Processes Involving N2. J. Chem. Eng. Data 2015, 60, 369–375. [Google Scholar] [CrossRef]
- Davidson, D.W.; Handa, Y.P.; Ratcliffe, C.I.; Tse, J.S. The Ability of Small Molecules to Form Clathrate Hydrates of Structure II. Nature 1984, 311, 142–143. [Google Scholar] [CrossRef]
- Lundgaard, L.; Mollerup, J. Calculation of Phase Diagrams of Gas Hydrates. Fluid Phase Equilib. 1992, 76, 141–149. [Google Scholar] [CrossRef]
- Van Hinsberg, M.G.E.; Scheerboom, M.I.M.; Schouten, J.A. The Vibrational Spectra of N2 in Clathrate Hydrates: A New High Pressure Phase Transition. J. Chem. Phys. 1993, 99, 752–754. [Google Scholar] [CrossRef]
- Kuhs, W.F.; Chazallon, B.; Radaelli, P.G.; Pauer, F. Cage Occupancy and Compressibility of Deuterated N2-Clathrate Hydrate by Neutron Diffraction. J. Incl. Phenom. Mol. Recognit. Chem. 1997, 29, 65–77. [Google Scholar] [CrossRef]
- Chazallon, B.; Kuhs, W.F. In situ Structural Properties of N2-, O2-, and Air-Clathrates by Neutron Diffraction. J. Chem. Phys. 2002, 117, 308–320. [Google Scholar] [CrossRef]
- Hansen, T.C.; Falenty, A.; Kuhs, W.F. Lattice Constants and Expansivity of Gas Hydrates from 10 K up to the Stability Limit. J. Chem. Phys. 2016, 144, 054301. [Google Scholar] [CrossRef] [PubMed]
- Van Hinsberg, M.G.E.; Schouten, J.A. The Phase Diagram of Nitrogen Clathrate Hydrate. AIP Proc. 1993, 309, 271–274. [Google Scholar]
- Pauer, F.; Kipfstuhl, J.; Kuhs, W.F. Raman Spectroscopic Study on the Nitrogen Ratio in Natural Ice Clathrates in the GRIP Ice Core. Geophys. Res. Lett. 1995, 22, 969–971. [Google Scholar] [CrossRef]
- Pauer, F.; Kipfstuhl, J.; Kuhs, W.F. Raman Spectroscopic Study on the Spatial Distribution of Nitrogen and Oxygen in Natural Ice Clathrates and their Decomposition to Air Bubbles. Geophys. Res. Lett. 1996, 23, 177–180. [Google Scholar] [CrossRef]
- Champagnon, B.; Panczer, G.; Chazallon, B.; Arnaud, L.; Duval, P.; Lipenkov, V. Nitrogen and Oxygen Guest Molecules in Clathrate Hydrates: Different Sites Revealed by Raman Spectroscopy. J. Raman Spectrosc. 1997, 28, 711–715. [Google Scholar] [CrossRef]
- Chazallon, B.; Champagnon, B.; Panczer, G.; Pauer, F.; Klapproth, A.; Kuhs, W.F. Micro-Raman Analysis of Synthetic Air Clathrates. Eur. J. Mineral. 1998, 10, 1125–1134. [Google Scholar] [CrossRef]
- Sasaki, S.; Hori, S.; Kume, T.; Shimizu, H. Microscopic Observation and In Situ Raman Scattering Studies on High-Pressure Phase Transformations of a Synthetic Nitrogen Hydrate. J. Chem. Phys. 2003, 118, 7892–7897. [Google Scholar] [CrossRef]
- Petuya, C.; Damay, F.; Chazallon, B.; Bruneel, J.-L.; Desmedt, A. Guest Partitioning and Metastability of the Nitrogen Gas Hydrate. J. Phys. Chem. C 2018, 122, 566–573. [Google Scholar] [CrossRef]
- Van Klaveren, E.P.; Michels, P.J.; Schouten, J.A.; Klug, D.D.; Tse, J.S. Stability of Doubly Occupied N2 Clathrate Hydrates Investigates by Molecular Dynamics Simulations. J. Chem. Phys. 2001, 114, 5745–5754. [Google Scholar] [CrossRef]
- Van Klaveren, E.P.; Michels, P.J.; Schouten, J.A.; Klug, D.D.; Tse, J.S. Molecular Dynamics Simulation Study of the Properties of Doubly Occupied N2 Clathrate Hydrates. J. Chem. Phys. 2001, 115, 10500–10508. [Google Scholar] [CrossRef]
- Klauda, J.B.; Sandler, S.I. Phase Behavior of Clathrate Hydrates: A Model for Single and Multiple Gas Component Hydrates. Chem. Eng. Sci. 2003, 58, 27–41. [Google Scholar] [CrossRef]
- Zhu, J.; Du, S.; Yu, X.; Zhang, J.; Xu, H.; Vogel, S.C.; Germann, T.C.; Francisco, J.S.; Izumi, F.; Momma, K.; et al. Encapsulation Kinetics and Dynamics of Carbon Monoxide in Clathrate Hydrate. Nat. Commun. 2014, 5, 4128. [Google Scholar] [CrossRef] [PubMed]
- Petuya, C.; Damay, F.; Talaga, D.; Desmedt, A. Guest Partitioning in Carbon Monoxide Hydrates by Raman Spectroscopy. J. Phys. Chem. C 2017, 121, 13798–13802. [Google Scholar] [CrossRef]
- Desmedt, A.; Bedouret, L.; Ollivier, J.; Petuya, C. Neutron Scattering of Clathrate and Semiclathrate Hydrates. In Gas Hydrates: Fundamentals, Characterization and Modeling; Broseta, D., Ruffine, L., Desmedt, A., Eds.; Wiley-ISTE: London, UK, 2017; Volume 1, pp. 1–62. [Google Scholar]
- Chazallon, B.; Noble, J.A.; Desmedt, A. Spectroscopy of Gas Hydrates: From Fundamental Aspects to Chemical Engineering, Geophysical and Astrophysical Applications. In Gas Hydrates: Fundamentals, Characterization and Modeling; Broseta, D., Ruffine, L., Desmedt, A., Eds.; Wiley-ISTE: London, UK, 2017; Volume 1, pp. 63–112. [Google Scholar]
- Le Bail, A. Accuracy in Powder Diffraction II: Proceedings of the International Conference May 26–29; Prince, E., Stalick, J.K., Eds.; NIST Special Publication, US Government Printing Office: Washington, DC, USA, 1992; Volume 846, pp. 142–153.
- Rodriguez-Carvajal, J. FULLPROF: A program for Rietveld refinement and pattern matching analysis. In Proceedings of the Satellite Meeting on Powder Diffraction of the XV Congress of the IUCr, Toulouse France, 16–19 July 1990; p. 127. [Google Scholar]
- Giannasi, A.; Celli, M.; Ulivi, L.; Zoppi, M. Low Temperature Raman Spectra of Hydrogen in Simple and Binary Clathrate Hydrates. J. Chem. Phys. 2008, 129, 084705. [Google Scholar] [CrossRef] [PubMed]
- Nakahara, J.; Shigesato, Y.; Higashi, A.; Hondoh, T.; Langway, C.C., Jr. Raman Spectra of Natural Clathrates in Deep Ice Cores. Philos. Mag. B 1988, 57, 421–430. [Google Scholar] [CrossRef]
- Sugahara, K.; Tanaka, Y.; Sugahara, T.; Ohgaki, K. Thermodynamic Stability and Structure of Nitrogen Hydrate Crystal. J. Supramol. Chem. 2002, 2, 365–368. [Google Scholar] [CrossRef]
- Klobes, B.; Desmedt, A.; Sergueev, I.; Schmalzl, K.; Hermann, R.P. 129Xe nuclear resonance scattering on solid Xe and 129Xe clathrate hydrate. EPL Europhys. Lett. 2013, 103, 36001. [Google Scholar] [CrossRef]
- Sugahara, K.; Sugahara, T.; Ohgaki, K. Thermodynamic and Raman Spectroscopic Studies of Xe and Kr Hydrates. J. Chem. Eng. Data 2005, 50, 274–277. [Google Scholar] [CrossRef]
- Sum, A.K.; Buruss, R.C.; Sloan, E.D. Measurement of Clathrate Hydrates via Raman Spectroscopy. J. Phys. Chem. B 1997, 101, 7371–7377. [Google Scholar] [CrossRef]
- Subramanian, S.; Sloan, E.D. Trends in Vibrational Frequencies of Guest Trapped in Clathrate Hydrate Cages. J. Phys. Chem. B 2002, 106, 4348–4355. [Google Scholar] [CrossRef]
- Schober, H.; Itoh, H.; Klapproth, A.; Chihaia, V.; Kuhs, W.F. Guest-host Coupling and Anharmonocity in Clathrate Hydrates. Eur. Phys. J. E 2003, 12, 41–49. [Google Scholar] [CrossRef] [PubMed]
- Van der Waals, J.H.; Platteeuw, J.C. Advances in Chemical Physics; Interscience: New York, NY, USA, 1959; Volume 2, pp. 1–57. [Google Scholar]
- Angus, S.; de Reuck, K.M.; Armstrong, B. International Thermodynamic Tables of the Fluid State 6-Nitrogen; Pergamon: Oxford, UK, 1977. [Google Scholar]
- Munck, J.; Skjold-Jorgensen, S.; Rasmussen, P. Phase Behaviour and Structural Aspects of ternary clathrate hydrate systems. The role of additives Computations of the formation of gas hydrates. Chem. Eng. Sci. 1988, 43, 2661–2672. [Google Scholar] [CrossRef]
- Parrish, W.R.; Prausnitz, J.M. Dissociation Pressures of Gas Hydrates Formed by Gas Mixture. Ind. Eng. Chem. Process Des. Dev. 1972, 11, 26–35. [Google Scholar] [CrossRef]
- Van Cleeff, A.; Diepen, G.A.M. Gas Hydrates of Nitrogen and Oxygen. Recueil des Travaux Chimiques des Pays-Bas 1960, 79, 582–586. [Google Scholar] [CrossRef]
- Marshall, D.R.; Saito, S.; Kobayashi, R. Hydrates at high pressures: Part I. Methane-Water, Argon-Water, and Nitrogen-Water Systems. AIChE J. 1964, 10, 202–205. [Google Scholar] [CrossRef]
- Jhaveri, J.; Robinson, D.B. Hydrates in the Methane-Nitrogen System. Can. J. Chem. Eng. 1965, 43, 75–78. [Google Scholar] [CrossRef]
- Asiaee, A.; Raeissi, S.; Shariati, A. Considering multiple occupancy of cavities in clathrate hydrate phase equilibrium calculations. J. Chem. Thermodyn. 2011, 43, 822–827. [Google Scholar] [CrossRef]
- Rasoolzadeh, A.; Shariati, A. Considering double occupancy of large cages in nitrogen and oxygen hydrates at high pressures. Fluid Phase Equilib. 2017, 434, 107–116. [Google Scholar] [CrossRef]
- Tsimpanogiannis, I.N.; Papadimitriou, N.I.; Stubos, A.K. On the limitation of the van der Waals-Platteeuw-based thermodynamic models for hydrates with multiple occupancy of cavities. Mol. Phys. 2012, 110, 1213–1221. [Google Scholar] [CrossRef]





© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Petuya, C.; Damay, F.; Desplanche, S.; Aupetit, C.; Desmedt, A. Ageing and Langmuir Behavior of the Cage Occupancy in the Nitrogen Gas Hydrate. Crystals 2018, 8, 145. https://doi.org/10.3390/cryst8040145
Petuya C, Damay F, Desplanche S, Aupetit C, Desmedt A. Ageing and Langmuir Behavior of the Cage Occupancy in the Nitrogen Gas Hydrate. Crystals. 2018; 8(4):145. https://doi.org/10.3390/cryst8040145
Chicago/Turabian StylePetuya, Claire, Françoise Damay, Sarah Desplanche, Christian Aupetit, and Arnaud Desmedt. 2018. "Ageing and Langmuir Behavior of the Cage Occupancy in the Nitrogen Gas Hydrate" Crystals 8, no. 4: 145. https://doi.org/10.3390/cryst8040145
APA StylePetuya, C., Damay, F., Desplanche, S., Aupetit, C., & Desmedt, A. (2018). Ageing and Langmuir Behavior of the Cage Occupancy in the Nitrogen Gas Hydrate. Crystals, 8(4), 145. https://doi.org/10.3390/cryst8040145